Application of the Cross Wavelet Transform to Solar Activity and Major Earthquakes Occurred in Chile ()
1. Introduction
Many terrestrial processes show trends and changes in the long-term: global temperature, geomagnetic activity and Earth’s rotation. It is a challenge to find a relationship between them and also to identify possible extraterrestrial factors that may contribute to these changes in the long-term.
The major source of energy near the Earth is the Sun, therefore it is natural to think about a connection between different elements of solar activity and terrestrial pro- cesses.
The aim of this work is to find statistical relationships between the number of sunspots and earthquakes in Chile.
Earthquakes distribution on the earth’s surface is not homogeneous; they generally occur in areas with different tectonic boundaries. One example is the largest earthquake of magnitude Ms = 9.5 which occurred in Valdivia on May 22, 1960 (the largest in the history of mankind), originated due to the sudden movement of the Nazca plate beneath the South American plate. The contact zone between the plates was located about 130 km from the coast to the west. Chile is one of the countries with the most seismic activity in the world. One destructive earthquake occurred every ten years in the last century [1] .
In previously published work, seismic periodicities in different countries were analyzed as in [2] [3] [4] .
Reference [11] found relationships between increased solar events during the last half of the twentieth century and the intensification of earthquake events. Reference [12] linked the maximum frequency of earthquakes during periods of moderate and high solar activity.
Statistical studies related to solar activity were previously published by [13] and [14] .
Reference [15] studied the transitions of solar dynamo and its link to rapid climate changes, presenting some of the solar-terrestrial variables as the causes of triggering seismic activity.
In this paper, surface seismic magnitude values (MS) for earthquakes in Chile and the yearly mean total sunspots number (SSN) were studied using:
・ Periodograms obtained by fast Fourier transform;
・ Cross Wavelet Transform and wavelet coherence analysis.
2. Data
The number of sunspots (SSN) is the index that measures the number and size of sunspots. Yearly mean total sunspot number is obtained by taking a simple arithmetic mean of the daily total sunspot number over all days of each year. The values were obtained by the World Data Center for the production, preservation and dissemination of the international sunspot number (WDC-SILSO, Royal Observatory of Belgium, Brussels) and are shown in Figure 1(a).
The Ms magnitude is the surface wave magnitude of earthquakes. It is a measure of seismic activity. The annual seismic data were obtained through the Servicio Sismológico from the Universidad de Chile (http://www.sismologia.cl/). Annual averages of earthquakes with Ms ≥ 7 for the period 1900-2015 were considered, see Figure 1(b).
3. Analysis of the Series
3.1. Sunspots Number and Surface Wave Magnitude of Earthquakes Periodograms Obtained by Fast Fourier Transform
To estimate the periodicities of MS and SSN, power spectra were performed. In Figure 2, power spectrum of MS series is presented in the terms of time. Predominant peaks around 4; 4.5; 5.5; 9; 11 and 13.5 are observed.
In Table 1 periodicities obtained by different authors are shown.
Figure 3 shows the power spectrum for SSN series. Two picks are observed in 10.5 and 20 years. These picks correspond to the ones cited by Schawabe and Hale respectively. Periodicity of 11 years was observed for the first time by Heinrich Schwave (1789-1875) and periodicity of 22 years takes the name of George Ellery Hale (1868-
![]()
Figure 1. (a) Number of sunspots (annual average) from 1900-2015. (b) Mayor Seismic moment of earthquakes in Chile (annual average) from 1900-2015.
![]()
Figure 2. Periodogram for Ms for the period 1900-2015.
![]()
Figure 3. Periodogram corresponding to SSN for period 1900-2015 (the maximum corresponds to 11 years).
![]()
Table 1. Periodicities for MS previously published.
1938) who discovered solar spots due to the action of the solar magnetic field limiting the sun radiation emission. In 1961, Harold Babcock and Horace Babcock observed that this magnetic field has two perpendicular components between them: the polar and toroidal that invert the polarity after each Schwave cycle, therefore a whole cycle corresponds to Hale cycle [15] .
3.2. Wavelet Analysis of Sunspot Number and Surface Wave Magnitude of Earthquakes Considering Annual Maximum Values
The temporal correlation between annual averages MS (energy release sequences of strong earthquakes in Chile) and SSN (Sunspots Number) were analyzed using the Continuous Wavelet Transform (CWT) as a band-pass filter. A wavelet is a function that is localized in time and frequency. Morlet wavelet (1) (with ω0 = 6) was utilized because it provides a good balance between time and frequency localization:
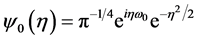 (1)
(1)
where ω0 is dimensionless frequency and η is dimensionless time.
Figure 4(a) and Figure 4(b) show the periodic oscillation of the annual seismic moment of mayor earthquakes in Chile and the sunspot numbers. Figure 4(a) remarks the 11 yrs. periodicity of Sunspot Numbers along the complete period of time analyzed. Figure 4(b) shows an 11 years periodicity on 1995 to 1980, a small 8 - 11 yrs. periodicity between 1990-2000; 3 - 6 yrs. between 1925-1935 and 1955-1965 of Msserie.
![]()
Figure 4. (a) The wavelet real part contour of annual sunspot numbers series. (b) The wavelet real part contour of seismic energy release series. (c) The thick black contour designates the 5% significance level against red noise and the cone of influence (COI) where edge effects might distort the picture is shown as lighter shade. The relative phase is shown as arrows (with in-phase pointing right, anti-phase pointing left and SSN leading Ms by 90˚ pointing straight down).
We examined the two time series together to find a link in some way. From two CWTs we constructed the Cross Wavelet Transform (XWT) which will expose their common power and relative phase in time-frequency space. We used the cross wavelet transform (XWT) for examining relationships in time frequency space between both time series. The theoretical distribution of cross wavelet transform of two time series is given in [16] . We used the corresponding software at
http://noc.ac.uk/using-science/crosswavelet-wavelet-coherence. The result is showed in Figure 4(c).
We applied a measure of Wavelet Transform Coherence (WTC) between the two CWTs, to find significant coherence, if the two series are physically related we would expect a consistent or slowly varying phase. Monte Carlo methods are used to calculate the statistical significance against red noise backgrounds.
Wavelet Transform Coherence (WTC) of the SSN and Ms are shown in Figure 5. There are clearly common features in the wavelet power of the two series such as the significant peak in the ~11 year band around 1959 (Maximum of 19-Solar Cycle). This coherence seems to be stable between 1930 and 2000.
4. Results and Discussions
From the above analysis, it can conclude that wavelets provide a new tool for the comparition of the periods of earthquakes activity and solar activity.
It could be a possible qualitative mechanism caused by solar activity that would influence seismic activity (proposed [17] ).
![]()
Figure 5. Squared WTC coherence between SSN and Ms series. It confirms the coherence’s intermittency around 8 - 12 years band. The horizontal dashed lines are the approximated bounds of 95% confidence level zones.
A summary of the effects of solar activity on the seismic activity should include the following elements:
・ pressure pulses associated with fast solar wind or CME that compresses the magnetosphere;
・ auroralelectrojet would be strengthened;
・ atmospheric gravity waves would be generated and transmitted to lower altitudes;
・ zonal winds would be strengthened westward;
・ changes in surface air pressure;
・ pressure balance of tectonic plates would be interrupted and tension accumulation is enough to trigger seismic activity.
5. Conclusions
By using the method of Fast Fourier Transform to study the periodicity in MS for the last hundred years, periodicities of 4; 4.5; 5.5; 9; 11 and 13.5 were found for earthquakes. Some of these results were previously obtained (see Table 1).
According to the statistical study done, periodicities of approximately 8 - 12 years are observed in both SSN and MS. Similar periodicities are also observed in the orbital movements and orbital synodical of large planets. Reference [18] showed evidence that seismic activity could be caused by internal tides produced by the gravitational force of large planets and the moon.
From the study of the temporal correlation between SSN y MS, it was applied an independent method based on Wavelet Transform (squared WTC) [19] . It confirms the coherence’s intermittency around 8 - 12 years band.
It can be inferred that there is a connection between solar and seismic activities.
This study does not include the prediction of earthquakes. In order to do so, it should also be taken into account the Sun-Earth interaction as an extra variable.
Acknowledgements
This research work is part of project No. 20020090100131 (UBACYT), Argentina.