Part 2: Review of Tokamak Physics as a Way to Construct a Device Optimal for Graviton Detection and Generation within a Confined Small Spatial Volume, as Opposed to Dyson’s “Infinite Astrophysical Volume” Calculations ()
1. Review of the Dyson Argument Which We Cite for Use in Tokamaks
Our first goal is to show that Dyson’s arguments as given in [1] as to the impossibility of Graviton detection no longer apply to Tokamaks. Note, Dyson in [1] derived criteria as to the probability one could obtain physical phenomenon theoretically modelled by the Gertsenshtein effect [2] . The Gertsenshtein effect [2] is the coupling of magnetic fields, gravitons, and photons. In the Dyson treatment [1] of the Gertsenshtein effect [2] , Dyson hypothesized distances up to many light years for an interaction of magnetic fields, gravitons and photons, for experimental signals which could be detected on the Earth’s surface. This assumed geometry of many light years distance lead to the predicted Gertshenshtein effect [2] unable to allow for graviton detection. In contrast to this assumed vast distances for the Gertshenshtein effect in reference [1] , the author has devised via tokamak generation of gravity waves which is discussed in this article which lead to an interaction length of meters for the magnetic field, gravitons, and photons. The reduced length is due to the magnetic field which the gravitons interact with, being inside the detector itself, thereby insuring a 100% probability for the Gertsenshtein effect occurring. This is commensurate with predictions given in reference [3] . The Tokamak example brings up an important point, that even if one wants to measure gravitational waves, the Gertshenshtein effect for gravitons, magnetic field, and photons is within the small 3 dimensional geometry of the detector, with an enormous magnetic field. To do this note that we are talking about a Tokamak of the type described in [4] . Having the Gerteshenshtein effect in such a small volume dramatically raises the likelihood of detection of gravitons, via resultant photons being picked up by the 3DSR device which in this care would be put above the Tokamak given in [5] .
2. Probability for the Gertsentshtein Effect, as Described by Dyson for the Tokamak GW Experiment
The Gertentshtein effect is linked to how there is a linkage, signal wise, between gravitons and photons, and we are concerned as to what is a threshold as to insure that GW may be matched to the photons used by Dr. Li and others [5] to signify GW in a detector. To do so let us look at the Dyson criteria as a minimum threshold for the Gertentshtein effect happening [1] , namely
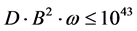 (1)
(1)
The propagation distance is given by D, the magnetic field by B, and the frequency of gravitational radiation is given by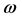 . We assume that the gravitational frequency is commensurate with the gravitational frequency of gravitons, i.e. that they are, averaged out one and the same thing. In doing so, making use of [1] we suppose on the basis of analysis that D is of the order of 10 to the 2nd power, since D is usually measured in centimetre, and by [1] we are thinking of about a 1 meter If B is of the order of 10 to the 9th Gauss Hertz, as deemed likely by the geometry as suited for [4] , then we have that if the GW frequency,
. We assume that the gravitational frequency is commensurate with the gravitational frequency of gravitons, i.e. that they are, averaged out one and the same thing. In doing so, making use of [1] we suppose on the basis of analysis that D is of the order of 10 to the 2nd power, since D is usually measured in centimetre, and by [1] we are thinking of about a 1 meter If B is of the order of 10 to the 9th Gauss Hertz, as deemed likely by the geometry as suited for [4] , then we have that if the GW frequency, 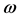 is likewise about 10 to the 9th Hertz , that Equation (1) is easy to satisfy.
is likewise about 10 to the 9th Hertz , that Equation (1) is easy to satisfy.
The problem though is that we need to go to the Li Dectector for 3DSR, and step beyond the first document’s Gertenshehtein effect becomes then having the probability below become exactly zero, in the case of the first equation in the bunch of Eq. Given in Equation (2) below, due to the tininess of D (distance)
 (2)
(2)
We will have a different measurement protocol in place than the Gertenshehtein effect.
Keep in mind that the GW detector is, as given in the 2nd part of this article that if it is situated about five meters above the Tokamak, i.e. presumably the one in Hefei, PRC [4] . Note, that, ironically, Dyson gets much larger values of Equation (2) than the above setting the frequency to be a huge value , by postulating GW frequency inputs as to the value of 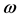 about 10 to the 20th Hertz, i.e. our value of
about 10 to the 20th Hertz, i.e. our value of 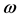 is likewise about 10 to the 9th Hertz, much lower. If one has such a high frequency, as given by Dyson, the of course, Equation (2) would then be close to zero for the probability of the Gertentshtein effect happening. i.e. our analysis indicates that a medium high GW frequency, presumably close to 10 to the 9th Hertz, and D 10 to the 2nd power, presenting satisfaction of both Equation (1) and Equation (2). Note the main point though, for large values of D, Equation (1) will not hold, making Equation (2) not relevant, and that means in terms of the Dyson analysis, that far away objects generating gravitons will not be detectable. Via the Gertentshtein effect. However, in our case we use the 3DSR effect since the Gertsenshtein effect is ruled out. Having said that, we have our modus operandi, which is to attempt to look at the way Plasma physics could lead to graviton and GW generation. This means looking at [5] , and also considering the phenomenology as given in [6] which of course is to be kept relevant to the restrictions as given in [5] [7] .
is likewise about 10 to the 9th Hertz, much lower. If one has such a high frequency, as given by Dyson, the of course, Equation (2) would then be close to zero for the probability of the Gertentshtein effect happening. i.e. our analysis indicates that a medium high GW frequency, presumably close to 10 to the 9th Hertz, and D 10 to the 2nd power, presenting satisfaction of both Equation (1) and Equation (2). Note the main point though, for large values of D, Equation (1) will not hold, making Equation (2) not relevant, and that means in terms of the Dyson analysis, that far away objects generating gravitons will not be detectable. Via the Gertentshtein effect. However, in our case we use the 3DSR effect since the Gertsenshtein effect is ruled out. Having said that, we have our modus operandi, which is to attempt to look at the way Plasma physics could lead to graviton and GW generation. This means looking at [5] , and also considering the phenomenology as given in [6] which of course is to be kept relevant to the restrictions as given in [5] [7] .
3. Introduction to the Plasma Physics
Russian physicists Grishchuk and Sachin [8] obtained the amplitude of a Gravitational wave (GW) in a plasma as
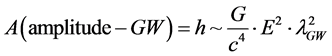 (3)
(3)
This should be compared with [9] , and we can diagram the situation out as follows [10] .
Note that a simple model of how to provide a current in the Toroid is provided by a transformer core. This diagram is an example of how to induce the current I, used in the simple Ohms law derivation referred to in the first part of the text.
Here, E is the electric field whereas  is the gravitational wavelength for GW generated by the Tokamak in our model. In the original Griskchuk model, we would have very small strain values, which will comment upon but which require the following relationship between GW wavelength and resultant frequency.
is the gravitational wavelength for GW generated by the Tokamak in our model. In the original Griskchuk model, we would have very small strain values, which will comment upon but which require the following relationship between GW wavelength and resultant frequency.
Note, if , so we will be assuming a baseline of the order of setting
, so we will be assuming a baseline of the order of setting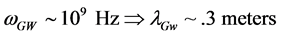 , as a baseline measurement for GW detection above the Tokamak. Furthermore, we will write the strain, introduced by (massive) Gravitons, as given by [11] . The precise values of the strain due solely to an Ohms law treatment of current, and the electric field will lead to, by first principles comparison of magnitude of terms using [9]
, as a baseline measurement for GW detection above the Tokamak. Furthermore, we will write the strain, introduced by (massive) Gravitons, as given by [11] . The precise values of the strain due solely to an Ohms law treatment of current, and the electric field will lead to, by first principles comparison of magnitude of terms using [9]
 (4)
(4)
where
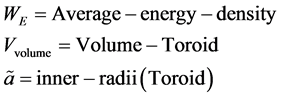 (4a)
(4a)
This Equation (2) above is due to the 1st term of a two part composition of the strain, with the 2nd term of the strain value significantly larger than the first term and due to ignition of the Plasma in the Tokamak. The first term of strain is largely due to what was calculated by Grishkuk [8] et al. The second term is due to Plasma fusion burning. This plasma fusion burning contribution is due to non-equilibrium contributions to Plasma ignition, which will be elaborated on in this document. Note that the first term in the strain derivation is due to the electric field within a Toroid, not Plasma fusion burning, and we will first of all discuss how to obtain the requisite strain, for the electric field contribution to the current, inside a Tokamak. Making use of Ohms law.
4. Comment as to the Derivation of Strain Generated by an Electric Field, and Small Strain Values in the Tokamak
We will examine the would-be electric field, contributing to a small strain values similar in part to Ohms law. A generalized Ohm’s law ties in well with Figure 1 and Figure 2
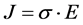 (5)
(5)
In order to obtain a suitable electric field, to be detected via 3DSR technology [4] [5] [11] , we will use a generalized Ohm’s law as given by Wesson [3] [10] (page 146), where E and B are electric and magnetic fields, and v is velocity. We should understand that this undercuts the use of Figure 2.
![]()
Figure 1. We outline the direction of Gravitational wave “flux”. If the arrow in the middle of the Tokamak ring perpendicular to the direction of the current represents the z axis, we represent where to put the GW detection device as 5 meters above the Tokamak ring along the z axis. This diagram was initially from Wesson [3] .
![]()
Figure 2. Flux change provided by a transformer core, in the simple current model first referred to in this paper. This figure is from Wesson [10] .
 (6)
(6)
As discussed with Dr. Wen Hao in November, 2014, in Chongqing University, the term in Equation (6) given as ![]() deserves special commentary. If
deserves special commentary. If ![]() is perpendicular to
is perpendicular to ![]() as occurs in a simple equilibrium case, then of course, Equation (6) would be, simply put, Ohms law, and spatial equilibrium averaging would then lead to
as occurs in a simple equilibrium case, then of course, Equation (6) would be, simply put, Ohms law, and spatial equilibrium averaging would then lead to
![]() (7)
(7)
What saves the contribution of Plasma burning as a contributing factor to the Tokamak generation of GW, with far larger strain values commencing is that one does not have the velocity of ions in Plasma perpendicular to B fields in the beginning of Tokamak generation. It is, fortunately for us, a non equilibrium initial process, with thermal irregularities leading to both terms in Equation (7) contributing to the electric field values.
We will be looking for an application for radial free electric fields being applied e.g., Wesson [3] [10] (page 120)
![]() (8)
(8)
Here, ![]() = ion density, jth species,
= ion density, jth species, ![]() = ion charge, jth species,
= ion charge, jth species, ![]() = radial electric field,
= radial electric field, ![]() = perpendicular velocity, of jth species,
= perpendicular velocity, of jth species, ![]() = magnetic field, and
= magnetic field, and ![]() = pressure, jth species. The results of Equation (5) and Equation (6) are
= pressure, jth species. The results of Equation (5) and Equation (6) are
![]() (9)
(9)
Here, the 1st term is due to![]() , and the 2nd term is due to
, and the 2nd term is due to
![]() with the 1st term generating
with the 1st term generating ![]() in terms of
in terms of
GW amplitude strain 5 meters above the Tokamak, whereas the 2nd term has an ![]() in terms of GW amplitude above the Tokamak. The article has contributions from amplitude from the 1st and 2nd terms separately. The second part will be tabulated separately from the first contribution assuming a minimum temperature of
in terms of GW amplitude above the Tokamak. The article has contributions from amplitude from the 1st and 2nd terms separately. The second part will be tabulated separately from the first contribution assuming a minimum temperature of ![]() as from Wesson [10] .
as from Wesson [10] .
5. GW h Strain Values When the First Term of Equation (9) Is Used for Different Tokamaks
We now look at what we can expect with the simple Ohm’s law calculation for strain values. As it is, the effort lead to non-usable GW amplitude values of up to ![]() for GW wave amplitudes 5 meters above a Tokamak, and
for GW wave amplitudes 5 meters above a Tokamak, and ![]() in the centre of a Tokamak. i.e. this would be using Ohm’s law and these are sample values of the Tokamak generated GW amplitude, using the first term of Equation (9) and obtaining the following value [8] with a change as
in the centre of a Tokamak. i.e. this would be using Ohm’s law and these are sample values of the Tokamak generated GW amplitude, using the first term of Equation (9) and obtaining the following value [8] with a change as
![]() (9a)
(9a)
We summarize the results of such in our first table as given for when ![]() and with conductivity
and with conductivity ![]() and with the following provisions as to initial values. What we observe are a range of Tokamak values which are, even in the case of ITER (not yet built) beyond the reach of any technological detection devices which are conceivable in the coming decade. This table and its results, assuming fixed conductivity values
and with the following provisions as to initial values. What we observe are a range of Tokamak values which are, even in the case of ITER (not yet built) beyond the reach of any technological detection devices which are conceivable in the coming decade. This table and its results, assuming fixed conductivity values ![]() as well as
as well as ![]() is why the author, after due consideration completed his derivation of results as to the 2nd term of Equation (9) which lead to even for when considering the results for the Chinese Tokamak in Hefei to have [6]
is why the author, after due consideration completed his derivation of results as to the 2nd term of Equation (9) which lead to even for when considering the results for the Chinese Tokamak in Hefei to have [6]
![]() (10)
(10)
Or values 10,000 larger than the results in ITER due to Equation (10).
We summarize the results of such in our first table as given for when ![]() and with information from Table 1 of Appendix A, so View Appendix A below which has useful data.
and with information from Table 1 of Appendix A, so View Appendix A below which has useful data.
Note that we are setting![]() ,
, ![]() , using Equation (11) above for Amplitude of GW.
, using Equation (11) above for Amplitude of GW.
What makes it mandatory to go the 2nd term of Equation (11) is that even in the case of ITER, 5 meters above the Tokamak ring, the GW amplitude is 1/10,000 the size of any reasonable GW detection device, and this including the new 3DSR technology (Li et al, 2009) [5] [11] . Hence, we need to come up with a better estimate, which is what the 2nd term of Equation (7) is about which is derived in the next section
6. Enhancing GW Strain Amplitude via Utilizing a Burning Plasma Drift Current: Equation (6)
The way forward is to go to Wesson, [10] (2011, page 120) and to look at the normal to surface induced electric field contribution
![]() (11)
(11)
If one has for ![]() as the radial velocity of ions in the Tokamak from Tokamak centre to its radial distance, R, from centre, and
as the radial velocity of ions in the Tokamak from Tokamak centre to its radial distance, R, from centre, and ![]() as the direction of a magnetic field in the “face” of a Toroid containing the Plasma, in the angular
as the direction of a magnetic field in the “face” of a Toroid containing the Plasma, in the angular ![]() direction from a minimal toroid radius of
direction from a minimal toroid radius of![]() , with
, with![]() , to
, to ![]() with
with![]() , one has
, one has ![]() for radial drift velocity of ions in the Tokamak, and
for radial drift velocity of ions in the Tokamak, and ![]() having a net approximate value of: with
having a net approximate value of: with ![]() not perpendicular to the ion velocity, so then [10]
not perpendicular to the ion velocity, so then [10]
![]() (12)
(12)
This should be considered within the constraints given by [11] as well as the geometry given in [12] for the Hefei Tokamak.
Also, as a first order approximation: From Wesson [10] (page 167) the spatial change in pressure denoted
![]() (13)
(13)
Here (ibid), the drift current, using![]() , and drift current
, and drift current ![]() for Plasma charges, i.e.
for Plasma charges, i.e.
![]() (14)
(14)
Figure 3 introduces the role of the drift current, in terms of Tokamaks [10]
Then one has
![]() (15)
(15)
Now, the behaviour of the numerical density of ions, can be given as follows, namely growing in the radial direction, then [10]
![]()
Figure 3. Typical bootstrap currents with a shift due to r/a where r is the radial direction of the Tokamak, and a is the inner radius of the Toroid. This figure is reproduced from Wesson [10] .
![]() (16)
(16)
This exponential behaviour then will lead to the 2nd term in Equation (9) having in the centre of the Tokamak, for an ignition temperature of ![]() a value of
a value of
![]() (17)
(17)
As shown in Figure 4 (copied from Wesson 2011), [10] there is a critical ignition temperature at its lowest point of the curve in the having ![]() as an optimum value of the Tokamak ignition temperature for
as an optimum value of the Tokamak ignition temperature for![]() , with a still per-
, with a still per-
missible temperature value of ![]() with a value of
with a value of![]() , due to from page 11, [10] the relationship of Equation (18), where
, due to from page 11, [10] the relationship of Equation (18), where ![]() is a Tokamak
is a Tokamak
confinement of plasma time of about 1-3 seconds, at least due to [10]
![]() (18)
(18)
Also, as shown in Figure 4, ![]() then one could have at the Tokamak centre, i.e. even the Hefei based Tokamak [10] [12]
then one could have at the Tokamak centre, i.e. even the Hefei based Tokamak [10] [12]
![]() (19)
(19)
This would lead to, for a GW reading 5 meters above the Tokamak, then lead to for then the Hefei PRC Tokamak [10] [12]
![]() (20)
(20)
Note that the support for up to 100 KeV for temperature can yield more stability in terms of thermal Plasma confinement as give in Figure 5 below, namely from [10] we have
![]()
Figure 4. The value of n ![]() required to obtain ignition, as a function of temperature. Figure reproduced from Wesson [10] .
required to obtain ignition, as a function of temperature. Figure reproduced from Wesson [10] .
![]()
Figure 5. Illustrating how increase in temperature can lead to the H mode region, in Tokamak physics where the designated equilibrium point, in Figure 5 is a known way to balance conduction loss with alpha particle power, which is a known way to increase ![]() i.e. Tokamak confinement of plasma time [9] .
i.e. Tokamak confinement of plasma time [9] .
7. Details of the Model in Terms of Terms of Adding Impurities to the Plasma to Get a Longer Confinement Time (Possibly to Improve the Chances of GW Detection)
We add this detail in, due to a question raised by Dr. Li who wished for longer confinement times for the Plasma in order to allegedly improve the chances of GW detection for a detector 5 meters above the Tokamak in Hefei. Wesson [10] (2011) stated that the confinement time may be made proportional to the numerical density of argon/neon seeded to the plasma [10] (page 180). This depends upon the nature of the Tokamak, but it is a known technique, and is suitable for analysis, depending upon the specifics of the Tokamak. i.e. this is a detail Dr. Li raised with his co-workers in Hefei, PRC in 2014 [12] .
8. Restating the Energy Density and Power Which Would Be in the Hefei Tokamak, Using the Formalism of Equation (4) Directly
![]() (21)
(21)
The temperature for Plasma fusion burning, is then about between 30 to 100 KeV, as given by Wesson [10] .
The corresponding power as given by Wesson is then for the Tokamak [10]
![]() (22)
(22)
The tie in with Equation (20) by Equation (22) can be seen by first of all setting the E field as related to the B field, via E (electrostatic)~![]() as equivalent to a magnetic field B~
as equivalent to a magnetic field B~![]() as given by [9] . In a one second interval, if we use the input power as an experimentally supplied quantity, then the effective E field is
as given by [9] . In a one second interval, if we use the input power as an experimentally supplied quantity, then the effective E field is
![]() (23)
(23)
What is found is, that if Equation (22) and Equation (23) hold. Then by Wesson [10] , pp. 242-243, if ![]() Then the temperature of a Tokamak, to good approximation would be between 30 to 100 KeV, and then one has [10]
Then the temperature of a Tokamak, to good approximation would be between 30 to 100 KeV, and then one has [10]
![]() (24)
(24)
Then the power for the Tokamak is
![]() (25)
(25)
Then, per second, the author derived the following rate of production per second of a ![]() graviton, as given by, if
graviton, as given by, if ![]()
![]() (26)
(26)
If there is a fixed mass for a massive graviton, the above means that as the wavelength decreases, that the number of gravitons produced between plasma burning temperatures of 30 to 100 KeV changes dramatically. The change in graviton number is not nearly so sensitive as to Plasma fusion burning as for 30 to 100 KeV temperature variation.
Numerical inputs into Equation (26) have indicated that there are roughly 1000 gravitons per second generated by Plasma fusion burning, with a strain value of ![]() 5 meters above the centre of the Hefei Tokamak [12] . If so, then the long confinement time of the Hefei Tokamak, for plasmas, would indicate a chance that a detector may be able to obtain a graviton signal. That depends upon if
5 meters above the centre of the Hefei Tokamak [12] . If so, then the long confinement time of the Hefei Tokamak, for plasmas, would indicate a chance that a detector may be able to obtain a graviton signal. That depends upon if ![]() is, with the equipment available actually detectable. If so, then the next task is the extremely time consuming process of experimental verification of the measurements, and answering questions as to the reliability of the obtained data sets.
is, with the equipment available actually detectable. If so, then the next task is the extremely time consuming process of experimental verification of the measurements, and answering questions as to the reliability of the obtained data sets.
9. Looking at the Role of Gravitons, in Massless and Massive Gravity Configurations
We do note that there will be a complimentary relationship between presumed brane world generated GW [13] , which has the similar order of magnitude to the Tokamak generated GW and gravitons. Hence, due to [13] we will bring up some material which is related to the nature of gravitons, from first principles.
Note that in the early universe, the following may be considered as complimentary, i.e. that we are looking at material from [14] [15] . To start of with consider how to obtain massive gravitons, and to do that we start with material from [16] .
![]() (27)
(27)
Which in turn may help us understand when the formation of this value occurred, i.e. [17]
![]() (28)
(28)
We are supposing that Equation (27) and Equation (28) holds at the formation of a Schwartzshield mass of the Universe radius. Also, here is our candidate as to the formation of an initial time step. As given.
![]() (29)
(29)
Then, up to a point, if the above is in terms of seconds, and N sufficiently large, we could be talking about an initial non zero entropy, along the lines of the number of nucleated particles, at the start of the cosmological era. As given by making use of quantum infinite statistics as well as our adaptation of it [18]
![]() (30)
(30)
Initial entropy would be small, but non zero, and would be affected by ![]() strongly, i.e. the initial degrees of freedom assume would play a major role as far as how initial entropy and initial time steps would be initiated. i.e. in this configuration, there is room then for in the early universe, a rest mass of a graviton, of approximately 10^ −62 grams, which would tie an initial non zero entropy, as in Equation (30), with an initial time step, as given in Equation (29), with the number, n~N of gravitons as given with mass m, of Equation (28), as possibly conferring information transfer, i.e. looking at what shows up as a proportionality factor as far as how to obtain entropy is in having the following set up, i.e. if we look eventually at the Schwartzhield radius of the universe as occurring at redshift z = 1000, about 300,000 years after the big bang, then
strongly, i.e. the initial degrees of freedom assume would play a major role as far as how initial entropy and initial time steps would be initiated. i.e. in this configuration, there is room then for in the early universe, a rest mass of a graviton, of approximately 10^ −62 grams, which would tie an initial non zero entropy, as in Equation (30), with an initial time step, as given in Equation (29), with the number, n~N of gravitons as given with mass m, of Equation (28), as possibly conferring information transfer, i.e. looking at what shows up as a proportionality factor as far as how to obtain entropy is in having the following set up, i.e. if we look eventually at the Schwartzhield radius of the universe as occurring at redshift z = 1000, about 300,000 years after the big bang, then
![]() (31)
(31)
Furthermore, there is a linkage which can be made to Seth Lloyds number of operations, i.e. [16]
![]() (32)
(32)
Whereas if t (initial) ~ t (H) as given below
![]() (33)
(33)
The entropy of the universe, as given by S~N, with the linkage as given by Ng. [19] would tie into an initial frequency range, which would be extremely high, and that due to the smallness of the wavelength, as given by Equation (33) above.
If initial wavelength, as given by Equation (33) is inversely proportional to an INITIAL version of energy, then there could be a version of this affecting the mass of a graviton as given by![]() , being re written, as through use of Equation (29) as of the rest mass of a graviton about Planck time leading to
, being re written, as through use of Equation (29) as of the rest mass of a graviton about Planck time leading to
![]() (34)
(34)
i.e. before Planck time, we would have the graviton mass as effectively zero, and it would about the time of Planck time, scale as given in Equation (34) with graviton rest mass eventually being in tandem with Equation (28)
Then, if the following hold before and after Planck time [18]
![]() (35)
(35)
Equation (34) and Equation (35) define the range of values of the Planck mass, as given, and also, the Pre Octonionic (Pre Planckian time) regime to Planckian time regime as given by
What we are considering is the following transformation, simply put. And this will be hopefully detected by a change in phase, given by use of reference [6] style phase ![]()
In addition we use [19]
![]() (36)
(36)
10. Now about Conditions to Obtain the Relevant Data for Phase ![]()
This paper examines geometric changes that occurred in the earliest phase of the universe, leading to values for data collection of information for phase![]() , and explores how those geometric changes may be measured through gravitational wave data. The change in geometry is occurring when we have first a pre quantum space time state, in which, in commutation relations [20] (Crowell, 2005) in the pre Octionic space time regime no approach to QM commutations is possible as seen by Equation (37), i.e in Pre Octonionic, Pre Planckian space time [20]
, and explores how those geometric changes may be measured through gravitational wave data. The change in geometry is occurring when we have first a pre quantum space time state, in which, in commutation relations [20] (Crowell, 2005) in the pre Octionic space time regime no approach to QM commutations is possible as seen by Equation (37), i.e in Pre Octonionic, Pre Planckian space time [20]
![]() (37)
(37)
In the situation when we approach quantum “octonionic gravity applicable” geometry, Equation (34) becomes [20]
![]() (38)
(38)
Having said that, if Gravitons are from the early electro weak era, in terms of production, in early universe conditions, the situation is definable via [15] .
We will elaborate upon this, but we have to state that purely massless gravitons are commensurate with the Pre Planckian era, and that conditions given in [14] hold.
i.e. for the Tokamak, we are working with the present day era of Equation (35) given above.
11. Examination of Our Write up about Gravitons and the Tie in with the HUP, and Cosmological Constant with the Work of Yan-Gang Miao, and Ying-Jie Zhao
In [21] there is a use of a relationship between the size of a spatial interval of space-time and the cosmological constant. What is of interest, is that due to what the authors call a suppression index, which they call “n”, that the authors up to a point partially confirm the results we have been talking of. i.e., the difference is that they use their results to confirm the existence of a cosmological constant in its present value, but do not discuss the pre Planckian space-time considerations we have brought up. It is of note though that their suppression index, “n” is of the magnitude of present day estimations of entropy (if we take Entropy as a counting of “particles” in space-time). In doing so, we take note that the “suppression” index so obtained was of the order of 10^121, which is according to [22] tied into estimations of early to late space-time dynamics, which is given credence in [23] . The noteworthy matter to bring to the attention of the readers is that there is in formula 10 of reference [21] an explicit “vacuum energy” expression, which has the minimum length, delta x, of the order of Planck’s length as part of a formula leading to “vacuum energy” in formula 10 of reference [21] which is presumed to be of the magnitude of the Cosmological “constant”. Left unsaid though is then the derivation of the factor “n” as part of a “suppression” meme of reducing an initially huge energy value, by 10^−121 as given in Equation (11) of reference [21] . However in dong so, the factor “n” has the value of 10^123 which the authors then say was corrected to be of the value of 10^121.
Here is the take away. If one is making the identification of S~“n”, as in a counting algorithm, for entropy along the lines of Ng’s infinite quantum statistics, as given in [18] , then the suppression factor “n” is of the magnitude of the present entropy of the universe which could be predicted via following [22] [23] . Hence, we make the following identification, namely following [18] [21]
![]() (39)
(39)
If we literally took this as gospel, we would be assuming the existence of an enormous suppression factor index “n” would be enough if in tandem with entropy, to state, if “n” were fixed, that we would have the existence of a cosmological constant of today’s value, if formula 10 of reference [21] held. Needless to say though if “n” ~ N~entropy (early universe) were considerably smaller than 10^121, then the cosmological “constant” of the early universe would be considerably larger than what it is today. By many orders of magnitude.
The equations in question from [21] reads as follows, namely
![]() (40)
(40)
The vacuum energy in question is the same as the cosmological constant, if and only if the factor “n” is so enormous. Aside from the numerology, which is suggestive, there is a basic inconsistency which we wish to find an answer to because the “vacuum energy” starts off with a Planck’s length, but the presumed ![]() suggests Planckian physics.
suggests Planckian physics.
We wish to find a resolution between this apparent inconsistency in future work, but we applaud the insight of [21] as linking “vacuum energy”, “n” and the minimum length, which is in their estimation Planck length.
Needless to say, up to a point they may be arguing for “quintessence” if much smaller “n” as N which is proportional to early universe entropy existed. We do know though by the supernova candle, that by the time of the formation of the first stars, that the cosmological “constant” was stable and of the same value as of the present era. This is a topic which the research group is well aware of and which deserves specific study and review.
12. Conclusion. GW Generation Due to the Thermal Output of Plasma Burning
Further elaboration of this matter in the experimental detection of experimental data sets for massive gravity lies in the viability of the expression derived, namely Equation (21). ![]() for a GW detected 5 meters above a Tokamak represents the extreme limits of what could be detected, but it is within the design specifications of what Dr. Li et al. (2009) [4] [5] presented for PRD readership. The challenge, as frankly brought up in discussions in Chongqing University is to push development of 3DSR hardware to its limits, and use the Hefei Tokamak configuration as a test bed for the new technology embodied in the Plasma fusion burning generation of Gravitation waves.
for a GW detected 5 meters above a Tokamak represents the extreme limits of what could be detected, but it is within the design specifications of what Dr. Li et al. (2009) [4] [5] presented for PRD readership. The challenge, as frankly brought up in discussions in Chongqing University is to push development of 3DSR hardware to its limits, and use the Hefei Tokamak configuration as a test bed for the new technology embodied in the Plasma fusion burning generation of Gravitation waves.
The importance of the formulation is in the explicit importance of temperature. i.e. a temperature range of at least![]() . In making this range for Equation (25), care must also be taken to obtain a sufficiently long confinement time for the fusion plasma in the Tokamak of at least 1 second or longer, and this is a matter of applied engineering dependent upon the instrumentation of the Tokamak in Hefei, PRC.
. In making this range for Equation (25), care must also be taken to obtain a sufficiently long confinement time for the fusion plasma in the Tokamak of at least 1 second or longer, and this is a matter of applied engineering dependent upon the instrumentation of the Tokamak in Hefei, PRC.
Furthermore, .Wen, Li, and Fang, proved in [13] the likelihood of brane world generation of HFGW which are close to the values of strain and frequency which could be generated by the Tokamak described above.
We also should be aware, of taking consideration of the extreme non linearity of the conductivity of plasmas as discussed in [24] as the kinks and irregularities in the magnetic field, are a serious contributor to the irregularities in the MHD simulations which will be part of a future study, of the effects of gravity wave and graviton generation later on.
This marks the conclusion of this submitted document for Advances in High Energy Physics, By Dr. Andrew Walcott Beckwith.
Acknowledgements
Gary Stevenson was largely responsible for the inputs of Appendix A, and Amara Angelica also asked questions as to engineering issues which lead to the formulation of the equations in this document. Thanks to both of them for cleaning many parts of the Tokamak work so presented. Also, several of the diagrams came directly from Gary Stevenson, as illustrations of engineering concepts used in section 3 of this document.
The author thanks. Dr. Yang Xi whom imbued the author with the background in the U of Houston to obtain a doctorate in physics Also, my recently deceased father is thanked whom discussed decades ago with me as to the worth of five dimensional geometry, in cosmology. Also, the Chongqing University physics department is thanked for the affiliation which has led to this article being formed in the first place for review, and that also Dr. Fangyu Li, who encouraged me to explore such issues. This work is supported in part by National Nature Science Foundation of China grant No. 11375279.
Statement as to there being no Conflict of interest: The author declares that there is no conflict of interest as to the publication of this document to the best of his knowledge.
Statement being that there is no conflict of interest due to the support of the National Nature foundation of China for the publishing of this document.
The present document is presented to JHEPGC, on account of the refusal of Hindawi, in particular Advances in High energy physics to heed the recommendation of two invited Editors, for inclusion of this document in a special issue of Quantum gravity and applications. Contrary to the wishes of the two invited editors for the special edition, Advances in High energy physics authorized. Due to a violation of protocol, by Advances in high energy physics, this document and its companion piece was pulled from Advances in high energy physics and re submitted to the JHEPGC journal. This is due to Advances in high energy physics refusing to follow journalistic review protocol and micromanaging the acceptance of this document when in fact it was deemed worthy of acceptance by the invited editors.
Appendix A
![]()
Submit or recommend next manuscript to SCIRP and we will provide best service for you:
Accepting pre-submission inquiries through Email, Facebook, LinkedIn, Twitter, etc.
A wide selection of journals (inclusive of 9 subjects, more than 200 journals)
Providing 24-hour high-quality service
User-friendly online submission system
Fair and swift peer-review system
Efficient typesetting and proofreading procedure
Display of the result of downloads and visits, as well as the number of cited articles
Maximum dissemination of your research work
Submit your manuscript at: http://papersubmission.scirp.org/
Or contact jhepgc@scirp.org