Received 16 May 2016; accepted 14 August 2016; published 17 August 2016

1. Introduction
The paper summarizes the contributions of the three schools of thought or philosophies of mathematics―logi- cism [1] , intuitionism-constructivism (constructivism for short) [2] and formalism [3] and their rectification― that constitute the new foundations of mathematics [4] . Their critique-rectification undertaken in [4] including the critique-rectification of the real number system [5] summarized below is the main contribution of [4] . The main contribution of logicism is the discovery of the Russell Antimony [6] and that of intuitionism the rejection of the indirect proof as a case of self-reference [4] [7] . L. E. J. Brouwer’s requirement not only that every concept and conclusion of a theorem are not vacuous (i.e., they exist) but also constructible or demonstrable advanced intuitionism to the philosophy of constructivism [8] [9] . Mathematics made another major advance when David Hilbert discovered a century ago that the concepts of individual thought being inaccessible to others are ambiguous and required that mathematical concepts be objects in the real world called concepts such as words, symbols, numerals, figures and chess pieces subject to consistent basic premises or axioms which gave rise to the philosophy of formalism [3] . This resolves the problem with the equation 1 = 0.99×××, which is wrong and akin to the statement apple = orange, since 1 and 0.99××× are distinct objects. The problem is resolved by defining “=” as the relation “the same as”. Then 1 ¹ 0.99××× and “=” is an equivalence relation and therefore satisfies the identity, reflexivity and transitivity properties which need not be taken as axioms.
Until today, mathematicians have not grasped the significance of Hilbert’s discovery to the extent that all textbooks in mathematics assume 1 = 0.99××× When this issue was raised in internet forums in 1997, especially, SciMath, it sparked a howl of protest and controversy with a whisk of name calling that lasted over a decade and spilled over into many websites.
2. Critique-Rectification of Traditional Mathematics
The full critique-rectification of traditional mathematics and its foundations done in [4] completes the construction of the new mathematics and its foundations started by Hilbert. While he required the concepts of a mathematical system or space to be subject to consistent axioms or basic premises to avoid contradiction or paradox he still left a big hole in mathematics with admission of ambiguity, e.g., undefined and vacuous concepts, and retention of the indirect proof which has been rejected by constructivism for compelling reason. We complete the rectification by rejecting the indirect proof once again and requiring that every concept be defined by the axioms and their properties and behavior be derived from or supported by them.
One of the field axioms of the real number system [10] , the trichotomy axiom, is false according to the following counterexample [5] :
Given two rationals x, y we can tell if x < y or x > y. Even then, we cannot line up all the rationals on the real line under the ordering < due to the ambiguity of the infinite number of rationals between any two given rationals and this is due to the ambiguity of the concept infinity. However, we can proceed with the following scenario: start with a certain rational interval [A, B] with A < C < B, and find a nested sequence of rational intervals [An, Bn], with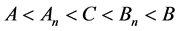 , for each
, for each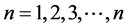 . At each stage, we want to make sure that
. At each stage, we want to make sure that
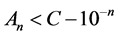 ,
,  , and
, and .
.
Since the nonterminating decimal C is defined to be the limit of a sequence of rationals (from the left and from the right), we can choose the end points An, Bn, of intervals [An, Bn] as members of two sequences {An}, {Bn} where {An} is a monotonic increasing sequence and {Bn} a monotonic decreasing sequence of rationalssatisfying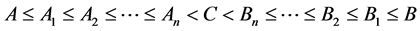 , and for each n,
, and for each n, 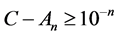 and
and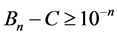 . This process can be continued as long as we are able to identify An, Bn to be such that An < C < Bn, i.e. as far as we know the decimal representation of C with its n decimal digits. It cannot be taken further since we are unable to find An+1, Bn+1 with error of 10−(n+1) and establish An+1 < C < Bn+1, with C being known only to n places. No matter how large the number n is, we still have the disadvantage of not getting the next interval [An+1, Bn+1]. Consequently, we have to acknowledge the inherent trouble involved with understanding and dealing with nonterminating decimals and with the concept of infinity. This example shows that the real number system has no ordering under the relation < and the trichotomy axiom which says, given two real numbers x, y, only one of the following holds: x < y, x = y, x > y, is unverifiable.
. This process can be continued as long as we are able to identify An, Bn to be such that An < C < Bn, i.e. as far as we know the decimal representation of C with its n decimal digits. It cannot be taken further since we are unable to find An+1, Bn+1 with error of 10−(n+1) and establish An+1 < C < Bn+1, with C being known only to n places. No matter how large the number n is, we still have the disadvantage of not getting the next interval [An+1, Bn+1]. Consequently, we have to acknowledge the inherent trouble involved with understanding and dealing with nonterminating decimals and with the concept of infinity. This example shows that the real number system has no ordering under the relation < and the trichotomy axiom which says, given two real numbers x, y, only one of the following holds: x < y, x = y, x > y, is unverifiable.
The counterexample says that the real number system is not linearly ordered by the relation “<” and collapses traditional calculus. We summarize the requirements for error-, ambiguity- and contradiction-free mathematical space; the sources of these defects are laid out in [4] .
1) Every concept is defined by the axioms. Although undefined concepts may be introduced initially in the construction of a mathematical space the choice of the axioms is not complete until every concept is defined. While the choice of axioms is arbitrary depending on what the mathematical space is intended for, once chosen the mathematical space becomes deductive, i.e., every theorem follows from the axioms.
2) Avoidance of vacuous concept such as, root of the equation x2 + 1 = 0, denoted by  which yields the contradiction,
which yields the contradiction,  , from which follows 1 = 0 and I = 0. The concept i is vacuous because the above equation has no root. Ill-defined concepts are vacuous.
, from which follows 1 = 0 and I = 0. The concept i is vacuous because the above equation has no root. Ill-defined concepts are vacuous.
3) Avoidance of infinity that cannot be contained by replacing its traditional definition with the concept inexhaustibility as its essential property so that an infinite set cannot be contained in a finite set and if one tries to put its elements in a finite set some element will be left out at every step. This property of infinite set invalidates the axiom of choice when applied to infinite set [10] [11] . It makes the concept irrational as nonperiodic nonterminating decimal ambiguous, ill-defined since this property is not verifiable. The same argument collapses Cantor’s diagonal method [12] . Set theory [13] and the proof of the Banach-Tarski paradox are flawed [14] .
4) Avoidance of self-reference [4] , the source of the Russell paradoxes [15] [16] of which the most famous is the Russell antimony [6] . Russell’s remedy for his paradoxes is his type theory [17] that rejects them. The avoidance of self-reference collapses nondenumerable and non-measurable sets and the universal and power sets. They do not exist. The indirect proof is self-referent and is also rejected.
Requirement 1) invalidates proof of theorem involving concepts from two distinct mathematical spaces, e.g., Gödel’s incompleteness theorems [18] . By rejecting the indirect proof which is self-referent and a contradiction, Fermat’s last theorem (FLT) [19] cannot be disproved except by a counterexample.
By meeting all the requirements of the new foundations of mathematics stipulated in [4] and applying them to the reconstruction of the real number system we build a new mathematical space free of errors, ambiguity and contradiction (paradox) called the constructivist real number system.
The new methodology―qualitative mathematics―of the new mathematics is the mathematical or qualitative model of rational thought [20] and mathematical component and tool of the new scientific methodology of science―qualitative mathematics and modeling―introduced in and the main contribution of [21] , that shifts the subject matter of science from the appearances of nature (natural phenomena) to nature itself and lifts traditional science to the new science [22] articulated by the grand unified theory (GUT) [23] .
3. Critique of the Real Number System R and Its Foundations
We continue the critique of mathematics beyond [4] into the foundations of the real number system R, upon which most of traditional mathematics is anchored, to build the constructivist real number system R*. As noted in [4] , Cantor’s diagonal method [12] failed to construct a nondenumerable set but succeeded only in generating a countably infinite set of cardinality Ào and no other set of greater cardinality exists since the power set of a set does not. The real number system is presently defined by the field axioms [10] . Aside from having a number of undefined concepts, two of its axioms, the axiom of choice or its variant, the completeness axiom, and the trichotomy axiom are false.
4. The Constructivist Real Number System
The first constructivist mathematical spaces are the modern calculus of variations, optimal control theory and functional analysis built on generalized curves and surfaces discovered and developed by L. C. Young in a series of papers that started in the 1930s [24] - [28] and concluded in the book, Lectures in the Calculus of Variations and Optimal Control Theory [29] where the norm is the Young Measure [30] . This is the appropriate norm for the normed spaces considered by Young that make them consftructivist at the same time.
This paper, its extensions to other mathematical spaces [31] - [39] [41] [48] , theoretical applications to the natural sciences [20] [23] [40] [42] - [49] and technological applications [50] - [52] are constructivist. The requirement of constructability of concepts and proofs of theorems makes it impossible to prove a negative proposition such as FLT deductively. It can only be proved by a counterexample.
4.1. The Axioms of R*
Axiom 1. R*contains the elements 0, 1.
Axiom 2. The addition table (Figure 1).
Axiom 3. The multiplication table (Figure 2).
Axiom 1 says 0, 1 Î R*; they are the additive and multiplicative identities defined by Figure 1 and Figure 2 respectively, which are partial because the entries are digits that are sums or products of digits. Their extension to the full figures in the scientific notation or the metric system (Hindu Arabic or base 10 numerals) is quite familiar and will not concern us here. Given the updated definition of the relation “=”, how do we interpret the equation, 2 + 3 = 5 where the left and right sides of the equation are different? It means that the image of the binary mapping f+(2,3) = 2 + 3 is 5 in accordance with the addition figure. Together with Axioms 1 and 2 are, indeed, axioms because they insure the existence of the integers, define addition and multiplication as binary operations on them and specify their properties. We first construct the digits or basic integers: 2 = 1 + 1, 3 = 2 + 1, 4 = 3 + 1, 5 = 4 + 1, 6 = 5 + 1, 7 = 6 + 1, 8 = 7 + 1, 9 = 8 + 1 which apply only to basic integers that are sums and products. The extension of the two binary operations beyond the digits is quite clear from the tables; can also be proved by mathematical induction. We define the base integer 10 = 9 + 1 and use the metric system of numeration or scientific notation for large and small numbers.
Since the figure define finite sums and products the laws of addition and multiplication of arithmetic as well as the laws of signs which follow from the automorphism between the positive and negative numbers can be verified from them and need not be taken as axioms. In either case, finite mathematical induction can be applied if needed. The system of Hindu-Arabic numerals quantitatively models the metric system of measurement; so does the scientific notation.
4.2. The Inverses and Terminating Decimals
The additive inverse of an integer x, denoted by −x, satisfies the equation,
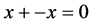 (1)
(1)
The prefix “−” is called the negative sign and “−x” is called “negative or additive inverse of x; when a decimal has no prefix it is understood that its prefix is “+” and the decimal is positive. The difference between integers x and y, denoted by x−y, is defined as x + −y. This operation called subtraction is the inverse of addition. It is clear that the additive inverse of the additive inverse of a decimal x, i.e., − −x (this notation is confusing and we replace it by −(−x)) which is x. This notation is consistent with the distributivity of signs with respect to addition which can be checked from Figure 1. The other rules of sign agree with the tables.
To avoid confusion, we may write the product of two integers a and b as a(b) or ab. The multiplicative inverse of a nonzero integer x, denoted by 1/x, (called reciprocal of x) satisfies,
 (2)
(2)
Provided x does not have a prime factor other than 2 and 5. The quotient of two integers x and y, denoted by x/y, where y has no prime factor other than 2 and 5, is a number z that satisfies the equation, x = yz. Note that if x, y are relatively prime and the divisor has a prime factor other than 2 and 5 the quotient is not defined being a nonterminating decimal. Thus, only terminating decimals are defined in R. When the quotient of x by y is defined we denote it by x/y.
In scientific notation we write an integer N as follows:
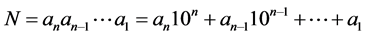 (3)
(3)
where the ajs, ![]() , are digits. A terminating decimal in the metric system is written as follows:
, are digits. A terminating decimal in the metric system is written as follows:
![]() (4)
(4)
where ![]() is the integral part,
is the integral part, ![]() the decimal part which is well-defined since 10 has only the factors 2 and 5 and its reciprocal, 1/10 = 0.1, is a terminating decimal. Thus, a terminating decimal is defined. If x and y are relatively prime, continued division will not yield a terminating decimal and the quotient is ill-de- fined. Therefore, we extend the real number system to include not only the nonterminating decimals but also its closure in a suitable norm that we will be introduced later.
the decimal part which is well-defined since 10 has only the factors 2 and 5 and its reciprocal, 1/10 = 0.1, is a terminating decimal. Thus, a terminating decimal is defined. If x and y are relatively prime, continued division will not yield a terminating decimal and the quotient is ill-de- fined. Therefore, we extend the real number system to include not only the nonterminating decimals but also its closure in a suitable norm that we will be introduced later.
4.3. The Nonterminating Decimals
A sequence of terminating decimals of the form,
![]() (5)
(5)
where N is a nonterminating decimal, is the g-limit (g-lim) of its nth g-term,
![]() (6)
(6)
as n ® ¥. This is called the g-nom of the nonterminating decimal (6); thus, the g-norm of a nonterminating decimal is the decimal itself. This, a nonterminating decimal has been defined for the first time and R* and every digit is computable. For example, the digits of p can be computed from its infinite series expansion [53] . A decimal is normal if every digit is chosen from the digits at random. The g-limit of (5) is the nonterminating decimal (6) provided the nth digits are not all 0 beyond the index n. In this case, we say that the g-sequence (5) converges to the nonterminating decimal (6) in the g-norm. Otherwise, (6) is terminating. A decimal consists of the integral part, the integer left of the decimal point, and the decimal part, the sequence of digits right of the decimal point.
We formally define an integer as the integral part of adecimal. The concept is no longer vacuous in R* since the decimals have been completely defined. The field axioms of R and Peano’s axioms [54] do not adequately define the natural numbers À [4] but since they are isomorphic to the integers under the mapping N → N.00×××, N integer, they are also integers in the sense of Ito [56] . (Special problems for undergrads are possible. For instance, an undergrad may study properties of a normal decimal).
In R the rationals coincide with the terminating decimals which are periodic. Since being nonterminating nonperiodic is not verifiable, the concept nonterminating nonperiodic decimal is vacuous and does not exist. Therefore, this concept is ill-defined, ambiguous. There are special nonterminating decimals which can be computed aside from p such as the natural logarithmic base e which can be computed from their series expansions; likewise, radicals can be computed [55] .
The nth g-term of a nonterminating decimal repeats every preceding g-term so that if finite initial g-terms are deleted the g-terms and g-limit of the remaining g sequence are unaltered. Thus, a nonterminating decimal has many g-sequences belonging to the equivalence class of its g-limits.
Since addition and multiplication and their inverse operations, subtraction and division, are defined only on terminating decimals computing a nonterminating decimal can only approximate it by the nth g-term (n-trunca- tion) of g-term. The same approximation holds for the difference, product and quotient (if defined). Computation involving terminating decimals alone is exact unless the result is nonterminating. This scheme holds for any combination of binary operations. Thus, we have retained standard computation but avoided the ambiguity and contradictions of the real number system the only difference being that every concept is defined in R*. We have also avoided vacuous approximation because nonterminating decimals are g-limits of their g-sequences which exist and belong to R*. Moreover, we have contained the ambiguity of nonterminating decimals by approximating them by their nth g-terms. This is an example of containment of ambiguity which is admissible in a mathematical space. In fact, containment of ambiguity is a standard technique in approximation theory. Converting an ordinary sequence to a g-sequence is obvious.
As we raise n in (6), the tail digits of the nth g-term of any decimal recedes to the right indefinitely, i.e., it becomes steadily smaller until it is unidentifiable from the tail digits of the rest of the decimals. Although it tends to 0 in the standard norm it never reaches 0 in the g-norm since the tail digits are never all equal to 0; it is also not a decimal since the digits are not fixed nor is it a real number. It is a set-valued number belonging to R* called algebraic continuum (continuum for short) denoted by d*; it is distinct from the topological continuum defined in terms of open sets. R* is an extension of R (the terminating decimals) so that every real number is an element of R*. The continuum d* is a special element of R* not belonging to R.
We introduce the general exponent and exponential (base e) where the exponent is allowed to take values along nonterminating decimals. Again, the exponent is the g-limit of the g-sequence of terminating decimals so that it is approximated by the nth g-sequence at desired error just as a non-terminating decimal is approximated.
For example, if a is a number, ![]() , as x ® a.
, as x ® a.
4.4. The Dark Number d*
Consider the sequence of decimals,
![]() (7)
(7)
where δ is any of the decimals, 0.1, 0.2, 0.3, ×××, 0.9, a1, ×××, ak, and the digits are not all 0 simultaneously. We call the nonstandard sequence (7) d-sequence and its nth term the nth d-term. For fixed combination of δ and the ajs, ![]() , in (7) the nth d-term is a terminating decimal and as n increases indefinitely it traces the tail digits of some nonterminating decimal and becomes smaller and smaller until it is indistinguishable from the tail digits of the other decimals. As n → ∞ the nth d-term recedes to the right and tends to some number d, its d-limit in the d-norm, which is never 0 (since the ajs are not simultaneously 0 and each d-term is not 0). Clearly the d-limit of (7) for all choices of the ajs,
, in (7) the nth d-term is a terminating decimal and as n increases indefinitely it traces the tail digits of some nonterminating decimal and becomes smaller and smaller until it is indistinguishable from the tail digits of the other decimals. As n → ∞ the nth d-term recedes to the right and tends to some number d, its d-limit in the d-norm, which is never 0 (since the ajs are not simultaneously 0 and each d-term is not 0). Clearly the d-limit of (7) for all choices of the ajs, ![]() , is the continuum d* and every element of d*, which is the d-limit of (7) for fixed δ and aj, is indistinguishable from the rest of the d-limits of (7) for all the other choices of δ and ajs. The continuum d* is countably infinite since any countably infinite set of sequences is countably infinite (We can generalize this to the statement: the countable union of countably infinite set is a countably infinite set). Thus, d* is set-valued and a continuum (negation of discrete) of dark numbers and the decimals are joined by the continuum d* at their tails. Thus, the set of decimals is a continuum, countably infinite and discrete and the terminating decimals, the real numbers, are embedded in it. While the nth d-term of (7) becomes smaller and smaller with indefinitely increasing n it is greater than 0 no matter how large n is so that if x is a decimal, 0 < d < x. If an equation, function or proposition is satisfied by every dark number d we may substitute d* for it so that we can write 0 < d* < x in the above inequality. Both the decimals and d* being countably infinite have cardinality Ào and no other set of numbers has greater cardinality according to [4] . Cantor’s diagonal method proves neither the existence of nondenumerable set nor that of a continuum; it proves only the existence of countably infinite set, i.e., the set of off-diagonal elements consisting of countable union of countably infinite sets which is countably infinite. The off-diagonal elements are even ill-defined since we know nothing about their digits.
, is the continuum d* and every element of d*, which is the d-limit of (7) for fixed δ and aj, is indistinguishable from the rest of the d-limits of (7) for all the other choices of δ and ajs. The continuum d* is countably infinite since any countably infinite set of sequences is countably infinite (We can generalize this to the statement: the countable union of countably infinite set is a countably infinite set). Thus, d* is set-valued and a continuum (negation of discrete) of dark numbers and the decimals are joined by the continuum d* at their tails. Thus, the set of decimals is a continuum, countably infinite and discrete and the terminating decimals, the real numbers, are embedded in it. While the nth d-term of (7) becomes smaller and smaller with indefinitely increasing n it is greater than 0 no matter how large n is so that if x is a decimal, 0 < d < x. If an equation, function or proposition is satisfied by every dark number d we may substitute d* for it so that we can write 0 < d* < x in the above inequality. Both the decimals and d* being countably infinite have cardinality Ào and no other set of numbers has greater cardinality according to [4] . Cantor’s diagonal method proves neither the existence of nondenumerable set nor that of a continuum; it proves only the existence of countably infinite set, i.e., the set of off-diagonal elements consisting of countable union of countably infinite sets which is countably infinite. The off-diagonal elements are even ill-defined since we know nothing about their digits.
Like a nonterminating decimal, an element of d* is unaltered if finite d-terms are altered or deleted from its d-sequence. When δ = 1 and ![]() (7) is called the basic or principal d-sequence of d*, its d-limit the principal element of d*; principal in the sense that all its d-sequences can be derived from it. Thus, the principal d-sequence of d* is,
(7) is called the basic or principal d-sequence of d*, its d-limit the principal element of d*; principal in the sense that all its d-sequences can be derived from it. Thus, the principal d-sequence of d* is,
![]() (8)
(8)
obtained from the iterated difference,
![]() (9)
(9)
excess remainder of 0.1;
![]() , excess remainder of 0.01;
, excess remainder of 0.01;
![]() , excess remainder of 0.001; …
, excess remainder of 0.001; …
Taking the g-limits of the extreme left side of (9) and recalling that the g-limit of a decimal is itself and denoting by dp the d-limit of the principal d-sequence on the rightmost side we have,
![]() (10)
(10)
Since all the elements of d* share its properties then whenever we have a statement “an element d of d* has property P” we may write “d* has property P”, meaning, this statement is true of every element of d*. This applies to any equation involving an element of d*. Therefore, we have,
![]() (11)
(11)
Like a decimal, we define d* as the d-norm of d*, i.e., the d-norm of d* is d*. We state our findings:
Theorem. The d-limit of the indefinitely receding to the right nth d-terms of d* is a continuum that coincides with the g-limits of the tail digits of the nonterminating decimals traced by them as the ajs vary along the digits.
If x < 1 and a nonzero decimal, there is no difference between (0.1)n and x(0.1)n as they become indistinguishably small, i.e., as n increases indefinitely. This is analogous to the sandwich theorem of calculus that says, lim(x/six) = 1, as x ® 0 [57] . In our case, if 0 < x < 1, 0 < x(0.1)n < (0.1)n and both extremes tend to 0 so must the middle term and they become indistinguishably small as n increases indefinitely. If x > 1, we simply reverse the inequality and obtain the same conclusion. Therefore, we may write, xdp = dp (where dp is the principal element of d*) and since the elements of d* share this property we may write xd* = d*, meaning, that xd = d for every element d of d*. We consider d* the equivalence class of its elements. In the case of x + (0.1)n and x, we look at the nth g-terms of each and, as n increases indefinitely, x + (0.1)n and x become indistinguishable. Now, since (0.1)n > ((0.1)m)n > 0 and the extreme terms both tend to 0 as n increases indefinitely, so must the middle term tend to 0 so that they become indistinguishably small (the reason d* is called dark for being indistinguishable from 0 yet greater than 0).
A decimal integer has the form N.99×××; if x is an integer or decimal integer (having the form x = N.99×××, N = 0, 1, ×××) we have,
![]() ,
,![]() (12)
(12)
![]()
![]()
The first line of (12) says that d* cannot be separated from an integer but the second and third lines say that d* can be separated from a decimal integer.
It follows that the g-closure of R, i.e., its closure in the g-norm, is R* which includes the additive inverses and well-defined multiplicative inverses and d*. We also include in R* the upper bounds of the divergent sequences of terminating decimals and integers (a sequence is divergent if the nth terms are unbounded as n increases indefinitely, e.g., the sequence 8, 88, ×××) called unbounded number u* which is countably infinite since the countable union of countable sequences is. We call the divergent sequence of an unbounded number u s-sequence. Like d* it is set-valued. We follow the same convention for u*: whenever we have a statement “u has property P for every element u of u*” we can simply say “u* has property P). Then u* satisfies for given x,
![]() (13)
(13)
Neither d* nor u* is a decimal and their properties are solely determined by their sequences. Then d* and u* have the following dual or reciprocal properties and relationship:
![]() (14)
(14)
Numbers like u*-u*, d*/d* and u*/u* are still indeterminate but indeterminacy is avoided by computation with the g- or d-terms or s-terms. Thus, we now have a well-defined arithmetic of infinitesimal and infinity where d* and u* are counterparts of each other in the constructivist real number system respectively.
We can check that associativity, commutativity and distributivity of multiplication with respect to addition follow from the axioms and need not be taken as axioms. We note that the rules of signs apply to the decimals but not d*.
The decimals are linearly ordered by the lexicographic ordering by “<” defined as follows: two elements of R*are equal if corresponding digits are equal. Let
![]() (15)
(15)
Then,
![]() (16)
(16)
and, if x is any decimal we have,
![]() (17)
(17)
The trichotomy axiom follows from the lexicographic ordering of R*. This is the natural ordering sought by mathematicians among the real numbers but it does not hold there because there is no linear ordering of R according to the above counterexample.
(Dark number d* mathematically models the superstring, fundamental building block of matter [33] ).
4.5. Mathematical Duals
Mathematical systems are better understood by bringing in the notion of dual systems as it introduces symmetry that may be useful. We consider divergent sequences, i.e., sequences whose terms become bigger and bigger and indistinguishable from each other, as the dual of convergent sequences. In this sense they also form an algebraic continuum. We look at d* as the dual of u* and R* that of the system of additive and multiplicative inverses. Thus, R* is a semi-field, the nonzero integers forming a semi-ring since some of them have no multiplicative inverses. Like d*, u* cannot be separated from the decimals, i.e., there is no boundary between either of them and the decimals and between finite and infinite. Thus, there is no boundary to cross between finite and infinite and that beyond a certain finite decimal everything else is infinite. This is what is meant by the expression u* + x = u* for any decimal x. Duality is also seen in this case: let l> 1 be a terminating decimal then the sequence ln, ![]() diverges to u* but (1/l)n,
diverges to u* but (1/l)n, ![]() converges to d*, i.e.,
converges to d*, i.e.,![]() .
.
4.6. Isomorphism between the Integers and Decimal Integers
The decimal integers are decimals of the form,
![]() (18)
(18)
We note that 1 + 0.99… is not defined in R since 0.99××× is nonterminating but we can write 0.99××× = 1 − d* so that 1 + 0.99××× = 1 + 1 −d* = 2 −d* =1.99×××; we now define 1 + 0.99××× = 1.99××× or, in general, N ? d* = (N − 1).99××× Twin integers are pairs (N, (N − 1).99×××), N = 1, 2, ×××; their first and second components are isomorphic.
Let f be the mapping N → (N − 1). 99××× and extend it to the mapping d* → 0 even if d* is not a decimal; then we show that f is an isomorphism between the integers and decimal integers:
![]() (19)
(19)
Thus, addition of decimal integers is the same as addition of integers. Next, we show that multiplication is also an isomorphism.
![]() (20)
(20)
We have now established the isomorphism between the integers and the decimal integers (first established in [30] ) with respect to both operations so that both subspaces of R* are integers in the sense of [56] . We include in the isomorphism the map d* ® 0, so that its kernel is the set {d*, 1} from which follows,
![]() (21)
(21)
(The second equation of (21) can be proved also by mathematical induction for a given n).
We exhibit other properties of 0.99××× Let K be an integer, M.99××× and N.99××× decimal integers. Then
![]() (22)
(22)
![]() (23)
(23)
![]() (24)
(24)
To verify that 2(0.999×××) = 1.99×××, we note that (1.99×××)/2 = 0.99×××
![]() (25)
(25)
![]() (26)
(26)
4.7. Adjacent Decimals and Recurring 9s
Two decimals are adjacent if they differ by d*. Predecessor-successor pairs and twin integers are adjacent. For example, 74.5700××× and 74.5699××× are adjacent.
Since the decimals have the form N.a1a2×××an×××, N = 0, 1, 2, ×××, the digits are identifiable and, in fact, countably infinite and linearly ordered by lexicographic ordering. Therefore, they are discrete or digital and the adjacent pairs are also countably infinite. However, since their tail digits form a continuum, R* is a continuum with the decimals its countably infinite discrete subspace.
A decimal is called recurring 9 if its tail decimal digits are all equal to 9. For example, 4.3299… and 299.99××× are recurring 9s; so are the decimal integers. (In an isomorphism between two algebraic systems, their operations are interchangeable, i.e., they have the same algebraic structure but differ only in notation).
The recurring 9s have interesting properties. For instance, the difference between the integer N and its recurring 9, (N− 1).99…, is d*; such pairs are called adjacent because there is no decimal between them. In the lexicographic ordering the smaller of the pair of adjacent decimals is the predecessor and the larger the successor. The average between them is the predecessor. Thus, the average between 1 and 0.99××× is 0.99××× since (1.99×××)/2 = 0.99…; this is true of any recurring 9, say, 34.5799××× whose successor is 34.5800××× Conversely, the g-limit of the iterated or successive averages between a fixed decimal and another decimal of the same integral part is the predecessor of the former.
Since adjacent decimals differ by d* and there is no decimal between them, i.e., we cannot split d* into nonempty disjoint sets, we have another proof that d* is a continuum. The counterexample to the trichotomy axiom shows that an irrational number in the real number system cannot be expressed as limit of sequence of rationals since the closest it can get to it is some rational interval which still contains rationals whose relationship to it is unknown, another expression of the ambiguity of the concept irrational [4] .
The g-sequence of a nonterminating decimal reaches its g-limit, digit by digit, an advantage in computation with the g-norm. Moreover, a nonterminating decimal is an infinite series of its digits:
![]() (27)
(27)
4.8. R* and Its Subspaces
We add the following results to the information we now have about the various subspaces of R* to provide a full picture of the structure of R*. The next theorem is a definitive result on the continuum R*; it does not hold in R.
Theorem. In the lexicographic ordering R* consists of adjacent predecessor-successor pairs (each joined by d*); hence, the g-closure R* of R is a continuum.
However, the decimals form countably infinite discrete subspace of R* since there is a scheme for labeling them by integers. (An integer is a decimal with 0 decimal digits) We can imagine them as forming a right triangle with one edge horizontal and the vertical one extending without bounds. The integral parts are lined up on the vertical edge and joined together by their branching digits between the hypotenuse and the horizontal that extend to d* which is adjacent to 0 (i.e., differs from 0 by d*) at the vertex of the horizontal edge.
Corollary. R* is non-Archimedean but Hausdorff in both the standard and the g-norm and the subspace R of decimals are countably infinite, hence, discrete but Archimedean and Hausdorff.
Clearly, R coincides with the set of terminating decimals. The following is a theorem in R* [55] .
Theorem. Every real number is isolated from the rest.
This theorem, originally proved in R [39] , says that an irrational isnot the limit of a sequence of rationals in the standard norm contrary to traditional mathematics. Here is another surprise that contradicts a theorem in R.
Theorem. The rationals and irrationals are separated, i.e., they are not dense in their union (the first indication of discreteness of the decimals).
This theorem, proved in R [31] [55] shows, again, the inconsistency of R stemming from the ambiguity of irrational. The next theorem holds in R* but not in R.
Theorem. The largest and smallest elements of the open interval (0,1) are 0.99××× and d*, respectively [30] .
Theorem. An even number greater than 2 is the sum of two primes
This is the 274-year-old conjecture in the real number system called Goldbach’s conjecture [58] (first proved in [39] using earlier concepts of R*). Like Fermat’s equation, it is indeterminate and not resolvable in R.
Corollary. Every integer or terminating decimal has dark component inseparable from it.
4.9. Important Results; Resolution of a Paradox
We highlight some of the major results in R*.
1) Every convergent sequence has a g-subsequence defining a decimal adjacent to its standard limit. If the g-limit of a sequence is terminating then it coincides with its standard limit.
2) It follows from 1) that the standard limit of a sequence of terminating decimals can be found by evaluating the g-limit of its g-subsequence which is adjacent to it. This is an alternative way of computing the limit of ordinary sequence.
3) In [59] several counterexamples to the generalized Jourdan curve theorem for n-sphere are shown where a continuous curve has points in both the interior and exterior of the n-sphere, n = 2, 3, ×××, without crossing the n-sphere. This is a paradox in RN+1 about the general Jourdan curve theorem. Our explanation is: the functions cross the n-sphere through dark numbers. This has some implications for homotopy theorems in topology.
4) Given two decimals and their g-sequences and respective nth g-terms An, Bn we define the nth g-distance as the g-norm ![]() of the difference between their nth g-terms. Their g-distance is the g-lim
of the difference between their nth g-terms. Their g-distance is the g-lim![]() , as n ® ¥, which is adjacent to the standard norm of the difference. Advantage: the g-distance is the g-norm of their decimal difference; the difference between nonterminating decimals cannot be evaluated otherwise. Moreover, this notion of distance can be extended to n-space, n > 2, 3, ×××, and the distance between two points can be evaluated digit by digit in terms of their components without the need for evaluating roots. In fact, any computation in the g-norm yields the results directly, digit by digit, without the need for intermediate computation such as evaluation of roots in standard computation. (The decimals are “glued” together by d* to form the continuum R*).
, as n ® ¥, which is adjacent to the standard norm of the difference. Advantage: the g-distance is the g-norm of their decimal difference; the difference between nonterminating decimals cannot be evaluated otherwise. Moreover, this notion of distance can be extended to n-space, n > 2, 3, ×××, and the distance between two points can be evaluated digit by digit in terms of their components without the need for evaluating roots. In fact, any computation in the g-norm yields the results directly, digit by digit, without the need for intermediate computation such as evaluation of roots in standard computation. (The decimals are “glued” together by d* to form the continuum R*).
5) Every sequence of elements of R* bounded below has a greatest lower bound and every sequence of elements of R* bounded above has a least upper bound. This retrieves in R* a flawed theorem in R. It follows that R* is well ordered. This is not so for R. Moreover, if the standard limit of a sequence of decimals exists then it is constructible since it is adjacent to the g-limit of some g-sequence which is a decimal.
We have identified some ambiguous concepts of R, defined some of them in R* and discarded those that cannot be fixed, e.g., the irrationals. We also identified some theorems in R that are false in R*, e.g., that the fractions are dense in the real number system. In fact, all theorems there that rely on the axiom of choice are false, e.g. the Heine-Borel theorem and existence of nonmeasurable set [10] and compactness and completeness theorems. We resolve FLT by a counterexample that proves it false in R*. The formulation of FLT in R is ambiguous and has no solution because R is ambiguous. Therefore, its resolution requires the rectification of R, which is R* and its reformulation there. The reformulation simply requires that Fermat’s equation,
![]() (28)
(28)
be extended to R* so that the resolution of FLT will be done in R*.
4.10. The Counter Examples to FLT
Although it is sufficient to resolve this 379-year-old FLT by a counterexample, we shall show that there are countably infinite counterexamples to it.
Given the impossibility of proving a negative proposition or statement we use the negation of FLT, i.e. the existence of solution of Fermat’s Equation (28). That would be a counterexample to FLT. We summarize the properties of the digit 9.
1) A finite string of 9s differs from its nearest power of 10 by 1, e.g., 10100 − 99×××9 = 1.
2) If N is an integer, then (0.99×××)N = 0.99××× and, naturally, both sides of this equation have the same g-se- quence. Therefore, for any integer N, ((0.99×××)10)N = (9.99×××)10N.
3) ![]()
Then the exact solutions of Fermat’s equation are given by the triples![]() ,
, ![]() , that clearly satisfy Fermat’s equation (first proved in,
, that clearly satisfy Fermat’s equation (first proved in,
![]() (29)
(29)
for n = NT > 2. Moreover, for k = 1, 2, ×××, the triples (kx, ky, kz) also satisfy Fermat’s equation. They are the countably infinite counterexamples to FLT that prove the conjecture false [60] .
4.11. Advantages of the G-Norm
The advantages of the g-norm over other norms are as follows:
a) It avoids some indeterminate forms.
b) Since the g-norm of a decimal is the decimal itself, computation with it yields the answer directly, digit by digit, and avoids intermediate approximations of standard computation. It means considerable reduction in computer time for large computation.
c) Since the standard limit is adjacent to the g-limit of some g-sequence, evaluating it reduces to finding some nonterminating decimal adjacent to it; the decimal is approximated by the appropriate nth g-term. Both the com- putation and approximation are precise. In fact, the exact margin of error is d*. This applies to the result of any computation: it is adjacent to some nonterminating decimal and the latter is found using the g-norm.
d) In iterated computation along successive refinements of sequence xj that tends to a as j ® ¥, the iteration is simplified by taking averages between the sequence of points xj and its g-limit.
e) Approximation by the nth g-term or n-truncation contains the ambiguity of a nonterminating decimal.
f) Calculation of distance between two decimals with the g-norm is direct, digit by digit. It involves no root or radical at all.
g) In general radicals in computation, e.g., taking root of a prime, is avoided, by using the nth g-term approximation or n-truncation to any desired margin of error where accuracy is measured by the number of digits of the result obtained.
The g-norm is the natural norm for computation since a) it puts rigor in computation in accordance with the new definition of the previously ill-defined nonterminating decimals in terms of the defined terminating decimals, b) the margin of error is precisely determined and c) the result of computation is obtained digit by digit and avoids intermediate unnecessary calculation proceeds directly to the result digit by digit.
Constructivism requires that proof of existence alone is not sufficient. In the case of a normed space like the setting (mathematical space) for the rectified calculus of variations and optimal control theory the appropriate norm is the Young Measure [29] . For the constuctivist real number system, the g-norm is the appropriate one. At this time, the rectified mathematical spaces aside from the calculus of variations and optimal control theory are the new foundations of mathematics [4] , this constructivist real number system R* and the complex vector plane [61] . There are also new mathematics that form part of the mathematics of the grand unified theory which are constructivist, namely, the generalized integral [62] and the generalized physical fractal [38] . They constitute quite a tiny portion of mathematics. Thus, the immediate task for mathematicians and graduate students is quite huge: application of similar critique-rectification to their extensions to real and complex analysis and their foundations. Another big task is critique rectification of the other major fields of mathematics such as nonlinear analysis, algebra, topology and probability theory, to transform them to new mathematics.