Ferroelectricity in Layered Perovskites as a Model of Ultra-Thin Films ()
Received 22 February 2016; accepted 9 August 2016; published 12 August 2016

1. Introduction
Ferroelectric compounds exhibit many attractive physical properties such as piezoelectricity, pyroelectricity, high-dielectric constant, non-linear optical effects and bistable nature. Therefore various works have been focused on integration of ferroelectric thin films into devices such as ferroelectric nonvolatile memory (FRAM), metal-oxide-semiconductor field-effect transistors (MOSFETs) and sensor devices [1] - [5] . Although ferroelectrics were embedded as a functional element in the form of thin films, our concern is whether the ferroelectricty can persist even in ultra-thin films or not. Since the ferroelectricty originates fundamentally from electrostatic long-range force in dielectric media, it is important to know the exact size effect of thin films on ferroelectricity and the critical thickness where the ferroelectricity may disappear. However, various experiments of ferroelectric thin films have not been enough investigated because of its difficulty to grow a good quality of ultra-thin films and to estimate the effects of misfit strain due to their substrate. It is of fundamental interest in two- dimensional structure of ferroelectric thin films experimentally and theoretically since early times [6] . There have been many attempts to study the size effect on ferroelectricity, leading to a rich literature of both experimental and theoretical works [7] [8] . Especially, studies of ferroelectric perovskites are activated and are promised as one of the best sources of ferroelectric thin films since the perovskite oxides show prominent high dielectric constants and piezoelectric properties.
The similarity of dielectric and structural features has been observed between BaTiO3 thin films and Bi- layered perovskite compounds. This means that both materials have a common mechanism for ferroelectric activity. Among Bi-layered perovskites, SrBi2Ta2O9 (abbreviated as SBT) has been extensively studied as a candidate for ferroelectric non-volatile memory devices. Based on dielectric and structural similarities, it may be reasonable to consider that SBT is a good model for an ideal ferroelectric thin film, free from any misfit lattice strain. The close analogy between Bi-layered perovskites and ferroelectric thin films was pointed out simply in a previous paper [9] . In this review, we will discuss the ferroelectric stability in ultra-thin films in detail from the viewpoint of the nature of Bi-layered perovskites.
2. BaTiO3: Bulk Crystal and Thin Film
We will simply introduce fundamentals of barium titanate, BaTiO3, in the form of bulk crystal and thin films. Perovskite oxides have a basic structure of ABO3, where A and B are cations. This structure has been considered to be a prototype for many ferroelectrics just as for ferromagnetic, semiconducting and superconducting materials, depending on the composition. The first perovskite oxide showed ferroelectricity was BaTiO3, which is cubic with space group Pm3m in the high-temperature paraelectric phase. It undergoes a ferroelectric phase transition at 409.5 K (Tc) and has a tetragonal structure (P4mm) at room temperature. The characteristic features associated with this phase transition are known such as a large dielectric anomaly. The dielectric constant (ε) is over 14,000 at Tc (Figure 1) and follows the Curie-Weiss law, ε = C/(T − Tc) with C = 14,000 K [10] . The saturated spontaneous polarization (Ps) of the tetragonal phase is about 26 μC/cm2 along the tetragonal c-axis.
This phase transition has been explained by the so-called “soft mode theory” after Cochran where a transverse optic (TO) mode softens [11] [12] . The decrease in the frequency of TO mode (ωTO) towards Tc results in the large dielectric anomaly as we should remember the following LST (Lyddane-Sachs-Teller) relation,
![]()
Figure 1. Dielectric constant of BaTiO3 bulk crystal associated with a paraelectric-ferroelectric phase transition [10] . The recent Tc has been reported as 409.5 K in high quality sample as shown in Figure 4. The red line shows dielectric constants of SrBi2TaO9 bulk ceramics with Tc = 608 K [9] .
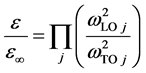 (1)
(1)
where ε, ε∞ are dielectric constant of static and at the frequency f = ∞. The ωLO and ωTO are frequencies of longitudinal optic and transverse optic modes of the j-th branch, respectively.
Recently, however, it has been recognized that the dielectric constant begins to decrease with decreasing its sample thickness below 2000 Å (Figure 2) [13] - [19] . The peak value of dielectric anomaly is only 380 at 1000 Å. Moreover, the dielectric constant shows broad and non Cure-Weiss behavior as in Figure 3 [13] - [19] . This evidence indicates that the well-established soft mode may be modified in the sense of lattice dynamics of ultra- thin films of BaTiO3.
![]()
Figure 2. Thickness dependence of dielectric constant of BaTiO3 thin films on Pt/MgO substrate at room temperature [13] - [19] . With decreasing thickness, the dielectric constant monotonically decreases from 700 (8000 Å thick) to 100. The solid line is a guideline. Detailed discussion is given in reference [17] .
![]()
Figure 3. Temperature dependence of dielectric constant of BaTiO3 thin films with thickness of 4000 Å and 1000 Å. Dielectric constant of SrBi2Ta2O9 (SBT) bulk ceramics is also referred for comparison where its Tc is shifted to that of BaTiO3 bulk crystal [13] - [19] . The dotted line is the Tc of BaTiO3 bulk crystal (Tc = 409.5 K). More detailed comparison is given in Figure 13.
The specific heat (Cp) shows a sharp and clear λ-type anomaly at Tc in bulk BaTiO3 [20] . In the case of thin films, it changes to a broad, small and characteristic one with decreasing film thickness [21] . The spontaneous polarization (Ps) is related to the anomalous specific heat (ΔCp) in the mean field theory as
 (2)
(2)
This suggests that the temperature dependence of an order parameter (spontaneous polarization Ps) looks to be weak and linear. The upper and lower temperatures of anomalous specific part are shown by short arrows in Figure 5(a). Following the above thermodynamic relation, Equation (2), Tc should be understood as the upper temperature, but it is rather difficult to determine Tc definitely for this film. For film with 60 Å thick, large hysteresis phenomena are observed as shown in Figure 5(b). The sharp anomalous specific heat observed in the single bulk crystal changes to diffuse one in thin films. It plays somewhat in different and diffusive manners from the usual mean-field behavior (Ps ~ (T − Tc)1/2). As pointed out by many researches, these novel ferroelectric properties are essentially due to the two-dimensionality in thin films.
![]()
Figure 4. Temperature dependence of specific heat of BaTiO3 bulk single crystal around the paraelectric-ferroelectric phase transition point Tc (409.5 K) [20] .
![]() (a) (b)
(a) (b)
Figure 5. Temperature dependence of specific heat of BaTiO3 epitaxial thin films on SrTiO3 substrate; (a) 2000 Å thick and (b) 60 Å thick [21] . The arrows show the direction of heating and cooling. The short arrows indicate the beginning and end temperature of anomalous specific heat in (a).
The similar weak dielectric nature has been pointed out in Bi-layered perovskite compounds [9] SrBi2Ta2O9 (SBT), which has been extensively studied as a candidate for ferroelectric non-volatile memory devices. As discussed in the next section, this similarity of crystal structure and dielectric properties between thin films of BaTiO3 and Bi-layered perovskites shows that both materials have a common mechanism resulted in dielectric nature. Moreover it suggests us that SBT may be a good model for an ideal ferroelectric thin film, though SBT itself is a bulk crystal.
3. Bi-Layered Perovskite
A series of layered perovskite compounds were discovered by Aurivillius in 1949 [22] . Among these compounds, the ferroelectric activities of Bi-layered perovskites were first discovered by Smolenskii et al. [23] . The general chemical formula of Bi-layered perovskites is Am−1Bi2BmO3m+3, which is rewritten as [Bi2O2]2+∙[Am−1BmO3m+1]2−. It consists of the regular staking of semiconducting [Bi2O2] slabs and perovskite-like octahedral [BO6] groups, as described by Haeni et al. [24] . Various A and B cations are allowed for these compounds such as A = Na+, K+, Ba2+, Ca2+, Pb2+, Sr2+, Bi3+, and B = Fe3+, Ti4+, Nb5+, Ta2+, W6+. For example, Bi2WO6 (m = 1), SrBi2Ta2O9 (m = 2) and Bi4Ti3O12 (m = 3) are well studied. Additional members can be generated by allowing the number of stacking (m) of perovskite-like group. The family of Bi-layered perovskites has the face-centered orthorhombic unit cell as shown in Figure 6.
The reported Bi-layered perovskites are summarized in Table 1 [25] [26] . The unit cell is highly anisotropic and pseudo two-dimensional as given in Table 2. The -O-B-O-B-O- linkage along the pseudo-tetragonal unique c-axis is interrupted by the existence of semiconducting Bi2O2 layers. The perovskite BO6 layers and Bi2O2 layers are linked by weak van der Waals interaction. This structure is similar to that of thin perovskite films with
![]()
Figure 6. Crystal structures of Bi-layered perovskites depending on stacking number (m) of perovskite-like groups. The atoms in Am−1Bi2BmO3m+3 within the unit cell are shown as green ball (A), red ball (B), yellow ball (O) and blue ball (Bi). The long axis is the c-axis. The crystal structure is orthorhombic but nearly tetragonal at room temperature. The BO6 are shown as octahedra. Bulk BaTiO3 with simple perovskite structure has been recognized as a structure with expanded Bi-layered perovskite with infinity perovskite units (m = ∞).
![]()
Table 1. Dielectric properties of layered perovskites (Bi2O2)2+(Am−1BmO3m+1)2−. *Ferroelectricity has not been confirmed.
![]()
Table 2. Crystal system and the existence of soft mode of typical Bi-layered perovskites.
m-layers. Among these compounds, Bi4Ti3O12 with m = 3 has a monoclinic structure (Pc) and a polarization along the a- and c-axis, which indicates that this crystal has an relatively strong interaction along the c-axis and shows the three-dimensional character. Therefore, compounds with m = 3 (Bi4Ti3O12, BaBi3Ti2NbO12, and PbBi3Ti2NbO12) are not included in the following discussion on two-dimensional nature, although Bi4Ti3O12 is an interesting ferroelectric compound for thin film application.
4. SrBi2Ta2O9 (SBT)
Strontium bismuth tantalate SrBi2Ta2O9 is the most important material in the application for FRAM, because of its low fatigue, low coercive field and Pb-free compound [1] [2] . The room-temperature structure is orthorhombic A21am (a = 5.531, b = 5.534, c = 25.984 Å), with 28 atoms in the unit cell. It consists of perovskite-type [SrTa2O7]2− groups (two layers of TaO6 octahedra) and semiconducting [Bi2O2]2+ layers. They are stacked alternately along the pseudo- tetragonal c-axis as shown in Figure 7 [27] - [33] . Ferroelectricity in SrBi2Ta2O9 with m = 2 was discovered below 608 K (Tc) by Smolenskii et al. in 1961 [23] . SrBi2Ta2O9 undergoes two phase transitions at 608 K and 850 K. This crystal shows small and broad dielectric anomaly around Tc where the maximum value is only 260 (Figure 8). A broad anomaly in specific heat has been observed as shown in Figure 9. This non Curie-Weiss like dielectric anomaly is observed not only in SrBi2Ta2O9 but also in other Bi-layered perovskites commonly.
The spontaneous polarization (Ps) is relatively large and 5.8 ~ 10 μC/cm2 along the a-axis (not along the pseudo-tetragonal c-axis) at room temperature [25] , while Ps = 26 μC/cm2 along the tetragonal c-axis in BaTiO3 [34] .
The high-temperature paraelectric phase is tetragonal with space group I4/mmm (a = 3.927, and c = 25.142 Å at 1000 K), where the TaO6 octahedra take antiparallel arrangements along the tetragonal c-axis [30] . The shape of TaO6 octahedron is not perfect and is elongated along the c-axis even in the paraelectric tetragonal phase, which means the TaO6 octahedon has a dipole moment. The Ta-O bond lengths owned commonly by adjacent octahedra along the tetragonal c-axis are a little bit short, and chemical bonds directed to Bi2O2-layer are long. In the ferroelectric phase, this crystal favors canted octahedral TaO6 arrangements below Tc. This structure results in the net spontaneous polarization along the a-axis and no polarization along the pseudo-tetragonal c-axis.
![]()
Figure 7. Crystal structure Bi-layered perovskite SrBi2Ta2O9, which consists of Bi2O2 semiconducting layers interleaved with TaO6 perovskite groups. This orthorhombic structure is nearly tetragonal with a ~ b. The c-axis is perpendicular to these stacking layers.
![]()
Figure 8. Small and broad dielectric behavior in SrBi2Ta2O9 around Tc. The peak value is only 260 and the half width of this anomaly is over 400 K.
![]()
Figure 9. Temperature dependence of specific heat of SrBi2Ta2O9 single crystal [33] .
The crystal structures of the high-temperature paraelectric phase at 1000 K and the ferroelectric phase at room temperature are schematically shown in Figure 10 [33] [35] - [38] . The TaO6 octahedra are distorted in both phases. Even in the high-temperature paraelectric phase, the octahedra are elongated along the pseudo-tetragonal c axis and locate in an antiparallel way. The distortion parameter of one TaO6 octahedron, p, defined as
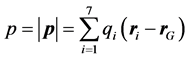 (3)
(3)
is estimated to be 1.365 debye along the c-axis as shown in Table 3, where qi and ri and are charge and position of each constituent ion, and rG is the gravity center of TaO6 [30] .
The parameter p is calculated as (0, 0, 1.365) in debye unit in the high-temperature paraelectric phase. In the ferroelectric phase, the absolute value is 1.682 debye, which is almost the same as that of the high-temperature paraelectric phase within errors. If the distortion of octahedron corresponds to a dipole moment, it should be probable that SrBi2Ta2O9 prefers an antiferroelectric structure along the c-axis, when the distorted octahedra are mainly responsible for the ferroelectric activity. Furthermore, these distorted octahedra have a canted arrangement in the ferroelectric phase as shown in Figure 10(a).
![]()
![]() (a) (b)
(a) (b)
Figure 10. Schematic crystal structures of (a) the ferroelectric (space group; A21am) and (b) the paraelectric phases (space group; I4/mmm) of SrBi2Ta2O9. Arrows show estimated dipole arrangements of TaO6 octahedra [27] .
![]()
Table 3. Estimated dipole moment of TaO6 octahedron of the ferroelectric phase at 300 K (A21am) and the high-tempera- ture paraelectric phase (I4/mmm) at 1000 K [27] .
5. Phase Transition in SrBi2Ta2O9
The structural arrangement of TaO6 octahedra in SBT reminds us canted-ferromagnets (or weak ferromagnets) discussed by Moriya [39] and in layered canted-ferromagnets by de Gennes [40] . The free energy is given in terms of sublattice polarizations P1, P2 and an angle θ between them as
![]() , (4)
, (4)
where the third symmetrical term is a well-known exchange interaction, which prefers an antiparallel configuration. The last term is an antisymmetric Dzialoshinski-Moriya interaction, which forces dipoles to tilt from the c-axis. These two terms are considered to be induced by the two-dimensional size effect of thin films. If θ = π and α1 = α2, the above expression is the same as that for antiferroelectrics proposed by Kittel [41] . Because of the last Dzialoshinski-Moriya term, the above free energy favors the canted arrangement of dipoles rather than antiferroelectric one as shown in Figure 10(a).
From the condition, ¶F/¶θ = 0, we get the following relation
![]() . (5)
. (5)
As the observed value of θ is 154˚ at room temperature, the coefficient δ of the antisymmetrical term is about half of the symmetrical term γ in this compound. From the relations
![]() , (6)
, (6)
we have an expression for the dielectric susceptibility as
![]() (7)
(7)
When we assume![]() , c is given as
, c is given as
![]() . (8)
. (8)
It is evident that the dielectric susceptibility shows a small cusp just like antiferroelectrics. As θ may vary from π to 154˚ with decreasing temperature, the coefficient γ' changes gradually. Dielectric constant may show a broad anomaly around Tc, which is smeared by two-dimensional surface strain as pointed out by Tagantsev et al. [42] . The weak dielectric behavior may be attributed to the two-dimensional effect, which favors for dipole moment to cant from the tetragonal axis in SrBi2Ta2O9.
6. Dielectric Behavior in Sr2Bi4Ti5O18 with m = 5
The phase transitions in Bi-layered perovskites with m = 5 have not been known in comparison to SrBi2Ta2O9. The crystal structure is orthorhombic with space group B2cb at room temperature (Figure 11) [43] . The high-temperature phase is believed to be tetragonal (I4/mmm). The dielectric measurement of Sr2Bi4Ti5O18 at frequencies of 1 kHz, 10 kHz and 100 kHz was shown in Figure 12 [44] . Two dielectric anomalies of Sr2Bi4Ti5O18 were found at 551 K (Tc) and 730 K (TH) although Mouri et al. reported dielectric anomalies at 428 K and 558 K [45] . The anomaly at Tc is almost the same as reported by Subbarao [25] . The successive phase transitions were reported also in Pb2Bi4Ti5O18 with m = 5 [45] [46] . Very little is known about dielectric behavior in Bi-layered perovskites, in particular, at high temperatures.
![]()
Figure 11. Crystal structure of Bi-layered perovskite Sr2Bi4Ti5O18 with m = 5 (half of the unit cell). The c-axis is perpendicular to the stacking layers of Bi2O2 semiconducting layer and perovskite-like groups.
The peak value of dielectric constant is about 1700 (100 kHz) which is one order larger than SrBi2Ta2O9 (ε ~ 260) but smaller than that of BaTiO3 (ε ~ 14,000). The rather sharp dielectric anomaly was observed in the case of Bi-layered perovskites with m = 5. This evidence is consistent with the Curie-Weiss behavior in soft mode of Sr2Bi4Ti5O18 discussed later.
7. Similarities of Dielectric Properties of BaTiO3 Ultra-Thin Film and SrBi2Ta2O9
Recently, Onodera et al. pointed out close similarities between Bi-layered perovskites and ferroelectric thin films, and discussed the ferroelectric instability in thin films with two-dimensionality [9] [47] . Figure 1 and Figure 13 show a comparison of dielectric behavior in bulk BaTiO3 crystal, BaTiO3 thin film (1000 Å) and Bi-layered perovskite SrBi2Ta2O9. The small and broad dielectric anomalies are commonly observed in BaTiO3 thin films and bulk SrBi2Ta2O9 crystal.
The decrease and non-clear behavior of dielectric constant were also observed around Tc with decreasing film thickness in (Ba, Sr)TiO3 thin films. In this case, Hwang analyzed this decrease by considering the effects of a finite charge-screening length of metal electrodes and an intrinsic dead layer of the surface [48] .
![]()
Figure 12. Dielectric constant of Sr2Bi4Ti5O18 at 1 kHz, 10 kHz and 100 kHz. Two clear anomalies were found at 551 K (Tc) and 730 K (TH).
![]()
Figure 13. The dielectric constant versus (T-Tc) of BaTiO3 thin films and bulk Bi-layered perovskite SrBi2Ta2O9.
As discussed previously, the two-dimensional crystal structures are clear in both crystals. The crystal structure of Bi-layered perovskites is highly anisotropic along the pseudo-tetragonal c-axis. The single crystal of SrBi2Ta2O9 is easily cleaved along the pseudo-tetragonal c-axis, because the semiconducting Bi2O2 layer interacts weakly with upper and lower perovskite-like groups by van der Waals interaction. These dielectric and structural evidences may suggest the common mechanism for dielectric properties and the appearance of ferroelectricity for two crystals.
Experiments of Bi-layered perovskites are much easier than those of ultra-thin films because Bi-layered perovskites are bulk crystals. In addition, Bi-layered perovskites are free from misfit strain between ultra-thin film and substrate. The thickness was controlled by the stacking number of perovskite layers, m, of Bi-layered perovskites. The analogy in crystal structure and dielectric behavior between BaTiO3 ultra-thin films and Bi-layered perovskites may give us a perspective for the size effect and the ferroelectric nature of ultra-thin films.
8. Soft Mode and Relaxation Behavior in Bi-Layered Perovskites
The soft modes of Bi-layered perovskites were studied extensively for Sr-compounds with m = 2, 4, 5, because Sr-compounds have a clear soft mode, while the exact crystal structures and phase transitions of this series of compounds have not been clarified yet.
8.1. Soft Mode in SrBi2Ta2O9 (m = 2)
The soft mode of SrBi2Ta2O9 has been studied by Raman scattering by several researchers [38] [49] - [52] . The square of soft mode frequency decreases toward to the highest phase transition temperature TH (850 K) and shows a clear anomaly at Tc (610 K). The soft mode exists in the ferroelectric phase and shows highly overdamped behavior near Tc, although dielectric constant does not show any clear anomaly at Tc. An anomaly was detected in the share strain c44 at 850 K [53] , which corresponds to the high-temperature phase transition at TH. The temperature dependence of![]() , the square of soft mode frequency, and the damping factor Γ of SrBi2Ta2O9 are shown in Figure 14. The soft mode frequency does not follow the Curie-Weiss law. This soft mode disappears at Tc, which means that this mode becomes Raman inactive above Tc. The extrapolated temperature where this soft mode vanishes is 860 K, just close to TH.
, the square of soft mode frequency, and the damping factor Γ of SrBi2Ta2O9 are shown in Figure 14. The soft mode frequency does not follow the Curie-Weiss law. This soft mode disappears at Tc, which means that this mode becomes Raman inactive above Tc. The extrapolated temperature where this soft mode vanishes is 860 K, just close to TH.
In low frequency region from 0.3 to 1.7 cm−1, the relaxation mode of SrBi2Ta2O9 was observed which is generally observed in order-disorder type ferroelectrics [53] . The increase in intensity of relaxation mode was observed with increasing temperature towards Tc. This means that the soft mode exists even in Bi-layered compound with two perovskite layers, although an order-disorder nature is induced additionally.
![]()
Figure 14. Temperature dependence of soft mode frequency and a normalized damping factor Γ(T)/Γ(TR.T.) in SrBi2Ti2O9 [38] [51] [52] . The phase transitions of SrBi2Ta2O9 are observed at 608 K (Tc) and 850 K (TH).
8.2. Soft Mode in SrBi4Ti4O15 (m = 4)
The crystal structure of Bi-layered perovskites with m = 4 is orthorhombic (A21am) at room temperature [54] . The rotation of TiO6 octahedra and displacement of Ti ions have been reported from their hypothetical tetragonal structure at high temperatures. The soft mode behavior of Bi-layered perovskites with m = 4 was studied systematically by Kojima [55] . The ferroelectric-like phase transitions have been reported at 803 K for SrBi4Ti4O15 and 1063 K for CaBi4Ti4O15 respectively although the detailed series of phase transitions have not been confirmed yet. Figure 16 shows the temperature dependence of the square of the frequency ![]() of underdamped soft modes [55] . It is found that this soft mode was observed up to about 580 K but showed softening towards Tc = 803 K as observed in SrBi2Ta2O9. Similar soft mode behavior was reported also in CaBi4Ti4O15.
of underdamped soft modes [55] . It is found that this soft mode was observed up to about 580 K but showed softening towards Tc = 803 K as observed in SrBi2Ta2O9. Similar soft mode behavior was reported also in CaBi4Ti4O15.
The existence of an additional intermediate phase may be possible also for this series of compounds. The lattice parameters show slight kinks around 600 K for SrBi4Ti4O15 and 750 K for CaBi4Ti4O15 [54] , although no detailed works have been done for phase transitions.
8.3. Soft Mode in Sr2Bi4Ti5O18 (m = 5)
The soft mode spectrum shows large temperature dependence at two temperatures, Tc (551 K) and TH (730 K) [56] ; the underdamped soft mode is clearly observed at room temperature, but changes to an overdamped one above 460 K. The extrapolation of soft mode frequency and line width shows an anomaly at TH. Moreover, an additional relaxation mode due to an order-disorder nature was observed which may be induced by the two- dimensionality in Sr2Bi4Ti5O18. In an order-disorder phase transition, the relaxation time diverges at Tc, and the HWHM (half width half maximum) becomes zero at Tc. This typical order-disorder character observed in Sr2Bi4Ti5O18 may be related to the layered structure, i.e. coupling of polarization fluctuation in intra-layer and inter-layer.
The similar behavior in soft modes is found in SrBi2Ta2O9, SrBi4Ti4O15 and Sr2Bi4Ti5O18 as shown in Figures 15-17. The plot of square frequencies of soft modes shows a change from the non Curie Weiss behavior to rather the Curie-Weiss like behavior. This tendency corresponds well to relatively large and sharp dielectric anomalies in SrBi4Ti4O15 and Sr2Bi4Ti5O18, with increasing the stacking layer m. Additional relaxation modes were observed in SrBi2Ta2O9 and Sr2Bi4Ti5O18. These evidences show that there are both displacive (soft mode) and order-disorder (relaxational) characters in Bi-layered perovskites. The effect due to an order-disorder character increases with decreasing m, which induce an extra intermediate phase between the high-temperature paraelectric and the low-temperature paraelectric phases. The nature of ferroelectricity in BaTiO3 has been investigated intensively since BaTiO3 has a simple ABO3 crystal structure and exhibits typical ferroelectric phase transitions. The soft mode theory has explained well dielectric properties [11] [12] . However, the order-disorder nature has
![]()
Figure 15. Temperature dependence of soft mode in SrBi4Ti4O15 and CaBi4Ti4O15 after Kojima [55] .
![]()
Figure 16. Temperature dependence of square frequency (red point) of soft mode and its line width (blue point) in Sr2Bi4Ti5O18 [56] . The solid line and red curve indicate the Curie-Weiss law and the universal scaling law, respectively.
![]()
Figure 17. Intensity and HWHM (half width half maximum) of relaxation mode in Sr2Bi4Ti5O18 [56] .
been reported in the critical region near Tc observed by diffuse scattering in BaTiO3 [57] - [59] . Recent NMR study also showed the coexistence of both order-disorder and displacive components in BaTiO3 in the tetragonal ferroelectric to cubic paraelectric transition [60] - [62] . The delicate balance between the soft mode behavior and relaxational order-disorder component will compete and modify dielectric properties and the sequence of phase transitions as observed in ultra-thin perovskite films and Bi-layered perovskites.
9. Ferroelectricity and Size Effect in Thin Films
Ferroelectricity in Bi-layered perovskites SrBi2Ta2O9, Sr2Bi4Ti5O18 does not appear along the pseudo-tetragonal c-axis but along the a-axis normal to the pseudo-tetragonal axis, while along the tetragonal c-axis in BaTiO3. Crystal structure analysis of SrBi2Ta2O9 shows that TaO6 octahedra is still distorted along the pseudo-tetragonal c-axis, and locate in antiparallel way even in the high-temperature paraelectric phase above 850 K. Although the -O-Ta-O-Ta-O- chain along the pseudo-tetragonal c-axis is interrupted by the existence of semiconducting Bi2O2 layers in SrBi2Ta2O9, the strongly correlated -O-Ta-O-Ta-O- chain should play an essential role for the appearance of ferroelectricity as those in BaTiO3 [63] .
The shape of octahedron is not a regular octahedron even in the high-temperature paraelectric phase; this indicates that Bi-layered perovskites are antiferroelectric in the high-temperature tetragonal phase. In the ferroelectric phase, this crystal favors canted octahedral arrangements below Tc, which results in the net spontaneous polarization along the a-axis. This situation is just the same as that reported in the case of weak ferromagnetic materials.
On the other hand, this pseudo-two-dimensional character of crystal structure is just suitable for fabrication of thin films. The spontaneous polarization (Ps) is relatively large (5.8 ~ 10 μC/cm2 along the a-axis at room temperature), while Ps = 26 μC/cm2 in a representative perovskite ferroelectric BaTiO3 along the tetragonal c-axis.
Ferroelectricity appears due to a delicate balance between long-range dipole-dipole interaction along the polar axis and short-range interaction. The typical dipolar correlation lengths for many ferroelectrics are Lc ~ 10 - 50 nm along the polar axis and La ~ 1 - 2 nm normal to the polar axis [64] . The needle-shaped correlation region is sketched in Figure 18, where La is several unit-cells, of the same order as the thickness of a 180˚ domain wall, and Lc is about 25 ~ 125 unit-cells in the case of BaTiO3. Therefore it is considered that the stability of the ferroelectricity may be affected by the thickness of the thin films.
Measurements of thickness dependence of ferroelectricity are generally not so easy, because of the preparation of good quality of thin films, additional surface effects such as depolarization fields and space-charge effects. These effects generally influence the ferroelectric behavior in thin films.
Tybell, Ahn and Triscone have examined the possibility of the existence of a critical thickness and showed the detection of the ferroelectricity in perovskite Pb(Zr0.2Ti0.8)3 films down to a thickness of 10 unit cells (40 Å) [65] . Bune et al. reported ferroelectric activity in ferroelectric polymer films with thickness of two layers (10 Å) and the near-absence of finite-size effects in these two-dimensional ferroelectrics which may be generated by coupling only within the plane of the film [66] . Recent ab initio studies have confirmed the possibility of retaining the ferroelectricity in ultra-thin films, and suggested the absence of the critical size effect [67] [68] .
On the other hand, Junquera and Ghosez reported first-principle calculations on a realistic model of perovskite thin films with metallic electrodes. They showed that BaTiO3 films with SrRuO3 electrodes have the critical thickness of 6 unit cells (~24 Å) and lose the ferroelectricity below this thickness, due to the depolarization field effect at the ferroelectric-metal interfaces (Figure 19) [69] .
Recent Raman scattering studies showed that ultra-thin BaTiO3 films grown commensurately on SrTiO3 substrate have a spontaneous polarization as thin as 4 unit cells (16 Å) [70] . The ferroelectric phase transition temperature Tc is shown as a function of BaTiO3 film thickness in Figure 20. Recent experimental and theoretical works showed the critical thickness is much smaller than those previously reported.
It should be pointed out that the close analogy of ferroelectric behavior between Bi-layered perovskite SrBi2Ta2O9 and thin film of so-called typical ferroelectric perovskite BaTiO3. The layered ferroelectric SrBi2Ta2O9 is a bulk crystal itself, but is considered to be a good example of ultra-thin ferroelectric model with
![]()
Figure 18. A sketch of ferroelectric correlation region in dipolar materials [64] .
![]()
Figure 19. First-principles calculations of the energy of BaTiO3 ferroelectric films with m unit cells as a function of the soft-mode distortion ξ [68] .
![]()
Figure 20. The plot of the ferroelectric phase transition temperature Tc versus film thickness of BaTiO3 films grown on SrTiO3 substrates, observed by Raman scattering [69] .
two monolayers of perovskite TaO6 units, free from any misfit lattice strain and interface charge layer with electrodes. We summarized recent dielectric properties of Bi-layered perovskites and discuss the analogy of these two types of ferroelectrics.
10. Critical Thickness
As Sr2Bi4Ti5O18 is a compound with five perovskite layers sandwiched by Bi2O2 semiconducting layers, it is considered as a model of thin film with five perovskite unit cells. While the ferroelectricity of thin films may be suppressed below six unit cells after Junquera and Ghosez [67] , Sr2Bi4Ti5O18 shows ferroelectricity along the a-axis, but not along the tetragonal c-axis. Similar dielectric properties have been observed in SrBi2Ta2O9 which have two perovskites. The spontaneous polarization appears along the a-axis and the dielectric constant shows rather weak temperature dependence. Even in the case of SrBi2Ta2O9 (m = 2) and Sr2Bi4Ti5O18 (m = 5), the soft mode still exists, although it becomes to be highly overdamped near Tc and additional relaxation modes appear.
According to the calculations of local field by Luttinger and Tisza [71] , the strong ferroelectric interaction between Ti (or Ta) ions and O ions appears along the tetragonal c-axis. Although the strongly correlated -O-Ti-O-Ti-O- chain plays an important role for the appearance of ferroelectricity in bulk BaTiO3 [63] , the -O-Ta-O-Ta-O- chain along the pseudo-tetragonal c axis is interrupted by the existence of semiconductor Bi2O2 layers in SrBi2Ta2O9. The strength of interaction between Ti ion and adjacent O ions is half, but antiferroelectric in the a-b plane after Luttinger and Tisza. However, the configuration of distortion parameter p in Figure 10 suggests us that the interaction is strong but antiferroelectric along the pseudo tetragonal c-axis, and ferroelectric along the a-axis in SrBi2Ta2O9. It is expected that a crossover of ferroelectric to antiferroelectric interaction will be realized in thin films of BaTiO3, considering the similarity of dielectric properties between SrBi2Ta2O9 bulk crystal and thin BaTiO3 films mentioned above. It might be expected that the canted arrangement of dipole moments may be induced even in thin BaTiO3 films by the two-dimensionality.
The suppression of ferroelectricity has been reported for nana-particles of BaTiO3 and PbTiO3, where the critical size is 20 ~ 30 unit cells [72] . However the dipole-dipole interactions within plane are retained and contribute to ferroelectric activity in the case of thin films.
11. Summary
The dielectric and structural analogy between Bi-layered perovskites and ferroelectric thin films suggests that the bulk Bi-layered ferroelectrics are a good model of ferroelectric ultra-thin films with a few layers of perovskite units, free from any misfit lattice strain with substrate and surface charges at the interface with electrodes. In ultra-thin perovskite films, ferroelectric interactions are still prominent and the octahedra prefer an antiferroelectric arrangement rather than ferroelectric one along the tetragonal or pseudo-tetragonal axis (normal to the plane). In the case of a few layers less than m = 6, octahedra have a dipole moment, though first principles calculation suggests the soft-mode distortion ξ = 0, i.e. a non-polar structure [68] . The soft mode may exist even in ultra-thin films but changes to be highly overdamped near the ferroelectric phase transition temperature Tc. Moreover an additional relaxation mode is induced, which means an occurrence of coupling with displacive (soft mode) and order-disorder (relaxation mode) nature in ultra-thin films. As Sr2Bi4Ti5O18 has perovskite-like groups less than six unit cells, it is a critical material whether the ferroelectricity persists or not. The mechanism mentioned above might be applicable for SrBi2Ta2O9 with two perovskite units (m = 2) and Sr2Bi4Ti5O18 with five perovskite groups (m = 5), because of the close similarity in dielectric behavior. Based on experiments on Bi-layered perovskites, it may be possible that there is no critical thickness for the appearance of ferroelectricity in ferroelectric thin films in principle.
NOTES
![]()
*Corresponding author.