Optimal Portfolios of an Insurer and a Reinsurer under Proportional Reinsurance and Power Utility Preference ()
Received 11 December 2015; accepted 25 December 2015; published 29 December 2015
Subject Areas: Financial Mathematics
1. Introduction
The first study on optimal reinsurance was done by Bruno de Finetti as pointed out by Centeno and Simões [1] . In his study, de Finetti analyses the optimal retention limit for quota-share proportion reinsurance policies, under one year period and infinite time horizon using mean-variance criteria. There have been several studies thereafter, on the effect of reinsurance on the ultimate probability of ruin (Gerber [2] ; Waters [3] ; Bowers et al., [4] , Centeno, [5] ; Hesselager, [6] ). Most of these earlier studies concentrated on the effect of reinsurance on the adjustment coefficient [1] .
Schimidli [7] considered the risk process modelled by Cramer-Lundberg model and found the optimal unlimited proportional reinsurance strategy that minimized the infinite time ruin probability. He proved the existence of a smooth solution of the corresponding Hamilton-Jacobi-Bellman equation as well as verification theorem. Some numerical examples with exponential, shifted exponential and Pareto claims were carried out. The corresponding problem has been also studied by Hipp and Vogt [8] for the case of dynamic XL reinsurance.
[7] considered a classical risk model and allowed investment into a risky asset modelled as a Black-Scholes model as well as (proportional) reinsurance in the Cramer-Lundberg set up and solved the problem on determination of optimal reinsurance and investment strategies that minimized probability of ruin using the Hamilton- Jacobi-Bellman approach. He found optimal levels of investment and reinsurance that minimized the ruin probability of only investment in risk asset.
Taksar and Markussen [9] extended the analysis of this study by proposing a diffusion model with investment and proportional reinsurance.
Hipp and Plum [10] considered a risk process modelled as a compound Poisson process. They seek to minimize the ruin probability of the risk process by choosing suitable investment strategies for the market index (risky asset). They computed the optimal strategy using the Bellman equation and proved the existence of a smooth solution and a verification theorem, and give explicit solution in some cases with exponential claim size distribution, as well as numerical results in a case with Pareto claim size. This problem had been also studied by Browne [11] for the case then the insurance business is modelled by Brownian motion with drift, and the risky assert is modelled by geometric Brownian motion.
Liu and Yang [12] extended the model studied by [10] by including risk-free asset. They assumed that the insurance company receives premiums at constant rate and that it can invest in the money market and in a risky asset. They investigated behavior of various claim-size distributions numerically and computed the optimal investment strategy and the solution of the associated HJB equation under each assumed claim-size distribution. They also consider the effect of changes in various factors, like stock volatility, on the optimal investment strategies and survival probability.
Castillo and Parrocha [13] considered an insurance company with the fixed amount available for investment in a portfolio consisting of one risky assert and one risk-free asset and gave a numerical algorithm for solving the resulting HJB equation.
Irgens and Paulsen [14] considered the problem of maximizing the expected utility of the assets of an insurance company at a terminal time by reinsurance and investments into a diffusion-perturbed classical risk process. In their study they assumed that the company is allowed to invest its surplus in either a risk-free asset or a risky one.
Paulsen et al. [15] considered the diffusion perturbed classical risk process compounded by a linear Brownian motion and allowed for stochastic return on investments and presented sufficient conditions for the survival probability function to be four times continuously differentiable, which in particular implies that the survival probability is the solution to a second order integro-differential equation. Transforming this equation into an ordinary Volterra-integral equation of the second kind, they analyzed properties of its numerical solution by applying the order-four block-by-block method in conjunction with Simpson’s rule. Their study only allows investments and does not incorporate any type of reinsurance.
Paulsen [16] studied the ruin models with investment income. This survey treats the problem of ruin in a risk model when assets earn investments. In addition to a general presentation of the problem, the study also presented the relevant integro-differential equations, exact and numerical solutions, asymptotic results, ruin probabilities in the presence of investments and possibly reinsurance. In particular, the study considered the case where the insurer has possibility of purchasing a proportional reinsurance contract as well as investing in both risk-free and risky asset with the purpose of minimizing probability of ruin. The main emphasis was on continuous time models, though discrete time models were also covered.
Meng and Zhang [17] considered an insurance company whose surplus is modelled by Brownian motion with drift and that the surplus can be invested in a risky or nonrisk asset with the objective of minimizing the probability of ruin of insurer. They formally established that XL reinsurance treaty is optimal among the class of plausible reinsurance treaties. They also obtained optimal retention level as well as providing an explicit expression of the minimal probability of ruin.
Kasumo [18] considered a diffusion-perturbed risk process incorporated with proportion reinsurance and investment process of Black-Scholes type. The main purpose was to determine the role of investment and proportional reinsurance on the minimization of probability of ultimate ruin of an insurance company. The HJB equation for this problem was derived and the corresponding Volterra-integral differential equation which was then transformed into a linear Volterra integral equation of the second kind. He solved the integral equation using block-by-block numerical method for the retention percent that minimizes the probability of ultimate ruin.
Mata [19] studied the excess of loss reinsurance and reinstatement problem and provide a methodology to calculate the distribution of the aggregate losses for two or more consecutive Layers when there are a limited number of reinstatements.
In this study we consider the risk reserve of an insurer and a reinsurer to follow Brownian motion with drift and tackled their portfolio optimization problem. The optimized values of the insurer and the reinsurer are calculated. Also calculated are the insurer’s and the reinsurer’s optimal investment in the risky asset and then the discount value,  , that would warrant reinsurance.
, that would warrant reinsurance.
To make for clear understanding of this work, we defined the following few terms;
Insurance: Insurance is an arrangement by which a company gives customers financial protection against loss or harm such as theft or illness in return for a payment called a premium (Encarta World English Dictionary [20] ).
Reinsurance: This is the transfer of risk from a direct insurer (the cedent) to a second insurance carrier (the reinsurer). It may also be defined as insurance for insurers. It serves the purpose of offering protection to cedents against very large individual claims or fluctuations in their aggregate portfolio of risks, as well as diversifying the financial losses caused by it [1] .
Risk: This is the probability of loss to an insurer or the amount that an insurer is in danger of losing [20] .
Optimal portfolio: An optimal portfolio is a portfolio in which the risk-reward combination is such that it yields the maximumreturns (provides the highest utility) possible under the current and anticipated circumstances. Its mathematical formulation was provided the University of California's noble laureate economist Harry Markowitz (born 1927) in 1952.
Portfolio reinsurance: The practice whereby an insurer transferssome or all of the risk attached to a portfolio to another insurer, or reinsurer. Insurers use portfolio reinsurance to reducethe risk of having to pay large claims in the event of significant losses to the value of the portfolio.
2. Model Formulation and the Model
Suppose the claim process  of an insurance company is described by;
of an insurance company is described by;
 , (1)
, (1)
where a and b are positive constant and  a standard Brownian motion defined on a complete probability space
a standard Brownian motion defined on a complete probability space .Assuming also that the premium rate is;
.Assuming also that the premium rate is;
 (2)
(2)
with safety loading (security risk premium) .
.
Using equation (1), the surplus process of the insurer is given by;
 (3)
(3)
The insurance company has the permission to purchase proportional reinsurance to reduce her risk and pays reinsurance premium continuously at the rate of  where
where  is safety loading of the reinsurer and
is safety loading of the reinsurer and 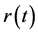 is the proportion reinsured at time t.
is the proportion reinsured at time t.
The surplus of the insurance company is then given as;
 , (4)
, (4)
for the insurer, and
 , (5)
, (5)
for the reinsurer, (Danping et al. [21] ).
Assuming that the insurer and reinsurer invest their surplus in the same market consisting of two assets: a risky asset (stock) and a riskless asset (bond) which rate of return is a linear function of time, let the prices the riskless and be risky assets  and
and ![]() respectively, then, the equations governing the dynamics of the dynamics of the riskless asset and the risky asset are given by stochastic differential equations;
respectively, then, the equations governing the dynamics of the dynamics of the riskless asset and the risky asset are given by stochastic differential equations;
![]() (6)
(6)
and
![]() , (7)
, (7)
(Osu and Ihedioha, [22] [23] ), respectively.
![]() and
and ![]() denote the appreciation rate (mean) and the volatility of the risky asset, respectively.
denote the appreciation rate (mean) and the volatility of the risky asset, respectively. ![]() is another standard Brownian motion defined on
is another standard Brownian motion defined on ![]() and,
and,
![]() . (8)
. (8)
Both the insurer and there insurer hold the risky asset as long as.
![]() . (9)
. (9)
Let ![]() represent the amount invested in the risky asset at time t by the insurer and
represent the amount invested in the risky asset at time t by the insurer and ![]() the amount invested in the risky asset at time t by there insurer. For the insurer, there insurance-investment strategy
the amount invested in the risky asset at time t by there insurer. For the insurer, there insurance-investment strategy ![]() is called admissible if it is
is called admissible if it is ![]() -progressively measurable and satisfies,
-progressively measurable and satisfies, ![]() , that is;
, that is;
![]() , (10)
, (10)
and for the reinsurer the strategy ![]() is called admissible if it is
is called admissible if it is ![]() -progressively measurable and satisfies,
-progressively measurable and satisfies,![]() , that is;
, that is;
![]() (11)
(11)
Assume that ![]() and
and ![]() are the total wealth of insurer and the reinsurer, respectively, then their investments in the riskless asset are
are the total wealth of insurer and the reinsurer, respectively, then their investments in the riskless asset are ![]() and
and![]() , respectively.
, respectively.
For the corresponding admissible strategies,![]() and
and![]() , and the policy
, and the policy![]() , the wealth processes of the insurer and the reinsurer evolve according to the stochastic differential equations(SDEs);
, the wealth processes of the insurer and the reinsurer evolve according to the stochastic differential equations(SDEs);
![]() , (12)
, (12)
for the insurer, and
![]() , (13)
, (13)
for the reinsurer (Wokiyi, [24] ).
Substituting the expressions for, ![]() ,
, ![]() ,
, ![]() , and
, and![]() , in Equations (12) and (13) we get;
, in Equations (12) and (13) we get;
![]() (14)
(14)
for the insurer and;
![]() (15)
(15)
for the insurer.
The quadratic variations of the wealth processes of the insurer and the reinsurer are;
![]() (16)
(16)
![]() (17)
(17)
Suppose the investor has a power utility function, the Arrow-Pratt measure of relative risk aversion (RRA) or coefficient of relative risk aversion is defined as;
![]() , (18)
, (18)
where w is the wealth level of an investor. The special case being considered is where the utility function is of the form,
![]() (19)
(19)
which has a constant relative risk aversion parameter![]() , the investors’ (the insurer and the reinsurer) problem can therefore be written as:
, the investors’ (the insurer and the reinsurer) problem can therefore be written as:
![]() (20a)
(20a)
where
![]() (20b)
(20b)
and ![]() a generator which in our case shall be derived via Ito lemma,
a generator which in our case shall be derived via Ito lemma,
Subject to:
![]()
for the insurer and;
![]()
for the reinsurer.
3. The Optimization
3.1. The Case of the Insurer
The theorem that follows gives the optimization of the insurer’s wealth;
Theorem 1: The optimal policy that maximizes the expected power utility at terminal time T is to invest at each time![]() ;
;
![]() (21)
(21)
with optimal proportion reinsured,
![]() (22)
(22)
and value function;
![]() (23)
(23)
where ![]() is a suitable function such that at the terminal time T,
is a suitable function such that at the terminal time T,
![]() (24)
(24)
Proof:
We derive the Hamilton-Jacobi-Bellman (HJB) partial differential equation starting with the Bellman equation:
![]() (25)
(25)
where ![]() denotes the wealth of the insurer at time
denotes the wealth of the insurer at time![]() .
.
Rewriting Equation (25) as,
![]() ,
,
and dividing both sides of the equation by ![]() and taking limit as
and taking limit as ![]() tends to zero, the Bellman equation becomes;
tends to zero, the Bellman equation becomes;
![]() (26)
(26)
Ito’s lemma (Miao, [25] ), which states that;
![]() . (27)
. (27)
For the insurer, substituting in the Ito’s lemma for ![]() and
and ![]() using Equations (14) and (16), we obtain differential stochastic equation (SDE):
using Equations (14) and (16), we obtain differential stochastic equation (SDE):
![]() (28)
(28)
Equation (28) simplifies to;
![]() (29)
(29)
Applying (29) to the Bellman Equation (26) and taking expectation, we get the HJB equation;
![]() (30)
(30)
where,
![]() , (31)
, (31)
satisfying terminal condition,
![]() . (32)
. (32)
Observing the homogeneity of the objective function, the restriction and the terminal condition, we conjecture that the value function V must be linear to![]() .
.
Let
![]() (33)
(33)
be such a value function, such that at the terminal date, T
![]() , (34)
, (34)
then
![]() (35)
(35)
Substituting Equation (35) into Equation (30), we obtain; the new H-J-B equation,
![]() (36)
(36)
To obtain the optimal value ![]() of
of![]() , we differentiate (36), with respect to
, we differentiate (36), with respect to ![]() and evaluate to obtain:
and evaluate to obtain:
![]() . (37)
. (37)
This simplifies to;
![]() . (38)
. (38)
This is the insurance company’s optimal investments in the risky asset, stock, that is both horizon and wealth dependent.
Also, differentiating Equation (36) with respect to ![]() and simplifying gives the optimal proportion of reinsured as;
and simplifying gives the optimal proportion of reinsured as;
![]() . (39)
. (39)
This reduces to;
![]() (40)
(40)
The solution of the HJB Equation (36) is thus; replacing ![]() and
and ![]() with their corresponding optimal values
with their corresponding optimal values ![]() and
and ![]() as in (38) and (40) respectively and rearranging, we obtain;
as in (38) and (40) respectively and rearranging, we obtain;
![]() (41)
(41)
From which we obtain,
![]() . (42a)
. (42a)
Since t is the dominating variable, as implied in our choice of![]() , therefore, we have;
, therefore, we have;
![]() , (4.2b)
, (4.2b)
where
![]() (43)
(43)
The integral of the differential equation of the function g is obtained as;
![]() ,
,
where ![]() and
and![]() .
.
That is;
![]()
![]()
![]() . (44)
. (44)
Applying the terminal condition, ![]() ,
,
![]() . (45)
. (45)
This implies that the horizon dependent solution to the insurance company’s investment problem is:
![]() . (46)
. (46)
This is the maximized expected power utility value at time t under optimal investment policy.
3.2. The Case of the Reinsurer
For the reinsurer, we state the following theorem 2.
Theorem 2: The optimal policy to maximize the expected power utility at T is to invest at each time![]() ;
;
![]() (49)
(49)
with optimal proportion reinsured,
![]() (50)
(50)
and value function;
![]() (51)
(51)
where ![]() is a suitable function.
is a suitable function.
Proof:
Adopting Equations (25) to (35) and replacing![]() ,
, ![]() , and
, and ![]() with their respective equivalents,
with their respective equivalents, ![]() ,
, ![]() , and
, and ![]() in Equation (36) we obtain the HJB equation;
in Equation (36) we obtain the HJB equation;
![]() (52)
(52)
which reduces to;
![]() (53)
(53)
To obtain the optimal investment in the risky asset, Equation (53) is differentiated with respect to![]() , thus;
, thus;
![]() . (54)
. (54)
Solving for ![]() in equation (54) gives the required optimal value;
in equation (54) gives the required optimal value;
![]() . (55)
. (55)
The differentiation of (53) with respect to ![]() and simplifying gives the optimal proportion reinsured as;
and simplifying gives the optimal proportion reinsured as;
![]() (56)
(56)
From equation (55) which when reduced gives;
![]() (57a)
(57a)
where,
![]() (57b)
(57b)
the definite integration within ![]() gives;
gives;
![]()
![]()
![]() . (58)
. (58)
Applying the terminal condition, ![]() ,
,
![]() . (59)
. (59)
This implies that the horizon dependent solution to the insurance company’s investment problem is:
![]() . (60)
. (60)
3.3. The Equality of the Insurer’s and the Reinsurer’s Strategies
Here we find the condition under which the proportion reinsured by the Insurer equals the amount accepted to be insured by the Reinsurer.
Therefore, we equate the values of ![]() in both cases and solve for the discount ratio
in both cases and solve for the discount ratio![]() .
.
That is;
![]() . (61)
. (61)
This reduces to;
![]() . (62a)
. (62a)
where;
![]() (62b)
(62b)
Therefore,
![]() , (63)
, (63)
where, wr and wi are the Reinsurer’s and the Insurer’s portfolio weights in the risky asset, respectively.
Clearly, the optimal policies that maximize the expected power utility and the value functions for both the insurer and the Reinsurer are horizon dependent.
4. Conclusions
In this study, we consider the optimal investment problem for both an insurer and a reinsurer. The basic claim process is assumed to follow a Brownian motion with drift and the Insurer could purchase proportional reinsurance from the Reinsurer.
The Reinsurer and the Insurer were allowed to invest in a risky and a risk-free assets and expressions for their optimal portfolios obtained solving the corresponding HJB equations. The discount value, ![]() , that would warrant reinsurance, according to the optimal reinsurance proportion chosen by the insurer was obtained.
, that would warrant reinsurance, according to the optimal reinsurance proportion chosen by the insurer was obtained.