A Basis for Causal Scattering Waves, Relativistic Diffraction in Time Functions ()
Received 3 March 2016; accepted 18 June 2016; published 22 June 2016

1. Introduction
Similarities between optics and quantum mechanics have long been recognized. One example of this symmetry was obtained by Moshinsky [1] who addressed the following non-relativistic, quantum, 1D shutter problem. Consider a monoenergetic beam of free particles moving parallel to the x-axis. For negative times, the beam is interrupted at  by a perfectly absorbing shutter perpendicular to the beam. Suddenly, at time
by a perfectly absorbing shutter perpendicular to the beam. Suddenly, at time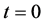 , the shutter is opened, allowing for
, the shutter is opened, allowing for 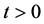 the free time-evolution of the beam of particles. What is the transient density observed at a distance x from the shutter? The shutter problem implies solving, as an initial value problem, the time-dependent Schrödinger equation with an initial condition given by
the free time-evolution of the beam of particles. What is the transient density observed at a distance x from the shutter? The shutter problem implies solving, as an initial value problem, the time-dependent Schrödinger equation with an initial condition given by
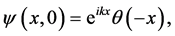 (1)
(1)
where 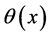 denotes the step function defined as:
denotes the step function defined as: 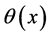 = (1 if
= (1 if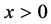 ) or (0 if
) or (0 if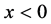 ). For
). For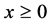 , Moshinsky proves that the free propagation of the beam has the exact solution given by:
, Moshinsky proves that the free propagation of the beam has the exact solution given by:
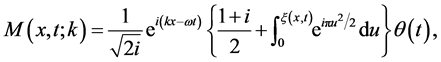 (2)
(2)
where the integral is the complex Fresnel function: 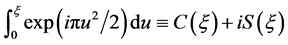 and
and  is given by
is given by
 . For
. For  the probability density
the probability density  is then
is then
![]() (3)
(3)
The right-hand side in Equation (3) is similar to the mathematical expression for the light intensity in the optical Fresnel diffraction by a straight edge [2] . For a fixed position![]() , the plot of the probability density
, the plot of the probability density ![]() as a function of time is shown in Figure 1.
as a function of time is shown in Figure 1.
These temporal oscillations are a pure quantum phenomenon, and similar oscillations arise at the moment of closing and opening gates in nanoscopic circuits [3] . With adequate potentials added to the model, it has been used to study transient dynamics of tunneling matter waves [4] - [7] , and the transient responses to abrupt changes of the interaction potential in semiconductor structures and quantum dots [8] [9] . For a review on the subject see [10] [11] . There is, in summary, a strong motivation for a thorough understanding of transient time oscillation in beams of matter.
One of the main problems in physics is to find, for the S matrix of an interaction, restrictions which proceed from general principles such as causality [12] . Notice that there is a close relation between diffraction in times wave functions and those wave functions which are needed for a causal description. From Equation (2) we see that for ![]() the wave function
the wave function ![]() is causal and the shutter solution can then be used as a basis
is causal and the shutter solution can then be used as a basis
function for causal scattering. Indeed, for an arbitrary function, ![]() , and assuming an initial
, and assuming an initial
condition given by:
![]() (4)
(4)
then the free time evolution of the initial condition becomes
![]() (5)
(5)
It is evident that if we want a relativistic solution for![]() , we need, instead of
, we need, instead of![]() , the corresponding relativistic solution to the shutter problem.
, the corresponding relativistic solution to the shutter problem.
As far as we know nobody has ever reported the exact relativistic solution to the shutter problem. Moshinsky worked this problem and gave an approximated answer. In a couple of articles [13] [14] , he discussed the shutter problem using the Klein-Gordon and the Dirac equations. Using approximated solutions Moshinsky arrives to the conclusion that only for the Schrödinger equation the wave function ![]() does resemble the expression that appear in the optical theory of diffraction. In his conclusions [13] , Moshinsky emphatically denies the existence
does resemble the expression that appear in the optical theory of diffraction. In his conclusions [13] , Moshinsky emphatically denies the existence
![]()
Figure 1. Probability density for non-relativistic diffraction in time.
of diffraction in time in the relativistic case. In the case of photons this is obviously true, the d’Alembert’s solution does not allow such time oscillations. However, for particles with mass different from zero, in full disagreement with Moshinsky’s conclusions, we report here that relativistic diffraction in time oscillations is indeed present.
The purpose of the present paper is to derive the exact solutions for the Dirac and Klein-Gordon shutter problems. The exact transient Dirac spinors are expressed in terms of integral-defined-functions which are the relativistic equivalent of the Fresnel integrals. In partial agreement with Moshinsky’s conclusions we find that indeed the relativistic densities do not resemble the mathematical expression for intensity of light that appears in the theory of diffraction in Optics. In spite of this, when our exact relativistic densities are plotted versus time, the plots show transient oscillations which resemble a diffraction pattern. For this reason in this article we claim that impressive diffractions in time oscillations do exist in the relativistic realm. Furthermore, the Dirac and Klein-Gordon densities look quite different, which implies that relativistic diffraction in time distinguishes between spin 0 and 1/2.
2. The Dirac Shutter Problem
Consider, for relativistic particles of spin 1/2, the shutter problem. We want to find out the spinor wave function ![]() which is the solution of the one-dimensional Dirac equation:
which is the solution of the one-dimensional Dirac equation:
![]() (6)
(6)
where ![]() is the
is the ![]() Pauli matrix and
Pauli matrix and ![]() the Compton length. The initial condition corre- sponds, for
the Compton length. The initial condition corre- sponds, for![]() , to a plane wave to the left of the shutter and zero to the right. Three quantum numbers are needed to classify the Dirac free particle solutions, namely, the momentum
, to a plane wave to the left of the shutter and zero to the right. Three quantum numbers are needed to classify the Dirac free particle solutions, namely, the momentum![]() , the positive or negative energies
, the positive or negative energies![]() , where
, where![]() , and helicity
, and helicity![]() . We select the initial condition assuming a positive energy
. We select the initial condition assuming a positive energy ![]() and a plane wave propagating along the z direction
and a plane wave propagating along the z direction![]() . As for the initial helicity,
. As for the initial helicity,
![]() (7)
(7)
we choose the initial state with a well defined direction of spin, for instance parallel to the direction of motion,![]() . Then in the shutter problem we have an incident plane wave given by
. Then in the shutter problem we have an incident plane wave given by
![]() (8)
(8)
For free particles, the helicity ![]() is a constant of motion. The initial direction of spin,
is a constant of motion. The initial direction of spin, ![]() , will be conserved at all positive times. As a consequence the two components of the wave function (
, will be conserved at all positive times. As a consequence the two components of the wave function (![]() and
and![]() ) which are zero at the initial time will remain zero at all positive times:
) which are zero at the initial time will remain zero at all positive times:
![]() (9)
(9)
In terms of the remaining two components ![]() the Dirac shutter problem is the solution of the equation,
the Dirac shutter problem is the solution of the equation,
![]() (10)
(10)
with the initial condition:
![]() (11)
(11)
where![]() . The normalization factor N is chosen as
. The normalization factor N is chosen as![]() ; in this way the probability density
; in this way the probability density![]() and the density current
and the density current ![]() transform initially, as a four-vector
transform initially, as a four-vector![]() .
.
We use the Compton length ![]() to define dimensionless variables:
to define dimensionless variables:![]() ,
, ![]() ,
, ![]() , and
, and![]() . Using these variables we derive in Appendix A the exact solution of the Dirac shutter problem. To simplify the notation, for n integer, we denote by
. Using these variables we derive in Appendix A the exact solution of the Dirac shutter problem. To simplify the notation, for n integer, we denote by ![]() the integral-defined complex functions
the integral-defined complex functions
![]() (12)
(12)
where ![]() is the Bessel function of the first kind of order n. Notice that
is the Bessel function of the first kind of order n. Notice that ![]() is a removable singularity for
is a removable singularity for![]() , hence the functions
, hence the functions ![]() are analytic. In fact the integrand can be explicitly written analytic by eliminating the denominator. Indeed, using repeatedly the recurrence relation for the Bessel functions,
are analytic. In fact the integrand can be explicitly written analytic by eliminating the denominator. Indeed, using repeatedly the recurrence relation for the Bessel functions, ![]() , we we can write
, we we can write
![]() (13)
(13)
Clearly the real and imaginary parts of ![]() are analytic oscillating functions.
are analytic oscillating functions.
From Appendix A, for the right side of the shutter![]() , we have the exact Dirac shutter solution:
, we have the exact Dirac shutter solution:
![]() (14)
(14)
Notice the function ![]() which shows the relativistic condition that no wave function exists until
which shows the relativistic condition that no wave function exists until![]() . This property is missing in the Schrödinger solution.
. This property is missing in the Schrödinger solution.
3. Dirac Diffraction in Time
Given the Dirac wave function ![]() in Equation (14), we can calculate the probability density
in Equation (14), we can calculate the probability density ![]() given by
given by
![]() (15)
(15)
In Figure 2, for fixed values of ![]() (
(![]() ) and
) and![]() , we show a typical plot of the Dirac density
, we show a typical plot of the Dirac density![]() . Surprisingly we find damped oscillations which resemble the Schrödinger diffraction in time oscillations. For this reason we call this plot a relativistic diffraction in time process. However, the Dirac oscillations are clearly different from the Schrödinger ones (see Figure 1). For
. Surprisingly we find damped oscillations which resemble the Schrödinger diffraction in time oscillations. For this reason we call this plot a relativistic diffraction in time process. However, the Dirac oscillations are clearly different from the Schrödinger ones (see Figure 1). For ![]() notice the impressive double oscillations which are unique to the Dirac theory.
notice the impressive double oscillations which are unique to the Dirac theory.
![]()
Figure 2. Dirac diffraction in time probability densities ![]() and
and![]() .
.
As expected, for a relativistic solution, the Dirac density vanishes for times![]() . The down oscillation, immediately following,
. The down oscillation, immediately following, ![]() , is also a relativistic property.
, is also a relativistic property.
4. The Klein-Gordon Shutter Problem
For relativistic particles with spin 0, the Klein-Gordon shutter problem is, by definition, the solution ![]() of the equation:
of the equation:
![]() (16)
(16)
where![]() . The initial conditions correspond to a plane wave to the left of the shutter and zero to the right.
. The initial conditions correspond to a plane wave to the left of the shutter and zero to the right.
![]() (17)
(17)
where![]() . Therefore at
. Therefore at![]() , when the shutter is suddenly opened, we have the initial con-
, when the shutter is suddenly opened, we have the initial con-
ditions:
![]() (18)
(18)
Similar to the Dirac problem, in terms of the dimensionless variables:![]() ,
, ![]() ,
, ![]() , and
, and![]() , we find the exact solution of this Klein-Gordon problem in Appendix B. At a fixed distance
, we find the exact solution of this Klein-Gordon problem in Appendix B. At a fixed distance![]() , on the right side of the shutter, we have the exact Klein-Gordon shutter solution:
, on the right side of the shutter, we have the exact Klein-Gordon shutter solution:
![]() (19)
(19)
or in simplified notation
![]() (20)
(20)
The presence of ![]() means, as expected, that the wave function vanishes for
means, as expected, that the wave function vanishes for![]() , where z is the distance from shutter to the particle detector.
, where z is the distance from shutter to the particle detector.
Given the Klein-Gordon wave function![]() , we have a charge density given by (charge
, we have a charge density given by (charge![]() ),
),
![]() (21)
(21)
![]()
Figure 3. Typical Klein-Gordon diffraction in time for the charge density,![]() .
.
In Figure 3, for fixed values of ![]() and
and![]() , we show a typical plot of the charge density versus time for the Klein-Gordon solution. The impressive damped oscillations, shown in Figure 3, clearly resemble the optical Fresnel diffraction pattern by an straight edge. The double oscillation which is present in the Dirac solution is missing now.
, we show a typical plot of the charge density versus time for the Klein-Gordon solution. The impressive damped oscillations, shown in Figure 3, clearly resemble the optical Fresnel diffraction pattern by an straight edge. The double oscillation which is present in the Dirac solution is missing now.
Notice that the asymptotic behavior of ![]() is not 1, as it occurs in the Schrödinger solution. In the particular case of
is not 1, as it occurs in the Schrödinger solution. In the particular case of ![]() and
and ![]() (
(![]() ) shown in Figure 3, the stationary density is
) shown in Figure 3, the stationary density is![]() , which is the correct prediction for the shutter's initial conditions (18). In fact
, which is the correct prediction for the shutter's initial conditions (18). In fact
![]() (22)
(22)
Therefore, for ![]() the predicted stationary density is 1.4.
the predicted stationary density is 1.4.
5. Conclusions
We derived the exact solutions for the Klein-Gordon and the Dirac shutter problems. In agreement with Moshinsky we find that the relativistic solutions do not resemble the analytic expression that appears in the theory of diffraction in Optics. In spite of this, we prove that when the exact Dirac and Klein-Gordon densities are plotted versus time, the following happens: 1) both densities show transient oscillations which in some way resemble the optical diffraction pattern; 2) the Dirac density looks quite different from the Klein-Gordon one, which implies that transient time oscillations depend strongly on the particle spin.
For these reasons and in total disagreement with Moshinsky’s conclusions [13] , we claim that impressive diffractions in time oscillations do exist in the relativistic realm. For spin 0 and 1/2 particles, we prove that diffraction in time oscillations exists only for particles of rest mass different from zero; photons do not show such time oscillations.
Appendix A
With the help of the dimensionless variables given by:![]() ,
, ![]() ,
, ![]() , and
, and ![]() , the Dirac Equation (10) may be rewritten as
, the Dirac Equation (10) may be rewritten as
![]() (23)
(23)
and the initial condition becomes:
![]() (24)
(24)
Taking the Laplace transform (![]() ) in Equation (23), and denoting
) in Equation (23), and denoting
![]() , Equation (23) becomes a matrix differential equation
, Equation (23) becomes a matrix differential equation
![]() (25)
(25)
which holds in the range![]() . Due to the presence of the step function
. Due to the presence of the step function![]() , the origin
, the origin ![]() is a singular point where we demand that the function
is a singular point where we demand that the function ![]() must be continuous. We break the infinite range into the ranges (
must be continuous. We break the infinite range into the ranges (![]() ) and (
) and (![]() ). For the left side of the shutter,
). For the left side of the shutter, ![]() denotes the solution of the dif- ferential equation:
denotes the solution of the dif- ferential equation:
![]() (26)
(26)
and for the right side, ![]() denotes the solution of
denotes the solution of
![]() (27)
(27)
Both functions ![]() and
and ![]() must be bounded (
must be bounded (![]() at
at![]() ) and (
) and (![]() at
at![]() ), and must be continuous at the interface
), and must be continuous at the interface![]() .
.
Because the matrix
![]() (28)
(28)
has eigenvalues given by:![]() , with corresponding orthogonal eigenvectors given by:
, with corresponding orthogonal eigenvectors given by:
![]() (29)
(29)
then, taking into account the boundary conditions at![]() , we have the general solutions for the matrix dif- ferential equations:
, we have the general solutions for the matrix dif- ferential equations:
![]() (30)
(30)
![]() (31)
(31)
where![]() . The constants A and B are fixed from the condition at the interface:
. The constants A and B are fixed from the condition at the interface:
![]() . We have then a set of two algebraic equations with solutions:
. We have then a set of two algebraic equations with solutions:
![]() (32)
(32)
![]() (33)
(33)
Substituting Equation (32) into Equation (30) and Equation (33) into Equation (31), we get the solution for Dirac shutter problem in the (![]() ) space. For
) space. For![]() , where the particle detector is located, we have the solution:
, where the particle detector is located, we have the solution:
![]() (34)
(34)
Notice the singular points at ![]() (simple poles) and
(simple poles) and ![]() (branch points), all of them locate in the imaginary axis. Therefore, by the Nyquist stability criterion, the time dependent solution
(branch points), all of them locate in the imaginary axis. Therefore, by the Nyquist stability criterion, the time dependent solution ![]() is an oscillatory bounded solution.
is an oscillatory bounded solution.
To simplify the final notation, we express the inverse Laplace transforms (![]() ) with the help of the integral-defined complex functions (
) with the help of the integral-defined complex functions (![]() ):
):
![]() (35)
(35)
Using Laplace Transforms Tables [15] and the convolution theorem we find the following results, valid for![]() ,
,
![]() (36)
(36)
![]() (37)
(37)
Next, we use the relation
![]() (38)
(38)
to obtain
![]() (39)
(39)
Finally, using the identities
![]() (40)
(40)
and
![]() (41)
(41)
we obtain
![]() (42)
(42)
Therefore, for![]() , the Dirac final solution is given by:
, the Dirac final solution is given by:
![]() (43)
(43)
where each inverse Laplace transform has been previously calculated. We claim that Equation (43) is the exact Dirac wave function for the shutter problem, valid for ![]() and
and ![]() (
(![]() ).
).
Appendix B
In a similar way to the Dirac solution, the Klein-Gordon shutter problem can be written in terms of dimen- sionless variables:
![]() (44)
(44)
with initial conditions given by:
![]() (45)
(45)
Taking the Laplace transform (![]() ) of Equation (44) we find the differential equations:
) of Equation (44) we find the differential equations:
![]() (46)
(46)
and
![]() (47)
(47)
Here both functions ![]() and
and ![]() must be bounded: (
must be bounded: (![]() at
at![]() ) and (
) and (![]() at
at![]() ). The two functions and their first derivatives must be continuous at the interface
). The two functions and their first derivatives must be continuous at the interface![]() .
.
Taking into account the boundary conditions at![]() , the solutions of Equations (46) and (47) are:
, the solutions of Equations (46) and (47) are:
![]() (48)
(48)
![]() (49)
(49)
where![]() . The constants A and B are fixed from the conditions at the interface:
. The constants A and B are fixed from the conditions at the interface: ![]() and
and![]() , and their first derivatives,
, and their first derivatives, ![]() and
and![]() , must be continuous at
, must be continuous at![]() . We have then a set of two coupled algebraic equations with solutions given by:
. We have then a set of two coupled algebraic equations with solutions given by:
![]() (50)
(50)
![]() (51)
(51)
Substituting Equation (50) into Equation (48) and Equation (51) into Equation (49) we have the solutions:
![]() (52)
(52)
![]() (53)
(53)
Finally, we need to invert the Laplace transforms (![]() ). We find in Laplace Transforms Tables [15] the following results valid for
). We find in Laplace Transforms Tables [15] the following results valid for![]() :
:
![]() (54)
(54)
![]() (55)
(55)
We have then the final solutions, for![]() :
:
![]() (56)
(56)
and for ![]() we get the incident and reflected wave:
we get the incident and reflected wave:
![]() (57)
(57)
We claim that Equations (56) and (57) are the exact Klein-Gordon wave functions for the shutter problem. It’s
no surprising to find the Bessel functions ![]() and
and![]() , they are just the
, they are just the
Green’s function and its derivative respectively for the Klein-Gordon equation [16] .