Cooling of Granules in Vibrating, Suspended Bed: Engineering Simulation ()
Received 29 January 2016; accepted 26 May 2016; published 30 May 2016

1. Introduction
Currently, a widely used method of materials production in several industries is in the form of granules. The granules come out of the press or granulator at a relatively high temperature of 90˚C - 120˚C and must be cooled to 30˚C - 50˚C―depending on the season and the geographic position of the enterprise. The temperature and humidity of the granules at the output must be reduced to the limits for the conservation of their properties and persistent storage. For cooling the granules two basic types of coolers are used: refrigerating vertical columns and horizontal conveyor coolers. The former have a preferential distribution [1] [2] . Cooling of the granules should be done with cold air so that different-sized granules can be distributed uniformly and cooled to the required temperature. It is also necessary to determine the thermal parameters of air and dust at the exit for further treatment in the air-cyclone. To solve the first problem we used a vertical apparatus (Figure 1) with a vibrating, suspended layer of granules (Vibrating Fluidized Bed―VFB). This unit was used by us previously [3] to dry heat-sensitive materials. In such an apparatus the hot granules fall from the granulator with a predetermined flow rate, initial temperature and initial humidity fall into the chambers of the cooler with gas-permeable, vibrating blades and asymmetrically arranged side entrances of air. Cold air is fed through the blades and side entrances
![]()
Figure 1. Cooler of granules with vibrating fluidized bed.
towards the falling granules, forming at the same time the VFB. The layer flows from one chamber of the cooler to another, thereby cooling the granules proceeds in continuous mode. In the apparatus, due to vibrations, there is an intensive heat exchange of granules with a stream of cold air and short-term contact with each other. Dust particles, together with droplets of moisture that are formed, are removed from the apparatus through openings in the side walls of the cooler (Figure 1). The granules are cooled to a final temperature and humidity using parameters set by users. Cooling of the granules in a vibrating fluidized bed is a complicated deterministic and stochastic process with the fluctuations of the basic physical parameters. Various mathematical models of the processes occurring in the fluidized bed can be found in reviews [4] - [6] . Various heat and mass transfer correlations for fluidized beds are widely represented in [7] . Synchronous vibrations of the blade and the regime of fluidization affect the final temperature and moisture content of granules and any other process parameters of the cooling process.
The Problems of this Report
・ Problem 1: An algorithm for calculation and design of the cooler with the vibrating fluidized bed of granules.
・ Problem 2: Determining the number of cooling chambers n and technological parameters which ensure cooling hot granules with a predetermined flow rate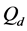 , from initial temperature
, from initial temperature 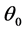 and humidity
and humidity 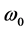 to final values of temperature and humidity
to final values of temperature and humidity 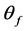 and
and 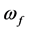 by a mass influx of moisture into the layer
by a mass influx of moisture into the layer 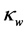 and the mass flow of dust and moisture from the layer (dust-moisture-carryover)
and the mass flow of dust and moisture from the layer (dust-moisture-carryover)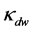 .
.
・ Problem 3: Comparatively analyzing the efficacy of the investigational cooler with the typical industrial apparatuses which is used to cool the granules in the fluidized bed.
2. Heat and Mass Balance Equations
By using the scheme of convective heat transfer [8] - [10] , we write the equations of heat and material balances for continuous cooling of the granules in the cooler with the vibrating fluidized bed (Figure 1) in the form of:
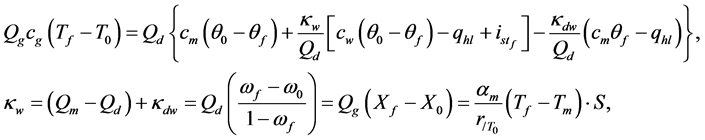 (1)
(1)
where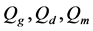 , respectively, the mass flow rate of gas (air), hot (dry) and moist granules, kg/s;
, respectively, the mass flow rate of gas (air), hot (dry) and moist granules, kg/s; 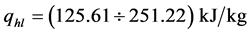 ―heat loss to the environment;
―heat loss to the environment;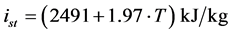 ―enthalpy superheated steam at a temperature of air―T.
―enthalpy superheated steam at a temperature of air―T.
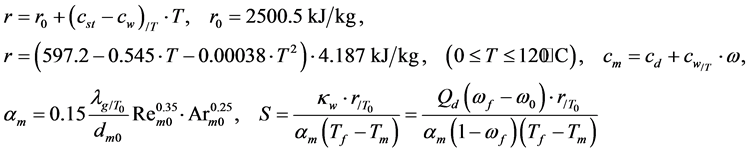 (2)
(2)
there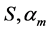 ―respectively, heat transfer surface and the heat transfer coefficient from the layer of hot granules to the cooling air. From Equation (1) the formulas for the final temperature
―respectively, heat transfer surface and the heat transfer coefficient from the layer of hot granules to the cooling air. From Equation (1) the formulas for the final temperature 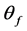 and final moisture
and final moisture ![]() of cooling granules follows:
of cooling granules follows:
![]() (3)
(3)
where![]() , heat loss in the cooler.
, heat loss in the cooler.
Method of Intervals for Calculating of Parameters
To create an algorithm for calculation of the final values ![]() in (3) and determination of the required geometry of the device it is advisable to use the method of the intervals, considered in [11] . According to this method, parameters
in (3) and determination of the required geometry of the device it is advisable to use the method of the intervals, considered in [11] . According to this method, parameters ![]() and
and ![]() can be represented as finite values of temperature
can be represented as finite values of temperature ![]() and humidity
and humidity ![]() of the granules at the outlet of the chambers of the cooler, i.e. in the form:
of the granules at the outlet of the chambers of the cooler, i.e. in the form:
![]() (4)
(4)
3. Regime of Fluidization
Mode of fluidization (by the criteria ![]() and
and![]() ), the velocity
), the velocity![]() , and mass flow rate of air
, and mass flow rate of air![]() , passing through the gas-permeable, perforated blades towards the layer of cooled granules, we determine by empirical relationships, mentioned in [7] [8] [12] :
, passing through the gas-permeable, perforated blades towards the layer of cooled granules, we determine by empirical relationships, mentioned in [7] [8] [12] :
![]() (5)
(5)
For the pressure drop ![]() between inlet and outlet from the enter layer of granules, and between additional side entries into the bed,
between inlet and outlet from the enter layer of granules, and between additional side entries into the bed, ![]() exit from it (Figure 1). Taking into account the permeability
exit from it (Figure 1). Taking into account the permeability ![]() and porosity
and porosity ![]() of grid of blades we have:
of grid of blades we have:
![]() (6)
(6)
4. Dust-Moisture-Carryover from the Fluidized Bed of Granules
Speed entrainment of dust particles and moisture from the layer of granules ![]() we will determine by the empirical formulas [11] [12] :
we will determine by the empirical formulas [11] [12] :
![]() (7)
(7)
We imagine the mass flow of dust and moisture carryover from the layer of granules ![]() as a function of the most important parameters of the process:
as a function of the most important parameters of the process:
![]() (8)
(8)
Then, using p-theorem [13] , function (8) can be converted to criterial dependence of the type:
![]() (9)
(9)
The factor in (9), comprising a heat transfer coefficient![]() , is a specific form as shown in (2). Therefore, the calculated formula for
, is a specific form as shown in (2). Therefore, the calculated formula for ![]() would be:
would be:
![]() (10)
(10)
Here ![]() is the average time of cooling of the layer of hot granules in one chamber of the cooler.
is the average time of cooling of the layer of hot granules in one chamber of the cooler.
5. Geometrical and Technological Parameters of the Cooling Process the Granules
For the geometric and technological parameters of the cooling process of the granules we will use a formula similar to that of [8] .
The height of the humidified layer of granules on the blade ![]() and the height of the space occupied by dust and moisture carried away from the bed
and the height of the space occupied by dust and moisture carried away from the bed![]() :
:
![]() (11)
(11)
The height and volume of chambers of cooler are![]() ; overall height and overall volume of the device are
; overall height and overall volume of the device are![]() :
:
![]() (12)
(12)
where ![]() is the height, occupied by the vibrator, disposed under the shoulder blade (Figure 1).
is the height, occupied by the vibrator, disposed under the shoulder blade (Figure 1).
The average mass flow rate of cooling granules ![]() and the total flow rate of the cooling air entering the device
and the total flow rate of the cooling air entering the device![]() :
:
![]() (13)
(13)
The average heat flow ![]() and the total amount of heat
and the total amount of heat![]() , transferred from the hot granules to the cold air during stay in the device
, transferred from the hot granules to the cold air during stay in the device![]() :
:
![]() (14)
(14)
The total mass of moisture, introduced into the layer![]() , the general
, the general ![]() and specific dust-moisture carry over from layer
and specific dust-moisture carry over from layer ![]() during cooling
during cooling![]() :
:
![]() (15)
(15)
For the relationship of geometrical and physical parameters of the cooling process of granules below we propose a model of a dynamics of the vibrating, suspended bed of variable mass. The model is based on the representation VFB of cooled granules, as a system of material points of variable composition, that move with its center of mass under the influence of external and internal forces (Figure 2).
6. Dynamics of the Vibrating Fluidized Bed with Variable Mass: Mathematical Model
Consider the layer of granules, located above the perforated blade, and write the differential equation of motion of the mass center VFB in vector form:
![]() (16)
(16)
In equation (16) the forces ![]() are represented by specific formulas:
are represented by specific formulas:
![]() (17)
(17)
![]() are, respectively, the pressure force of the cooling gas on the layer of granules from the gas-permeable blade and from the input side (if any).
are, respectively, the pressure force of the cooling gas on the layer of granules from the gas-permeable blade and from the input side (if any).
![]() (18)
(18)
![]() are, respectively, the gravity and buoyancy forces.
are, respectively, the gravity and buoyancy forces.
![]() (19)
(19)
![]() are the normal reaction of the gas-permeable blade on VFB and the force of friction on the surface of the blade with friction coefficient f.
are the normal reaction of the gas-permeable blade on VFB and the force of friction on the surface of the blade with friction coefficient f.
![]() (20)
(20)
is the power of the impact of the vibrator on the gas-permeable blade with the layer of granules on it.
![]() (21)
(21)
is the resistance force layer granules, which takes into account the interaction of discrete granules with the flow of cold air [8] .
![]() is the average volumetric rate of the layer of granules.
is the average volumetric rate of the layer of granules.
![]() is the coefficient taking into account the rheological properties of the granules.
is the coefficient taking into account the rheological properties of the granules.![]() , coefficient of resistance
, coefficient of resistance ![]() is:
is:
![]() (22)
(22)
![]() is the “reactive power”, that depends on the mass flow of dust and
is the “reactive power”, that depends on the mass flow of dust and
moisture, carried away from the bed of granules![]() , speed entrainment particles of dust and moisture
, speed entrainment particles of dust and moisture ![]() and speed of the center of mass of the layer
and speed of the center of mass of the layer![]() .
. ![]() is the variable mass layer of granules on the blade in the cooler chamber. The differential equations of motion of the mass center VFB in projections on the coordinate axes
is the variable mass layer of granules on the blade in the cooler chamber. The differential equations of motion of the mass center VFB in projections on the coordinate axes ![]() are derived from (16) and are presented below.
are derived from (16) and are presented below.
For the axis x-along the lines of movement of the layer (Figure 2):
![]() (23)
(23)
For the axis z:
![]() (24)
(24)
where ![]()
![]()
Figure 2. Dynamics of the vibrating fluidized bed with variable mass.
From Equation (24) under![]() , we find the normal reaction N:
, we find the normal reaction N:
![]() (25)
(25)
Equation (23) after the substitution of (25) can be represented in the form of a linear differential equation:
![]() (26)
(26)
![]() (27)
(27)
Solution of differential Equation (26) for the velocity of the center of mass ![]() and the coordinate of mass center of layer
and the coordinate of mass center of layer ![]() have the form:
have the form:
![]() (28)
(28)
where ![]() is the average speed of movement of the granules along the blade.
is the average speed of movement of the granules along the blade.
From (28) we obtain the equation to determine the residence time of the granules in the layer on the blade in a chamber of the cooler![]() :
:
![]() (29)
(29)
![]() is the proportionality factor, which is dependent on the accuracy of integration of Equation (28). Equation (29) for cooling time of the granules in the cooling chambers
is the proportionality factor, which is dependent on the accuracy of integration of Equation (28). Equation (29) for cooling time of the granules in the cooling chambers ![]() combines in a simple dependence the geometrical and physical parameters. The value for the time of cooling
combines in a simple dependence the geometrical and physical parameters. The value for the time of cooling![]() , obtained from the Equation (29), can be compared with the value
, obtained from the Equation (29), can be compared with the value ![]() from Equation (11), which uses the initial and averaged data.
from Equation (11), which uses the initial and averaged data.
Below is a procedure-algorithm for calculating a vertical rectangular cooler with VFB of granules (Figure 3).
7. The Algorithm for Calculating the Cooler with Vibrating Fluidized Bed of Granules
The algorithm is based on the above formulas and is reduced to determining the geometrical and technological parameters of the cooler, which provide the specified mode of cooling the granules and dust-moisture carryover from the VFB.
Tables 1-3, referenced in the algorithm, contain the initial data: geometry of permeable to gas, vibrated blade and layer of granules on it; kinematic, heat and mass transfer parameters, involved in the calculations, and the critical parameters-limitations, which are dependent on the requirements by the consumers. One example is cooling of granules of zeolite introduced specific numerical values of design parameters.
Table 4 gives the change cycle of temperature ![]() and humidity of granules
and humidity of granules ![]() to the required values, allowing definition of the necessary geometry of the cooler. The parameters of the cooling air
to the required values, allowing definition of the necessary geometry of the cooler. The parameters of the cooling air ![]() are determined from tables or
are determined from tables or ![]() diagrams shown, for example, in [14] [15] . The physical parameters-
diagrams shown, for example, in [14] [15] . The physical parameters- ![]() are given by formulas: (1), (3), (4), (10), (11) and (28).
are given by formulas: (1), (3), (4), (10), (11) and (28).
Table 5 and Table 6 show numerical values of the geometrical and technological parameters of the cooling process the granules of zeolite in the vertical, four - chambers cooler with VFB, selected according to the results of calculation.
![]()
Figure 3. The algorithm for calculating the geometrical and technological parameters of a vertical cooler with a vibrating, fluidized bed of granules.
Table 7 gives the numerical values of the coefficients in Equation (29), designed to determine the residence time of the granules in the cooling chamber. Equation (29) follows from the mathematical model of motion of the layer of granules along the vibrating blade.
Table 8 includes the raw data and critical parameters for comparison of typical industrial coolers with the fluidized bed of granules [1] [3] [16] .
![]()
Table 1. Geometrical parameters used in the algorithm.
![]()
Table 2. Physical parameters used in the algorithm.
![]()
Table 3. Critical parameters―limitations used in the algorithm.
![]()
Table 4. Cycle in temperature ![]() and humidity
and humidity ![]() of cooled granules.
of cooled granules.
![]()
Table 5. The process parameters for cooling granules.
![]()
Table 6. The geometrical parameters of the cooling process the granules.
![]()
Table 7. The residence time of the granules in the cooling chamber.
![]()
Table 8. Baseline and critical values for comparison of the coolers with the fluidized bed of granules.
Table 9 shows the comparative characteristics of the tested vertical, four-chamber device and typical industrial coolers with the fluidized bed of granules (Type 1 and Type 2, Table 8). For comparison, it has taken critical process parameters of the cooling process, such as:![]() .
.
8. Conclusions
In order to implement the given mode of the cooling granules that takes into account dust-moisture carryover, the most effective shape is the vertical device, with a rectangular shape with sloping, gas-permeable blades and with the vibrating fluidized bed of granules on them (Table 9). In the example of cooling the zeolite granules from ![]() to
to ![]() at the mass flow of granules
at the mass flow of granules ![]() the number of cooling chambers is equal to
the number of cooling chambers is equal to ![]() (Table 4); thus, the cooling time of granules is equal to
(Table 4); thus, the cooling time of granules is equal to ![]() (Table 5 and Table 9).
(Table 5 and Table 9).
The proposed mathematical model of the dynamics of a vibrating, suspended layer, allows us to estimate the effect of geometrical and physical parameters on the time of cooling the granules in the cooling chamber![]() . This is accomplished by varying the coefficients
. This is accomplished by varying the coefficients ![]() in Equation (29). In particular, the time
in Equation (29). In particular, the time ![]() decisively influences the coefficients
decisively influences the coefficients![]() , associated with the mass flows
, associated with the mass flows ![]() and vibration parameters
and vibration parameters ![]() (Table 7).
(Table 7).
A comparative analysis of the technological parameters of the study device (Figure 1) and typical representatives of industrial devices (Table 8 and Table 9) was made. It was found that by all most important process parameters: the cooling time![]() , the specific flow rate of air and heat flow:
, the specific flow rate of air and heat flow:![]() , the considered cooler (Figure 1) with the vibrating, and suspended bed of granules were the most effective.
, the considered cooler (Figure 1) with the vibrating, and suspended bed of granules were the most effective.
The proposed algorithm for calculation of coolers with the VFB is based on the joint use of the equations of heat and mass balances (1) and the mathematical model of dynamics of a vibrating fluidized bed (Equation (16) and Equation (29)).
The algorithm takes into account the massive influx of moisture into the layer of granules ![]() and mass flow of dust and moisture from the layer
and mass flow of dust and moisture from the layer ![]() and makes it possible to solve design problems of cooling heat-stable and thermally unstable materials.
and makes it possible to solve design problems of cooling heat-stable and thermally unstable materials.
Acknowledgements
The authors would like to thank the head of the laboratory of special fertilizers (Haifa Chemicals Ltd.), Dr. B. Gordonov, for the invitation to the solution of problems for cooling the granules in the Chemical Industry of Israel.
![]()
Table 9. Process parameters for comparison of the coolers with the fluidized bed of granules.
Notations
![]() : oscillations amplitude of vibrator, m;
: oscillations amplitude of vibrator, m;![]() : specific heat capacity, kJ/kg C;
: specific heat capacity, kJ/kg C;![]() : diameter of granules, dust particles and moisture, m;
: diameter of granules, dust particles and moisture, m;![]() : diameter of grid holes, m;
: diameter of grid holes, m;![]() : acceleration of gravity, m/s2;
: acceleration of gravity, m/s2;![]() : permeability of grid blade, m2;
: permeability of grid blade, m2;![]() : oscillations frequency of vibrator, Hz;
: oscillations frequency of vibrator, Hz;![]() : length of blades, m;
: length of blades, m;![]() : mass of granules, m;
: mass of granules, m;![]() : number of cooling chambers;
: number of cooling chambers;![]() : number particles of dust and moisture;
: number particles of dust and moisture;![]() : pressure of cooling gas, Pa;
: pressure of cooling gas, Pa;![]() :
:
pressure drop of the heat carrier (air), Pa;![]() : mass flow rate, kg/s, kg/h;
: mass flow rate, kg/s, kg/h;![]() : total flow rate of gas, m3/h;
: total flow rate of gas, m3/h;![]() :
:
heat flow, kJ/h;![]() : heat of evaporation, kJ/kg;
: heat of evaporation, kJ/kg;![]() : heat exchange surface, m2;
: heat exchange surface, m2;![]() : sectional area of the granules layer, m2;
: sectional area of the granules layer, m2;![]() : current cooling time, s, min;
: current cooling time, s, min;![]() : temperature of the heat carrier (air), C;
: temperature of the heat carrier (air), C;![]() : velocity of the cooling gas (air), m/s;
: velocity of the cooling gas (air), m/s;![]() : velocity of the dust-moisture-carryover, m/s;
: velocity of the dust-moisture-carryover, m/s;![]() : velocity of the mass center of bed of granules, m/s;
: velocity of the mass center of bed of granules, m/s;![]() : vibrating, fluidized bed;
: vibrating, fluidized bed;![]() : air humidity, kg of moisture to kg of dry air;
: air humidity, kg of moisture to kg of dry air;![]() : coordinates, m.
: coordinates, m.
Greek symbols:![]() : heat transfer coefficient, kW/m2・C;
: heat transfer coefficient, kW/m2・C;![]() : width of the blade, m;
: width of the blade, m;![]() : surface tension, N/m;
: surface tension, N/m;![]() : porosity of grid;
: porosity of grid;![]() : temperature of granules, C;
: temperature of granules, C;![]() : coefficient of the thermal conductivity, kW/m C;
: coefficient of the thermal conductivity, kW/m C;![]() : dynamic coefficient of viscosity, Pa・s;
: dynamic coefficient of viscosity, Pa・s;![]() : kinematic coefficient of viscosity, m2/s;
: kinematic coefficient of viscosity, m2/s;![]() : density, kg/m3;
: density, kg/m3;![]() : area of “ live “ section of bed of granules, m2;
: area of “ live “ section of bed of granules, m2;![]() : concentration of granules in the bed;
: concentration of granules in the bed;![]() : the blade angle, degree; relative humidity of air, %;
: the blade angle, degree; relative humidity of air, %;![]() : absolute humidity of granules, kg of moisture to total weight.
: absolute humidity of granules, kg of moisture to total weight.
Superscript: *: Critical values-limitations.
Subscripts:![]() : gas permeable blade, lateral injection of gas (air);
: gas permeable blade, lateral injection of gas (air);![]() : apparatus;
: apparatus;![]() : average value;
: average value;![]() : bed of granules;
: bed of granules;![]() : center of mass, cooling;
: center of mass, cooling;![]() : dry (hot) granules;
: dry (hot) granules;![]() : dust-moisture-carryover;
: dust-moisture-carryover;![]() : gas (air);
: gas (air);![]() : granules, grid;
: granules, grid;![]() : heat loss in the cooler;
: heat loss in the cooler;![]() : material (granules), wet thermometer;
: material (granules), wet thermometer;![]() : sections (chambers) of cooler;
: sections (chambers) of cooler;![]() : superheated steam;
: superheated steam;![]() : water, humidity;
: water, humidity;![]() : initial and end values;
: initial and end values;![]() : sum value;
: sum value;![]() : unit vectors of the axes
: unit vectors of the axes![]() .
.
Dimensionless groups-Criteria:![]() : Reynolds number;
: Reynolds number;![]() : Archimedes number.
: Archimedes number.
NOTES
![]()
*Corresponding author.