Quantum Mechanical Approach for Rutherford Scattering and Nuclear Scattering with Born Approximation ()
Received 19 December 2015; accepted 19 January 2016; published 22 January 2016

1. Introduction
It has not been successful, to our knowledge, to find such reported results anywhere in any literature the world over. Theoretical results were developed, the verification of which would, however, be needed with experimental results on beam physics. Rutherford classical scattering [1] [2] and the fundamental results on quantum mechanics [3] [4] were revisited. Quantum mechanical scattering theory [5] and fundamental results with born approximation functions (energy profile) were reanalyzed by considering the nucleus analogous to onion. The phase shifts for each diverse case under consideration for quantum mechanical scattering are different with substitution of such phase shifts with appropriate selection of equations (reported in this manuscript). The shape of scattering eigenfunctions can be reproduced provided the wave vectors of incident and scattered beams are known. The diverse nuclear scattering cross-sections were determined. The modified Rutherford scattering formulas developed in this manuscript are applicable only to Coulombic field in the extra-nuclear region (range of scattering, R).
Quantum action deals with oscillatory behavior of matter waves (transverse waves) which configures a space of its own called a wave packet or quanta. 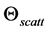 in quantum scattering cannot be measured directly but can be recorded with detectors and the same is the case with azimuth angle,
in quantum scattering cannot be measured directly but can be recorded with detectors and the same is the case with azimuth angle,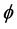 . However, formulas need testing for their validity on high energy accelerators. These formulas are good enough for Coulombic field (target material) provided the incident particles are also charged particles. For quantum mechanical scattering from the nucleus of the atom, highly energetic incident particles (charged or uncharged) are needed. Envisage the nucleus, like an onion with each of its layer exceptionally whirling and swirling. The surface of deformed or undeformed nucleus is considered like a quantum potential barrier and the interior of the nucleus like a quantum potential well with its brim changing shape continuously with overwhelming whirling and swirling effects. The rotational momentum, quadru pole and octo pole moments of the nucleus are assumed to be negligible compared to whirling and swirling effects. Energy profile (eigenfunctions) is deciphered both even and odd, for reflection and quantum mechanical tunneling from nuclear surface barrier, and then the quantum mechanical scattering profiles from each inside layer of the nucleus of the atom.
. However, formulas need testing for their validity on high energy accelerators. These formulas are good enough for Coulombic field (target material) provided the incident particles are also charged particles. For quantum mechanical scattering from the nucleus of the atom, highly energetic incident particles (charged or uncharged) are needed. Envisage the nucleus, like an onion with each of its layer exceptionally whirling and swirling. The surface of deformed or undeformed nucleus is considered like a quantum potential barrier and the interior of the nucleus like a quantum potential well with its brim changing shape continuously with overwhelming whirling and swirling effects. The rotational momentum, quadru pole and octo pole moments of the nucleus are assumed to be negligible compared to whirling and swirling effects. Energy profile (eigenfunctions) is deciphered both even and odd, for reflection and quantum mechanical tunneling from nuclear surface barrier, and then the quantum mechanical scattering profiles from each inside layer of the nucleus of the atom.
It is assumed that the parity of scattering remains conserved. The quantum mechanical scattering its self is an asymmetrical second order process. Diverse scattering cross sections can be determined for each of the described above cases with known phase shifts and the wave vectors for scattered particles. With scattering profiles (scattering eigenfunctions), the shape and size of scattering through modeling and simulation can be reproduced.
2. Theory and Discussions
The scattering cross-section and indeed the differential scattering cross-section in Rutherford scattering depend on measurable entities like 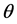 and
and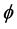 . The differential scattering cross-section is a manifestation of either geometrical/structural factor or atomic/ion form factor and used only in classical scattering mechanisms. The geometrical/structure factor with X-ray reflections and diffractions from crystal lattices can be determined followed by fold and other symmetries. The atomic/ion form factors provide the strength of atoms or ions in the crystal lattices and that is accomplished with classical scattering formulas. The impact parameter in Rutherford scattering provides the strength of interaction of the incident (usually charged particles) with the Coulombic field (target material), as a consequences of which, scattering profiles are studied. We shall not, deliberate on the conditionalities of Rutherford scattering (simultaneous use of laboratory and centre of mass coordinate systems) [1] .
. The differential scattering cross-section is a manifestation of either geometrical/structural factor or atomic/ion form factor and used only in classical scattering mechanisms. The geometrical/structure factor with X-ray reflections and diffractions from crystal lattices can be determined followed by fold and other symmetries. The atomic/ion form factors provide the strength of atoms or ions in the crystal lattices and that is accomplished with classical scattering formulas. The impact parameter in Rutherford scattering provides the strength of interaction of the incident (usually charged particles) with the Coulombic field (target material), as a consequences of which, scattering profiles are studied. We shall not, deliberate on the conditionalities of Rutherford scattering (simultaneous use of laboratory and centre of mass coordinate systems) [1] .
2.1. Case-I: Quantum Theory of Rutherford Scattering and Born approximations for Coulombic Fields inside the Nucleus
Using de Broglie hypothesis, 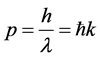 , where
, where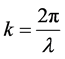 ,
, 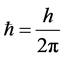 ,
, 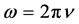 ,
,  ,
, 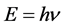 ,
,  and
and ![]() for incident and scattering particles, the Rutherford scattering formulas is modified for scattering cross-section and the impact parameter. First, writing the original Rutherford scattering formulas [1] ,
for incident and scattering particles, the Rutherford scattering formulas is modified for scattering cross-section and the impact parameter. First, writing the original Rutherford scattering formulas [1] ,
![]() (1)
(1)
where Ze is the charge of incident of particles, ![]() the charge of target material,
the charge of target material, ![]() the scattering angle,
the scattering angle, ![]() the azimuth angle, E the kinetic energy and
the azimuth angle, E the kinetic energy and ![]() the scattering cross-section. The impact parameter, s is
the scattering cross-section. The impact parameter, s is
![]() (2)
(2)
Changing ![]() inequations (1) and (2) with
inequations (1) and (2) with![]() , where
, where ![]() is reduced mass. We have
is reduced mass. We have
![]() (3)
(3)
With rigorous mathematical substitution of basic quantum mechanical entities, as mention in the above paragraph, a meaningful solution is obtained, i.e.,
![]() (4)
(4)
where c is the velocity of light, ![]() the wave length of incident beam,
the wave length of incident beam, ![]() the order of the incident beam and
the order of the incident beam and ![]() the scattering angle of the beam.
the scattering angle of the beam. ![]() in case of quantum mechanical scattering can not be mea- sured but can be recorded with detectors. The quantum mechanical impact parameters becomes
in case of quantum mechanical scattering can not be mea- sured but can be recorded with detectors. The quantum mechanical impact parameters becomes
![]() (5)
(5)
where ![]() is the frequency of incident beam and
is the frequency of incident beam and ![]() the circumference of the incident beam. h is Planck’s constant in both Equations (4) and (5).
the circumference of the incident beam. h is Planck’s constant in both Equations (4) and (5).
It is found that our both formulas (4) and (5), i.e., quantum mechanical scattering cross-section and the impact parameter follow the inverse square law of quantum action, ![]() (planck’s constant). Quantum impact parameters will determine the strength of the scattering process.
(planck’s constant). Quantum impact parameters will determine the strength of the scattering process.
If highly energetic incident particles are considered which could tunnel through the Coulomb’s barrier then the incident particles will suffer nuclear surface barrier and the potential well which is envisaged as the interior of the nucleus. The incident particles should have sufficient energy to tunnel through the nuclear surface barrier to get accommodated in the interior of the nucleus. These incident particles will make the nucleus to undergo scattering, of course, by the brim of the potential well and indeed by tunneling the other side of the nuclear surface barrier. For r > R (range of classical scattering) the Coulombic field is encountered. Now using certain conditions of Born approximation [5] . Writing first equation for the nuclear surface barrier and then
![]() (6)
(6)
For![]() , the incident particles are pulled into the interior of the nucleus and exciting the nucleus to the extent that partial waves are emitted, of course, with phase shifts,
, the incident particles are pulled into the interior of the nucleus and exciting the nucleus to the extent that partial waves are emitted, of course, with phase shifts, ![]() thereby providing information about energy changes and nuclear potentials.
thereby providing information about energy changes and nuclear potentials. ![]() and
and ![]() in equation (6) are Bessel and Neumann functions, respectively.
in equation (6) are Bessel and Neumann functions, respectively. ![]() gives the range in which nuclear scattering occurs. For
gives the range in which nuclear scattering occurs. For![]() , the incident particles have already tunneled through the Coulombic field and now face nuclear surface barrier. Applying conditions of tunneling, such as for even solution of
, the incident particles have already tunneled through the Coulombic field and now face nuclear surface barrier. Applying conditions of tunneling, such as for even solution of![]() , [5] ,
, [5] ,
![]() (7)
(7)
where a is the width of the surface nuclear barrier and v the potential energy. For odd solution of ![]()
![]() (8)
(8)
Reflection (scattering) from the nuclear surface barrier as well as transmission (quantum tunneling) of incident particles are evident. Some of the incident particles are pulled into the nucleus while some of them tunnel perfectly through the other side of the nuclear surface barrier. For reflection, ![]() , the incident particles will have an eigenfunction (energy profile) with phase shifts
, the incident particles will have an eigenfunction (energy profile) with phase shifts![]() , for transmission,
, for transmission, ![]() , (complete tunneling through the nuclear surface barrier) and during transmission
, (complete tunneling through the nuclear surface barrier) and during transmission![]() , we shall have
, we shall have ![]() s quite different for
s quite different for ![]() and for
and for![]() . During transmission,
. During transmission, ![]() , some of the particles are pulled in to the nucleus. The eigenfunctions for reflection and transmission from a nuclear surface potential barrier are reproduced below.
, some of the particles are pulled in to the nucleus. The eigenfunctions for reflection and transmission from a nuclear surface potential barrier are reproduced below.
![]() (9)
(9)
![]() (10)
(10)
The first term in the first part of Equation (9), i.e., ![]() shows the profile of incident beam.
shows the profile of incident beam. ![]() in equation (7) will be replaced by
in equation (7) will be replaced by ![]() because the phase shift is a manifestation of whirling and swirling effects of the nucleus and indeed of deformed nuclear surface potential barrier. The dependence of azimuth quantum number l on swirling effects follow the Eulerian angles which are assumed to be very negligible.
because the phase shift is a manifestation of whirling and swirling effects of the nucleus and indeed of deformed nuclear surface potential barrier. The dependence of azimuth quantum number l on swirling effects follow the Eulerian angles which are assumed to be very negligible.
For![]() , the incident particles are either pulled in to the nucleus
, the incident particles are either pulled in to the nucleus ![]() or tunneled through the nuclear surface potential barrier after capture, therefore, equation (6) will take the shape
or tunneled through the nuclear surface potential barrier after capture, therefore, equation (6) will take the shape
![]() (11)
(11)
The incident particles having sufficient energy will tunnel through the other side of the nuclear surface potential barrier [5] ,![]() .
.
![]() (12)
(12)
The incident particles which are captured by the nucleus ![]() will have no effect for either
will have no effect for either
![]() or
or ![]() because the incident particles which are captured for a while will be scattered from within different layers inside the nucleus. Rewritingequation (10)
because the incident particles which are captured for a while will be scattered from within different layers inside the nucleus. Rewritingequation (10)
![]() (13)
(13)
The wave vector in Equation (12) shows the quantum action of scattered particles either for complete tunneling or tunneling after capturing by the nucleus. The emission of partial waves, in either cases, is a manifestation of asymptotic dependence of Bessel and Neumann functions, respectively. The partial waves from inside (quantum well) of the nucleus differ from partial waves emitted, as a consequence of tunneling, through the nuclear surface potential barrier. The asymptotic conditions of Bessel and Neumann functions, respectively are written
![]() (14)
(14)
The Neumann function ![]() will decide the asymptotic conditions of quantum mechanical scattering from the inside of the nucleus and the Bessel function,
will decide the asymptotic conditions of quantum mechanical scattering from the inside of the nucleus and the Bessel function, ![]() for asymptotic conditions of quantum mechanical scattering from the surface of the nucleus. The azimuthal quantum number, l in the Neumann functions,
for asymptotic conditions of quantum mechanical scattering from the surface of the nucleus. The azimuthal quantum number, l in the Neumann functions, ![]() is dependent on “whirling and swirling”, as a consequence of which, phase shifts for quantum mechanical scattering from inside the nucleus are manifestation of complex behaviour.
is dependent on “whirling and swirling”, as a consequence of which, phase shifts for quantum mechanical scattering from inside the nucleus are manifestation of complex behaviour.
2.2. Case-II: Quantum Mechanical Bulk Tunneling and Scattering with Born Approximations Both for Ruptured and Unruptured Nucleus
With asymptotic conditions for particles which tunneled through the surface potential barrier, captured within the nucleus and then scattered with phase shift,
![]()
and then only the Neumann function will work,
![]()
equation (12) will take the following shape:
![]() (15)
(15)
Using second part of Equation (9), which is for transmission,the eigenfunctions(energy profile) for different values of ![]() corresponding to inside layers within the nucleus will become
corresponding to inside layers within the nucleus will become
![]() (16)
(16)
Equation (15) shows that scattered particles from inside various layers of the nucleus. For![]() , we can have
, we can have
![]() (17)
(17)
Equation (16) represents the energy profile for particles which are scattered from the inside of the nucleus for any value of azimuthal quantum number. it is assumed that ![]() for any value of l, i.e.,
for any value of l, i.e., ![]() (un deformed),
(un deformed), ![]() (deformed) because the direction cosine is normal to any place on the surface of a sphere (spherical harmonics for the nucleus). Thus, equation (15) can be written as
(deformed) because the direction cosine is normal to any place on the surface of a sphere (spherical harmonics for the nucleus). Thus, equation (15) can be written as
![]() (18)
(18)
For ![]() (un deformed nucleus),
(un deformed nucleus), ![]()
![]() (19)
(19)
For![]() , the nucleus will be deformed and then internal quantum mechanical scattering will occur. In such a trivial situation,
, the nucleus will be deformed and then internal quantum mechanical scattering will occur. In such a trivial situation, ![]() ,
, ![]() , the radius
, the radius ![]() inside the nucleus will change
inside the nucleus will change
![]() (20)
(20)
For ![]() (un deformed nucleus),
(un deformed nucleus), ![]() , which implies that it is equivalent to quantum tunneling behaviour of the nucleus soon after capturing the incident particles.
, which implies that it is equivalent to quantum tunneling behaviour of the nucleus soon after capturing the incident particles.
2.3. Case-III: Quantum Mechanical Surface Tunneling and Scattering with Born Approximations Both for Ruptured and Unruptured Nucleus
For ![]() especially the quantum mechanical tunneling and scattering from the surface of the nuclear potential barrier,we shall use part 1 of equation (9) and make
especially the quantum mechanical tunneling and scattering from the surface of the nuclear potential barrier,we shall use part 1 of equation (9) and make ![]() in Equation (12), we have
in Equation (12), we have
![]() (21)
(21)
the incident particles after tunneling the Coulombic field will encounter the nuclear surface potential barrier, where ![]() is applicable. Using second term of first part of Equation (9) for quantum mechanical scattering from the surface of the nucleus whether deformed or undeformed, on Equation (20), we have
is applicable. Using second term of first part of Equation (9) for quantum mechanical scattering from the surface of the nucleus whether deformed or undeformed, on Equation (20), we have
![]() (22)
(22)
![]() (23)
(23)
![]() (24)
(24)
With ![]() (un deformed nucleus)
(un deformed nucleus)
![]() (25)
(25)
where ![]() is the radius of un deformed nucleus. For
is the radius of un deformed nucleus. For![]() ,
,![]() . The
. The ![]() exists for undeformed nucleus.
exists for undeformed nucleus.
With![]() , i.e.,
, i.e., ![]()
![]() (26)
(26)
where rl = radius of the screened nuclear surface.
![]()
For![]() , equation (23) will correspond to scattering from screened nuclear surface Potential barrier.
, equation (23) will correspond to scattering from screened nuclear surface Potential barrier.
2.4. Case-IV: Calculations of Scattering Cross-Sections with Diverse wave Vectors and Phase Shifts Both for Ruptured and Unruptured Nucleus
The diverse nuclear quantum mechanical scattering cross-sections for each of the described above cases can be determined by using a generally accepted universal formula available in reference books [3] -[5]
![]() (27)
(27)
3. Conclusion
we infer the following conclusions from this study: Quantum theory of Rutherford Scattering is established. Born approximations for coulombic fields inside the nucleus of the atom are determined for reflection and transmission with corresponding wave vectors, phase shifts and eigenfunctions. Bulk Quantum mechanical tunneling and scattering with born approximations both for ruptured and unruptured nucleus of the atom are deciphered with corresponding wave vectors, phase shifts and eigenfunctions. Surface quantum mechanical tunneling and scattering with born approximations for ruptured and unruptured nucleus of the atom are studied with corresponding phase shifts and eigenfunctions. Diverse Quantum mechanical scattering cross-sections with corresponding phase shifts and eigenfunctions for bulk and surface behavior of layers inside the nucleus of the atom will help to resolve and understand the exchange fields inside the nucleus of the atom.