Received 11 October 2015; accepted 26 December 2015; published 29 December 2015

1. Introduction
Electron-molecule collision cross sections from very low energy up to threshold play a pivotal role in determining electron transport properties and electron energy distribution of a swarm of electrons drifting through various gases. Per-fluorinated compounds (PFC) are widely used in electrical industries, plasma-assisted fabrication of microcircuits, surface hardening, agriculture, and medicinal fields. PF3 is also a potential reagent for the gas- phase synthesis in microelectronic doping [1] . Theoretical works based on Binary Encounter Bethe (BEB) and Complex Potential Method (CPM) for total electron ionization cross sections of PF3, both evaluated by M. Vinodkumar et al. [1] are available till now.
This letter reports the results of the single differential cross sections (SDCS) as a function of secondary electron energy and double differential cross sections (DDCS) as a function of secondary electron energy and incident angle of electron, by using modified Jain and Khare semi-empirical approach [2] -[8] . Modified Jain and Khare semi-empirical approach is the only formulation that evaluates the energy dependent partial cross sections for molecules in electron ionization. To the best of my knowledge, no other data (experimental and/or theoretical) of differential cross sections are available till now. The sum/or total of the partial ionization cross sections (PICS) leading to the formation of various cations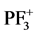 ,
, 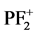 , PF+,
, PF+, 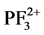 ,
,  , P+, PF2+, F+, and P2+ resulting through the direct and dissociative ionization of PF3 by electron collision show good agreement with the available theoretical data [1] . The results of partial ionization cross sections (PICS) of PF3 molecule were not available till now. We have also evaluated the ionization rate coefficients for integral ionization cross sections by using Maxwell-Boltzmann energy distribution [9] [10] . These results are important in plasma simulations.
, P+, PF2+, F+, and P2+ resulting through the direct and dissociative ionization of PF3 by electron collision show good agreement with the available theoretical data [1] . The results of partial ionization cross sections (PICS) of PF3 molecule were not available till now. We have also evaluated the ionization rate coefficients for integral ionization cross sections by using Maxwell-Boltzmann energy distribution [9] [10] . These results are important in plasma simulations.
2. Theoretical
The partial integral cross sections by using modified Jain-Khare semi-empirical approach [2] -[8] leading to the production of ith type of ion is given by
 (1)
(1)
Here all symbols have their usual meanings as defined in Refs [2] -[8] .
Single differential cross sections (SDCS) formulism can be obtained by differentiation of (Equation (1)) w.r. to secondary electron energy i.e.
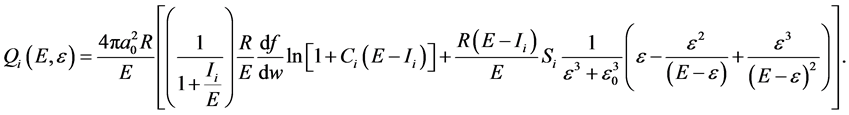 (2)
(2)
For the evaluation of double differential cross sections (DDCS) we have used formula derived by Kumar et al. [7]
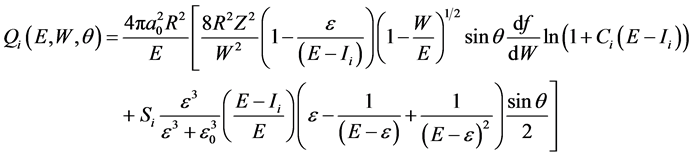 (3)
(3)
and the total cross section is obtained by
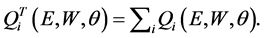 (4)
(4)
For PF3 molecule the oscillator strengths and ionization potentials of various cations (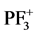 ,
,  , PF+,
, PF+, 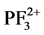 ,
, 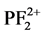 , P+,
, P+, 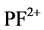 , F+, and P2+) are taken from the experimental results of J. W. Au et al. [11] . Here, experimental partial oscillator strengths of
, F+, and P2+) are taken from the experimental results of J. W. Au et al. [11] . Here, experimental partial oscillator strengths of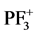 ,
, 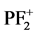 , PF+,
, PF+, ![]() ,
, ![]() , P+,
, P+, ![]() , F+, and P2+ are available up to 130 eV. For higher energies, the same data have been extrapolated by the Thomas-Reiche-Kuhn (TRK) sum rule, within 10% error bars [11] . The value of collisional parameter (Ci = 0.03789) and mixing parameters (ɛ0 = 45 eV) have been calculated as for other molecules [2] -[8] .
, F+, and P2+ are available up to 130 eV. For higher energies, the same data have been extrapolated by the Thomas-Reiche-Kuhn (TRK) sum rule, within 10% error bars [11] . The value of collisional parameter (Ci = 0.03789) and mixing parameters (ɛ0 = 45 eV) have been calculated as for other molecules [2] -[8] .
Ionization rate coefficients are important quantities in plasma processes which are determined by using M-B distribution of temperature/energy [9] [10] for calculated partial and total ionization cross sections and are given as
![]() (5)
(5)
where k, T and m are the Boltzmann constant, absolute temperature and mass of the electron, respectively.
3. Results and Discussion
The phosphorus trifluoride molecule, which has trigonal pyramidal geometry and belongs to the C3v symmetry group, has a ground state (1A1) electronic configuration in the independent particle model [11] i.e.
![]()
![]()
Differential and partial integral ionization cross sections for PF3 were not available till now, therefore a wide scope is available for the researcher. Partial and total single differential cross sections (SDCS) as a function of secondary electron energy at fixed incident energies of 100 and 200 eV are evaluated and are shown in Figure 1. Partial and total double differential cross sections (DDCS) as a function of secondary electron energy and incident angle of electron at fixed incident electron energies of 100 and 200 eV, and fixed angles 30˚ and 60˚ are also evaluated and are shown in Figure 2. Angular behavior of DDCS at fixed incident electron energies 100 and 200 eV with fixed secondary electron energies 10 and 20 eV by varying scattering angle from 0˚ to 180˚ are also given in Figure 3 and 3D profile of DDCS as a function of secondary electron energy (in the range of 5 eV to Wmax/2) and angle (10˚ to 180˚) are represented in Figure 4 at fixed incident electron energies 100 and 200 eV. To the best of my knowledge, no other experimental and/or theoretical data of differential cross sections is available for comparison, till now. However, the qualitative behavior of the cross sections is the same as for other molecules [2] -[8] . The energy dependent cross sections are symmetric at Wmax/2, where the energies of primary and the secondary electrons are almost equal, except some irregular behavior at lower energy side. The present calculations account the contribution of exchange effects and resonances through the second part of the formulation (Equations (1)-(3)). In the present formulation (Equations (1)-(3)) the first part known as Born- Bethe cross section for slow secondary electron, corresponds to the growing contribution of the dipole-allowed interaction and resembles the photoionization cross-section and second part, the Mott cross section accounts for the electron exchange effect, is the non-dipole part which defines the knock-on collision. The figures clearly show the weight contribution of the molecular and atomic cations. The cross sections for molecular ions are much larger than the atomic ions.
The partial ionization cross sections corresponding to the formation of various cations![]() ,
, ![]() , PF+,
, PF+, ![]() ,
, ![]() , P+, PF2+, F+, and P2+ in electron impact ionization of PF3 in the impinging electron energy range varying from ionization threshold to 1000 eV are evaluated and shown in Figure 5 along-with Table 1. Again there is neither experimental nor theoretical data available in the literature for comparison to the evaluated partial cross sections. Hence, the sum of partial ionization cross section, also called the total or counting ionization cross section have become important. In Figure 5, the evaluated total ionization cross sections are compared with the theoretical data of Binary Encounter Bethe (BEB) and Complex Potential Method (CPM), both are
, P+, PF2+, F+, and P2+ in electron impact ionization of PF3 in the impinging electron energy range varying from ionization threshold to 1000 eV are evaluated and shown in Figure 5 along-with Table 1. Again there is neither experimental nor theoretical data available in the literature for comparison to the evaluated partial cross sections. Hence, the sum of partial ionization cross section, also called the total or counting ionization cross section have become important. In Figure 5, the evaluated total ionization cross sections are compared with the theoretical data of Binary Encounter Bethe (BEB) and Complex Potential Method (CPM), both are
![]()
![]()
Figure 1. Partial and total single differential cross sections (SDCS) of PF3 at fixed impinging electron energies 100 and 200 eV.
evaluated by Minaxi Vinodkumar et al. [1] . The present calculations for the partial and the total ionization cross sections satisfy the necessary consistency checks to access their consistency and reliability. The following trivial consistency checks applied to check the reliability of our calculations include those of 1) the integral cross sections corroborate the area covered by the corresponding differential cross sections at the given energies. 2) The total ionization cross section is equal to the sum of the partial ionization cross sections. This condition is used in the summation method for calibration purposes. 3) The integral ionization cross sections in low energy regime obey the Wannier threshold law [12] [13]
![]() (6)
(6)
where m is defined in term of charge z on the residual ion i.e.
![]() (7)
(7)
These consistency checks provide the consistency and reliability of the present results.
We have also evaluated a set of ionization rate coefficients as a function of electron energy for the individual cations produced in electron collision with the PF3 molecule. The calculations are made using the calculated ionization cross sections and Maxwell-Boltzmann energy distribution (Equation (5)) and the results are presented in Figure 6.
4. Conclusion
The present calculation for energy dependent differential and integral ionization cross sections is an attempt
![]()
![]()
Figure 4. 3D profile of total double differential cross sections (DDCS) of PF3 at fixed impinging electron energies of 100 and 200 eV.
towards the wider applicability of a modified Jain-Khare semi-empirical formalism. First time, we have evaluated the differential and partial ionization cross sections leading to the various cations in electron-PF3 collision processes and the results are predictive to the experimentalist for measurement. However, the total ionization cross-sections revealed a reasonable good agreement with the available theoretical data. The ionization rate
![]()
Figure 6. Ionization rate coefficients corresponding to partial ionization cross sections (PICS) for electron ionization of PF3.
![]()
Table 1. Partial ionization cross sections (10−16 cm2) of PF3 molecule.
coefficients as a function of electron energy are also evaluated. These results are useful in plasma simulation and modeling.
Acknowledgements
Author is thankful to reviewers for good suggestions in improvement of paper quality.