Measurement of Bubble Behavior and Impact on Solid Wall Induced by Fiber-Holmium:YAG Laser ()
1. Introduction
For urinary stone disease treatment using shock waves, there are several effective methods of lithotripsy such as extracorporeal shock wave lithotripsy (ESWL) [1] , laser lithotripsy [2] -[7] , and electrohydraulic lithotripsy (EHL) [7] . We focus on bubble behavior induced by a fiber laser used for transurethral ureterolithotripsy (TUL) [2] [6] , and its resulting impact on a solid wall surface. In TUL, a single bubble is formed by a laser irradiated from the tip of a fiber, and shock waves formed by the collapse of the bubble propagate toward the stone.
There are many studies on the characteristic behavior and impact of laser-induced cavitation bubble collapse near a solid wall. There are also detailed observations for a single bubble induced by a focused laser that show characteristic collapse behavior and impact [8] -[13] . Those results show the existence of interesting impulsive behavior (such as a shock wave and a micro jet) associated with the mechanism of the impact and the erosion caused by a collapse of single bubble. In addition, behaviors of cavitation bubble collapse in flow field are also shown by using a high speed video camera synchronized with the impulsive force sensor and hydrophones [14] -[16] . Recently, there are many numerical studies on a single bubble collapse with associate to mechanism of impact [12] [17] -[20] . In the viewpoint of medical field, the effects of a nearby elastic wall surface on bubble collapse behavior have been made clear experimentally and numerically [17] [18] [21] . These show the characteristic bubble collapse with shock wave emission and micro-jet penetration.
In this study, we conduct several experiments to examine bubble behavior and its impact on a nearby solid wall following fiber laser-induced bubble collapse. Although there are some studies on the behavior of bubbles induced by a fiber laser [2] [4] , there appears to be few studies for in vivo treatment with detailed high-speed observations. In this study, we investigate the effects of laser irradiation conditions (laser power and fiber diameter) with and without solid boundaries (distance between the wall and fiber tip) to reveal the optimum laser irradiation conditions for the treatment of urinary stone disease.
2. Experimental Apparatus and Procedure
Figure 1 shows the experimental setup. The holmium:YAG (Ho:YAG) laser used in this experiment is applied in a vessel (100 mm in height, 120 mm in width and 50 mm in depth) filled with tap water (temperature Tw, dissolved oxygen content b) through a fiber (fiber diameters d = 360, 420, and 550 μm) connected to a laser source (Lumenis, VersaPulse Holmium YAG LASER 30W). The wave length and pulse duration of the laser are λ = 2.06 μm and τ = 250 μs, respectively. The energy of the laser is set to E = 0.5, 1.0, 1.5, and 2.0 J per pulse. The energy is immediately absorbed in water as heat due to the relatively long wavelength, and then a bubble is formed [4] . A laser-induced bubble is illuminated by a metal-halide light and observed by a high-speed video camera (Photron, SA5) located on the opposite side of the light. The frame speed of the camera is Fs = 100,000 frames per second (fps). The camera is synchronized with a hydrophone (B & K, 8013) that has flat frequency response within ±3 dB over the frequency range from 0.1 Hz to 180 kHz or a hand-made impulsive force sensor and can record the bubble aspect before and after the laser irradiation [14] -[16] .
Figure 2 shows a schematic diagram of a handmade PVDF (Polyvinylidene difluoride) impulsive force sensor. The PVDF film is fixed by an adhesive on an acrylic resin plate. The sensing area is made of an epoxy resin with 4 mm in diameter. The sensing area is covered by a silicone tube and a silicone gasket so as not to respond to impacts on the surrounding area. The fiber tip is placed on the center axis of the impulsive force sensor. The diameter of the sensor is sufficiently large relative to the bubble size at the collapse. Figure 3 shows output waveforms of the handmade sensor. Figure 3(a) is results in ball drop test (the ball is made of glass, mass m = 4.6 g)), and Figure 3(b) is in cavitation bubble. Both pulses rise just after the impact and then swing to the negative value due to the sensor characteristics. The handmade sensor is suitable for cavitation impulse measurements since the wave form rise is within about 5 μs as shown in Figure 3(b). Here, since the pulse duration is important for the calibration [22] , the calibration must be improved further.
The laser fiber is installed perpendicular (vertical wall surface) or parallel (horizontal wall surface) to the solid
![]()
Figure 2. Schematic diagram of impulsive force sensor.
![]()
Figure 3. Output of impulsive force sensor.
wall with the impulsive force sensor, as shown in Figure 4. The distance between the fiber tip and the wall surface is set as x for the former case or h = 0 to 5.5 mm for the latter case.
3. Results
3.1. Bubble Behavior and Impact in the Absence of a Wall Surface
Figure 5 shows the behavior of bubble formation and collapse in the absence of a wall surface. For all laser irradiation conditions, a single bubble forms immediately after irradiation (t = 0.05 ms) before reaching its maximum diameter and then collapsing with multiple rebounds. Figure 6 shows a time course of equivalent bubble radius with various fiber diameter and laser energy conditions. The bubble radius is equivalent radius calculated from the projected area in each image assuming axial symmetry. The maximum bubble radius Rmax becomes larger with an increase in laser power and fiber diameter. The life of the bubble also becomes longer with the increase in the maximum bubble radius. The bubble collapses and then rebounds with some radius because a bubble contains vapor and non-condensable gas. Here Rayleigh’s bubble collapse time tc, i.e., the time from the bubble maximum to the subsequent minimum, can be given by Equation (1) [11] ,
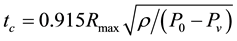 (1)
(1)
where ρ is the density of water, P0 is ambient pressure of the bubble, and Pv is the saturated pressure of water.
Figure 7 shows bubble collapse times calculated using Figure 6 and Equation (1). There is a tendency for these bubble collapse times to be longer than the values given by Equation (1) due to containing non-condensa- ble gas. Figure 8 shows changes in sound pressure measured by the hydrophone installed at 10 mm from the fiber tip. The sound pressure becomes larger with an increase in the maximum bubble radius. This tendency also agrees with previous studies [3] . The bubble becomes larger with an increase in laser power as shown in Figure 6, namely, the energy is stored in the bubble. The bubble will be compressed back to a small volume inverting the expansion. The energy is lost as a shock wave that is launched into the liquid upon collapse of the bubble. Since these studies have been carried out in many past studies, we transfer more detailed mechanism to reference [9] .
![]()
Figure 4. Arrangement of laser fiber and impulsive force sensor.
![]() Tw= 291 K, E = 0.5J, d = 420 μm, β = 8.5 mg/L
Tw= 291 K, E = 0.5J, d = 420 μm, β = 8.5 mg/L
Figure 5. Bubble formation and collapse behavior.
3.2. Bubble Behavior and Impact near a Wall Surface
Figure 9 shows examples of bubble collapse behavior and the associated impact waves near the solid wall that
![]() Tw= 292K, β = 7.2 mg/L, Fs = 100000 fps
Tw= 292K, β = 7.2 mg/L, Fs = 100000 fps
Figure 7. Changes in bubble collapse time.
![]() Tw= 292 K, β = 7.2 mg/L, Fs = 100000 fps
Tw= 292 K, β = 7.2 mg/L, Fs = 100000 fps
Figure 8. Changes in sound pressure against maximum bubble radius.
is perpendicular to the laser fiber. The bubble is formed in contact with the wall and collapses on the wall surface when the laser fiber is placed close to the wall as shown in Figure 9. Even when the fiber tip is placed at a distance from the wall, the bubble moves toward the wall with a collapsing and rebounding motion as shown in Figure 9(b) and Figure 9(c). The lower parts of Figures 9(a)-(c) show the resultant impulsive force measured by the impulsive force sensor synchronized with high-speed observation. The impulsive force reaches a peak value at the primary collapse of the bubble rather than at the instant of laser irradiation. Figure 10 shows bubble collapse behavior and the associated impact waves near the wall that is parallel to the fiber. The non-spherical bubble forms in contact with the wall surface and collapses toward the attaching wall surface when the laser fiber is placed close to the wall, as shown in Figure 10(a). The impulsive force also reaches a peak value at the point of primary collapse of the bubble in each condition.
For the vertical and horizontal wall surfaces, respectively, Figure 11 and Figure 12 show the relationship between impulsive force F and distance γ (=x/Rmax or h/Rmax) from the fiber tip to the wall surface. These plots are the average of 10 times and the error bars indicates the standard deviation. The impulsive force becomes larger with a decrease in distance γ. The magnitude of this impact is comparable with previous studies (such as reference [11] ) and can cause erosion on the material surface. In the case of the fiber arranged perpendicular to the wall, the impulsive force reaches its maximum value at a certain distance because placing the laser fiber directly at the wall limits cavitation bubble expansion, thereby restricting the amplitude of the resultant shock wave. Therefore, it is found that the high impact on the wall can be given effectively by the optimum distance
![]() (a) (b) (c)
(a) (b) (c)
Figure 9. Bubble collapse behavior and the resultant impulsive force near a vertical wall surface. (a) x = 0.33 mm; (b) x = 2.83 mm; (c) x = 3.79 mm.
![]() (a) (b) (c)
(a) (b) (c)
Figure 10. Bubble collapse behavior and the resultant impulsive force near a horizontal wall surface. (a) h = 0.28 mm; (b) h = 3.11 mm; (c) h = 4.03 mm.
![]()
Figure 11. Changes in impulsive force (vertical wall surface).
![]()
Figure 12. Changes in impulsive force (horizontal wall surface).
from a wall surface.
4. Conclusions
The bubble induced by a Ho:YAG fiber laser for a transurethral ureterolithotripsy is observed in detail using a high-speed video camera. Characteristic collapsing behavior of the bubble near the wall and its resulting impulsive force on the wall are found for various laser irradiation conditions.
1) The maximum bubble radius becomes larger with an increase in laser power and fiber diameter.
2) The impulsive force reaches its maximum value at the point of primary collapse of the bubble rather than at the instant of laser irradiation.
3) In the case of the fiber arranged perpendicular to the wall, there is the optimum fiber placement to give high impact on the wall surface because the cavitation bubble expansion and the resultant shock wave are limited by the existence of the wall.
Acknowledgements
This work was supported by JSPS KAKENHI Grant Number 24560211.
Nomenclature
d: Fiber diameter (mm)
E: Laser power (J)
F: Impulsive force (N)
h: Distance from fiber tip to surface of horizontal wall (mm)
r: Equivalent bubble radius (mm)
Rmax: Maximum bubble radius (mm)
tc: Rayleigh’s bubble collapse time in Ref. [11] (s)
Tw: Temperature of water (K)
x: Distance from fiber tip to surface of vertical wall (mm)
b: Dissolved oxygen content (mg/L)
γ: Ratio of distance from fiber tip to wall surface to maximum bubble radius (=x/Rmax or h/Rmax)