The Approximation of Hermite Interpolation on the Weighted Mean Norm

Xin Wang¹, Chong Hu², Xiuxiu Ma³

¹Department of Mathematics and Computer, Baoding University, Baoding, China.
²Institute of Nuclear Technology, China Institute of Atomic Energy, Beijing, China.
³Institute of Mathematical, North China Electric Power University, Baoding, China.

DOI: 10.4236/ajcm.2015.53033                

Abstract

We research the simultaneous approximation problem of the higher-order Hermite interpolation based on the zeros of the second Chebyshev polynomials under weighted Lp-norm. The estimation is sharp.

Keywords

Hermite Interpolation operator，Chebyshev polynomial，derivative approximation

1. Introduction

For 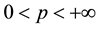and a non-negative measurable function u, the space 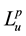is defined to be the set of measurable 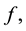such that

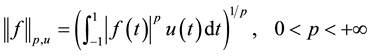
is finite. Of course, when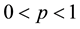, 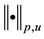is not a norm; nevertheless, we keep this notation for convenience.

For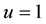, this is the usual 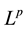space. For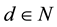, we write for the space of functions that have dth continuous derivative on.

We introduce a few notations. If 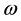is a Jacobi weight function, we write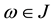. Let

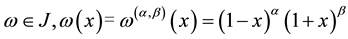. The Jacobi polynomials 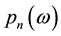are orthogonal polynomials with respect to the weight function, i.e.

It is well known that has distinct zeros in. These zeros are denoted by and the following order is assumed:

Later, when we fix, we shall write instead of.

For a given integer and, the Hermite interpolation is defined to be the unique polynomial of degree, denoted by, satisfying

for, where, if or then we have no interpolation at 1 or −1. We shall fix the integers and for the rest of the paper, and omit them from the notations. Thus, for example, we shall write instead of. Let

Vertesi and Xu [1] , Nevai and Xu [2] , and Pottinger considered the simultaneous approximation by Hermite interpolation operators.

We have researched the simultaneous approximation problem of the lower-order Hermite interpolation based on the zeros of Chebyshev polynomials under weighted Lp-norm in references [3] -[5] . We will research the simultaneous approximation problem of the higher-order Hermite interpolation in this article.

Let

be the zeros of, the nth degree Chebyshev polynomial of the second kind. For

, let be the polynomial of degree at most 3n − 1 which satisfies

Then the Hermite interpolation polynomial is given by

(1.1)

where

(1.2)

(1.3)

(1.4)

(1.5)

Theorem 1.

Let be defined as (1.1), for and, then we have

2. Some Lemmas

Lemmas 1. [6] Let be defined as (1.1), then

where, , is defined as function at before the commencement of the Taylor series of.

Lemma 2. [7]

If, then there exists a algebraic polynomial of degree at most such that

Let

be the zeros of, here, the nth degree Chebyshev polynomial of

the second kind. For, the well-known Lagrange interpolation polynomial of based on is given by

(2.1)

where

(2.2)

(2.3)

(2.4)

Lemma 3. [7] Let be defined as (2.4), for, and, we have

3. The Proof of Theorem 1

For, let be the polynomial of degree at most which satisfies Lemma 2. By the uniqueness of Hemite interpolation polynomial, it can be easily checked that,

(3.1)

We can conclude that

(3.2)

Firstly, we estimate. By (3.1), we have

(3.3)

Firstly, we estimate,

(3.4)

Let

(3.5)

be the polynomial of degree. By the uniqueness of Lagrange interpolation polynomial, it can be easily checked that,

(3.6)

By (3.5), (3.6) and Lemma 3, we can derive

(3.7)

Firstly, we estimate. Let

(3.8)

then

(3.9)

From Lemma 2 and (3.8), (3.9), we have that for

(3.10)

For, we have

(3.11)

We can conclude

(3.12)

Secondly, we estimate, and by Lemma 2, we get

(3.13)

Similarly

(3.14)

By (3.12), (3.13) and (3.14), we have

(3.15)

Similarly, we get

(3.16)

(3.17)

By (3.15), (3.16) and (3.17), we get

(3.18)

Similarly, we get

(3.19)

(3.20)

Secondly, we estimate, from Lemma 2,

(3.21)

From (3.2), (3.3), and (3.21), we can obtain the upper estimate

Funding

Hebei Science and Technology Research Universities Youth Fund project (QN20132001).

Conflicts of Interest

The authors declare no conflicts of interest.

References

[1]	Szabados, J. and Vestesi, P. (1992) A Survey on Mean Convergence of Interpolatory Processes. Journal of Computational and Applied Mathematics, 43, 3-18. http://dx.doi.org/10.1016/0377-0427(92)90256-W
[2]	Szabados, J. and Vertesi, P. (1990) Interpolation of Functions. World Scientific, Singapore.
[3]	Wang, X. (2011) The Approximation of Hermite Interpolation on the Weighted Mean Norm. Journal of Tianjin Normal University, 31, 7-12.
[4]	Xia, Y. and Xu, G.Q. (2010) The Approximation of Hermite Interpolation on the Weighted Mean Norm. Journal of Tianjin Normal University, 31, 6-10.
[5]	Xu, G.Q., Cui, R. and Wang, X. (2009) The Simultaneous Approximation of Quasi-Hermite Interpolation on the Weighted Mean Norm. International Journal of Wavelets, Multiresolution and Information Processing, 7, 825-837. http://dx.doi.org/10.1142/S0219691309003276
[6]	Xie, T.F. and Zhou, S.P. (1998) Real Function Approximation Theory. Hangzhou University Press, Hangzhou.
[7]	Kingore, T. (1993) An Elementary Simultaneous Polynomials. Proceedings of the American Mathematical Society, 118, 529-536. http://dx.doi.org/10.1090/S0002-9939-1993-1129881-X