1. Introduction
Proportional-integral-derivative (PID) control is certainly the most widely used control strategy today. It is estimated that over 90% of control loops employ PID control [1] . Over the last half-century, a great deal of academic and industrial effort has focused on improving PID control, but the trouble, which often suffers a serious loss of performance due to integrator windup, was not resolved in principle before general integral control [2] appeared in 2009.
After that various general integral controls along with the design techniques were presented. For example, general concave integral control [3] , general convex integral control [4] , constructive general bounded integral control [5] and the generalization of the integrator and integral control action [6] were all developed by resorting to an ordinary control along with a known Lyapunov function; general integral control designs based on linear system theory, sliding mode technique, feedback linearization technique, singular perturbation technique, equal ratio gain technique and power ratio gain technique were presented by [7] - [12] , respectively. Although general integral control has developed rapidly in theory, its practical applications have not been reported. Therefore, in consideration of its good control performance, it is appropriate at this time to compare the simplest general integral control (GI-PD) with PID control in order to promote its applications in practice.
Motivated by the cognition above, in conjunction with a second order uncertain nonlinear system, this paper makes some comparisons between PID control and GI-PD control. The main contributions are: under GI-PD control, it is demonstrated that: 1) the system matrix can be stabilized more easily; 2) it is more effective to deal with the uncertain nonlinear actions; 3) the trouble caused by integrator windup is resolved in principle, and then it has the better control performance; 4) the harmonization of the integral control action and PD control action can be achieved. Moreover, design example and simulation results verify the justification of our conclusions again. All these mean that GI-PD control has the stronger robustness and higher control performance than PID control. Consequently, GI-PD control has broader application prospects than PID control.
Throughout this paper, we use the notation  and
and 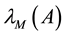 to indicate the smallest and largest eigenvalues, respectively, of a symmetric positive-define bounded matrix
to indicate the smallest and largest eigenvalues, respectively, of a symmetric positive-define bounded matrix , for any
, for any . The norm of vector
. The norm of vector 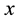 is defined as
is defined as , and that of matrix
, and that of matrix 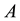 is defined as the corresponding induced norm
is defined as the corresponding induced norm  .
.
The remainder of the paper is organized as follows: Section 2 describes the system under consideration, assumption, and stability analysis of the closed-loop system. Section 3 compares Hurwitz stability of the system matrix. Section 4 demonstrates the robustness against the uncertain nonlinearity. Section 5 analyzes the control action. Example and simulation are provided in Section 6. Conclusions are presented in Section 7.
2. Problem Formulation
Consider the following controllable nonlinear system,
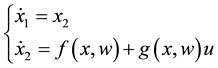 (1)
(1)
where  is the state;
is the state; 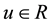 is the control input;
is the control input;  is a vector of unknown constant parameters and disturbances; the function
is a vector of unknown constant parameters and disturbances; the function  is the uncertain nonlinear actions, the uncertain nonlinear function
is the uncertain nonlinear actions, the uncertain nonlinear function  is continuous in
is continuous in 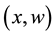 on the control domain
on the control domain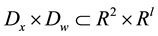 .
.
Assumption 1: There is a unique pair ![]() that satisfies the equation,
that satisfies the equation,
![]() (2)
(2)
so that ![]() is the desired equilibrium point and
is the desired equilibrium point and ![]() is the steady-state control that is needed to maintain equilibrium at
is the steady-state control that is needed to maintain equilibrium at![]() .
.
Assumption 2: Suppose that the functions ![]() and
and ![]() satisfy the following inequalities,
satisfy the following inequalities,
![]() (3)
(3)
![]() (4)
(4)
![]() (5)
(5)
![]() (6)
(6)
for all ![]() and
and![]() , where
, where![]() ,
, ![]() ,
, ![]() and
and ![]() are all positive constants.
are all positive constants.
For comparing PID and GI-PD control, the control law is taken as,
![]() (7)
(7)
where![]() ,
, ![]() and
and ![]() are the controller gains;
are the controller gains; ![]() and
and ![]() are the integrator gains.
are the integrator gains.
It is worth to note that although the control law (7) is GI-PD control, it is reduced to PID control as ![]() and
and![]() . Thus, under GI-PD and PID control, the closed-loop system can be written as the same form, that is,
. Thus, under GI-PD and PID control, the closed-loop system can be written as the same form, that is,
![]() (8)
(8)
By assumption 1 and choosing ![]() to be large enough, and then setting
to be large enough, and then setting ![]() and
and ![]() of the system (8), obtain,
of the system (8), obtain,
![]() (9)
(9)
Therefore, we ensure that there is a unique solution![]() , and then
, and then ![]() is a unique equilibrium point of the closed-loop system (8) in the domain of interest.
is a unique equilibrium point of the closed-loop system (8) in the domain of interest.
Now, defining![]() , and substituting (9) into (8), obtain,
, and substituting (9) into (8), obtain,
![]() (10)
(10)
where
![]()
and ![]() is a
is a ![]() matrix, all its elements is equal to zero except for
matrix, all its elements is equal to zero except for
![]() .
.
Moreover, it is worthy to note that the function ![]() is integrated into
is integrated into![]() ,
, ![]() and
and![]() .
.
By linear system theory, if the matrix ![]() is Hurwitz, and then for any given positive define symmetric matrix
is Hurwitz, and then for any given positive define symmetric matrix![]() , there is a unique positive define symmetric matrix
, there is a unique positive define symmetric matrix ![]() that satisfies Lyapunov equation
that satisfies Lyapunov equation![]() . Therefore, there exists a quadratic Lyapunov function,
. Therefore, there exists a quadratic Lyapunov function,
![]() (11)
(11)
Thus, using ![]() as Lyapunov function candidate, and then its time derivative along the trajectories of the closed-loop systems (10) is,
as Lyapunov function candidate, and then its time derivative along the trajectories of the closed-loop systems (10) is,
![]() (12)
(12)
where![]() .
.
Now, using the inequalities (3), (5), (6) and definition of![]() , we have,
, we have,
![]() (13)
(13)
where ![]() is a positive constant.
is a positive constant.
Substituting (13) into (12), obtain,
![]() (14)
(14)
It is obvious that if
![]() (15)
(15)
holds, we have![]() .
.
Using the fact that Lyapunov function ![]() is a positive define function and its time derivative is a negative define function if the inequality (15) holds, we conclude that the closed-loop system (10) is stable. In fact,
is a positive define function and its time derivative is a negative define function if the inequality (15) holds, we conclude that the closed-loop system (10) is stable. In fact, ![]() means
means ![]() and
and![]() . By invoking LaSalle’s invariance principle, it is easy to know that the closed-loop system (10) is exponentially stable.
. By invoking LaSalle’s invariance principle, it is easy to know that the closed-loop system (10) is exponentially stable.
Discussion 1: From the demonstration above, it is obvious that: for ensuring that the closed-loop system is exponentially stable, two key conditions are indispensable, that is, one is that the system matrix ![]() is Hurwitz and another is that the inequality (15) holds. Thus, for comparing GI-PD control with PID control, the differences of two key conditions above must be demonstrated. Moreover, the analysis of PID and GI-PD control action and performance is unnecessary, too. All these are addressed in the following Sections, respectively.
is Hurwitz and another is that the inequality (15) holds. Thus, for comparing GI-PD control with PID control, the differences of two key conditions above must be demonstrated. Moreover, the analysis of PID and GI-PD control action and performance is unnecessary, too. All these are addressed in the following Sections, respectively.
3. Hurwitz Stability
The polynomials of the system matrix ![]() under PID control and GI-PD control are,
under PID control and GI-PD control are,
![]() (16)
(16)
![]() (17)
(17)
By Routh’s stability criterion and the polynomials (16) and (17), Hurwitz stability conditions of the system matrix ![]() under PID control and GI-PD control can be obtained as follows:
under PID control and GI-PD control can be obtained as follows:
Under PID control, if![]() ,
, ![]() and
and ![]() are all positive constants, and the inequality,
are all positive constants, and the inequality,
![]() (18)
(18)
holds, and then the system matrix ![]() is Hurwitz.
is Hurwitz.
Under GI-PD control, if![]() ,
, ![]() and
and ![]() are all positive constants, and the inequality,
are all positive constants, and the inequality,
![]() (19)
(19)
holds, then the system matrix ![]() is Hurwitz.
is Hurwitz.
Compared with Hurwitz stability conditions of PID control, the one of GI-PD control has the following features:
1) The striking feature is that the role of gain ![]() manifests itself in two aspects: one is that the gain
manifests itself in two aspects: one is that the gain ![]() produces a special term
produces a special term ![]() such that the gain
such that the gain ![]() is enhanced, and then for achieving Hurwitz stability, it is not necessary to increase the value of
is enhanced, and then for achieving Hurwitz stability, it is not necessary to increase the value of![]() , even
, even ![]() can be taken as a negative constant; another is that the gain
can be taken as a negative constant; another is that the gain ![]() educes another special term
educes another special term ![]() such that it makes the inequality (19) holds more easily, and then for achieving Hurwitz stability, it is unnecessary to increase
such that it makes the inequality (19) holds more easily, and then for achieving Hurwitz stability, it is unnecessary to increase ![]() and/or
and/or![]() , or decrease
, or decrease![]() .
.
2) As![]() , if the system matrix
, if the system matrix ![]() with PID control is Hurwitz, and then the one with GI-PD control and
with PID control is Hurwitz, and then the one with GI-PD control and ![]() must be Hurwitz.
must be Hurwitz.
3) The gain ![]() is indispensable. For ensuring Hurwitz stability,
is indispensable. For ensuring Hurwitz stability, ![]() seems to be unfavorable, but
seems to be unfavorable, but ![]() is absolutely favorable.
is absolutely favorable.
4) There are two additional gains ![]() and
and ![]() in GI-PD control law. Therefore, more information can be exploited to stabilize the system matrix
in GI-PD control law. Therefore, more information can be exploited to stabilize the system matrix ![]() than PID control.
than PID control.
All these means that the system matrix ![]() under GI-PD control can be stabilized more easily than PID control.
under GI-PD control can be stabilized more easily than PID control.
4. Robustness against Uncertain Nonlinear Actions
For comparing PID control and GI-PD control robustness against uncertain nonlinear actions, we need to solve the Lyapunov equation ![]() with any given positive define symmetric matrix
with any given positive define symmetric matrix ![]() to obtain the solution of the matrix
to obtain the solution of the matrix![]() .
.
Under PID control, ![]() is,
is,
![]()
![]()
![]()
Under GI-PD control, ![]() is,
is,
![]()
![]()
![]()
For the sake of simplicity, we just consider the case of ![]() and
and![]() . Thus, by comparing
. Thus, by comparing ![]() with
with![]() , we have,
, we have,
![]() as
as ![]()
and then by ![]() and
and ![]() as
as![]() , we obtain,
, we obtain,
![]() as
as ![]()
where
![]()
It is easy to see that there exists ![]() such that
such that ![]() holds for all
holds for all![]() , and then by the in-
, and then by the in-
equality (15), we can conclude that GI-PD control is more effective to deal with the uncertain nonlinear actions than PID control. This means that under the case of the same gains![]() ,
, ![]() ,
, ![]() and
and ![]() along with moderately choosing
along with moderately choosing ![]() and
and![]() , GI-PD control can be designed to have the stronger robustness against the uncertain nonlinear actions than PID control.
, GI-PD control can be designed to have the stronger robustness against the uncertain nonlinear actions than PID control.
Discussion 2: Although the demonstration above aims at a special case, it is not hard to conclude that by synthesizing all the gains![]() ,
, ![]() ,
, ![]() ,
, ![]() and
and![]() , GI-PD control can be designed to have the stronger robustness with respect to the uncertain nonlinear actions than PID control since more information can be used to decrease the value of
, GI-PD control can be designed to have the stronger robustness with respect to the uncertain nonlinear actions than PID control since more information can be used to decrease the value of![]() .
.
5. Analysis of Control Action
No matter PID control or GI-PD control, Proportional and Derivative control actions are all identical, that is:
Proportional control action is proportional to the error. If the error is small, its corrective effect is small, and vice versa.
Derivative control action is proportional to the rate at which the error is changing. Its corrective effect attempts to anticipate a large error and prevent this future error.
Compared with PID control, the main difference of GI-PD control is the integrator, that is, the error derivative is introduced into the integrator. This lead to an important change of the integral control action, that is,
Under PID control, the integrator is![]() . Obviously, the integral control action continues to increase unless the error passes through zero, and then for making the integral control action tends to a constant, the error is usually needed to pass through zero repeatedly. Just the stubborn increase of integral control action results in the integrator windup.
. Obviously, the integral control action continues to increase unless the error passes through zero, and then for making the integral control action tends to a constant, the error is usually needed to pass through zero repeatedly. Just the stubborn increase of integral control action results in the integrator windup.
Under GI-PD control, the integrator is![]() . Thus, as
. Thus, as![]() , the integral control action does not increased and remains a constant; if the integral control action is large,
, the integral control action does not increased and remains a constant; if the integral control action is large, ![]() increases, and the integral control action instantly decreases, and vice versa. This shows that the effect of
increases, and the integral control action instantly decreases, and vice versa. This shows that the effect of ![]() is an attempt to anticipate and prevent an excess integral control action, and then integrator windup can be removed completely. Moreover, as
is an attempt to anticipate and prevent an excess integral control action, and then integrator windup can be removed completely. Moreover, as![]() , the integral control action is equivalent to the accumulation of PD control action. This means that the harmonization of the integral control action and PD control action can be achieved. All these means that GI-PD control has the better control performance than PID control.
, the integral control action is equivalent to the accumulation of PD control action. This means that the harmonization of the integral control action and PD control action can be achieved. All these means that GI-PD control has the better control performance than PID control.
6. Example and Simulation
Consider the pendulum system [13] described by,
![]()
where![]() ,
, ![]() is the angle subtended by the rod and the vertical axis, and
is the angle subtended by the rod and the vertical axis, and ![]() is the torque applied to the pendulum. View
is the torque applied to the pendulum. View ![]() as the control input and suppose we want to regulate
as the control input and suppose we want to regulate ![]() to
to![]() . Now, taking
. Now, taking![]() ,
, ![]() , the pendulum system can be written as,
, the pendulum system can be written as,
![]()
and then it can be verified that ![]() is the steady-state control that is needed to maintain equilibrium at the origin.
is the steady-state control that is needed to maintain equilibrium at the origin.
GI-PD control law is,
![]()
It is worth to note that as![]() , the control law above is PID control law. Thus, the closed-loop system can be written as,
, the control law above is PID control law. Thus, the closed-loop system can be written as,
![]()
where
![]() ,
,
![]() ,
,
and
![]() .
.
The normal parameters are ![]() and
and![]() , and in the perturbed case,
, and in the perturbed case, ![]() and
and ![]() are reduced to 1 and 5, respectively, corresponding to double the mass. Thus, we have,
are reduced to 1 and 5, respectively, corresponding to double the mass. Thus, we have,
![]() (20)
(20)
Now, taking![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() and
and![]() , and using Routh’s stability criterion, we have,
, and using Routh’s stability criterion, we have,
![]() (21)
(21)
![]() (22)
(22)
and then the system matrix ![]() under GI-PD control and PID control is Hurwitz. Thus, solving Lyapunov equation
under GI-PD control and PID control is Hurwitz. Thus, solving Lyapunov equation![]() , we obtain
, we obtain ![]() and
and![]() , and then by the inequality (15) and the bound condition (20), we have,
, and then by the inequality (15) and the bound condition (20), we have,
![]() (23)
(23)
![]() (24)
(24)
Thus, Under PID and GI-PD control, the asymptotical stability of the whole closed-loop system can all be ensured. Consequently, the simulations are implemented under the normal and perturbed cases, respectively. Moreover, in the perturbed case, we consider an additive impulse-like disturbance ![]() of magnitude 60 acting on the system input between 30s and 31s.
of magnitude 60 acting on the system input between 30s and 31s.
Figure 1 and Figure 2 showed the simulation results under normal and perturbed cases. From the simulation results and design procedure, the following observations can be made: 1) by Hurwitz stability conditions (21) and (22), stability margin of the system matrix ![]() under GI-PD control is larger than the one of PID control; 2) by stability conditions (23) and (24), GI-PD control has the stronger robustness with respect to the uncertain nonlinear action than PID control; 3) by Figure 1 and Figure 2, under GI-PD control, no matter normal case or perturbed case, the optimum responses can all be achieved in the whole control domain. However, under PID control, the overshoot is proportional to the initial error and the settling time is long. Due to the above experimental results, it could be concluded that GI-PD control has more broad application prospects than PID control.
under GI-PD control is larger than the one of PID control; 2) by stability conditions (23) and (24), GI-PD control has the stronger robustness with respect to the uncertain nonlinear action than PID control; 3) by Figure 1 and Figure 2, under GI-PD control, no matter normal case or perturbed case, the optimum responses can all be achieved in the whole control domain. However, under PID control, the overshoot is proportional to the initial error and the settling time is long. Due to the above experimental results, it could be concluded that GI-PD control has more broad application prospects than PID control.
![]()
Figure 1. System output under the normal case.
![]()
Figure 2. System output under the perturbed cases.
7. Conclusion
In conjunction with a second order uncertain nonlinear system, this paper makes some comparisons between PID control and GI-PD control. The main contributions are: under GI-PD control, it is demonstrated that: 1) the system matrix can be stabilized more easily; 2) it is more effective to deal with the uncertain nonlinear actions; 3) the trouble caused by integrator windup is resolved in principle, and then it has the better control performance; 4) the harmonization of the integral control action and PD control action can be achieved. Moreover, design example and simulation results verify the justification of our conclusions again. All these means that GI-PD control has the stronger robustness and higher control performance than PID control. Consequently, GI-PD control has broader application prospects than PID control.