Maximally Entangled States in the Hydrogen Molecule: The Role of Spin and Correlation ()
1. Introduction
Since Einstein, Podolsky and Rosen [1] and Schrödinger [2] investigated the non-classical properties of quantum systems and entered new concept as entanglement in to quantum physics, it had become strange property in interaction between particles. Recent study of the entanglement and achievement in this respect has become a useful resource for quantum communications and information processing [3] such as quantum teleportation [4,5], super dense coding [6], quantum key distribution [7], and quantum cryptography [8]. Entanglement also has been suggested as a quantitative measure for electron-electron (e-e) correlation in many body systems [9,10]. As a simple illustration of entanglement one can say that, if there is no way to write the states of two particles as a product of the states of two sub systems in the Hilbert space, then there will be an entangled system [11]. A lot of investigation has been done about measuring entanglement, such as the Wooters’ measure [12] and the Schliemann’s measure [13,14]. Through Gittings’ investigation [15], it is shown that all these entanglement measures are not suitable but the Zanardi’s measure [16,17] satisfies all desirable properties of entanglement measurement for fermionic systems.
H2 molecule is the simplest two electron systems that can be used to implement a many body calculation based on Hubbard model [18]. Traditional Hubbard model which is a priory many-body approach usually is used as a first attempt to calculate entanglement. This model gives maximum entanglement by setting e-e interaction parameter U = 0, which is a controversial conclusion [17,19,20]. To overcome this controversial conclusion a new calculation i.e. going beyond Hubbard model for obtaining entanglement of the non-magnetic ground and magnetic excited states of H2 molecule was done. The H2 molecule is one of the simplest realistic two electrons correlated systems in nature which our model can be implemented with considering all direct and exchange interaction terms beyond Hubbard model. This implementation lets us consider correlation effects more accurately in the wavefunctions. Zanardi’s measurement was employed for calculating entanglement. To find the effect of the spin on the entanglement of states; the ground and excited state of 
was investigated and surprisingly the results give maximally entangled states with non-zero U value. We also discuss the difference between maximally, moderately and zero entangled states based on their spin and correlation.
2. Calculation Method
The complete Hubbard Hamiltonian is defined as [21]:
(1)
where 
is hopping and on-site for electrons under the influence of both hydrogen nuclei and 
is all electron-electron interactions. The first term contains noninteracting part of Hamiltonian which can be written as:
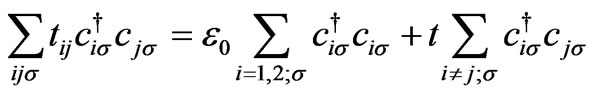(2)
(3)
where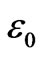 
is the energy of atomic orbital, 
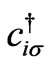and 
are fermionic creation and annihilation operators respectively on site i with spin s, and t stands for the hopping integral between two H atomic sites of the electrons with the same. The second term of Hamiltonian that contains e-e interaction part can be written as:
(4)
where Vijlm stands for all 
Coulomb interaction parameters between electrons, which is U as on-site Coulomb repulsion or V1111 and V2222, J as inter-site Coulomb repulsion or V1212 and V2121 and 
is density operator. The last terms X1 and X2 are the exchange interactions parameters that can only be interpreted by quantum mechanics and are demonstrated as:
(5)
We consider two electrons of 1s orbital of two H atoms of H2 molecule with both spin orientations up and down, therefore we have four states for a single electron and as the result for their combination there are C(4,2)=6 states which are represented with notation 
as :
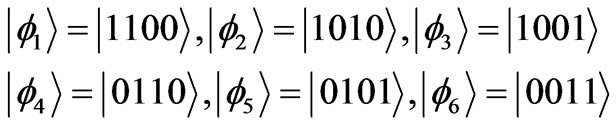(6)
With these sets of states, the Hamiltonian parameters were calculated and Hamiltonian matrix is:
(7)
All the diagonal elements contain a term tii, where it is twice 
and this one is roughly two times of the energy of an electron in the 1s state of atomic hydrogen i.e. 
and are not written here. Using ab initio energies for H2 and the values of 
and t from [22] the Hamiltonian parameters, U, J, X1 and X2, were evaluated and are given in Table 1.
Entanglement measurement is defined by von Neumann’s entropy as [16]:
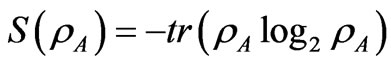(8)
where A is the label of one of the subsystems which in our model is 1s orbital of one of the Hydrogen atoms and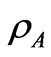 
is reduced density matrix defined as:
(9)
where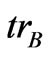 
stands for tracing over all sites except the B sites and 
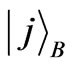is eigenstates for B subsystem 
i.e. 
and
. After this calculation, the reduced density matrix for the ground state (not normalized) becomes:
(10)
For other states, reduced density matrices have been evaluated accordingly and their entanglements were calculated. The resultant entanglement values are listed in Table 2. By action of S2 operator on the 
eigenstates, one could find total spin of each state in
Table 1. The calculated values of Hamiltonian parameters.
which 
and 
have S2 = 0, but 
has S2 = 2. Some of the eigenvalues and eigenstates of hydrogen molecule are dependent to parameters of Hamiltonian via
, where 
and
. All eigenvalues and S2, eigenstates (not normalized) and also their related Sz are summarized in Table 2.
3. Results and Discussion
Based on the results summarized in Table 2 the wavefunction of the ground and third excited state are dependent upon e-e correlation parameters via 
with
. Both of these states are nonmagnetic (S = 0). The wavefunctions of the first and second excited states are explicitly independent from Hamiltonian parameters. There is no dependency of any wavefunction to exchange interaction X2. Using parameters of Table 1 and von Neumann’s entropy given by Equation (6) an entanglement value of 
was obtained for ground and third excited states. The maximum available value of entanglement is 
for a system with the Hilbert space dimension of the smaller subsystem as d [23]. Accordingly, for H2 molecule the maximum available entanglement is 2 and much closed values were obtained for these states. The results of maximally entangled ground and third excited states can be explained by the corresponding wave functions of these states. The wavefunctions of the ground and third excited states are superposition of four body basis of the systems i.e. 
and 
with equal coefficients since the value of 
become 1 by considering the values of Table 1, therefore is maximum mixing and hence maximum entanglement. From Table 2, one can realize that the first excited state 
is a spin triplet state with S = 1 and its wavefunction is independent from correlation parameters. The value of entanglement for the Sz = 0 eigenfunction is 1 while its value for the eigenfunction with Sz = ±1 is zero. The difference between entanglements of the degenerate wavefunctions with different Sz can be explained by their related wavefunctions. The 
state is a linear combination of 
and 
whereas the 
state are separable (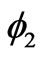
or
). The importance of the nonzero spin of the first excited state is that this kind of state can be detected by Electron Paramagnetic Resonance (EPR) under some condition [24]. When the magnetic field is absent the wavefunction is a superposition of the degenerate wavefunctions with different Sz values. The calculated entanglement of this degenerate state is zero. However, after switching on the magnetic field, a wavefunction with distinct value of entanglement emerges. According to Table 2 the values of the Sz and entanglement for this state are 0 and 1 respectively. This might be a way for switching to a pure entangled state.
The second excited state 
is nonmagnetic, S = 0, where the wavefunction is independent from correlation parameters. The calculated entanglement is 1. The wavefunction of this state is only a linear combination of two basis set 
and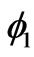
, hence its entanglement is smaller than ground and third excited states. By comparing the values of the entanglement listed in Table 2, one can conclude that the nonmagnetic states (S = 0) in which the wavefunction depend on interaction parameters are maximally entangled and the magnetic states whose wavefunctions are independent from correlation parameters are not entangled at all. Using von Neumann’s entropy given by Equation (6), the variation of entanglement for the ground state of H2 molecule with respect to the combination of correlation parameters 
were calculated and is plotted in figure 1. It is clear from this figure that the value of entanglement is maximum for x = 0 (U = J). This conclusion resolves the unphysical gesture previously reported by other groups [17,19,20] who obtained maximally entangled ground state with U = 0. The U = 0 result imply that the maximally entangled ground state is not attainable for the H2 molecule since the ab initio value of U is 11.8 (Table 1), which is very far from zero. But the conclusion of our model indicates that the maximally entangled ground state is for U = J. This has a meaningful physical interpretation which states that in such physical system where the inter-atomic distance is very small, the on-site Columbic repulsion U can be very close to the inter-site Columbic repulsion J. Indeed the H2 molecule is the best example of such systems when the inter-atomic distance is minimum or d = 0.7A, the ab initio value of U and J from Table 2 gives x = 0.06. Also by using Hubbard parameters of Table 1, we obtained x = 0.06 and this value of x gives maximally entangled ground state. The maximum entanglement value for x = 0.06 is 1.99. As is observable in Figure 1, for the extreme limit or U much greater than t, i.e. strongly correlated systems the entanglement becomes smaller and tends to 1. The non-magnetic property of this state sets a limit on minimum available entanglement for this state. This point can be explained by using eigenfunction listed in Table 2. For 
much greater than 1, one of the
or 
goes to zero and the other one becomes very large. In both cases the ground state wavefunctions listed in Table 2 reduce from extension over the four components to an extension over two components similar to 
state. Therefore the corresponding value of entanglement reduces from 2 to 1. This also can be explained by tendency of the strongly correlated systems with U much greater than t to unpaired electronic configuration in atomic orbital such as 
and 
states. Such states tend to have parallel spin and as the result the entanglement reduces. In the case of H2 where the values of t, U and J are the same order of magnitude (See Table 1) and as the consequence x is much close to zero the molecule is in moderately correlated regime and one can obtain the maximum available entanglement as it is apparent in Figure 1. In this scheme both spatial and spin have to be considered for correlated wavefunction [17]. Neglecting exchange interaction in our model (X1 = X2 = 0), results to considerable change in energy levels of the system (see
Table 2). However, the dependence of the ground and third excited state wavefunctions on the exchange parameters is only to X1 and putting X1 = 0 yields x = 0.07
(U-J)/4(t+X1)
Figure 1. Entanglement for the ground state of Hydrogen molecule versus e-e interaction arameters.
and as the result the maximum entanglement becomes 1.99 which is the same as the case of nonzero X1 (x = 0.06). Hence in order to have maximally entangled states, the most effective parameters are direct Columbic interaction parameters U and J and exchange interaction parameters do not alter the value of entanglement significantly.
4. Conclusions
A many electron calculation on a simplest realistic two electron system i.e. H2 molecule was applied. Going beyond traditional Hubbard model lets to account correlation effects accurately in the many electron wavefunction of the ground and excited states. Using ab initio e-e interaction parameters gives a moderately correlated regime for the molecule and consequences maximally entangled ground and third excited state. The wavefunctions of the not magnetic (S = 0) ground and third excited states explicitly depend on correlation parameters whereas the first excited states which is magnetic (S2 = 2 and Sz 
0) is not entangled. The second excited state is not magnetic but its wavefunction does not depend on correlation parameters therefore it is a moderately entangled state. In any case, by switching on a magnetic field an entangled state with Sz = 0 can be extracted from a not entangled degenerate magnetic state. We suggest that in a realistic molecular scale system, there is two criteria for finding maximally entangled electronic states, first the system should be in moderately correlated regime and second the system should have a non-magnetic (Sz = 0) electronic state.