On the Relationship between Estimate and Its t Value

Yuji Matsuoka¹, Shigeyuki Hamori^2*

¹Graduate School of Economics, Kobe University, Kobe, Japan.
²Faculty of Economics, Kobe University, Kobe, Japan.

DOI: 10.4236/tel.[phone]                

Abstract

It is generally believed that the signs of the estimated coefficient and its t value should be the same. This paper, however, shows that there may be an inconsistency in the signs of the estimated coefficient and its t value when we use the group mean dynamic OLS estimator developed by Pedroni (2001).

Keywords

t Value, Group Mean Dynamic OLS Estimator, Panel Data

1. Introduction

This paper shows the possibility of inconsistency in the signs of the group mean dynamic OLS estimator and its t value. According to basic econometrics and statistics, the t value is calculated by dividing the estimated coefficient by its standard error. Because the standard error is always positive, the sign of the t value becomes identical to the sign of the estimated coefficient [1] [2] .

Pedroni [3] developed the group mean dynamic OLS estimator―a useful technique to obtain an estimator for a dynamic heterogeneous panel model. However, because this estimator is calculated by summing the estimation result of every cross section, there is a possibility of inconsistency in the signs. We provide a very simple example of this phenomenon.

The remainder of this paper is as follows: Section 2 provides the model; Section 3 shows the simulation; Section 4 concludes.

2. Model

We consider the estimation of the following model by using dynamic OLS.

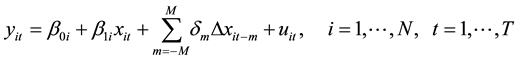
where 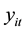is the dependent variable, is the independent variable, and 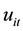is the error term. To obtain the

group mean dynamic OLS estimator, we separately estimate this equation using every cross section. Then, we calculate the estimator with each estimated coefficient and 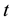value in the following manner:

,

.

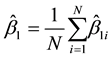3. Simulation

3.1. Simulation Design

We show the possibility of inconsistency using a simulation. For simplicity, we assume that and equal 2 and 1000, respectively. Furthermore, we drop the lag and lead terms. The model is rewritten as follows:

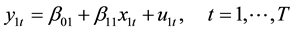
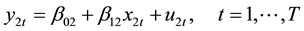
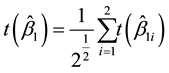
The simulation strategy is as follows. First, we provide the values of, , 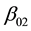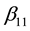and as

Case 1:

Case 2:

,

Case 3:

.

Second, we randomly generate the values of and and and using standard normal distributions. Then, we calculate the values of and. Third, we estimate the above equation and calculate the

estimator by using the generated data. This simulation is performed 10,000 times using STATA.

3.2. Simulation Results

Case 1 and Case 2:

In this case, we expect that both cross sections take identical signs. Thus, we do not need to be concerned with the inconsistency. The result also shows consistency: every 10,000 samples take the same signs in the group mean dynamic OLS estimator and its t value.

Case 3:

In this case, the estimation of each cross section is expected to take opposite signs. Then it might be possible that inconsistency in the signs of the group mean dynamic OLS estimator and its t value occurs. Table 1 presents the result.

Table 1. Results of case 3.

4. Conclusion

In this paper, we show that there may be an inconsistency in the signs of the estimated coefficient and its t value when we use the group mean dynamic OLS estimator developed by Pedroni (2001).

Acknowledgements

We are grateful to three anonymous referees for their helpful comments and suggestions.

Conflicts of Interest

The authors declare no conflicts of interest.

References

[1]	Stock, J.S. and Watson, M.W. (2011) Introduction to Econometrics. 3rd Edition, Addison-Wesley, Boston.
[2]	Wooldridge, J.M. (2013) Introductory Econometrics: A Modern Approach. 5th Edition, South-Western Pub, Mason.
[3]	Pedroni, P. (2001) Purchasing Power Parity Tests in Cointegrated Panels. Review of Economics and Statistics, 83, 727-731. http://dx.doi.org/10.1162/003465301753237803