The Promotion Rule under Imperfect Observability of the Employee’s Ability ()
1. Introduction
The Peter Principle claims that an employee is promoted to the rank at which the employee exhibits his incompetence. Lazear [1] attributes the observation to the statistical mean reversion. The employer promotes an employee if the employee’s performance exceeds a certain threshold. When the employee’s performance depends partly on luck, a lucky employee is more likely to be promoted. The promoted employee’s performance necessarily declines, on average, because the good luck does not persist after his promotion. Lazear [1] argues that the observed decline has nothing to do with misassignment, because the employer accounts for the mean reversion of the employee’s performance when setting the promotion threshold. Lazear [1] qualitatively characterizes the promotion threshold, and provides several numerical examples for this threshold, but does not provide the closed-form solution. This note provides the closed-form solution for the model under normality assumptions on ability and productivity-shock distributions to explicitly demonstrate the model’s rich implications.
2. Setup
An employer hires an employee whose performance in period 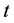 depends on ability
depends on ability  and a random shock
and a random shock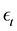 . There are two periods in the production, and there are two types of jobs. Output in period
. There are two periods in the production, and there are two types of jobs. Output in period 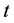 is
is
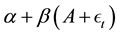 in the easy job and
in the easy job and 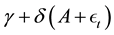 in the difficult job. Given
in the difficult job. Given 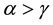 and
and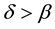 , an employer
, an employer
with high productivity has a comparative advantage in the difficult job. If the employer is risk neutral and can
observe the employee’s ability, the employer assigns the employee with  to the difficult
to the difficult
job. The challenge for the employer is assigning the employee to either a difficult job or an easy job in period two, after observing the noisy measure of ability 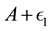 that can be backed out from the first-period output in either job.
that can be backed out from the first-period output in either job.
The employer knows the probability density function  and
and . The ability
. The ability  has a unimodal and
has a unimodal and
symmetric distribution. The productivity shock 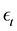 is independently distributed across periods and symmetri- cally distributed with a zero mean. With knowledge of the distributions, the employer updates the subjective ability distribution of a specific employee using the error-ridden index of his ability.
is independently distributed across periods and symmetri- cally distributed with a zero mean. With knowledge of the distributions, the employer updates the subjective ability distribution of a specific employee using the error-ridden index of his ability.
The employer promotes the employee if the first-period performance  exceeds a threshold
exceeds a threshold . The employer’s problem is to set the threshold
. The employer’s problem is to set the threshold ![]() to maximize the expected output:
to maximize the expected output:
![]() (1)
(1)
using the fact that ![]() is independent from
is independent from ![]() and
and ![]() and has a zero mean.
and has a zero mean.
The first-order condition of the output maximization problem is:
![]() (2)
(2)
Lazear [1] does not explicitly solve the problem. Instead, he rearranges the first-order condition so that
![]() (3)
(3)
by replacing ![]() or
or![]() . Assuming
. Assuming ![]() to be a unimodal and symmetric distribution and that less
to be a unimodal and symmetric distribution and that less
than one half of the employees should be promoted (![]() is above the median of ability distribution),
is above the median of ability distribution),
![]() . Then
. Then![]() , which implies
, which implies
![]() (4)
(4)
Since ![]() and
and![]() ,
, ![]() follows. This is how Lazear [1] shows that the
follows. This is how Lazear [1] shows that the
employer inflates the promotion threshold to account for the expected decline after a promotion. He also points to the deflated promotion threshold when more than one half of the employees should be promoted (![]() is below the median of the ability distribution).
is below the median of the ability distribution).
3. The Closed-Form Solution
We obtain the closed-form solution for the model, assuming ![]() and
and![]() . With
. With
these assumptions, we can rewrite the first-order condition such that
![]() (5)
(5)
The terms in the exponential function can be decomposed into terms that do not contain the random variable ![]() and a term containing it, as follows:
and a term containing it, as follows:
![]() (6)
(6)
where![]() .
.
Using this result, the first-order condition becomes:
![]() (7)
(7)
By defining![]() , the equation becomes
, the equation becomes
![]() (8)
(8)
Using the facts that the probability-density function of the normal distribution with mean ![]()
and variance ![]() integrates to 1 and has the expected value
integrates to 1 and has the expected value![]() , the first-order condition
, the first-order condition
becomes:
![]() (9)
(9)
Dividing the first-order condition by common factors renders:
![]() (10)
(10)
This leads to the solution:
![]() (11)
(11)
4. Implications
Lazear [1] provides numerical solutions on page 147 under the normality assumptions on![]() ,
, ![]() and the
and the
parameter values![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
,![]() . For the case of
. For the case of![]() , Lazear’s [1]
, Lazear’s [1]
![]() is close to our solution
is close to our solution![]() . For the case of
. For the case of![]() , Lazear’s [1]
, Lazear’s [1] ![]() is again close to
is again close to
our solution![]() . The examples make a point that the employer sets a higher threshold if the performance depends heavily on luck, because the employer expects a severe performance decline in the second period.
. The examples make a point that the employer sets a higher threshold if the performance depends heavily on luck, because the employer expects a severe performance decline in the second period.
The closed-form solution preserves the predictions in the original model. In a typical case in which fewer than
one half of employees are eligible for promotion, ![]() , in order to compensate for the expected decline, the employer sets a higher threshold for promotion than the case when the employer perfectly observes the employee’s ability. This threshold premium is larger when the employer knows that the first-period performance depends heavily on luck and depends lightly on ability so that
, in order to compensate for the expected decline, the employer sets a higher threshold for promotion than the case when the employer perfectly observes the employee’s ability. This threshold premium is larger when the employer knows that the first-period performance depends heavily on luck and depends lightly on ability so that ![]() is larger. The argument reverses when more than one half should be promoted,
is larger. The argument reverses when more than one half should be promoted,![]() . The employer thus discounts the threshold, expecting a future rise of the employee’s performance, particularly when the first-period output depends heavily on luck and depends lightly on ability.
. The employer thus discounts the threshold, expecting a future rise of the employee’s performance, particularly when the first-period output depends heavily on luck and depends lightly on ability.
Acknowledgments
This work was supported by JSPS KAKENHI Grant Numbers 23330079 and 11J02356. This support is greatly appreciated.