1. The Model
It is a common practice to use toroidal coils in measuring alternating currents at a distance. A wire carrying a sinusoidally oscillating current passes through a toroid (Figure 1), and the emf induced in the coil is measured [1] [2] . Such a usage of toroidal coils was first suggested by A. P. Chattock already in the 19th century [3] , and by W. Rogowski and W. Steinhaus some decades later [4] . Recently, scientists have been developing new materials for the magnetic core of toroidal coils [5] .
The use of toroids, or Rogowski Coils, has been justified by the fact that the position of the point at which the wire passes through the toroid does not affect the induced emf at all. Hence, even if the wire is almost in contact
![]()
Figure 1. The cylindrical magnetic field lines generated by a sinusoidally oscillating current induce a sinusoidally oscillating emf in a toroidal coil around the current-carrying conductor.
with the inner surface of the toroid, the voltage will be the same as if the wire went through the geometric centre of the toroid.
We wanted to check whether this claim is correct or not.
Consider an ideal toroid of inner radius 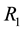 and of outer radius
and of outer radius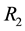 , and having altogether
, and having altogether 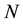 turns of wire. Let a straight wire carrying a sinusoidally oscillating current,
turns of wire. Let a straight wire carrying a sinusoidally oscillating current,
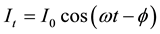
pass through the toroid perpendicularly at a point whose Cartesian coordinates are  relative to an origin at the geometric centre of the toroid, as shown in Figure 2.
relative to an origin at the geometric centre of the toroid, as shown in Figure 2.
We calculate the emf induced in the toroid by letting the angle 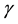 vary from 0 to
vary from 0 to 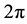 in steps of
in steps of  radians, and integrating the contributions of all the differential amounts of turns of wire,
radians, and integrating the contributions of all the differential amounts of turns of wire,  , associated with each step. Because of the chosen symmetry, the magnitude of
, associated with each step. Because of the chosen symmetry, the magnitude of  can be calculated simply as:
can be calculated simply as:

For each value of the angle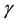 , we integrate the contributions of all the infinitesimal area elements
, we integrate the contributions of all the infinitesimal area elements  of the toroid’s cross-sectional surface. This is done by letting the variable
of the toroid’s cross-sectional surface. This is done by letting the variable  grow from 0 to
grow from 0 to , and integrating the emfs associated with each such area element. For the magnitude of
, and integrating the emfs associated with each such area element. For the magnitude of![]() , see Figure 3.
, see Figure 3.
According to Figure 3, the size of a differential area element is given by:
![]()
These two results yield that the differential amount of emf induced in the toroid when the angle ![]() increases by
increases by ![]() radians can be calculated as:
radians can be calculated as:
![]()
This yields:
![]()
![]()
Figure 2. The current-carrying straight conductor passes perpendicularly through the toroid at the Cartesian coordinates (x,y) relative to the geometric centre of the coil.
![]()
Figure 3. The cross-sectional area of the toroid is divided into differentially small elements, dA.
The Law of Cosines, when applied to our geometry, yields an expression for the ratio of ![]() to
to![]() :
:
![]()
In the expression above, the value of the term ![]() can be expressed in terms of the angle
can be expressed in terms of the angle![]() :
:
![]()
Further,
![]()
If one combines all the results together, the emf induced in the toroid can be calculated as follows:
![]()
2. Results of the Calculations
We used the mathematical software Mathcad, and solved the induced emf for a toroid and a current with the following specifications:
![]()
We did the calculus with a mesh of about 2000 node points inside the toroid, and solved the magnitude of for each point separately. The calculation revealed that the induced emf is completely independent of the position of the wire; the induced voltage was found to be 39.6 mV for all the points, see Figure 4.
![]()
Figure 4. Our numerical analysis reveals exactly the same value of the induced emf for any point inside the toroidal coil.
Our analysis thus verifies the claim that the emf induced in a toroid is independent of the position of a wire passing through the toroid―at least when the wire is perpendicular to the plane of the toroid.
Note that our analysis was done simply by assuming that the current-carrying wire is extremely thin and straight, and that the wire passes through the toroid perpendicularly. In a more general analysis, one would need to consider the cross-sectional shape and dimensions of the wire, as well as the wire’s orientation relative to the plane of the toroid.
3. Conclusion
Our analysis gives confirmation to the generally stated claim that the magnitude of the measured sinusoidally oscillating current is independent of the point at which the current-carrying wire passes through the toroid. Besides this result, we feel that this paper yields a valuable model for solving cases with similar geometry.