1. Introduction
The symmetric ring of quotients  of a semiprime ring
of a semiprime ring  is probably the most comfortable ring of quotients of
is probably the most comfortable ring of quotients of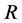 . This notion was first introduced by W.S. Martindale [1] for prime rings and extended to the semiprime case by Amitsur [2] . Recall that a ring
. This notion was first introduced by W.S. Martindale [1] for prime rings and extended to the semiprime case by Amitsur [2] . Recall that a ring  is said to be semiprime (resp. prime) if
is said to be semiprime (resp. prime) if 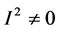 for every nonzero ideal
for every nonzero ideal  of
of 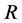 (resp. if
(resp. if 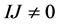 for all nonzero ideals
for all nonzero ideals 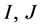 of
of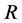 ). The center
). The center 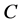 of
of 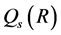 is called the extended centroid of
is called the extended centroid of , and the
, and the ![]() -subring
-subring ![]() of
of ![]() generated by
generated by ![]() is called the central closure of
is called the central closure of![]() . A semiprime
. A semiprime ![]() is said to be centrally closed whenever
is said to be centrally closed whenever![]() . For every
. For every![]() , we will denote
, we will denote ![]() and
and ![]() the left and right multiplication operators, respectively, by
the left and right multiplication operators, respectively, by ![]() on
on![]() . The multiplication ring of
. The multiplication ring of![]() ,
, ![]() , is defined as the subring of
, is defined as the subring of ![]() generated by the identity operator
generated by the identity operator ![]() and the set
and the set![]() . The goal of this paper is to give a semiprime extension of the following well-known result (see for instance [3] , Theorem A.9):
. The goal of this paper is to give a semiprime extension of the following well-known result (see for instance [3] , Theorem A.9):
“If the multiplication ring of a centrally closed prime ring ![]() has a finite rank operator over
has a finite rank operator over ![]() then
then ![]() contains an idempotent
contains an idempotent ![]() such that
such that ![]() is a division algebra finitely generated over
is a division algebra finitely generated over![]() ”.
”.
It is also well know that the extended centroid of a prime ring is a field, however, for a semiprime ring, we can only assert that said extended centroid is a von Neumann regular ring. This is the cause of the difficulty of extending this result. The starting point of this path relies on the fact that each subset ![]() of
of ![]() has an associated idempotent
has an associated idempotent ![]() of the extended centroid
of the extended centroid ![]() (see [4] , Theorem 2.3.9) and on a consequence (see [4] , Theorem 2.3.3 and Proposition 1.1 below) of the Weak Density Theorem ([4] , Theorem 1.1.5).
(see [4] , Theorem 2.3.9) and on a consequence (see [4] , Theorem 2.3.3 and Proposition 1.1 below) of the Weak Density Theorem ([4] , Theorem 1.1.5).
2. Tools
We shall assume throughout this paper that ![]() is a centrally closed semiprime ring. First of all, we recall that if
is a centrally closed semiprime ring. First of all, we recall that if ![]() is the set of all idempotents in
is the set of all idempotents in ![]() has a partial order given by
has a partial order given by ![]() iff
iff![]() . Moreover,
. Moreover, ![]() is a Boolean algebra for the operations
is a Boolean algebra for the operations
![]()
In fact, [5] , Theorem 1.8 remains valid in case that ![]() is a ring, and so this Boolean algebra is complete, that is, every subset of
is a ring, and so this Boolean algebra is complete, that is, every subset of ![]() admits supremum and infimum. We will use the properties of the idempotent associated to a subset referred to in ([4] , Theorem 2.3.9 (i) and (ii)) without notice.
admits supremum and infimum. We will use the properties of the idempotent associated to a subset referred to in ([4] , Theorem 2.3.9 (i) and (ii)) without notice.
Given a ![]() -submodule
-submodule ![]() of
of![]() , we will say that
, we will say that ![]() is
is ![]() -finitely generated if there exist
-finitely generated if there exist ![]()
![]() such that
such that![]() .
.
Next, we establish our main tool.
Proposition 1.1 Let ![]() be a
be a ![]() -finitely generated
-finitely generated ![]() -submodule of
-submodule of![]() , and let
, and let![]() . Then there exists
. Then there exists ![]() such that: a)
such that: a)![]() , b)
, b) ![]() and c)
and c)![]() .
.
Proof. We denote![]() . If
. If![]() , then
, then![]() . Suppose that
. Suppose that![]() . If
. If![]() , then we take
, then we take![]() . In other case, take
. In other case, take![]() , for some
, for some![]() . By ([4] Theorem 2.3.9), there exists
. By ([4] Theorem 2.3.9), there exists ![]() such that
such that ![]() and
and![]() . In particular,
. In particular, ![]() , and
, and![]() . Thus, the family
. Thus, the family ![]() of all nonzero idempotents satisfying
of all nonzero idempotents satisfying ![]() and
and ![]() is not empty. Let
is not empty. Let![]() . Note that
. Note that ![]() because of completeness of
because of completeness of![]() , and, of course,
, and, of course,![]() . If
. If![]() , then, by ([4] , Theorem 2.3.3), there exists
, then, by ([4] , Theorem 2.3.3), there exists ![]()
such that ![]() and
and![]() . But, since
. But, since![]() , we have
, we have ![]() and so
and so ![]()
for all![]() . Hence
. Hence![]() , that is,
, that is, ![]() , which is a contradiction with
, which is a contradiction with![]() . Therefore
. Therefore
![]() belongs to
belongs to![]() . Take
. Take![]() . Let us see that
. Let us see that![]() . Indeed, for every
. Indeed, for every![]() , we can write:
, we can write:
![]() (1)
(1)
Moreover, if there exists ![]() and
and ![]() such that
such that
![]()
then![]() . Take
. Take ![]() such that
such that ![]() and
and ![]() is an idempotent in
is an idempotent in![]() . It is clear that
. It is clear that![]() , and so
, and so ![]() by maximality. Thus,
by maximality. Thus, ![]() and
and![]() . Finally, note that:
. Finally, note that:
![]()
Thus, the sum is direct. Note that ![]() verifies properties a), b) and c).
verifies properties a), b) and c). ![]()
As a consequence, we have the following:
Corollary 1.2 Let ![]() be a nonzero C-submodule of
be a nonzero C-submodule of ![]() and
and ![]() such that
such that![]() . Then there exists
. Then there exists ![]() such that
such that![]() .
.
Proof. If ![]() take
take![]() . In other case,
. In other case,![]() . By Proposition 1.1, there is
. By Proposition 1.1, there is ![]() such that
such that ![]() and
and![]() . Thus,
. Thus, ![]() , and so,
, and so,![]() .
. ![]()
Note that if ![]() then it may be that
then it may be that ![]() but
but![]() . This forces us to make a convenient definition of set
. This forces us to make a convenient definition of set ![]() -linearly independent. We will say that
-linearly independent. We will say that ![]() nonzero elements
nonzero elements ![]() of R are C-linearly independent (or that the set
of R are C-linearly independent (or that the set ![]() is
is ![]() -linearly independent) if, for all
-linearly independent) if, for all![]() ,
, ![]() implies
implies ![]() for all
for all![]() , or equivalently, if the
, or equivalently, if the ![]() -linear envelope
-linear envelope ![]() of the subset
of the subset
S satisfies:![]() . Note that for every
. Note that for every ![]() and
and![]() , if
, if ![]() and
and ![]() are nonzero, then
are nonzero, then
the sets ![]() and
and ![]() are C-linearly independent and both generate the C-module
are C-linearly independent and both generate the C-module![]() . In general, any C-finitely generated C-module
. In general, any C-finitely generated C-module ![]() can be obtained as the C-linear envelope of C-linearly independent sets with different cardinal. In this sense, in ([4] Theorem 2.3.9. (iv)) is asserted that one can select a C-linearly independent set with a minimal number of generators under certain conditions. In any case, certain properties of the vector spaces remain true for the C-submodules: the next results, probably well-known, are obtained as a consequence of Proposition 1.1.
can be obtained as the C-linear envelope of C-linearly independent sets with different cardinal. In this sense, in ([4] Theorem 2.3.9. (iv)) is asserted that one can select a C-linearly independent set with a minimal number of generators under certain conditions. In any case, certain properties of the vector spaces remain true for the C-submodules: the next results, probably well-known, are obtained as a consequence of Proposition 1.1.
Corollary 1.3 Let ![]() be a subset of
be a subset of ![]() and
and ![]() two C-finitely generated C-submodules of
two C-finitely generated C-submodules of ![]() such that
such that![]() . Then there are
. Then there are ![]() such that the subset of
such that the subset of ![]()
![]()
is ![]() -linearly independent, and
-linearly independent, and![]() .
.
Proof. If![]() , we take
, we take![]() . In other case, by Proposition 1.1, there exists
. In other case, by Proposition 1.1, there exists ![]() such that
such that
![]() . Now, if
. Now, if ![]() then take
then take![]() , and if
, and if ![]() then,
then,
by Proposition 1.1, there exists ![]() such that
such that![]() . To conclude, it is enough to repeat this procedure
. To conclude, it is enough to repeat this procedure ![]() times.
times. ![]()
Corollary 1.4 If ![]() is a C-finitely generated C-submodule then there exist
is a C-finitely generated C-submodule then there exist ![]() and
and ![]()
such that![]() .
.
Proof. Let ![]() such that
such that![]() . By Corollary 1.3 we can assume that the set
. By Corollary 1.3 we can assume that the set ![]() is C-linearly independent.
is C-linearly independent.
It is clear that![]() . By Proposition 1.1, there exist
. By Proposition 1.1, there exist ![]() such that, for every
such that, for every
![]() ,
, ![]() and
and
![]()
Hence,
![]()
Therefore, ![]() Analogously, since
Analogously, since ![]() with
with
![]() and
and![]() , we have
, we have
![]()
and so,![]() .
.
By repeating this procedure, there are ![]() such that
such that
![]()
and hence,![]() . Therefore, since,
. Therefore, since, ![]() with
with ![]() and
and![]() , and, for each
, and, for each![]() ,
, ![]() with
with ![]() and
and![]() , we deduce that
, we deduce that
![]()
and so,![]() . Again, by Corollary 1.3, we obtain
. Again, by Corollary 1.3, we obtain ![]()
![]() -linear independent
-linear independent
elements of ![]() such that
such that![]() .
. ![]()
Let ![]() be a right ideal of R. We say that
be a right ideal of R. We say that ![]() is a
is a ![]() -minimal right ideal if for every nonzero right ideal
-minimal right ideal if for every nonzero right ideal ![]() of
of ![]() contained in
contained in![]() , there exists some
, there exists some ![]() such that
such that![]() . Note that if
. Note that if ![]() is prime then, since
is prime then, since ![]() is a field,
is a field, ![]() , and so, the concepts of
, and so, the concepts of ![]() -minimal right ideal and minimal right ideal agree.
-minimal right ideal and minimal right ideal agree.
Recall that for a subset ![]() of
of ![]() the left annihilator
the left annihilator ![]() will be denoted by
will be denoted by![]() . The right annihilator
. The right annihilator ![]() is similarly defined.
is similarly defined.
Proposition 1.5 Let ![]() be a
be a ![]() -minimal right ideal of
-minimal right ideal of![]() . Then there exists an idempotent
. Then there exists an idempotent ![]() and
and ![]() such that
such that![]() . As a consequence
. As a consequence ![]() is a
is a ![]() -minimal ideal of
-minimal ideal of![]() .
.
Proof. Since ![]() and R is semiprime,
and R is semiprime, ![]() , and hence there exists
, and hence there exists ![]() such that
such that![]() . Note that this implies the existence of some
. Note that this implies the existence of some ![]() such that
such that![]() . Since
. Since![]() , there exists
, there exists ![]() such that
such that![]() . Note that
. Note that![]() , and then:
, and then:![]() , that is,
, that is,![]() . Since
. Since ![]() is a right ideal of
is a right ideal of![]() , if
, if![]() , by minimality there exists
, by minimality there exists ![]() such that
such that![]() . But, since
. But, since![]() , we have
, we have![]() , a contradiction. Hence,
, a contradiction. Hence, ![]() (
(![]() because
because![]() ). Then
). Then![]() . Since
. Since ![]() is
is ![]() -minimal, there exists some
-minimal, there exists some ![]() such that
such that![]() .
. ![]()
We finalized this section with a desirable result, which is similar to the well-known result for minimal right ideals (see for instance [4] , Proposition 4.3.3).
Proposition 1.6 Let ![]() be an idempotent of
be an idempotent of![]() . The following assertion are equivalent:
. The following assertion are equivalent:
1) ![]() is
is ![]() -minimal right ideal of
-minimal right ideal of![]() .
.
2) For every ![]() there exist
there exist ![]() and
and ![]() such that
such that![]() .
.
Proof. (1) ![]() (2). Since
(2). Since ![]() is an idempotent, it is clear that
is an idempotent, it is clear that ![]() is the unit of
is the unit of![]() . Take
. Take![]() . It is clear that
. It is clear that![]() , and so, since xR is right ideal of R, there exists
, and so, since xR is right ideal of R, there exists ![]() such that
such that![]() . In particular, there is
. In particular, there is ![]() such that
such that![]() . Therefore
. Therefore![]() .
.
(2) ![]() (1)
(1)
Let I be a nonzero right ideal of R such that![]() . Let us see that there exists
. Let us see that there exists ![]() such that
such that![]() . Indeed, if we take
. Indeed, if we take![]() , by semiprimeness of R, there exists
, by semiprimeness of R, there exists ![]() such that
such that![]() . Note that
. Note that ![]() for every
for every![]() . Consequently,
. Consequently, ![]() is a nonzero element of
is a nonzero element of![]() , and hence there are
, and hence there are ![]() and
and ![]() such that
such that![]() . Therefore
. Therefore![]() , and so,
, and so,![]() . Thus
. Thus![]() .
. ![]()
A nonzero idempotent q of R is said to be ![]() -minimal when the above assertions are fulfilled.
-minimal when the above assertions are fulfilled.
3. Theorem
In this section we will prove a semiprime extension of [3] , Theorem A.9. Concretely,
Theorem 2.1 Let R be a centrally closed semiprime ring. Then ![]() has a C-finite rank operator if, and only if,
has a C-finite rank operator if, and only if, ![]() contains a
contains a ![]() -minimal idempotent
-minimal idempotent ![]() such that
such that ![]() is
is ![]() -finitely generated.
-finitely generated.
We begin this proof with an another consequence of Proposition 1.1,which is an improvement of Corollary 1.2 to case![]() . Given a nonzero C-module M C-finitely generated, we will say that
. Given a nonzero C-module M C-finitely generated, we will say that ![]() when- ever
when- ever
![]()
Lemma 2.2 Let ![]() be a nonzero
be a nonzero ![]() -submodule of
-submodule of ![]() and suppose that, for every
and suppose that, for every ![]() such that
such that![]() ,
,![]() . If
. If ![]() for some
for some ![]() then there exists
then there exists ![]() such that
such that
![]() .
.
Proof. It is clear that![]() . By Proposition 1.1, there exist
. By Proposition 1.1, there exist ![]() such that
such that
![]()
and![]() , in fact,
, in fact,![]() . Moreover,
. Moreover,
![]()
Hence,
![]()
If![]() , then
, then
![]()
that is, ![]() , and this is a contradiction. Thus,
, and this is a contradiction. Thus, ![]() and
and
![]()
Note that if ![]() then
then![]() , which is a contradiction. By Proposition 1.1, there exist
, which is a contradiction. By Proposition 1.1, there exist ![]() such that
such that
![]()
and![]() . Therefore, since
. Therefore, since ![]() with
with ![]() and
and
![]() , it is clear that
, it is clear that
![]()
Hence,
![]()
If![]() , then
, then ![]() is contained in
is contained in ![]() summands, which is a contradiction. Hence, since
summands, which is a contradiction. Hence, since![]() , we have
, we have
![]()
Note that if![]() , then
, then![]() , which is a contradiction. By repeating this procedure, we find
, which is a contradiction. By repeating this procedure, we find ![]() such that,
such that, ![]() ,
, ![]() , and
, and
![]() .
.
Therefore, denoting![]() , again by Proposition 1.1, there exists
, again by Proposition 1.1, there exists ![]() such that
such that ![]() and,
and,
![]()
and hence,
![]() ,
,
or even
![]() .
.
Of course, ![]() because
because![]() , and so,
, and so,![]() . Thus, take
. Thus, take![]() .
. ![]()
The next result is an immediate consequence of the Weak Density (see [4] , Theorem 2.3.3). We will denote by ![]() the operator
the operator ![]() for all
for all![]() .
.
Lemma 2.3 Let![]() . Assume that
. Assume that ![]() or
or ![]() are C-linearly inde- pendent sets such that
are C-linearly inde- pendent sets such that![]() . Then there are
. Then there are ![]() and
and ![]() such that
such that
![]() .
.
Proof. Assume that ![]() are C-linearly independent. If
are C-linearly independent. If ![]() for all
for all ![]() then,
then,
since![]() , we deduce that
, we deduce that![]() , is a contradiction. For simplicity, we
, is a contradiction. For simplicity, we
can suppose that![]() . By [4] (Theorem 2.3.3), there exists
. By [4] (Theorem 2.3.3), there exists ![]() with
with![]() , such that
, such that
![]() and
and ![]() for all
for all![]() . Put
. Put![]() , and note that, for every
, and note that, for every![]() , we have:
, we have:
![]() .
.
As a consequence:![]() . Moreover, by [4] (Corollary 2.3.10),
. Moreover, by [4] (Corollary 2.3.10),![]() .
. ![]()
First step in the proof of Theorem
Proposition 2.4 If ![]() has a
has a ![]() -finite rank operator then there are
-finite rank operator then there are ![]() such that
such that ![]() is
is ![]() - finitely generated.
- finitely generated.
Proof. First of all, given a nonzero operator ![]() with C-finite rank we can find an operator of the
with C-finite rank we can find an operator of the
form![]() , which has also C-finite rank. In fact, the most general form of G is:
, which has also C-finite rank. In fact, the most general form of G is: ![]()
for some![]() , and
, and![]() . We can take an element
. We can take an element ![]() such that
such that![]() , because in other case we would have
, because in other case we would have![]() , a contradiction. Analogously, there exists some
, a contradiction. Analogously, there exists some ![]() such that
such that![]() . Now,
. Now, ![]() is a nonzero operator with the desired form. Moreover, if
is a nonzero operator with the desired form. Moreover, if ![]() is
is ![]() -finitely generated then
-finitely generated then ![]() is also
is also ![]() -finitely generated. Secondly, taking in mind Corollary 1.3, we can assume without loss of generality that the set
-finitely generated. Secondly, taking in mind Corollary 1.3, we can assume without loss of generality that the set ![]() is C-linearly independent. Finally, by Lemma 2.3 there are
is C-linearly independent. Finally, by Lemma 2.3 there are ![]()
and ![]() such that
such that![]() , and so,
, and so, ![]() is also
is also ![]() -finitely generated.
-finitely generated. ![]()
Second step in the proof of Theorem is a consequence of Lemma 2.2, and its proof can be obtained from a careful reading of the proof of [4] (Lemma 6.1.4).
Proposition 2.5 Let ![]() such that
such that ![]() is
is ![]() -finitely generated. Then there exist a
-finitely generated. Then there exist a ![]() -minimal idempotent
-minimal idempotent ![]() such that
such that ![]() is
is ![]() -finitely generated.
-finitely generated.
Proof. Without loss of generality we can assume that![]() . Since, in other case, if we take
. Since, in other case, if we take ![]()
then![]() . Suppose further that
. Suppose further that![]() , for
, for![]() . By Corollary 1.3, we can assume that
. By Corollary 1.3, we can assume that
the sum is direct. Consider the set
![]() .
.
It is clear that![]() . Take m as the minimum of H and
. Take m as the minimum of H and ![]() such that
such that ![]() for some
for some![]() . Let
. Let![]() . If
. If![]() , then
, then![]() , which is a contradiction because of semiprimeness of
, which is a contradiction because of semiprimeness of![]() . Thus
. Thus![]() . Let
. Let ![]() be a right ideal of
be a right ideal of ![]() and
and![]() , where
, where![]() . Setting
. Setting
![]() we note that
we note that![]() . Note that if
. Note that if ![]() then
then![]() , a contradiction with the semi-
, a contradiction with the semi-
Primeness. Take![]() , it is clear that
, it is clear that![]() . Note that
. Note that ![]() satisfies the hypothesis either of the Corollary 1.2 (if
satisfies the hypothesis either of the Corollary 1.2 (if![]() ) or of the Proposition 2.2 (if
) or of the Proposition 2.2 (if![]() ), in any case, there is
), in any case, there is ![]() such that
such that![]() . In particular,
. In particular,![]() . Therefore,
. Therefore, ![]() , that is,
, that is, ![]() is a
is a ![]() -minimal right ideal of
-minimal right ideal of![]() . By Proposition 1.5, there exist
. By Proposition 1.5, there exist![]() , and
, and ![]() such that
such that
![]() . Clearly
. Clearly![]() , and so
, and so ![]() where
where![]() . Hence
. Hence ![]() and
and
so ![]() is
is ![]() -finitely generated.
-finitely generated. ![]()
Finally, the converse is obvious.
Funding
Supported by the Junta de Andaluca Grant FQM290.