Bianchi Type-V Cosmological Model with Linear Equation of State in Brans-Dicke Theory of Gravitation ()
1. Introduction
Einstein’s [1] general theory of relativity has provided a sophisticated theory of gravitation which has been very successful in describing gravitational phenomenon and also served as a basis for models of the universe. The homogeneous isotropic expanding model based on general relativity appears to provide a grand approximation to the observed large-scale properties of the universe. However since Einstein first published his theory of gravitation there have been many criticisms of general relativity because of lack of certain “desirable” features in the theory. For example, Einstein himself pointed out that general relativity does not account satisfactorily for inertial properties of matter i.e. Mach’s principle is not substantiated by general relativity. So, in recent years, several theories of gravitation have been proposed as alternatives for Einstein’s theory. The most important among them are scalar-tensor theories of gravitation formulated by Jordan [2] , Brans and Dicks [3] , Nordtvedt [4] , Ross [5] and Schmidt et al. [6] .
Brans and Dicke [3] introduced a scalar-tensor theory of gravitation involving a scalar function in addition to the familiar general relativistic metric tensor. In this theory the scalar field has the dimensions of inverse of the gravitational constant and its role is confined to its effect on gravitational field equations. Several aspects of Brans-Dicke cosmology have been extensively investigated by many authors. The work of Singh and Rai [7] give a detailed survey of Brans-Dicke cosmological models discussed by several authors. Nariai [8] , Reddy and Rao [9] , Banerjee and Santos [10] , Singh et al. [11] , Ram [12] , Ram and Singh [13] , Berman et al. [14] , Reddy et al. [15] are some of the authors who have investigated several aspects of this theory. Reddy and Venkateswara Rao [16] and Reddy et al. [17] discussed Bianchi type I cosmological models with negative constant declaration parameter in scalar tensor theories formulated by Brans-Dicke theory and Saez-Ballester theory [18] .
There has been considerable interest in the study of spatially homogeneous, anisotropic cosmological models. Bianchi type  space-times, being a straight forward generalization of FRW universe with negative curvature are interesting to study because they contain isotropic special cases and allow arbitrarily small anisotropy levels at any instant of cosmic time. Fransworth [19] , Collins [20] , Maartens and Nel [21] , Wainwright et al. [22] have studied Bianchi type
space-times, being a straight forward generalization of FRW universe with negative curvature are interesting to study because they contain isotropic special cases and allow arbitrarily small anisotropy levels at any instant of cosmic time. Fransworth [19] , Collins [20] , Maartens and Nel [21] , Wainwright et al. [22] have studied Bianchi type 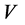 cosmological models. Nayak and Sahoo [23] have investigated Bianchi type
cosmological models. Nayak and Sahoo [23] have investigated Bianchi type  models for matter distribution admitting anisotropic pressure and heat flow. Several authors studied Bianchi type
models for matter distribution admitting anisotropic pressure and heat flow. Several authors studied Bianchi type  in different context [24] -[37] .
in different context [24] -[37] .
Motivated by above discussion, in this paper we have obtained exact solutions of the Einstein field equations of Bianchi type 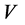 Cosmological model with linear equation of state in the scalar tensor theory of gravitation proposed by Brans and Dicke.
Cosmological model with linear equation of state in the scalar tensor theory of gravitation proposed by Brans and Dicke.
2. The Metric and Field Equations
We consider Bianchi type- space time described by the line element
space time described by the line element
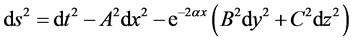 (1)
(1)
where 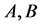 and
and 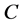 are function of cosmic time
are function of cosmic time .
.
Brans-Dicke field equations for combined scalar and tensor field are
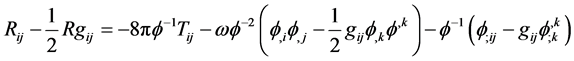 (2)
(2)
and
 (3)
(3)
where 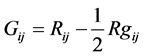 is an Einstein tensor,
is an Einstein tensor, 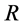 is the scalar curvature,
is the scalar curvature, 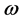 is a dimension less constant and
is a dimension less constant and
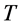 is the stress energy tensor of the matter, comma and semicolon denote partial and co-variant differentiation respectively.
is the stress energy tensor of the matter, comma and semicolon denote partial and co-variant differentiation respectively.
Also, we have energy-conservation equation
 (4)
(4)
are consequences of the field Equations (2) and (3).
The energy momentum tensor
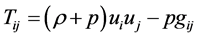 (5)
(5)
where 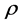 is the energy density of the perfect fluid and
is the energy density of the perfect fluid and  is its pressure. Here the four velocity vector
is its pressure. Here the four velocity vector  and
and  satisfy the standard relations
satisfy the standard relations
 (6)
(6)
Here we use line are quation of state given by Thirukkanesh and Maharaj [38] for perfect fluid as
 (7)
(7)
where 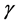 and
and 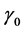 constants.
constants.
In the moving coordinate system, we have from (5) and (6)
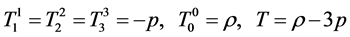 (8)
(8)
Now the Brans-Dicke field Equations (2) and (3) for the metric (1) with the help of (5) to (7) can be written as
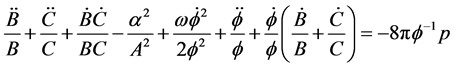 (9)
(9)
 (10)
(10)
 (11)
(11)
 (12)
(12)
 (13)
(13)
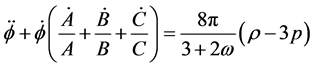 (14)
(14)
where dot over the field variables denote differentiation with respect to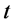 .
.
Spatial volume and the scale factor for the metric (1) are defined respectively, by
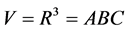 (15)
(15)
 (16)
(16)
The physical quantities of observational interest in cosmology are the expansion scalar , shear scalar
, shear scalar  and the mean anisotropic parameter
and the mean anisotropic parameter 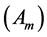 defined as
defined as
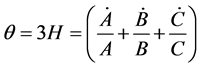 (17)
(17)
 (18)
(18)
 (19)
(19)
3. Solutions and the Model
From Equations (10) and (11), we get
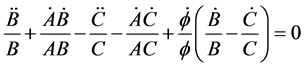 (20)
(20)
Integrating Equation (13), we obtain
 (21)
(21)
where 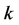 is a constant of integration. The constant
is a constant of integration. The constant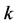 , without loss of generality, can be chosen as unity so that we have, from Equation (21),
, without loss of generality, can be chosen as unity so that we have, from Equation (21),
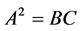 (22)
(22)
In order to get a deterministic solution, we take the following plausible physical condition:
i) The shear scalar  is proportional to scalar expansion
is proportional to scalar expansion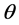 , which leads to the linear relationship between the metric potentials
, which leads to the linear relationship between the metric potentials 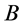 and
and , that is,
, that is,
 (23)
(23)
where 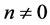 is a constant.
is a constant.
ii) With the help of special law of variation of Hubble’s parameter proposed by Berman [39] yields constant deceleration parameter models of the universe,
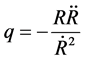 (24)
(24)
this admits the solution
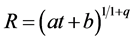 (25)
(25)
where 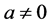 and
and 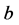 are constants of integration.
are constants of integration.
This equation implies that the condition for accelerated expansion of universe is .
.
Now from Equations (16), (22), (23) and (25), we obtain
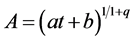 (26)
(26)
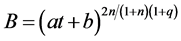 (27)
(27)
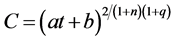 (28)
(28)
Now considering a well-accepted power law relation [40] -[42] between scale factor  and scalar field
and scalar field 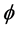 of the form
of the form
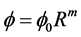 (29)
(29)
where  is the constant of proportionality.
is the constant of proportionality.
Using Equation (23) in Equation (20), we obtain
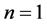
Using this value of 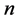 in Equations (26)-(28) and by a suitable choice of coordinates and constants (i.e. take
in Equations (26)-(28) and by a suitable choice of coordinates and constants (i.e. take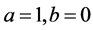 ), the metric (1) can be written as
), the metric (1) can be written as
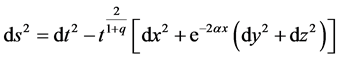 (30)
(30)
Further we find the volume , mean Hubble parameter
, mean Hubble parameter , expansion scalar
, expansion scalar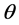 , shear scalar
, shear scalar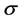 , and mean anisotropic parameter
, and mean anisotropic parameter 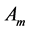 as
as
 (31)
(31)
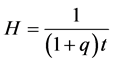 (32)
(32)
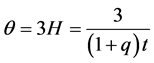 (33)
(33)
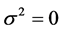 (34)
(34)
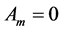 (35)
(35)
Using Equations (26)-(29), we get the energy density as
 (36)
(36)
With the help of Equations (7) and (36), we get the pressure as
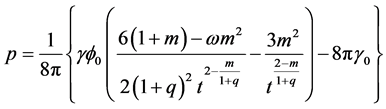 (37)
(37)
We observe that the scale factor 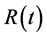 with respect to cosmic time grows rapidly as shown in Figure 1 while Figure 2 indicates that scalar field
with respect to cosmic time grows rapidly as shown in Figure 1 while Figure 2 indicates that scalar field  is increasing with evolution of universe. It can be easily seen from Figure 3 and Figure 4 that the volume goes on increasing as time increases and the energy density is decreasing function of time.
is increasing with evolution of universe. It can be easily seen from Figure 3 and Figure 4 that the volume goes on increasing as time increases and the energy density is decreasing function of time.
4. Conclusion
In this paper, we have obtained Bianchi type 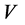 space-time with linear equation of state for perfect fluid in the Brans-Dicke theory. While solving Brans-Dicke’s field equations for Bianchi type
space-time with linear equation of state for perfect fluid in the Brans-Dicke theory. While solving Brans-Dicke’s field equations for Bianchi type  cosmological model, we have used a special law of variation of Hubble parameter proposed by Berman. It can also be observed that
cosmological model, we have used a special law of variation of Hubble parameter proposed by Berman. It can also be observed that 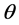 and
and  decrease with time and approach zero as
decrease with time and approach zero as 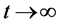 while they all become infinitely large as
while they all become infinitely large as 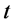 approaches zero. Also the model is expanding with time since
approaches zero. Also the model is expanding with time since  and it has initial singularity i.e. at
and it has initial singularity i.e. at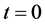 . It is also interesting to note that the average anisotropic parameter vanishes so that the model does not remain anisotropic throughout the evolution of the universe and the model becomes shear free. In this model the universe starts with finite volume and volume goes on increasing as time
. It is also interesting to note that the average anisotropic parameter vanishes so that the model does not remain anisotropic throughout the evolution of the universe and the model becomes shear free. In this model the universe starts with finite volume and volume goes on increasing as time  increases. Depending on the values of
increases. Depending on the values of  and other constant, the pressure is negative which works as dark energy. Also the generalized equation of state for
and other constant, the pressure is negative which works as dark energy. Also the generalized equation of state for  and
and 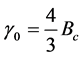 reduces to equation of state for strange quark matter.
reduces to equation of state for strange quark matter.
NOTES
*Corresponding author.