Darboux Transformation in Quantum Black-Scholes Hamiltonian and Supersymmetry ()
1. Introduction
As we know, there are several methods to study for the integrability model. One of the method, we focus here, is Darboux transformation. It is well known that the Darboux transformation [1] is one of the major tools for the analysis of physical systems and for finding new solvable systems. Using a linear differential operator, Darboux construct solutions of one ordinary differential equation in terms of another ordinary differential equation. It has been shown that the transformation method is useful in finding soliton solutions of the integrable systems [2-4] and constructing supersymmetric quantum mechanical systems [5-7]. Also, more general solvable cases were obtained by means of factorization methods [8] and via lie algebraic approaches [9-13]. Darboux transformation is known as one of the most powerful methods for finding solvable Schrodinger equations with constant mass, in the context of which it is also called supersymmetric factorization method [14]. On the other hand, during the past few years there has been great interest in studying problems of fiance using various tools of physics [15]. In that case also quantum mechanics has been used to analyze option pricing, stock market returns [16,17] and the Black-Scholes (BS) equation [18-21]. The BS equation plays an important role in option pricing. The solution of such equation may be found by mapping it into a Schrodinger-like equation. So, we take advantage from Darboux transformation to this equation and obtain the generalized form of BS equation.
The Darboux transformation has been extensively used in quantum mechanics in the search of isospectral potential for exactly Schrodinger equations of constant mass and position-dependent mass [22-27]. So, we take advantage from such transformation and obtain the effecttive potential, modified wave function and shape invariance condition and generators of supersymmetry algebra. For the BS Hamiltonian help us to transform of the corresponding potential.
This paper is organized as follows. We first introduce Quantum BS Hamiltonian and apply the Darboux transformation to such equation. In that case we show that the corresponding Hamiltonian changes to new form of potential. Finally, we study the supersymmetry version and shape invariance condition for transformed BS Hamiltonian.
2. Darboux Transformation and BS Hamiltonian
As we know the BS equation for option pricing with constant volatility is given by,
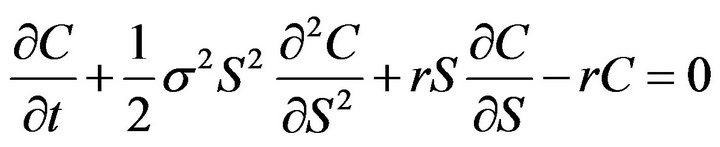 (1)
(1)
where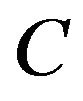 ,
, 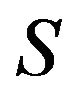 ,
,  and
and 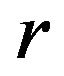 denote the price of the option, the stock price, the volatility of the stock price and the risk-free spot interest rate respectively. Now we consider the following generalized BS equation in (1 + 1)-dimension by using the Darboux transformation operator technique [26,27],
denote the price of the option, the stock price, the volatility of the stock price and the risk-free spot interest rate respectively. Now we consider the following generalized BS equation in (1 + 1)-dimension by using the Darboux transformation operator technique [26,27],
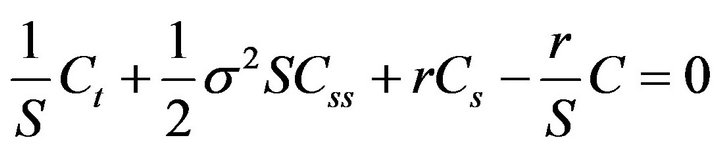 (2)
(2)
Now, we take ,
,  ,
, 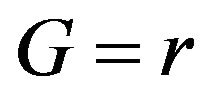 and the potential
and the potential . Here we can rewrite the above equation as,
. Here we can rewrite the above equation as,
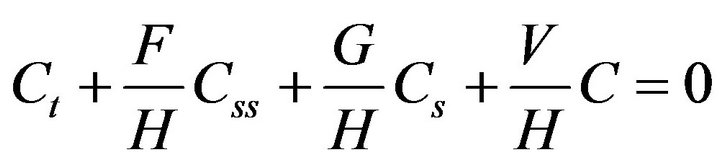 (3)
(3)
and
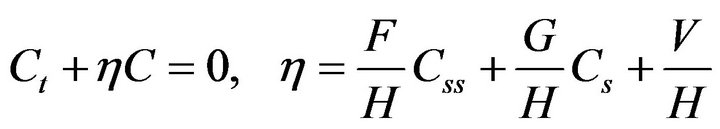 (4)
(4)
In order to have same Equation as (2) with different of potential,
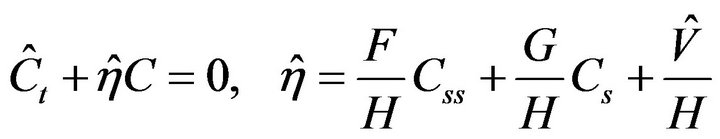 (5)
(5)
Here, we have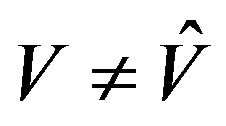 , this lead us to imply
, this lead us to imply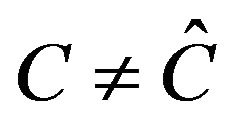 . In order to obtain the modified potential
. In order to obtain the modified potential 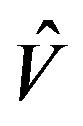 and corresponding wave function for Equation (5), we introduce operator
and corresponding wave function for Equation (5), we introduce operator  which are called Darboux transformation. The general form of such translation Durboux transformation will be as
which are called Darboux transformation. The general form of such translation Durboux transformation will be as
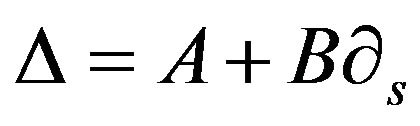 (6)
(6)
and we take special case as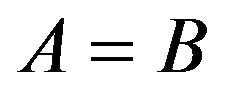 . Also we note here there are some following properties for this Darboux transformation,
. Also we note here there are some following properties for this Darboux transformation,
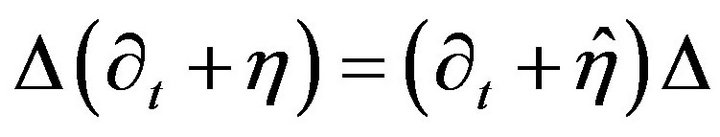 (7)
(7)
and
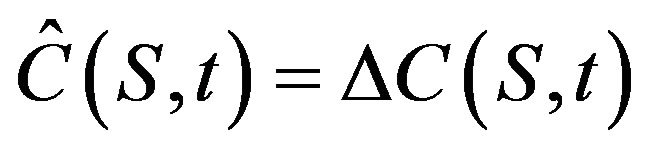 (8)
(8)
In order to obtain the parameter  we need to use the Equations (2) and (7), so we have,
we need to use the Equations (2) and (7), so we have,
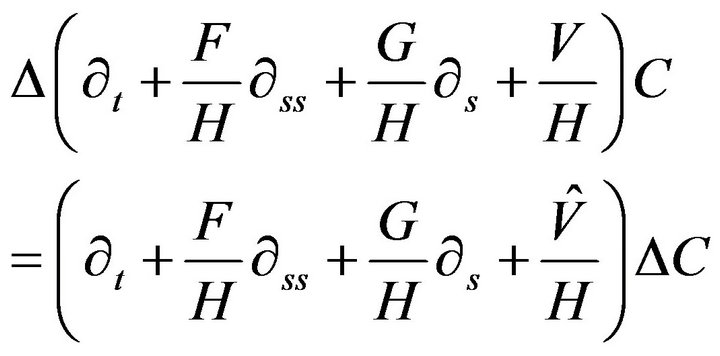 (9)
(9)
Making linear independence of  and its partial derivatives, we collect their respective coefficients and equal them to zero, from which we can obtain the following system about the functions
and its partial derivatives, we collect their respective coefficients and equal them to zero, from which we can obtain the following system about the functions  and
and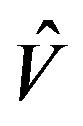 ,
,
 (10)
(10)
as we know the usual , so the
, so the 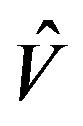 will be as,
will be as,
 (11)
(11)
which is modified potential and obtained by the Darboux transformation. By using the Equation (8) one can calculated the corresponding wave function 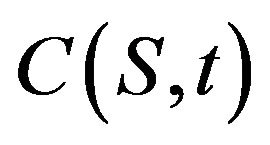 as,
as,
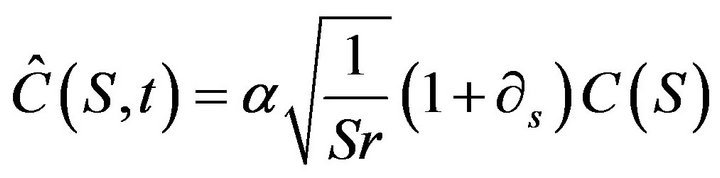 (12)
(12)
3. Supersymmetry and Darboux Transformation
In what follows we will prove that the formalism of supersymmetry for our generalized BS equation is equivalent to the Darboux transformation. Here we suppose the BS operator 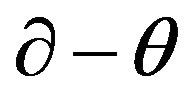 is self adjoint,
is self adjoint,
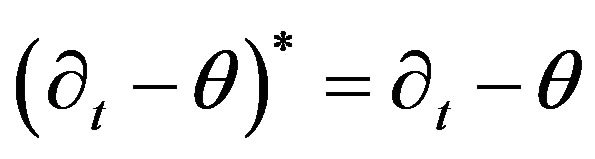 (13)
(13)
Taking the operation of conjugation on Darboux transformation (7), we obtain
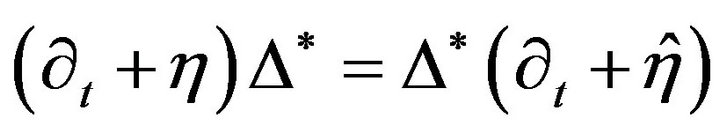 (14)
(14)
where the operator  adjoint to
adjoint to 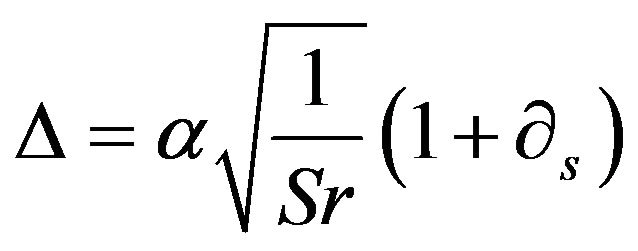 is given by,
is given by,
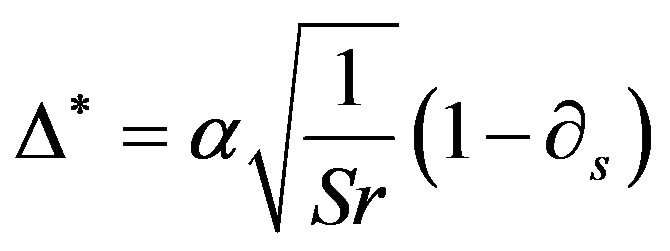 (15)
(15)
Equations (4) and (5) can then be rewritten as one single matrix equation of the form,
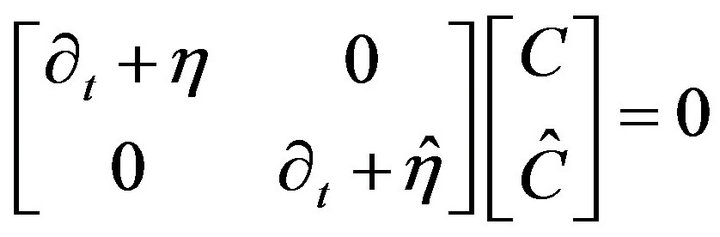 (16)
(16)
Assuming that 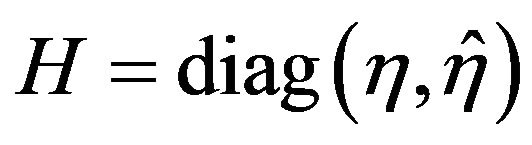 and
and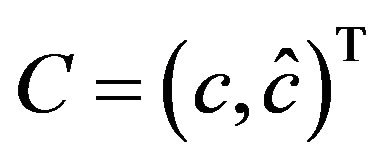 , the above equation can be written as,
, the above equation can be written as,
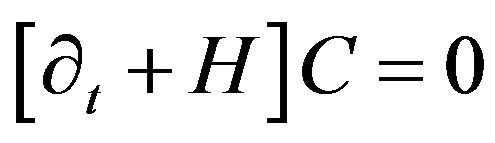 (17)
(17)
Two supercharge operators  and
and  are defined as follows,
are defined as follows,
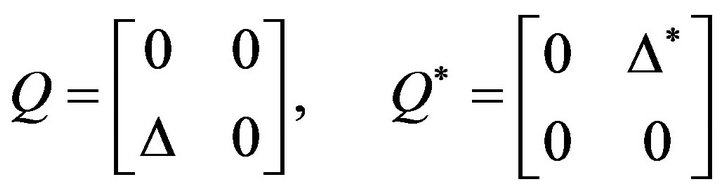 (18)
(18)
where  and
and  are the operator given by Equations (6) and (15), respectively. One can show that the Hamiltonian H satisfies the following expressions,
are the operator given by Equations (6) and (15), respectively. One can show that the Hamiltonian H satisfies the following expressions,


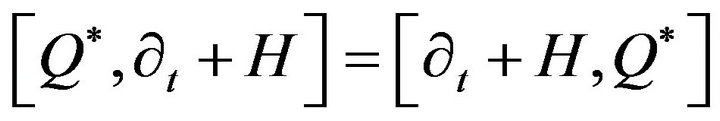 (19)
(19)
Considering the complementing relations of the supersymmetry algebra; the anti commutators 
and , we obtain the operators
, we obtain the operators 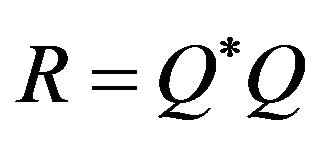 and
and
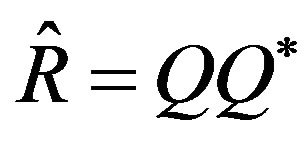 and consider the relations of them with our Hamiltonian
and consider the relations of them with our Hamiltonian 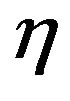 and
and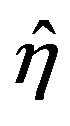 . So, one obtain the
. So, one obtain the 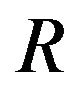 and
and  as follow,
as follow,
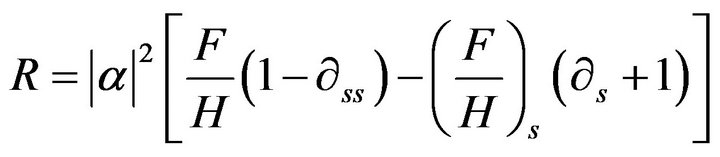 (20)
(20)
and
 (21)
(21)
where the indices 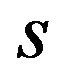 will be derivative with respect to
will be derivative with respect to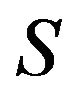 . In order to have shape invariance and supersymmetric algebra we need to obtain the
. In order to have shape invariance and supersymmetric algebra we need to obtain the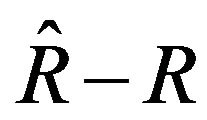 . If such value be constant and zero there is some supersymmetry partner for the such system. Otherwise we need to apply some condition in
. If such value be constant and zero there is some supersymmetry partner for the such system. Otherwise we need to apply some condition in 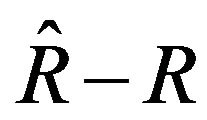 to have constant value. So, one can obtain the following equation for the
to have constant value. So, one can obtain the following equation for the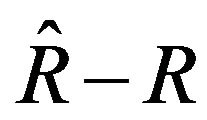 ,
,
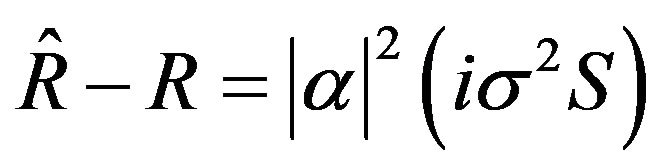 (22)
(22)
We mention here that if we want to supersymmetry algebra we need to have also the following commutation relation, and also anti-commutation relation between 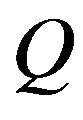 and
and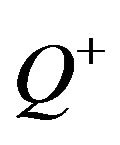 ,
,
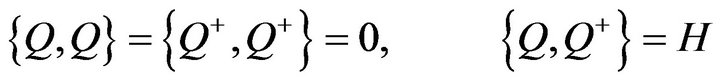 (23)
(23)
Finally we can say that the Equations (18), (19) and (23) lead us to apply the condition on the Equation (22) such that the expression 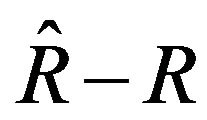 be constant. So, in that case,
be constant. So, in that case,  must be function of
must be function of 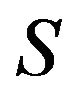 such as
such as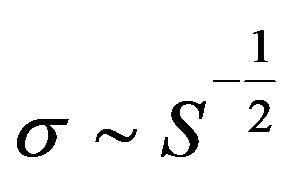 .
.
4. Conclusion
In this paper we studied the Black-Scholes (BS) equation. We used the first-order Darboux transformation and applied to the BS equation. In order to relate between supersymmetry and Darboux transformation we discussed the supersymmetry algebra and its commutation and anti-commutation super algebra. We have shown that for the satisfying such anticommutation supercharges the 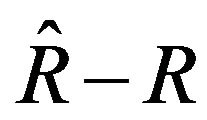 must be constant. Also, we applied the condition on the
must be constant. Also, we applied the condition on the 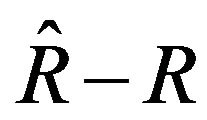 and shown that
and shown that  will be function of S as
will be function of S as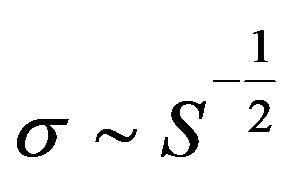 . This condition completely guarantees relation between supersymmetry and Darboux transformation.
. This condition completely guarantees relation between supersymmetry and Darboux transformation.