Intense Long-Lived Fluorescence of 1,6-Diphenyl-1,3,5-Hexatriene: Emission from the S1-State Competes with Formation of O2 Contact Charge Transfer Complex ()
1. Introduction
Carotenes constitute an important molecular class for photosynthesis. They are part of the light-harvesting complex, where they absorb visible light and transfer the energy to the reaction center of the photosystem. Alltrans-α,ω-diphenylpolyenes (also referred to as minicarotenes) are well established as model compounds for the bigger carotenoids such as β-carotene or lutein [1-6]. The latter absorb light and transfer the energy to the chlorophyll unit of the pigment [7]. Another function is protection of the photosynthetic apparatus against damage by highly reactive singlet oxygen [8]. The photophysical properties of these molecules are complex and not fully understood.
Because of its strong emission 1,6-diphenyl-1,3,5- hexatriene (DPH) is used as a fluorescence probe in biological membrane systems [9]. It is known, that the measured fluorescence lifetime is longer than that calculated using the Stickler-Berg [10] relationship and that the lifetime varies with the solvent [11,12]. Hudson and Kohler found evidence that the lowest excited singlet state in diphenyloctatetraene is the 1Ag-state with the 1Bu-state lying slightly above and applied this ordering of states to other polyenes as well [12-14]. Alford and Palmer stated that DPH emission occurs from both states [15]. In the gas phase fluorescence lifetimes up to 90 ns are reported and assigned to S1-(1Ag) state emission with intensity borrowing from the S2-(1Bu) state [16].
Combined density functional theory/multi reference configuration interaction (DFT/MRCI) calculations of the low-lying singlet and triplet states of mini-β-carotenes showed that the sequence of states depends on conjugation length and nuclear geometry [17]. According to the DFT/MRCI calculations for DPH the lowest excited singlet state upon vertical excitation is the 1Bu-state, while it switches order with the 1Ag-state during geometry relaxation [18]. Equilibration of the states takes place within less than a picosecond [19,20], due to the conical intersection between the S1 and S2 potential energy hypersurfaces [18].
Emission from the S1-state is symmetry forbidden, but it can occur via mixing with the S2-state, due to the small S1-S2 energy gap [21,22]. Unlike the S1-state, the energy of the S2-state is influenced by the solvent, which leads to a solvent dependent S1-S2 energy gap and because of the state mixing to a solvent dependent S1 radiative rate [14].
Saltiel et al. presented evidence showing that the fluorescence spectrum of DPH not only consists of the combined S1/S2-emission, but also of emission from the s-cis conformers of DPH [23-27]. Catalán confirmed the influence of s-cis conformers on the emission spectra also for other minicarotenes by calculations [28,29]. A detailed resolution of the emission spectra was accomplished by Turek et al. [30].
We observe DPH emission with an exceptionally long lifetime which decays non-exponentially under special experimental conditions. The long lifetime may be due to the emission originating from a forbidden transition. But to understand the unusual temporal behaviour, additional influences have to be considered. In this work we studied the temporal fluorescence behavior under different conditions like various DPH and O2 concentrations and different temperatures and excitation laser pulse energies to identify the various competing processes like formation of DPH-O2 contact charge-transfer (CCT) complexes, whose interplay leads to the linear decay of the longlived emission.
2. Materials and Methods
1,6-Diphenyl-1,3,5-hexatriene (98%, Aldrich) and cyclohexane (99+%, Acros Organics) were used without further purification. The nanosecond transient fluorescence setup (Applied Photophysics) utilizes the output from a frequency tripled (355 nm) pulsed Nd:YAG laser (Innolas) for photoexcitation. DPH samples were excited with 0.01 - 5 mJ pump energy at 5 ns laser pulse width and 1 or 10 Hz repetition rate. The short laser pulse is created by cutting out the maximum of the 12 ns pulse with a pockels cell. The pump beam of 1 cm diameter is directed into the sample (a flow-through 3.5 mL cuvette) perpendicular to the fluorescence sampling direction. If not stated otherwise, the solution reservoir was purged with nitrogen during the experiment to remove dissolved oxygen from the solutions. The same setup was used to saturate the solutions with oxygen. The samples were purged at least for 20 minutes before the start of the first measurement. The fluorescence light is dispersed behind the sample with a grating monochromator (Applied Photophysics) for wavelength selection. The output signal is detected by a photomultiplier (R928, Hamamatsu) and digitized by an oscilloscope (Agilent Infinium). Typically 16 laser pulses are averaged to record a kinetic trace at a selected wavelength. Stationary absorption spectra were recorded with a Cary 50 (Varian) spectrometer.
The simulations of the kinetic curves were performed with Mathcad 2001 (MathSoft, Inc.) by solving the coupled nonlinear partial differential equations by numerical integration.
3. Results and Discussion
1,6-Diphenyl-1,3,5-hexatriene in cyclohexane shows strong absorption in the region of 300 - 380 nm [11, 21,31]. The excitation wavelength used for our transient fluorescence measurements (355 nm) is near to the absorption maximum of DPH at 353 nm. The emission is very intense and can be detected in solutions with DPH concentrations below 10−10 mol/L (spectra not shown here). After excitation, the fluorescence kinetics can be investigated in our setup. Figure 1 shows the fluorescence decay of an 8.0 × 10−6 M DPH solution flushed with nitrogen. The fluorescence intensity reaches its maximum within the 5 ns laser pulse and subsequently decreases linearly until 50 ns after the laser pulse, where the decay becomes monoexponential. As can be seen in the inset, a monoexponential fit does not describe the observed fluorescence decay as well as a linear fit. Linear decay kinetics are characteristic for zero order reactions which are typical for systems saturated by the reactants (no concentration change) or for processes where the minority partner is regenerated, like a catalyst. The rate constant k obtained according to the rate law 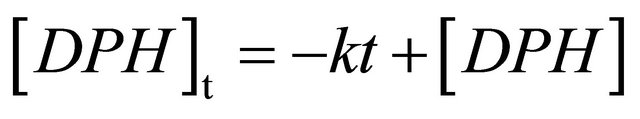 is 1.85 × 107 M∙s−1.
is 1.85 × 107 M∙s−1.
If the same sample is flushed with oxygen, fluorescence intensity and lifetime decrease dramatically (Figure 1).
Under these conditions the fluorescence decay is best described monoexponentially, with a decay time of τ = 4.59 ns ± 0.11 ns, which corresponds to the laser pulse width. Thus, the observed decay time does represent an upper limit for the emission lifetime. The dispersed emission spectra after laser excitation of both the nitrogen and oxygen flushed samples agree with the stationary emission spectrum, except for the distortion in the region around 470 nm, where S1→Sn absorption takes place [32,33]. If the oxygen flushed sample is flushed

Figure 1. Kinetic curves of DPH (8.0 × 10−6 M) at 427 nm upon excitation at 355 nm with 1 mJ pulse energy. The sample flushed with nitrogen shows long-lived fluorescence. In the presence of oxygen intensity and lifetime of the fluorescence are decreased significantly. The decay of the nitrogen flushed sample can be seen on a larger scale in the inset with monoexponential (dashed line) and linear (dotted line) fits.
again with nitrogen, the long-lived fluorescence reappears. The decrease of fluorescence lifetime and intensity is thus not caused by oxygen induced chemical degradation of DPH but by a reversible physical process.
Both intensity and lifetime of the long-lived fluorescence increase with increasing concentration, see Figure 2. At concentrations below 1 μM the fluorescence decays exponentially. Above 1 μM the decay shows the aforementioned linear behaviour. Therefore the fluorescence lifetimes shown in Figure 2 were obtained by monoexponential ([DPH] < 1 μM) and linear ([DPH] > 1 μM) fits of the kinetic traces. Upon excitation with 0.3 mJ pulse energy the lifetime reaches a plateau at 37 ns and does not increase further with increasing concentration. At concentrations below 1 μM the number of DPH molecules within the excitation volume is smaller than the number of photons irradiating the sample (Nphoton ≈ 5.4 × 1014). The saturation plateau at ≈ 1.8 µM DPH at 0.3 mJ pulse energy can thus be explained by nearly complete photon absorption if the number of molecules exceeds the number of photons sufficiently.
A further increase of fluorescence lifetime can be
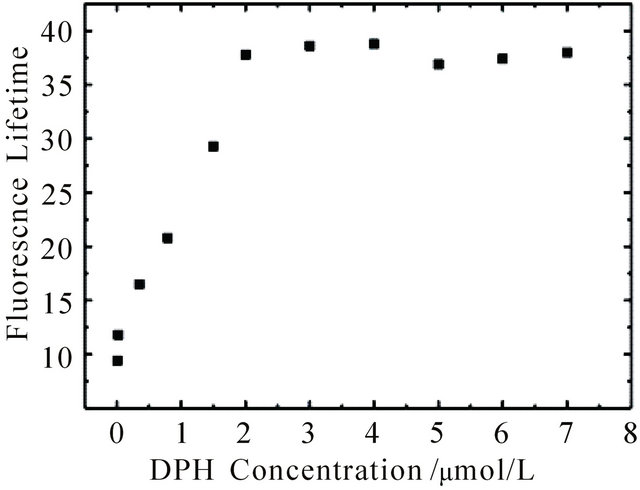
Figure 2. Fluorescence lifetime of DPH in cyclohexane at 427 nm upon excitation at 355 nm with 0.3 mJ pulse energy as a function of DPH concentration. The kinetic curves were fitted monoexponentially at concentrations below 1 mM (t given in ns) and linear above 1 mM (t given in ns∙M−1).

Figure 3. Dependence of fluorescence lifetime of DPH in cyclohexane (8.0 × 10−6 M) at 427 nm upon pumping at 355 nm on excitation laser pulse energy. For calculation of the lifetime the kinetic curves were fitted linear (t given in ns∙M−1).
reached by an increase of laser pulse energy. The effect of pulse energy on the fluorescence lifetime of an 8.0 × 10−6 M DPH solution is shown in Figure 3 between 0.01 mJ and 4 mJ. In the region of laser pulse energies below 1 mJ (Nphoton < 1.8 × 1015) the lifetime increases with increasing laser pulse energy, since the number of photons is smaller than the number of molecules (Nmolecule ≈ 2.4 × 1015). Above 1 mJ once again a plateau is reached (at 68 ns for the 8.0 × 10−6 M DPH solution). Our results thus show that fluorescence intensity and lifetime depend on the concentration of excited molecules.
The observed concentration dependence points to aggregate formation as possible cause of the long-lived fluorescence. It is well known that larger carotenoids are capable of forming aggregates easily. The emission of the aggregates is generally blue-shifted with respect to the emission of the monomer [34]. Increase of temperature leads to aggregate dissociation and a corresponding red-shift of the emission [35]. To check if the long-lived emission originates from DPH aggregates, the emission spectra of DPH were measured at different solution temperatures, spanning the complete range between melting point and boiling point of cyclohexane. The spectra at 8˚C and 75˚C are shown in Figure 4. While the shape of the kinetic traces stays unchanged (see Supplementary Material S1), it is evident that an increase of temperature leads to a decrease of fluorescence intensity. This behaviour is reversible, i.e. decreasing the temperature leads to recovery of the intensity. However, no spectral changes could be observed. Therefore, we assume that DPH does not form aggregates in cyclohexane to an appreciable extent in the concentration range of our studies. We attribute the change of intensity to an increased molecular diffusion rate at higher temperatures and therefore increased collisional quenching of the fluorescence.
The correct mechanism for generation of the longlived fluorescence has to take the following experimental findings into account: 1) fluorescence lifetimes up to ~70 ns, see Figure 1; 2) zero order decay of fluorescence intensity at short times followed by exponential decay,

Figure 4. Emission spectra of DPH in cyclohexane (8.0 × 10−6 M) upon excitation at 355 nm with 1 mJ pulse energy at 8˚C and 75˚C. The spectra were obtained by integrating the emission during the first 30 ns after the laser pulse.
see Figure 2; 3) significant population of the DPH triplet state, because we observed intense triplet-triplet absorption (spectra not shown here, since they are well known [19,20,24,36,37]; 4) fluorescence lifetime depends on the concentration of electronically excited DPH, see Figures 2 and 3; 5) fluorescence intensity decreases with increasing temperature, see Figure 4; 6) fluorescence is quenched by higher oxygen concentrations, see Figure 1.
Different mechanisms can be discussed to explain these effects. It is known, that triplet-triplet annihilation (TTA) generates excited singlet state population with an increased fluorescence lifetime. But TTA is diffusion controlled and therefore, at the concentrations studied in this work, too slow to explain the <5 ns fluorescence risetime observed in our experiments [38]. Furthermore, the triplet ground state of DPH is very low in energy, so that the energy available by triplet-triplet annihilation is insufficient to populate the lowest excited singlet state [24,39]. Additionally, the quantum yield for intersystem crossing (ISC) of DPH is very small (i.e. 0.02 in benzene and ethanol) [40]. Nevertheless the triplet state has to be included in any model explaining the long-lived fluorescence since ISC is a competing pathway for depopulation of excited singlet states.
Ionisation, followed by electron-cation recombination to electronically excited singlet states, could also cause long fluorescence lifetimes in principle. By the recombination process, the emitting singlet state becomes populated during a certain time period, which extends the emission time. To ionize DPH (ionisation potential: 7.27 eV [41]) upon excitation with 355 nm (3.49 eV) a multiphoton process is required. The lifetime should be longer in polar solvents but very short in nonpolar solvents, since electrons are not stabilized in nonpolar solvents and recombine immediately. All experiments presented here were performed in cyclohexane, a nonpolar solvent. We did observe long-lived fluorescence in other solvents as well, but no correlation between polarity of the solvent and fluorescence lifetime was discernible from our experiments. Therefore, ionisation and recombination is not considered to be important for the emergence of long-lived DPH fluorescence.
We instead propose the mechanism displayed in Figure 5 to explain the intense long-lived DPH fluorescence. The corresponding kinetic equations, which are used to simulate the observed kinetic traces, are as follows:
 (1.1)
(1.1)
 (1.2)
(1.2)

Figure 5. Proposed mechanism for emergence of long-lived fluorescence. Photoisomerization processes are not shown for clarity reasons.
 (1.3)
(1.3)
 (1.4)
(1.4)
After excitation of DPH into the S2-state a cascade of processes follows. Internal conversion into the S1-state (IC2,1) as well as the S0-state (IC2,0) occurs fast and competes with (short-lived) fluorescence (fl2) from the S2-state. The lifetime of the S1-state is affected by internal conversion into the ground state (IC1,0), reverse internal conversion into the S2-state (IC1,2) and intersystem crossing into the triplet state (ISC). Additionally, CCT complex formation with oxygen takes place. Other O2-induced processes may occur as competing processes, e.g. singulet oxygen formation or photoisomerization [42]. These processes, with the CCT complex formation as main reaction are described as oxygen quenching, (Q). In the simulation non-radiative, non-oxygen induced processes which depopulate the S1-state are indistinguishable from each other, as long as the emerging state or species has no influence on the S1-state. This includes IC1,0 and ISC as well as other possible processes, like isomerization (iso, not shown in Figure 5). Therefore it is appropriate to use a combined rate constant kdep for S1-state depopulation.
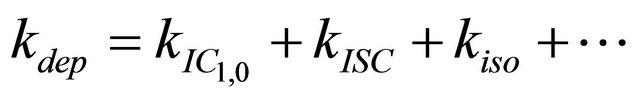 (2)
(2)
IC1,2 is not included, since here the S2-state is repopulated and subsequently IC2,1 takes place, which means that the S1-state population is influenced by the state emerging from this process. Emission from the S1-state to the electronic ground state (fl1) is symmetry forbidden and should be weak, but can be promoted by intensity borrowing via S1-S2-state mixing. The triplet state is depopulated within several microseconds (T) [24].
In the following we substantiate our model. Kohler and Spiglanin observed a DPH fluorescence lifetime of 90.7 ns in the gas phase and assigned this to emission from the S1-state which borrows intensity from the nearby S2-state [16]. We follow this assignment and ascribe the long-lived fluorescence observed in our experiments to emission from the S1-state. The zero order fluorescence decay of deoxygenated DPH solutions necessitates a quencher of constant concentration. The most obvious candidate is oxygen which at high concentrations quenches the long-lived fluorescence of DPH completely. Although the solutions were purged with nitrogen continuously during the experiments to avoid quenching, a small amount of oxygen remains in the solution [43]. It is well known, that molecular oxygen forms contact complexes with hydrocarbons [43-46], whether they are unsaturated, like DPH, or saturated, like cyclohexane (CH), from which absorption to the contact charge-transfer complex (M+O2−) can take place. Strong O2/CH CCT complex absorption occurs in the region around 210 nm [43]. A deconvolution of the broad absorption band was carried out for unpurged cyclohexane as well as for nitrogen and oxygen saturated cyclohexane, and the O2-CH contact complex concentrations were determined (see Table 1) using the extinction coefficients published by Brownrigg and Kenny [43] (for a detailed description see Supplementary Material S2). The same analysis was carried out for a solution of DPH in cyclohexane. By saturating the unpurged solutions with nitrogen, the contact complex concentration is reduced by at least an order of magnitude, but the amount of remaining oxygen is still considerable ([O2 − CH] = 0.2 mM).
We assume that oxygen bound in the O2-CH complex does not contribute to quenching of the DPH fluorescence in contrast to free oxygen which is in equilibrium with the O2-CH contact complex:
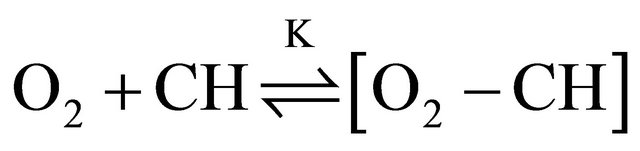 (3)
(3)
To include the oxygen quenching into the simulation it
Table 1. Concentration of the contact complex [O2-M] in cyclohexane and in a DPH/cyclohexane solution (8.0∙10−6 M), calculated from deconvoluted UV/Vis absorption and the extinction coefficients published by Brownrigg and Kenny [43].

a)Complex absorption too large to determine complex concentration.
is thus necessary to estimate the amount of free oxygen contained in the solution. It is safe to assume that the equilibrium is strongly located on the side of the contact complex [46-48]. For some hydrocarbons the value of the equilibrium constant K is known to be larger than 1000 M−1. In our case this would imply a free oxygen concentration on the order of 3 × 10−8 M. This is not sufficient to effectively quench the triplet state, but formation of contact complexes between oxygen and excited DPH molecules can occur and thereby depopulate the S1-state. Ultimately, the complexes will break apart under formation of ground or triplet state DPH and free O2 [44,45]. The O2-CH complex serves as oxygen reservoir, the concentration of free oxygen [O2] therefore can be regarded as constant. Directly after the laser pulse the O2 concentration is distinctly smaller than the concentration of S1-state DPH so that the reaction rate depends on constant [O2] and nearly constant [S1] which serves as excess reservoir. This results in zero order reaction kinetics. Later on, when [S1] and [O2] are in the same order of magnitude, the reaction rate depends on the declining S1 concentration and the constant [O2] and the quenching of S1-DPH becomes a first order reaction. With these assumptions made, the kinetic trace of the DPH fluorescence was simulated by fitting the rate constants to the experimental kinetic trace of the 8.0 × 10−6 M DPH solution, excited with 1 mJ pulse energy. The value for  was set to 5.0 × 104 s−1, according to the triplet state lifetimes known from literature [24,36,49,50] and [O2] to 0.03 µM according to the estimations described in Supplementary Materials S2.
was set to 5.0 × 104 s−1, according to the triplet state lifetimes known from literature [24,36,49,50] and [O2] to 0.03 µM according to the estimations described in Supplementary Materials S2.
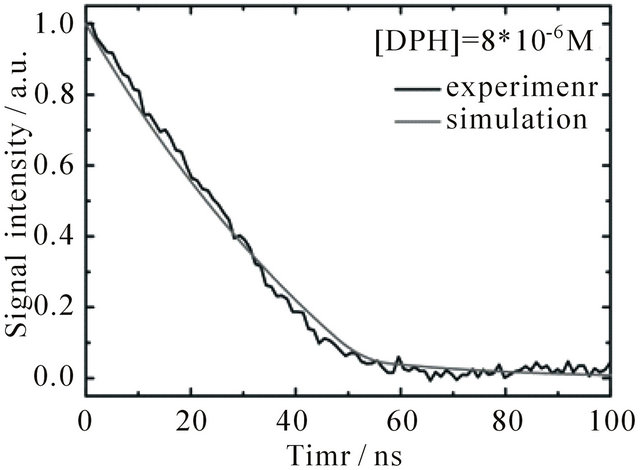
The fluorescence signal detected in the experiments is composed of contributions from the S1-state and the S2-state. For comparison to the emission intensity detected during the first 100 ns after the laser pulse, the time-dependent evolution of the combined S1 + S2 population derived from the simulation is depicted in Figure 6(a) (signal intensity simulation: grey curves). The resulting set of rate constants is displayed in Table 2 together with the time constants derived thereof.
The slope of the experimental data is emulated considerably better than by a simple monoexponential fit (see inset of Figure 1). Since the concentration of free oxygen could only be estimated, it is advisable to discuss the value of [O2]∙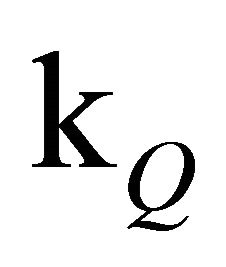 rather than
rather than  alone.
alone.
Apparently, the long emission lifetime detected in our experiments derives from the S1 fluorescence, while fluorescence from the S2-state with its lifetime of 1.3 ns (Table 2) cannot be held responsible. The value for  obtained in our simulation corresponds well with the Einstein probability of spontaneous emission (A21 = 7.98 × 108·s−1), which can be calculated via equation 4 with the oscillator strength ƒ21 = 2.16, determined by Ye et al.: [51,52].
obtained in our simulation corresponds well with the Einstein probability of spontaneous emission (A21 = 7.98 × 108·s−1), which can be calculated via equation 4 with the oscillator strength ƒ21 = 2.16, determined by Ye et al.: [51,52].
Table 2. Rate constants derived from the simulation of the time dependent fluorescence of DPH in cyclohexane (8.0 × 10−6 M), flushed with N2, upon excitation with 1 mJ pulse energy.

a)with free oxygen concentration [O2] = 0.03 µM.
 (a)
(a) (b)
(b)
Figure 6. Time dependent fluorescence of DPH in cyclohexane at 427 nm upon excitation with 1 mJ pulse energy, flushed with N2. The grey curves show the sum of concentrations of S1 and S2-state DPH obtained from the simulations. (a) [DPH] = 8.0∙10−6 M (b) [DPH] = 2.0∙10−8 M.
 (4)
(4)
Increasing the value for 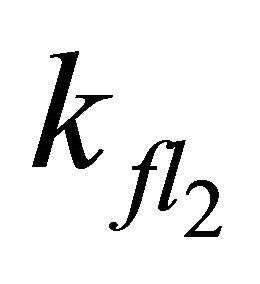 to the theoretical rate constant determined by Turek et al. [30] (
to the theoretical rate constant determined by Turek et al. [30] ( = 2.2 × 109·s−1) also leads to satisfying results with the rootmean-square deviation of the simulation slightly increasing from 0.005 to 0.006.
= 2.2 × 109·s−1) also leads to satisfying results with the rootmean-square deviation of the simulation slightly increasing from 0.005 to 0.006.
Simulations with  < 7.98 × 108 s−1 (even with
< 7.98 × 108 s−1 (even with  = 0) describe our experimental data equally well. However, exclusion of S2-state emission influences the simulation during the first picoseconds. Without S2-state emission, an increase of emission intensity should be observed within the first 5 ps. This rise should not be observable if emission from the S2-state occurs, since S2-state emission would superimpose the S1-state emission. Since this is far beyond our experimental time resolution, we can make no substantiated statement whether S2-state emission occurs in DPH or not.
= 0) describe our experimental data equally well. However, exclusion of S2-state emission influences the simulation during the first picoseconds. Without S2-state emission, an increase of emission intensity should be observed within the first 5 ps. This rise should not be observable if emission from the S2-state occurs, since S2-state emission would superimpose the S1-state emission. Since this is far beyond our experimental time resolution, we can make no substantiated statement whether S2-state emission occurs in DPH or not.
In simulations with larger  or smaller
or smaller  combined with smaller
combined with smaller  emission occurs from the S2-state with a long lifetime but the decay is strictly exponential rather than showing the described linear behaviour during the first 50 ns. The linearity can only be obtained by including the O2 quenching as competing process to S1 fluorescence.
emission occurs from the S2-state with a long lifetime but the decay is strictly exponential rather than showing the described linear behaviour during the first 50 ns. The linearity can only be obtained by including the O2 quenching as competing process to S1 fluorescence.
The simulation starting value for  was set to 1.6 × 1012 s−1, which is the value corresponding to the S2-state lifetimes obtained by Hirata et al. [20], but a better agreement with our experimental data was obtained with
was set to 1.6 × 1012 s−1, which is the value corresponding to the S2-state lifetimes obtained by Hirata et al. [20], but a better agreement with our experimental data was obtained with  = 7.3 × 1011 s−1. Decreasing
= 7.3 × 1011 s−1. Decreasing  to values below 1.0 × 1010 s-1 has no effect on the simulation at all, while higher values for this rate lead to insufficient simulations. Therefore 1.0 × 1010 s−1 is only an upper bound for
to values below 1.0 × 1010 s-1 has no effect on the simulation at all, while higher values for this rate lead to insufficient simulations. Therefore 1.0 × 1010 s−1 is only an upper bound for .
.
It was found that the fluorescence intensity decreases with increasing temperature, while the shape of the kinetic trace stayed unchanged. The emission intensity strongly depends on the S2-state concentration. At higher temperatures, depopulation of the S2-state by collisional quenching increases. Since this process competes with IC2,1, the S1-state is populated less at higher than at lower temperatures. This results in a decrease of emission intensity. Apparently, collisional quenching plays a minor role in S1-state depopulation.
With an adjusted initial [S2] the same rate constants can also be used to describe the fluorescence of DPH solutions at much lower concentrations, as is shown in Figure 6(b) for [DPH] = 2.0 × 10−8 M. In this case [S1] is already smaller than [O2] directly after the laser pulse. The fluorescence decay is therefore exponential, in the experiment as well as in the simulation. The emission in oxygen saturated solutions resembles the shape of the laser pulse with its lifetime of 5 ns, see Figure 1. In oxygen saturated solutions [O2] is much higher than the initial concentration of excited state DPH. This leads to a very fast O2-quenching of the S1-state in our simulations, which results in a fluorescence decay within 1 ns. Due to our 5 ns laser pulse it is not possible in our setup to measure the correct fluorescence lifetime of the oxygen saturated sample.
4. Conclusion
At high pulse energies of the excitation laser the singlet emission of 1,6-diphenyl-1,3,5-hexatriene can occur via two different pathways. On the one hand, after excitation to the S2-state, emission occurs directly from the pumped state. On the other hand, IC into the S1-state takes place. The fluorescence from the S1-state features long lifetimes since it is symmetry forbidden and can only occur by intensity borrowing via state mixing with the S2-state. The S1-state is depopulated by several processes like reverse IC, ISC and fluorescence. Most important competing process is the formation of DPH-O2 contact complexes, which at low oxygen concentrations considerably influences the kinetic trace of the long-lived fluorescence and quenches the long-lived fluorescence considerably at high oxygen concentrations.
5. Acknowledgements
The authors thank the Deutsche Forschungsgemeinschaft (KL 531/29-1) for financial support and Prof. Weinkauf for valuable discussions.
Supplementary Material 1

Figure S1: Kinetic curves of DPH (8.0 × 10−6 M in cyclohexane flushed with nitrogen) at 25˚C and 75˚C upon excitation at 355 nm with 1 mJ pulse energy. The emission intensity decreases with increasing temperature, while the shape of the kinetic curves does not depend on the temperature.
Supplementary Material 2

Figure S2. Absorption spectra and Gauss deconvolutions of the O2-CCT complex absorption in (a) unpurged cyclohexane; (b) cyclohexane saturated with nitrogen; (c) DPH in cyclohexane (8.0 × 10-6 M) purged with nitrogen. The experimental absorbance spectrum is depicted in black, the sum of the Gaussian curves in dashed grey (nearly perfect overlap with the black curve) and the individual Gaussian contributions in grey.
The intense curves with maxima <190 nm depend on O2 concentration and can thus be described to absorption of O2-M CCT complexes. In pure cyclohexane, neither purged with nitrogen or oxygen, the CCT absorption band can be emulated by one Gaussian curve with λmax = 186 nm and three distinctly smaller Gaussian curves with maxima at 199 nm, 194 nm and 218 nm. Unfortunately, it is not possible to investigate absorption bands below 190 nm with our UV/Vis spectrometer; hence λmax ≈ 186 nm is merely a guess. The sample was purged with nitrogen for 15 minutes and an absorption spectrum was measured every minute. Only the intensity of the Gaussian curve with λmax ≈ 186 nm had to be adjusted to emulate the spectra, while all other contributing curves remained unchanged. Therefore we assign this band to the O2-cyclohexane CCT complex absorption. The other bands are due to cyclohexane absorption and will be disregarded when calculating the contact complex concentration. Obviously the oscillator strengths of the CCT complex bands are much higher than those of uncomplexed cyclohexane in the displayed spectral range.
After seven minutes the sample was saturated with nitrogen and no changes could be observed in the absorption spectra any more. The measured CCT-absorption of the sample purged with oxygen for 15 minutes at 210 nm lies well beyond 1 O.D. where the Lambert-Beer law is not valid any more. For this sample no concentration could be determined. The concentration was calculated using the CCT extinction coefficients determined by Brownrigg and Kenny in the region of the red flank of the 186 nm band: ε210nm = 250 L∙mol−1∙cm−2, ε220nm = 120 L∙mol−1∙cm−2 and ε230nm =40 L∙mol−1∙cm−2 and the corresponding intensities obtained by our deconvolution. The concentrations given in table 1 in the article are obtained by averaging over the values for the different wavelengths.
The same analysis was performed for an 8.0 × 10−6 M DPH solution in cyclohexane. In this case not only the oxygen saturated but also the unpurged solution features an absorption band with intensities above 1 O.D. For the nitrogen saturated solution an additional Gaussian band with λmax = 189 nm was needed to emulate the CCT complex absorption, which may be due to formation of the O2-DPH CCT complex. Therefore, the summarized intensities of the Gaussian curves with λmax = 186 nm and λmax = 189 nm were used to calculate the combined contact complex concentration. It was assumed that the different CCT complexes exhibit comparable extinction coefficients.
NOTES