Local Existence and Uniqueness Theorem for a Nonlinear Schrödinger Equation with Robin Inhomogeneous Boundary Condition ()
1. Introduction
This paper is the continuation of an earlier one [1] where local existence and uniqueness theorem was presented for a one-dimensional nonlinear Schrödinger equation with initial condition and Dirichlet type inhomogeneous boundary condition on a finite domain
. As we know, many physically important nonlinear evolution equations in one spatial and one temporal dimensions have been found to possess exact solution by the method of inverse scattering transform (IST). For example, the IST technique has been applied to Cauchy problems in the infinite interval
for decaying [2],periodic [3] and self-similar potentials [4]. When a boundary condition is imposed, sometimes it is called forced problem [5] [6] [7] [8]. The physical importance of forced problems for the nonlinear integrable systems was discussed by Kaup [9]. Existence and uniqueness of the solution to the Korteweg-de Vries equation for
where
and
are given have been proven by Bona and Winther [10] [11].
The following nonlinear Schrödinger equation (NLS) posed in the quarter plane with Dirichlet inhomogeneous condition (k is a real constant) has been studied by the author:
(1.1)
The initial condition
and inhomogeneous boundary condition
are imposed. Existence and uniqueness of a global classical solution were proved via PDE method provided that the initial-boundary data are “nice” (cf. [12]). Further, (1.1) is shown to be well-posed [13].
For the NLS posed in the quarter plane with Robin inhomogeneous condition (
):
(1.2)
similar results were available [14] [15].
Solving such problems has important physical and mathematical implications. For example, (1.2) arises in the propagation of the optical solitons [16]. Also, the NLS with an additional term
on the right-hand side and
models water waves [17].
This paper will investigate a more general version of nonlinear Schrödinger equation
on a semi-infinite strip
. Robin type inhomogeneous boundary conditions are imposed on both endpoints. Using PDE method, we will prove the existence of a unique local classical solution.
2. Existence and Uniqueness of the Local Solution
In this paper, we study the following NLS with initial condition and Robin inhomogeneous boundary condition (
,
)):
(2.1)
here
is a real number and
. Using semigroup technique we prove that there exists a unique classical local solution.
We shall utilize the following notations and assume that
is an arbitrary real number throughout.
We assume that
have appropriate smoothness. In addition, they satisfy the necessary compatibility conditions to ensure the existence of solution at
and
,i.e.
and
.
Lemma 2.1. Let
,
. Then the operator A is the infinitesimal generator of a continuous semigroup of contractors  for
for . Here a is an appropriate positive constant depending on
. Here a is an appropriate positive constant depending on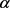 .
.
Proof. Let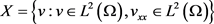 . Then X is a Banach space with a norm equivalent to
. Then X is a Banach space with a norm equivalent to 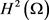 -norm. Let
-norm. Let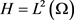 ,
, 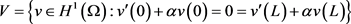 then
then  and V are dense in H. From Gagliardo-Nirenberg estimates (cf. [18] for details), we have
and V are dense in H. From Gagliardo-Nirenberg estimates (cf. [18] for details), we have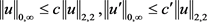 . Let
. Let 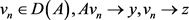 in H. Then clearly
in H. Then clearly  is a Cauchy sequence in X. Completeness of X implies that
is a Cauchy sequence in X. Completeness of X implies that 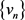 converges in X and
converges in X and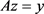 . Also,
. Also,

Similarly
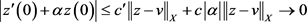
This shows that . Thus A is closed. To show that the resolvent set of A contains
. Thus A is closed. To show that the resolvent set of A contains , let
, let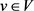 . Consider
. Consider
![]() (2.2)
(2.2)
Take the imaginary part of (2.2),
![]() (2.3)
(2.3)
By [18],there exists ![]() such that
such that
![]()
Then (2.3) becomes
![]()
If one sets ![]() then
then
![]()
By Theorem 2.3.3 of [19],for ![]() the operator
the operator ![]() maps
maps ![]() 1-1 onto H. Now let
1-1 onto H. Now let![]() . For
. For![]() , by taking the real part of (2.2), one has
, by taking the real part of (2.2), one has
the following inequality ![]() thus
thus![]() . By Hille-Yosida Theorem (cf. [20]), the unbounded and linear operator A is the infinitesimal generator of a continuous semigroup of a contractions
. By Hille-Yosida Theorem (cf. [20]), the unbounded and linear operator A is the infinitesimal generator of a continuous semigroup of a contractions ![]() for
for![]() .
.
Theorem 2.2 Local Existence-Uniqueness. For![]() ,
, ![]() , there exists a unique classical solution u for Equation (2.1) such that
, there exists a unique classical solution u for Equation (2.1) such that ![]() with either
with either ![]() as
as ![]() or
or![]() .
.
To prove this theorem, we first apply the following transformation:
![]() (2.4)
(2.4)
Substituting (2.4) into (2.1) yields
![]() (2.5)
(2.5)
where
![]()
![]()
One can converts (2.5) to an integral equation:
![]()
By similar analysis as in [12],G is locally Lipschitz in v under the norm of ![]() uniformly on
uniformly on ![]() and for each
and for each![]() , G is continuous from
, G is continuous from ![]() into
into ![]() (note
(note ![]() for any T). Thus one can use Theorem 6.1.7 in [20] to obtain the local existence-uniqueness theorem for (2.5) with
for any T). Thus one can use Theorem 6.1.7 in [20] to obtain the local existence-uniqueness theorem for (2.5) with ![]() with either
with either ![]() as
as ![]() or
or![]() . Since
. Since ![]() and
and ![]() uniformly on
uniformly on ![]() for any
for any![]() , we conclude immediately that u satisfies (2.1) and our existence and uniqueness are proved.
, we conclude immediately that u satisfies (2.1) and our existence and uniqueness are proved.
3. Conclusion
Famous nonlinear partial differential equations like nonlinear Schrödinger equation have important applications when the boundary value is not zero. For such equation posed in a semi-infinite strip, we used PDE method to prove that there exists a unique classical local solution, via semigroup theory. The PDE method presented in this paper to study the NLS is an approach different from the IST method in [7]. Along with [12],we try to provide some answers to the questions raised in [9]. There are some further research that could be done in this area. For example, we believe that well-posedness of (2.1) is a subject of research. Also, the existence of a global solution is an open problem. We will continue our work and report any further advances.
Acknowledgements
This research was supported by the William R. Kenan Jr. Professorship, a Brachman Hoffman Small Grant and a Wellesley College Faculty Award.