Properties of Fuzzy Length on Fuzzy Set

Jehad R. Kider, Jaafar Imran Mousa

Department of Mathematics and Computer Applications, School of Applies Sciences, University of Technology, Bagdad, Iraq.

DOI: 10.4236/oalib.1103068                

Abstract

The definition of fuzzy length space on fuzzy set in this research was introduced after the studies and discussion of many properties of this space were proved, and then an example to illustrate this notion was given. Also the definition of fuzzy convergence, fuzzy bounded fuzzy set, and fuzzy dense fuzzy set space was introduced, and then the definition of fuzzy continuous operator was introduced.

Keywords

Fuzzy Length Space on Fuzzy Set, Fuzzy Convergence, Fuzzy Cauchy Sequence of Fuzzy Point, Fuzzy Bounded Fuzzy Set and Fuzzy Continuous Operator

1. Introduction

Zadeh in 1965 [1] introduced the theory of fuzzy sets. Many authors have introduced the notion of fuzzy norm in different ways [2] - [9] . Cheng and Mordeson in 1994 [10] defined fuzzy norm on a linear space whose associated fuzzy metric is of Kramosil and Mickalek type [11] as follows:

The order pair 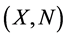is said to be a fuzzy normed space if X is a linear space and N is a fuzzy set on 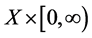satisfying the following conditions for every 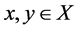and.

(i)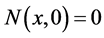, for all.

(ii) For all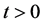, 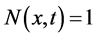if and only if.

(iii)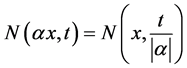, for all 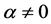and for all.

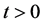(iv) For all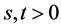, 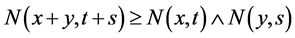where.

(v).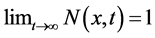

The definition of continuous t-norm was introduced by George and Veeramani in [12] . Bag and Samanta in [2] modified the definition of Cheng and Mordeson of fuzzy norm as follows:

The triple is said to be a fuzzy normed space if X is a linear space, 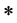is a continuous t-norm and N is a fuzzy set on satisfying the following conditions for every and.

(i), for all.

(ii) For all, if and only if.

(iii) for all.

(iv) For all,.

(v) For, is continuous.

(vi).

The definition of fuzzy length space is introduced in this research as a modification of the notion of fuzzy normed space due to Bag and Samanta. In Section 1, we recall basic concepts of fuzzy set and the definition of continuous t-norm. Then in Section 2 we define the fuzzy length space on fuzzy set after we give an example; then we prove that every ordinary norm induces a fuzzy length, and also the definition of fuzzy open fuzzy ball, fuzzy convergent sequence, fuzzy open fuzzy set, fuzzy Cauchy sequence, and fuzzy bounded fuzzy set is introduced. In Section 3, we prove other properties of fuzzy length space. Finally in Section 4, we define a fuzzy continuous operator between two fuzzy length spaces. Also we prove several properties for fuzzy continuous operator.

2. Basic Concept about Fuzzy Set

Definition 2.1:

Let X be a classical set of object, called the universal set, whose generic elements are denoted by x. The membership in a classical subject A of X is often viewed as a characteritic function from X onto {0, 1} such that if and if, {0, 1} is called a valuation set. If a valuation set is allowed to be real interval [0, 1] then A is called a fuzzy set which is denoted in this case by and is the grade of membership of x in. Also, it is remarkable that the closer the value of to 1, the more belong to. Clearly, is a subset of X that has no sharp boundary. The fuzzy set is completely characterized by the set of pairs:

[1] .

Definition 2.2:

Suppose that and are two fuzzy sets in Y. Then

(i).

(ii).

(iii).

(iv).

(v) [4] .

Definition 2.3:

Suppose that and be two fuzzy sets in and respectively then is a fuzzy set whose membership is defined by:

[8] .

Definition 2.4:

A fuzzy point p in Y is a fuzzy set with single element and is denoted by or. Two fuzzy points and are said to be different if and only if [11] .

Definition 2.5:

Suppose that is a fuzzy point and is a fuzzy set in Y. then is said to belongs to which is written by [2] .

Definition 2.6:

Suppose that h is a function from the set into the set. Let be a fuzzy set in W then is a fuzzy set in V its membership is:

for all d in V. Also when is a fuzzy set in V then is a fuzzy set in W its membership is given by: when and otherwise [5] .

Proposition 2.7:

Suppose that is a function. Then the image of the fuzzy point in V, is the fuzzy point in W with [11] .

Definition 2.8:

A binary operation is said to be t-norm (or continuous triangular norm) if the conditions are satisfied:

(i).

(ii).

(iii).

(iv) If and then [12] .

Examples 2.9:

When and then is a continuous t- norm [10] .

Remark 2.10:

, there is t such that and for every r, there is e such that, where p, q, t, r and e belongs to [0, 1] [12] .

Definition 2.11:

Let be a fuzzy set in Z and let τ be a collection of all subset fuzzy set in. then is called a fuzzy topological space on the fuzzy set if (i).

(ii) for any,.

(ii), for [10] .

Proposition 2.12:

Let be an arbitrary operator and and. Then if and only if [9] .

3. On Fuzzy Length Space

First we introduce the main definition in this paper.

Definition 3.1:

Let X be a linear space over field and let be a fuzzy set in X. let be a t- norm and be a fuzzy set from to [0,1] such that:

(FL₁) for all.

(FL₂) if and only if.

(FL₃) where.

(FL₄).

(FL₅) is a continuous fuzzy set for all and.

Then the triple is called a fuzzy length space on the fuzzy.

Definition 3.2:

Suppose that is a fuzzy length space on the fuzzy set then is con-

tinuous fuzzy set if whenever in then that is.

Proposition 3.3:

Let be a normed space, suppose that be a fuzzy set in Y. Put. Then, is a fuzzy normed space.

Proof:

Let and then

1) for all.

2).

3).

4).

Hence, is a fuzzy normed space.

Example 3.4:

Suppose that is a normed space and assume that is a fuzzy set in Y. Put

for all. Define.

Then is a fuzzy length space on the fuzzy set, is called the fuzzy length induced by.

Proof:

To prove is a fuzzy length space on the fuzzy set we must prove the five conditions of Definition 3.1:

(FL₁) Since for all so for all, where.

(FL₂) It is clear that for each if and only if.

(FL₃) If then for each, for each.

(FL₄) for each, where.

(FL₅) is continuous since is a continuous function.

Hence is a fuzzy length space on the fuzzy.

Definition 3.5:

Let be a fuzzy set in X, and assume that is a fuzzy length space on the

fuzzy. Let. So is said to be

a fuzzy open fuzzy ball of center and radius r.

We omitted the proof of the next result since it is clear.

Proposition 3.6:

In the fuzzy length space on the fuzzy, Let and with and. Then either or .

Definition 3.7:

The sequence in a fuzzy length space on the fuzzy is fuzzy converges to a fuzzy point if for a given, , then there exists a positive number K such that

Definition 3.8:

The sequence in a fuzzy length space on the fuzzy is fuzzy converges to a fuzzy point if

Theorem 3.9:

The two Definitions 3.8 and 3.7 are equivalent.

Proof:

Let the sequence is fuzzy converges to a fuzzy point, then for a given then there is a number K with for all, and hence. Therefore when.

To prove the converse, let when.

Hence when, there is K such that .

So. Therefore by Definition 3.7. ∎

Lemma 3.10:

Suppose that is a fuzzy length space on the fuzzy. Then for any.

Proof:

Let, then where

Lemma 3.11:

If is a fuzzy length space. Then,

(a) The operator is continuous.

(b) The operator is continuous.

Proof:

If and as then

Hence the operator addition is continuous function.

Now if, and and then

And this proves (b).

Definition 3.12:

Suppose that is a fuzzy length space and then is called fuzzy open if for every there is. A subset is called fuzzy closed if is fuzzy open.

The proof of the following theorem is easy and so is omitted.

Theorem 3.13:

Any in a fuzzy length space is a fuzzy open.

Definition 3.14:

Suppose that is a fuzzy length space, and assume that. Then the fuzzy closure of is denoted by or and is defined by is the smallest fuzzy closed fuzzy set that contains.

Definition 3.15:

Suppose that is a fuzzy length space, and assume that. Then is said to be fuzzy dense in if or.

Lemma 3.16:

Suppose that is a fuzzy length space, and assume that, Then if and only if we can find in such that.

Proof:

Let, if then we take the sequence of fuzzy points of the type. If, we construct the sequence of fuzzy points as follows: for each.

The fuzzy ball contains and since

Conversely assume that and then or the fuzzy open fuzzy ball of contains with, so is a fuzzy limit fuzzy point of. Hence.

4. Other Properties of Fuzzy Length on Fuzzy Set

Theorem 4.1:

Suppose that is a fuzzy length space and let that, then is fuzzy dense in if and only if for any we can find with for some.

Proof:

Let be a fuzzy dense in, and so then using 3.16 we can find with for all. Take,.

To prove the converse, we must prove that. Let then there is such that, where. Now take such that for each. Hence we have a sequence of fuzzy points such that for all that is so +

Definition 4.2:

Suppose that is a fuzzy length space. A sequence of fuzzy points is said to be a fuzzy Cauchy if for any given, there is a positive number K such that for all.

Theorem 4.3:

If is a sequence in a fuzzy length space with then is fuzzy Cauchy.

Proof:

If is a sequence in a fuzzy length space with. So

for any there is an integer K such that for all

. Now by Remark 2.10 there is such that. Now for each, we have

Hence is fuzzy Cauchy. ∎

Definition 4.4:

Suppose that is a sequence in a fuzzy length space let () be a sequence of positive numbers such that, then the sequence of fuzzy points is said to be a subsequence of.

Theorem 4.5:

Suppose that is a sequence in a fuzzy length space, if it is fuzzy converges to then every subsequence of is fuzzy converges to.

Proof:

Let be a sequence of fuzzy points in fuzzy converges to then. But is fuzzy Cauchy by Theorem (4.3) hence, as and. Now

.

Taking the limit to both sides as we get

Hence, fuzzy converges to, since was an arbitrary subsequence of. Therefore all subsequence of is fuzzy converges to.

Proposition 4.6:

Let be a fuzzy Cauchy sequence in a fuzzy length space con-

tains a such that, then fuzzy converges to

.

Proof:

Assume that is a fuzzy Cauchy sequence in. So for all, there is an integer K such that whenever

. Let be a subsequence of and. It follows that whenever. Since () is increasing sequence of positive integers. Now

Letting, we have.

Hence, the sequence it fuzzy converges to.

Definition 4.7:

Suppose that is a fuzzy length space and. Then is said to be fuzzy bounded if we can find q, 1 such that,.

Lemma 4.8:

Assume that is a fuzzy length space. If a sequence with then it is fuzzy bounded and its fuzzy limit is unique.

Proof:

Let, that is for a given r > 0 then we can find K with for all.

Put.

Using Remark 2.10 we can find with. Now for

Hence is fuzzy bounded in.

Suppose that and. Therefore and. Now . By taking the limit to both sides, as,. So, hence.

Definition 4.9:

Suppose that is a fuzzy length space on the fuzzy set. Then is said to be a fuzzy closed fuzzy ball with center and radius q,.

The proof of the following lemma is clear and hence is omitted.

Lemma 4.10:

Any in a fuzzy length space is a fuzzy closed fuzzy set.

Theorem 4.11:

A fuzzy length space is a fuzzy topological space.

Proof:

Suppose that is a fuzzy length space. Put if and only if there is such that. We prove that is a fuzzy topology on.

(i) Clear that and.

(ii) let and put. We will show that, let then for each. Hence there is such that. Put so for all, this implies that for all. Therefore, , thus.

(iii) Let. put. We will show that. Let, then for some, since then there exists such that, Hence. This prove that. Hence, is a fuzzy topological space. is called the fuzzy topology induced by.

Definition 4.12:

A fuzzy length space is said to be a fuzzy Hausdorff space if for any such that we can find and for some and such that.

Theorem 4.13:

Every fuzzy length space is a fuzzy Hausdorff space.

Proof:

Assume that is a fuzzy length space and let with.

Put, for some. Then for each s, , we can find q such that by Remark 2.10. Now consider the two fuzzy open fuzzy balls and. Then Since if there exists. Then

is a contradiction, hence is a fuzzy Hausdorff space.

5. Fuzzy Continuous Operators on Fuzzy Length Spaces

In this section, we will suppose is fuzzy set of X and be fuzzy set of Y where X and Y are vector space.

Definition 5.1:

Let and be two fuzzy length space on fuzzy set and respectively, let then The operator is said to be fuzzy continuous at, if for every there exist such that whenever satisfying. If T is fuzzy continuous at every fuzzy point of, then T it is said to be fuzzy continuous on.

Theorem 5.2:

Let and be two fuzzy length space, let. The operator is fuzzy continuous at if and only if whenever a sequence of fuzzy points in fuzzy converge to, then the sequence of fuzzy points fuzzy converges to.

Proof:

Suppose that the operator is fuzzy continuous at and let be a sequence in fuzzy converge to. Let be given. By fuzzy continuity of T at, then there exists such that whenever and, implies. Since

then we can find K with such that. Therefore when

implies. Thus.

Conversely, assume that every sequence in fuzzy converging to has the property that. Suppose that T is not fuzzy continuous at. Then there is and for which no, can satisfy the require-

ment that and implies. This means that for every there exists such that but. For every, there exist such that, but

. The sequence fuzzy converges to but the sequence does not fuzzy converge to (). This contradicts the assumption that every sequence in fuzzy converging to has the property that. Therefore the assumption that T is not fuzzy continuous at must be false.

Definition 5.3:

Let and be two fuzzy length spaces let and let the fuzzy point is a fuzzy limit of written by whenever if for every there is such that when and.

Proposition 5.4:

Let and be two fuzzy length spaces, let and. Suppose that is fuzzy limit fuzzy point of. Then if and only if for every sequence in such that and.

Proof: the argument is similar that of Theorem (5.3) and therefore is not included.

Proposition 5.5:

An operator T of the fuzzy length space into a fuzzy length space is fuzzy continuous at the fuzzy point if and only if for every there exist such that.

Proof: the operator is fuzzy continuous at if and only if for every there exist such that for all satisfying i.e. implies or this is equivalent to the condition

.

Theorem 5.6:

An operator is fuzzy continuous if and only if is fuzzy open in for all fuzzy open of where and are fuzzy length space.

Proof:

Suppose that T is fuzzy continuous operator and let be fuzzy open in we show that is fuzzy open in. Since and are fuzzy open, we may suppose that and.

Let then. Since is fuzzy open, then there is such that, since T is fuzzy continuous at by proposition 4.5 for this there exist such that.

Thus, every fuzzy point of is an interior fuzzy point and so if a fuzzy open in.

Conversely, suppose that is fuzzy open in for any fuzzy open of. Let for each, the fuzzy ball is fuzzy open in

since it follows that there exist such that by proposition 5.5, it follows that T is fuzzy continuous.

Theorem 5.7:

An operator is fuzzy continuous on if and only if is fuzzy closed in for all fuzzy closed of.

Proof:

Let be a fuzzy closed subset of then is fuzzy open in so that is fuzzy open in by theorem 4.6 but so is fuzzy closed in.

Conversely, suppose that is fuzzy closed in for all fuzzy closed subset of. But the empty fuzzy set and the whole space are fuzzy closed fuzzy set. Then is fuzzy open in and is fuzzy in. Since every fuzzy open subset of is of the type, where is suitable fuzzy closed fuzzy set, it follows by using theorem 5.6, that T is fuzzy continuous.

Theorem 5.8:

Let and and be three fuzzy length spaces let and be fuzzy continuous operator. Then the composition is a fuzzy continuous operator from into.

Proof:

Let be fuzzy open subset of. By theorem (5.6), is a fuzzy open subset of and another application of the same theorem shows that is a fuzzy open subset of, since it follows from the same theorem again that () is a fuzzy continuous.

Theorem 5.9:

Let and be two fuzzy length spaces let, then the following statements are equivalent.

(i) T is fuzzy continuous on.

(ii) For all subset of.

(iii) For all subset of.

Proof: (i) ⇒ (ii)

Let be a subset of, since is a fuzzy closed subset of, is fuzzy closed in. Moreover and so.

[Recall that is the smallest fuzzy closed fuzzy set containing].

Proof: (ii) ⇒ (iii)

Let be a subset of, then if, we have and thus.

Proof: (iii) ⇒ (i)

Let be a fuzzy closed fuzzy set in and fuzzy set. By theorem (4.7), it is sufficient to show that is a fuzzy closed in, that is, Now so that.

6. Conclusion

In this research the fuzzy length space of fuzzy points was defined as a generalization of the definition of fuzzy norm on ordinary points. Based on the defined fuzzy length, most of the properties of the ordinary norm were proved.

Conflicts of Interest

The authors declare no conflicts of interest.

References

[1]	Zadeh, L. (1965) Fuzzy Sets. Information and Control, 8, 338-452. https://doi.org/10.1016/S0019-9958(65)90241-X
[2]	Bag, T. and Samanta, S. (2003) Finite Dimensional Fuzzy Linear Spaces. Fuzzy Mathematics, 11, 678-705.
[3]	Bag, T. and Samanta, S. (2006) Fixed Point Theorems on Fuzzy Normed Spaces. Information Sciences, 176, 2910-2931. https://doi.org/10.1016/j.ins.2005.07.013
[4]	Wang, C.X. and Ming, M. (1993) Continuity and Boundness Mappings between Fuzzy Normed Spaces. Fuzzy Mathematics, 1, 13-24.
[5]	Felbin, C. (1992) Finite Dimensional Fuzzy Normed Linear Spaces. Fuzzy Sets and Systems, 48, 239-248. https://doi.org/10.1016/0165-0114(92)90338-5
[6]	Golet, I. (2010) On Generalized Fuzzy Normed Spaces and Coincidence Theorems. Fuzzy Sets and Systems, 161, 1138-1144. https://doi.org/10.1016/j.fss.2009.10.004
[7]	Kider, J. (2012) New Fuzzy Normed Spaces. Baghdad Science Journal, 9, 559-564.
[8]	Kider, J.R. (2013) Completeness of the Cartesian Product of Two Complete Fuzzy Normed Spaces. Engineering and Technology Journal, 31, 310-315.
[9]	Kider, J.K. and Hussain, Z.A. (2014) Continuous and Uniform Continuous Mapping on Standard Fuzzy Metric Spaces. Engineering and Technology Journal, 32, 1111-1119.
[10]	Cheng, S. and Mordeson, J. (1994) Fuzzy Linear Operators and Fuzzy Normed Linear Spaces. Bulletin of Calcutta Mathematical Society, 86, 429-436.
[11]	Kramosil, O. and Michalek, J. (1975) Fuzzy Metrics and Statistical Metric Spaces. Kybernetika, 11, 326-334.
[12]	George, A. and Veeramani, P. (1994) On Some Results in Fuzzy Metric Spaces. Fuzzy Sets and Systems, 64, 395-399. https://doi.org/10.1016/0165-0114(94)90162-7