A Computational Solution of Natural Convection Flow in a Rotating Fluid with Radiative Heat Transfer ()
Received 4 March 2016; accepted 10 June 2016; published 13 June 2016

1. Introduction
Extensive research efforts have been directed to the study of the theory of rotating fluids due to its application in Cosmical and Geophysical fluid dynamics, meteorology and engineering [1] . Bachelor [2] studied the Eckman layer flow on a horizontal plate. The flow past a horizontal plate has also been studied by [3] - [6] . In all these investigations, the effects of radiative heat transfer have been ignored. However, high temperature phenomena abound in solar physics, particularly in astrophysical studies. Naroua [7] presented a numerical simulation on un- steady hydromagnetic free convection near a moving infinite flat plate in a rotating medium. The temperatures involved are assumed to be very high so that the radiative heat transfer is significant, which renders the problem highly non-linear even with the assumption of a differential approximation for the radiative heat flux. Yamauchi et al. [8] presented modified finite-difference formulas for a general proposition of an interface that they applied to the propagating beam analysis of z-variant rib waveguides. They observed that a discretization error is satis- factorily reduced in tilted and tapered rib waveguides. Zhu et al. [9] analyzed explicit/implicit schemes for pa- rabolic equations with discontinuous coefficients. Numerical experiments, which were given for both linear and nonlinear problems, showed that their theoretical estimates were optimal in some sense. Khader and Ahmed [10] introduced a numerical simulation using finite difference method with the theoretical study for the problem of the flow and heat transfer over an unsteady stretching sheet embedded in a porous medium in the presence of a thermal radiation. Matsuoka and Nakamura [11] proposed a stable numerical scheme for a Cahn-Hilliard type equation with long-range interaction describing the micro-phase separation of diblock copolymer melts. They designed their scheme by using the discrete variational derivative method which is one of the structure preserving numerical methods. They observed that their proposed scheme has the same characteristic properties, mass conservation and energy dissipation, as the original equation does. They also discussed the stability and unique solvability of their proposed scheme.
This paper therefore incorporates radiative transfer into the study of natural convection in a rotating fluid the- reby widening the applicability of the results. For an optically thin gas in a transparent medium with the absorption coefficient α ( ) which will be assumed constant in the analysis, the radiative flux
) which will be assumed constant in the analysis, the radiative flux  satisfies the go- verning non-linear differential equation given by [12] :
satisfies the go- verning non-linear differential equation given by [12] :
 (1)
(1)
where 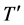 is the temperature of the fluid; subscript ∞ will be used to denote conditions in the undisturbed fluid and σ is the Stefan - Boltzmann constant.
is the temperature of the fluid; subscript ∞ will be used to denote conditions in the undisturbed fluid and σ is the Stefan - Boltzmann constant.
2. Mathematical Analysis
Consider the unsteady convective flow of an electrically conducting incompressible viscous fluid past an infinite vertical porous flat plate at z' = 0. Let the fluid and the plate be in a state of rigid rotation with uniform angular velocity Ω about the z' axis which is normal to the plate. A uniform magnetic field B0 is imposed along z'-axis and the plate is assumed to be electrically non-conducting. The temperature of the plate is maintained at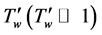 . Since the plate is infinite in extent, all the physical variables depend on z' and t' only. In a rotating frame of reference employing Equation (1), the governing equations for a transparent medium are given by:
. Since the plate is infinite in extent, all the physical variables depend on z' and t' only. In a rotating frame of reference employing Equation (1), the governing equations for a transparent medium are given by:
 (2)
(2)
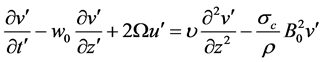 (3)
(3)
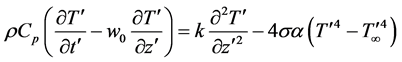 (4)
(4)
where 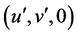 are the velocity components along x', y' and z' directions; ρ is the density; υ is the kinematic coefficient of viscosity; k is the thermal conductivity; Cp is the specific heat of the fluid; g is the acceleration due to gravity; β is the coefficient of volume expansion; σc is the electrical conductivity of the fluid and wo is the constant suction velocity.
are the velocity components along x', y' and z' directions; ρ is the density; υ is the kinematic coefficient of viscosity; k is the thermal conductivity; Cp is the specific heat of the fluid; g is the acceleration due to gravity; β is the coefficient of volume expansion; σc is the electrical conductivity of the fluid and wo is the constant suction velocity.
The boundary conditions are given by:
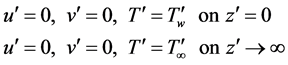 (5)
(5)
Introducing the following non-dimensional quantities
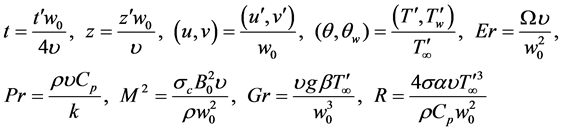 (6)
(6)
Equations (2)-(5) reduce to:

where
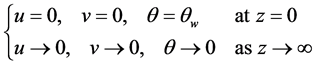 (10)
(10)
The above system of Equations (7)-(9) with boundary conditions (10) has been solved numerically by a generic software based on the Nakamura [13] scheme. Its’ genericity is in providing a common solution to the category of time dependent laminar fluid flow problems expressed in one spatial coordinate. It may be one- dimensional, two-dimensional or three-dimensional fluid flows. The mesh system is shown in Figure 1.
Equations (7)-(9) are coupled non-linear parabolic partial differential equations in u, v and q. First,  ,
, 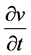
and ![]() are transformed using the backward difference approximation (which is stable) as shown in Equations
are transformed using the backward difference approximation (which is stable) as shown in Equations
(11)-(13):
![]()
where ![]() are derivatives with respect to z.
are derivatives with respect to z.
For the sake of simplicity, we write:
![]() ;
;
![]() ;
;
![]() .
.
Using the above formulation, Equations (11)-(13) take the form:
![]()
Using the central difference scheme which is unconditionally stable, Equations (14)-(16) reduce to:
![]()
At time step j + 1, Equations (17)-(19) reduce to:
![]()
If the problem is well defined, Equations (20)-(22) admit a solution but cannot be solved individually for each grid point i. The equations for all the grid points must be solved simultaneously. The set of equations for ![]() forms a tridiagonal system of equations as described by Nakamura [13] and shown in Equations (23)-(25).
forms a tridiagonal system of equations as described by Nakamura [13] and shown in Equations (23)-(25).
![]() (23)
(23)
where ![]() and
and ![]()
![]() (24)
(24)
where ![]() and
and ![]()
![]() (25)
(25)
where ![]() and
and ![]()
For each time step, the system of Equations (23)-(25) requires an iterative procedure due to the presence of non-linear coefficients. Successive substitution and iteration are continuously executed for each time step until convergence is reached.
3. Discussion of Results
In order to investigate the behavior of the velocity and temperature profiles, curves are drawn for various values of the parameters that describe the flow and are displayed in Figures 2-11.
![]()
Figure 2. Transient temperature profiles (θ).
![]()
Figure 3. Transient temperature profiles (θ).
![]()
Figure 4. Transient velocity profiles (u) for Gr = 10.
From Figure 2 and Figure 3, it is observed that:
1) the temperature profile (θ) increases due to an increase in time (t);
2) there is a fall in temperature profile (θ) due to an increase in Prandtl number (Pr);
3) there is a rise in temperature profile (θ) due to an increase in rotation parameter (Er);
4) there is an insignificant change in the temperature profile (θ) due to an increase in radiation parameter (R).
![]()
Figure 5. Transient velocity profiles (u) for Gr = 10.
![]()
Figure 6. Transient velocity profiles (u) for Gr = −10.
From Figure 4 and Figure 5, for Gr > 0 (in the presence of cooling of the plate by free convection currents) it is observed that:
1) the transient primary velocity profile (u) is backward;
2) the transient primary velocity profile (u) decreases due to an increase in time (t);
3) there is a rise in the transient primary velocity profiles (u) due to an increase in rotation parameter (Er) whereas the transient velocity field (u) decreases due to an increase in Prandtl number (Pr);
![]()
Figure 7. Transient velocity profiles (u) for Gr = −10.
![]()
Figure 8. Transient velocity profiles (v) for Gr = 10.
4) there is an insignificant change in the transient primary velocity profile (u) due to an increase in radiation parameter (R).
From Figure 6 and Figure 7, for Gr < 0 (in the presence of heating of the plate by free convection currents) it is observed that:
1) the transient primary velocity profile (u) increases due to an increase in time (t);
2) there is a fall in the primary velocity profile (u) due to an increase in rotation parameter (Er) whereas u rises with an increase in Prandtl number (Pr);
![]()
Figure 9. Transient velocity profiles (v) for Gr = 10.
![]()
Figure 10. Transient velocity profiles (v) for Gr = −10.
3) there is an insignificant change in the transient primary velocity profile (u) due to an increase in radiation parameter (R).
From Figure 8 and Figure 9, for Gr > 0 (in the presence of cooling of the plate by free convection currents), it is observed that:
1) the transient secondary velocity profile (v) increases due to an increase in time (t) and Prandtl number (Pr);
2) there is a fall in the transient secondary velocity profile (v) due to an increase in rotation parameter (Er)
![]()
Figure 11. Transient velocity profiles (v) for Gr = −10.
and radiation parameter (R).
From Figure 10 and Figure 11, for Gr < 0 (in the presence of heating of the plate by free convection currents), it is observed that:
1) the secondary velocity profile (v) is backward;
2) there is a fall in the secondary velocity profile (v) due to an increase in time (t) and Prandtl number (Pr);
3) there is a rise in the transient secondary velocity profile (v) due to an increase in rotation parameter (Er) and radiation parameter (R).
4. Conclusions
In this work, a simulation was carried out on the hydromagnetic free convective flow past a vertical infinite porous plate in a rotating fluid. Very large temperatures were assumed in the analysis in order to make the radia- tive heat transfer significant. The velocity and the temperature fields were computed using a generic software tool based on the Nakamura finite difference scheme. From the results obtained, we observed that:
1) the development of generic tools can drastically simplify the solution of fluid flow problems;
2) only the input equations and the relevant parameters need to be defined in a generic file which will be used as input to the simulation system.
3) the postfix code is very efficient in the computation of arithmetic expressions.