On Hom-Lie Pseudo-Superalgebras

Shengxiang Wang^1,2, Tingting Tao^2*

¹Department of Mathematics, Nanjing University, Nanjing, China.
²School of Mathematics and Finance, Chuzhou University, Chuzhou, China.

DOI: 10.4236/apm.2016.66029                

Abstract

The aim of this article is to introduce the notion of Hom-Lie H-pseudo-superalgebras for any Hopf algebra H. This class of algebras is a natural generalization of the Hom-Lie pseudo-algebras as well as a special case of the Hom-Lie superalgebras. We present some construction theorems of Hom-Lie H-pseudo-superalgebras, reformulate the equivalent definition of Hom-Lie H-pseudo-super-algebras, and consider the cohomology theory of Hom-Lie H-pseudo-superalgebras with coefficients in arbitrary Hom-modules as a generalization of Kac’s result.

Keywords

Hom-Associative Pseudo-Superalgebra, Hom-Lie Pseudo-Superalgebra, Hom-Lie Conformal Superalgebra, Hom-Annihilation Superalgebra, Cohomology

Received 19 March 2016; accepted 16 May 2016; published 19 May 2016

1. Introduction

The notion of conformal algebras [1] - [5] was introduced by Kac as a formal language describing the singular part of the operator product expansion in two-dimensional conformal field theory, and it came to be useful for investigation of vertex algebras (see [6] - [8] ). The concept of vertex algebras was derived from mathematical physics; it was first mathematically defined and considered by Borcherds in [9] to obtain his solution of the Moonshine conjecture in the theory of finite simple groups.

As a generalization of conformal algebras, Bakalov, D’Andrea and Kac [10] developed a theory of “multi- dimensional” lie conformal algebras, called Lie H-pseudo-algebras for any Hopf algebra H. Classification problems, cohomology theory and representation theory have been considered in [10] - [12] . In [13] , Boyallian and Liberati studied pseudo-algebras from the point of view of pseudo-dual of classical Lie coalgebra structures by defining the notions of Lie H-coalgebras and Lie pseudo-bialgebras.

In [33] , Ammar and Makhlouf introduced the notion of Hom-Lie superalgebras and provided a construction theorem from which one can derive a one parameter family of Hom-Lie superalgebras deforming the orthosymplectic Lie superalgebras. The notion of Hom-Lie superalgebras is a natural and meaningful generalization of Lie superalgebras which were introduced by Kac in [3] . Motivated by [4] [10] , in which Kac formulated the notion of conformal superalgebras and considered the classification theorem and representation theory of conformal superalgebras. We think whether we can extend the notions of Hom-Lie pseudo-algebras and conformal superalgebras to Hom-Lie pseudo-superalgebras.

Cohomology is an important tool in mathematics. Its range of applications contains algebra and topology as well as the theory of smooth manifolds or of holomorphic functions. The cohomology theory of Lie algebras was developed by Chevalley, Eilenberg and Cartan. Scheunert and Zhang introduced and investigated the cohomology groups of Lie superalgebras in [34] . Naturally, we think whether we can extend the notion of cohomology groups to Hom-Lie H-pseudo-superalgebras. This becomes our second motivation of the paper.

To give a positive answer to the questions above, we organize this paper as follows. In Section 2, we recall some basic definitions about Lie pseudo-algebras. In Section 3, we define Hom-Lie pseudo-superalgebras and introduce two construction theorems of Hom-Lie pseudo-superalgebras (see Proposition 3.12 and Theorem 3.13). In Section 4, we mainly discuss the annihilation superalgebras of Hom-pseudo-superalgebras (see Proposition 4.5). In Section 5, we determine some equivalent definitions of Hom-pseudo-superalgebras. In Section 6, we discuss the cohomology of Hom-Lie H-pseudo-superalgebras (see Theorem 6.1).

2. Preliminaries

In this section we recall some basic definitions and results related to our paper. Throughout the paper, all algebraic systems are supposed to be over a field k of characteristic 0, H always denotes a Hopf algebra with an antipode S. We summarize in the following the ungraded definitions of Hom-associative and Hom-Lie H-pseudo- algebras (see [14] ). The reader is referred to Sweedler [35] about Hopf algebras, the Sweedler-type notation for the comultiplication is denoted by:.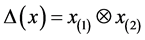

Recall that a pseudotensor category 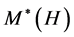is a category whose objects are the same objects as in the category 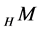of left H-modules, but with a non-trivial pseudotensor structure, see [10] .

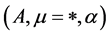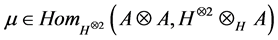A Hom-associative H-pseudo-algebra [14] is a triple consisting of a linear space A in, an operation and a homomorphism 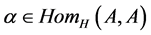satisfying

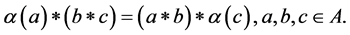(2.1)

A Hom-Lie H-pseudo-algebra [14] is a triple 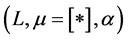consisting of a linear space L in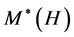, an operation 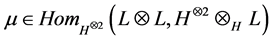and a homomorphism 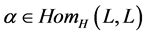satisfying the following axioms ():

1) Skew-commutativity:

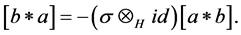(2.2)

2) Hom-Jacobi identity:

(2.3)

An elementary but important property of Hom-Lie H-pseudo-algebra is that each Hom-associative H-pseudo- algebra gives rise to a Hom-Lie H-pseudo-algebra via the commutator bracket.

A Hom-Lie H-conformal algebra ( [14] ) is a triple consisting of a linear space L in, an operation and a homomorphism satisfying the following axioms (and):

1) H-sesqui-linearity:

(2.4)

2) Skew-commutativity:

(2.5)

3) Hom-Jacobi identity:

(2.6)

Recall from Sun [14] we know that one can reformulate the definition of a Hom-Lie H-pseudo-algebra via a Hom-Lie H-conformal algebra.

3. Hom-Pseudo Superalgebras of Associative and Lie Types

In this section we will introduce the concept and construction theorems of Hom-H-pseudo-superalgebras of associative and Lie types, and show some examples of Hom-Lie H-pseudo-superalgebras that are neither Hom-Lie superalgebras nor Hom-Lie pseudo-algebras.

Definition 3.1. A Hom-associative H-pseudo-superalgebra is a triple consisting of a superspace A in, an even operation and an even homomorphism satisfying

(3.1)

in for all homogeneous elements

Example 3.2. For a one dimensional Hopf algebra H = k, a Hom-associative H-pseudo-superalgebra is just a Hom-associative superalgebra over k. If, then a Hom-associative H-pseudo-superalgebra is an associative H-pseudo-superalgebra.

A Hom-associative H-pseudo-superalgebra is called multiplicative if. For example, if, then the Hom-associative H-pseudo-superalgebra is multiplicative.

Let and be two (multiplicative) Hom-associative H-pseudo-superalgebras, an even homomorphism is said to be a morphism of Hom-associative H-pseudo-superalgebras if

(3.2)

Definition 3.3. Let be a Hom-associative H-pseudo-superalgebra and M be a superapace in A Hom-A-module is a triple, where is an even morphism in, is an even morphism in and satisfies the following properties ():

(3.3)

(3.4)

where.

Example 3.4. Let be a finite dimensional Hom-associative superalgebra, H be a Hopf algebra. Then is a Hom-associative H-pseudo-superalgebra with pseudoproduct given by

for all and homogeneous elements

Definition 3.5. A Hom-Lie H-pseudo-superalgebra is a triple consisting of a superspace L in, an even operation and an even homomorphism satisfying the following axioms:

1) Skew-commutativity:

(3.5)

2) Hom-Jacobi identity:

(3.6)

where a, b, c are homogeneous elements in L.

Here and further, is the parity of a.

Example 3.6. For a one dimensional Hopf algebra H = k, a Hom-Lie H-pseudo-superalgebra is just a Hom-Lie superalgebra over k. If, then a Hom-Lie H-pseudo-superalgebra is a Lie H-pseudo-superalgebra.

Example 3.7. Let H be a Hopf algebra and a 2-dimensional linear superspace, where is generated by x and is generated by y. Then is a Hom-Lie H-pseudo-superalgebra, where is a free pseudo-algebra of rank 2 with pseudoproduct given by in is any even homomorphism in

Example 3.8. Let be a finite dimensional Hom-Lie superalgebra, H be a Hopf algebra. Then is a Hom-Lie H-pseudo-superalgebra with pseudoproduct given by

for all and homogeneous elements

Example 3.9. Let H be a Hopf algebra and a 3-dimensional linear superspace, where A₀ is generated by x, y and A₁ is generated by z. Then is a Hom-Lie H-pseudo-superalgebra defined by any even homomorphism and operation

In particular, if, then the Hom-Lie H-pseudo-superalgebra is noting but the affine Hom-Lie superalgebra in [33] .

A Hom-Lie H-pseudo-superalgebra is called multiplicative if. For example, if, then the Hom-Lie H-pseudo-superalgebra is multiplicative.

Let and be two (multiplicative) Hom-Lie H-pseudo-superalgebras. An even homomorphism is said to be a morphism of Hom-Lie H-pseudo-superalgebras if

(3.7)

Definition 3.10. Let be a Hom-Lie H-pseudo-superalgebra and M a superspace in A Hom-L-module is a triple, where is an even morphism in, is an even morphism in and satisfies the following axioms:

(3.8)

where, a, b and m are homogeneous elements in L and M respectively.

In the following, we will show that the supercommutator bracket defined using the multiplication in a Hom- associative H-pseudo-superalgebra leads naturally to a Hom-Lie H-pseudo-superalgebra.

Lemma 3.11. Let be a Hom-associative H-pseudo-superalgebra. Then

1)

2)

3)

Proof. We only prove (3), and similarly for (1), (2). For any homogeneous elements let

On one hand we have

since H is cocommutative. Similarly, we have

as required. So (3) holds since A is Hom-associative. ,

Proposition 3.12. Given any Hom-associative H-pseudo-superalgebra, one can define the bracket pseudoproduct on homogeneous elements by

(3.9)

and then extending by linearity to all elements. Then is a Hom-Lie H-pseudo-superalgebra.

Proof. We shall show that the condition (3.9) leads A to be a Hom-Lie H-pseudo-superalgebra, in the sense of Definition 3.5. For this purpose, we first claim that the bracket pseudoproduct is both H-bilinear and skew- commutative, but these are easy to check. It remains to verify that the conditions (2) of Definition 3.5 are satisfied by the condition (3.9). Now we have the following calculations:

Immediately, we can obtain, then

It follows from Lemma 3.12 that

Furthermore, we have

Together with the above results, we finally obtain

The proof is completed. ,

Next we will construct Hom-Lie H-pseudo-superalgebras from Lie H-pseudo-superalgebras and even Hom- Lie superalgebra endomorphisms, generalizing the results for Hom-Lie H-pseudo-algebras in [14] and Hom-Lie superalgebras in [33] .

Theorem 3.13. Let be a Lie H-pseudo-superalgebra and an even endomorphisms of L. Defining

by for all homogeneous elements x, y in L, then

is a Hom-Lie H-pseudo-superalgebra.

Moreover, suppose that is another Lie H-pseudo-superalgebra and is an even endomorphisms of. If is a morphism of Lie H-pseudo-superalgebras that satisfies, then

(3.10)

is a morphism of Hom-Lie H-pseudo-superalgebras.

Proof. We shall show that satisfies the skew-commutativity and the Hom-Jacobi identity. For any homogeneous elements in L,

Since is an endomorphism of L,

Therefore we have

as needed. To show that f is a morphism of Hom-Lie H-pseudo-superalgebras, we do the calculations:

The proof is completed. ,

To provides another way to construct Hom-Lie H-pseudo-superalgebras and Hom-associative H-pseudo- superalgebras, we first recall the definition of current H-pseudo-algebras in [10] .

Let be a Hopf subalgebra of H and A an H'-pseudo-algebra. Then define the current H-pseudo-algebra by extending the pseudoproduct of A using the H-bilinearity. Explicitly, for any, define

(3.11)

if. Then is an H-pseudo-algebra which is Lie or associative when A is so.

Proposition 3.14. Let H' be a Hopf subalgebra of H and a Hom-Lie H'-pseudo-superalgebra. Then is a Hom-Lie H-pseudo-superalgebra, where is the multiplication of CurL. Moreover, there is a similar result in the case of Hom-associative H'-pseudo-superalgebras as well.

Proof. We only prove the case of Hom-Lie H'-pseudo-superalgebras, the Hom-associative case is similar. We denote

(3.12)

It is obviously that the skew-commutativity holds since is a Hom-Lie H'-pseudo-superalgebra. So it is sufficient to verify the Hom-Jacobi identity. For any, suppose

Since is a Hom-Lie H'-pseudo-superalgebra, we have

that is,

By the multiplication of, we obtain

Hence is a Hom-Lie H-pseudo-superalgebra. This ends the proof. ,

4. Hom-Annihilation Superalgebras

In this section we will study the annihilation superalgebras of Hom-H-pseudo-superalgebras. First of all we will give the definition of H-differential superalgebras.

Definition 4.1. An associative superalgebra Y is called an associative H-differential superalgebra if it is a left H-module such that, for all and homogeneous elements.

Let Y be an H-bimodule which is a commutative associative H-differential superalgebra. For a left H-module L, it is easy to see that is a left H-module via, for all and.

The definition of Hom-Lie H-differential-superalgebras can be obtained similarly.

Proposition 4.2. Let Y be a Hom-Lie H-differential-superalgebra and a Hom-Lie H-pseudo- superalgebra. Then A_YL is a Hom-Lie H-differential superalgebra, where the bracket and the action are given by

(4.1)

(4.2)

for all and, where.

Proof. First we shall show that A_YL is an H-module, but this is easy to check. It remains to verify that the conditions (1) and (2) in Definition 3.5 are satisfied. For this purpose, we take, and suppose

Since L is a Hom-Lie H-pseudo-superalgebra, then therefore we have

as required. Next we verify the Hom-Jacobi identity by the following calculations:

Similarly, by exchanging the status of the element, we have

By the Hom-Jacobi identity of L, we have

Hence

So A_YL is a Hom-Lie H-differential superalgebra. This completes the proof. ,

Remark 4.3. In particular, when, is a Hom-Lie H-differential superalgebra, we call it Hom-annihilation superalgebra of the Hom-Lie H-pseudo-algebra L and write for any homogeneous elements and

Remark 4.4. A similar statement holds for Hom-associative H-pseudo-superalgebras and Hom-modules as well. For example, if is a Hom-L-module, then is a Hom-A_YL- module with a compatible H-action, where

(4.3)

if for any homogeneous elements and

Proposition 4.5. Let be a Hom-Lie H-pseudo-superalgebra and Y a commutative associative H-differential superalgebra with a right action of H. Then is a Hom-Lie H-pseudo- superalgebra with bracket pseudoproduct

(4.4)

if for any homogeneous elements

Proof. According to the bracket pseudoproduct defined above, it is easy to see that H-bilinearity holds. To verify the Skew-commutativity and Hom-Jacobi identity, take and suppose

Since L is a Hom-Lie H-pseudo-superalgebra, therefore we have

That is, the skew-commutativity holds. So it is sufficient to verify the Hom-Jacobi identity. Since

we have

Similarly, by exchanging the status of the element, we have

By the Hom-Jacobi identity of L, we have

it follows that

So A_YL is a Hom-Lie H-pseudo-superalgebra. This completes the proof. ,

5. Hom-Lie Conformal Superalgebras

In this section we will reformulate the definition of Hom-Lie (or Hom-associative) H-pseudo-superalgebras. The resulting notion, equivalent to that of Hom-H-pseudo-superalgebras, will be called Hom-H-conformal superalgebras.

Let us start by racalling the definitions of the Fourier transform and the x-brackets in [10] . For an arbitrary Hopf algebra H, the Fourier transform is defined by F is an isomorphism with an inverse given by The significance of Fourier transform F is the identity

(5.1)

In order to reformulate the definition of a Lie (or associative) H-pseudo-algebra, Bakalov, D'Andrea and Kac introduced the bracket as the Fourier transform of:

That is,

Then for, the x-bracket is defined in [3] as follows:

Let be a Hom-Lie H-pseudo-superalgebra. For any homogeneous elements, suppose

Then we have

Similarly, we can obtain thus

Therefore

is equivalent to

So the definition of Hom-Lie H-pseudo-superalgebra can be equivalently reformulated as follows.

Definition 5.1. A Hom-Lie H-conformal superalgebra is a triple consisting of a superspace L in, an even operation and an even homomorphism satisfying the following axioms:

1) H-sesqui-linearity:

(5.2)

2) Skew-commutativity:

(5.3)

3) Hom-Jacobi identity:

(5.4)

where a, b, c are homogeneous elements in L and.

One can also reformulate Definition 4.1 in terms of x-brackets as below.

Definition 5.2. A Hom-Lie H-conformal superalgebra is a triple consisting of a superspace L in, an even operation and an even homomorphism satisfying the following axioms:

1) Locality:

(5.5)

2) H-sesqui-linearity:

(5.6)

3) Skew-super commutativity:

(5.7)

4) Hom-super Jacobi identity:

(5.8)

where and are dual bases of X and H, a, b, c are homogeneous elements in L, and.

In the following we will show that there is a simple relationship between the x-bracket of a Hom-Lie H-con- formal superalgebra and the commutator in its annihilation Hom-Lie H-pseudo-superalgebra defined in Proposition 4.5. Let be dual linear basis of H and X. Then we have

According to Proposition 4.2, we obtain

In other words,

Below we give one way of constructing Hom-modules over Hom-Lie H-pseudo-algebras, whose proofs are similar to that in [10] .

Proposition 5.3. Any Hom-module over a Hom-Lie H-pseudo-superalgebra has a natural structure of a Hom-A(L)-module, given by, where

(5.9)

for all homogeneous elements and. This action is compatible with the action of H, that is,

for all homogeneous elements and, and satisfies the locality

condition: for any homogeneous elements and.

Conversely, any Hom-A(L)-module satisfying the above conditions has a natural structure of an Hom-L-module, given by

(5.10)

where and are dual linear basis of H and X.

6. Cohomology of Hom-Lie H-Pseudo-Superalgebras

In this section, we will consider the cohomology of Hom-Lie H-pseudo-superalgebras, generalizing the results of Hom-Lie H-pseudoalgebras and Lie superalgebras.

Let be a Hom-Lie H-pseudo-superalgebra, is a Hom-L-module. Let be a natural number and let be the superspace of all homogeneous skew-symmetric cochains satisfies

(6.1)

Explicitly, has the following defining properties:

1) H-polylinearity: For any and,

(6.2)

2) Skew-supersymmetry: For any

(6.3)

where is the transposition of the ith and st factors.

The map is called even (resp. odd) when (resp.) for all even (resp. odd) elements, where the parity of the element is We denote the parity of the map by.

For, the map is defined as follows:

(6.4)

where is the permutation, is the permutation and the sign ^ indicates that the element below it must be omitted. In particular, for we have

(6.5)

and for we obtain

(6.6)

The fact that is most easily checked and the same argument is in the usual Lie superalgebra case in [26] [36] [37] and Hom-Lie H-pseudoalgebra case in [34] . The cohomology of the resulting complex is called the cohomology of with coefficients in and is denoted by

One can also modify the above definition by replacing everywhere by. Let consist of all skew-symmetric cochains. Then we can define a differential by (6.1) with replaced by everywhere; then again The corresponding cohomology will be called the basic cohomology of with coefficients in. In contrast, is sometimes called the reduced cohomology.

In the following we will show that the cohomology theory of Hom-Lie H-pseudo-superalgebras describes extensions and deformations, just as any cohomology theory.

Theorem 6.1. Let be a multiplicative Hom-Lie H-pseudo-superalgebra, and be a Hom-L-module, considering a Hom-Lie H-pseudo-superalgebra with respect to the zero pseudobracket, then the equivalence classes of H-split abelian extensions

(6.7)

of the Hom-Lie H-pseudo-superalgebra correspond bijectively to, the homogeneous component of degree zero of the reduced cohomology.

Proof. Let be an extension of L-modules, which is split over H. Choosing a splitting as an H-module, and denoting the pseudobracket of by, we have for all:

(6.8)

It is not hard to verify that is a homogeneous 2-cochain of degree zero, i.e., The Hom- super Jacobi identity of L and implies in the sense of (6.1).

Conversely, given an element of, we can choose a representative and define an action by (6.2). Then depends only on the. ,

Acknowledgements

The paper is partially supported by the Project Funded by China Postdoctoral Science Foundation (No. 2015M571725), the Key University Science Research Project of Anhui Province (Nos. KJ2015A294 and KJ2014A183) and the NSF of Chuzhou University (Nos. 2015qd01, 2014qd008 and 2014PY08).

NOTES

^*Corresponding author.

Conflicts of Interest

The authors declare no conflicts of interest.

References

[1]	Bakalov, B., Kac, V.G. and Voronov, A.A. (1999) Cohomology of Conformal Algebras. Communications in Mathematical Physics, 200, 561-598. http://dx.doi.org/10.1007/s002200050541
[2]	D’Andrea, A. and Kac, V.G. (1998) Structure Theory of Finite Conformal Algebras. Selecta Mathematica, 4, 377-418.
[3]	Kac, V.G. (1977) Lie Superalgebras. Advances in Mathematics, 26, 8-96. http://dx.doi.org/10.1016/0001-8708(77)90017-2
[4]	Kac, V.G. (1998) Vertex Algebras for Beginners. 2nd Edition, American Mathematical Society, Providence. http://dx.doi.org/10.1090/ulect/010
[5]	Kac, V.G. (1997) The Idea of Locality. In: Doebner, H.-D., et al., Eds., Physical Applications and Mathematical Aspects of Geometry, Groups and Algebras, World Scientific, Singapore, 16-32.
[6]	Kac, V.G. Formal Distribution Algebras and Conformal Algebras. XII-th International Congress in Mathematical Physics (ICMP’97), Brisbane, 8 April 1999, 80-97.
[7]	Yuan, L.M. (2014) Hom Gel’fand-Dorfman Bialgebras and Hom-Lie Conformal Algebra. Journal of Mathematical Physics, 55, Article ID: 043507. http://dx.doi.org/10.1063/1.4870870
[8]	Yuan, L.M. (2016) A Lie Conformal Algebra of Block Type. arXiv, No. 1601.07388.
[9]	Borcherds, R.E. (1986) Vertex Algebras, Kac-Moody Algebras, and the Monster. Proceedings of the National Academy of Sciences of the United States of America, 83, 3068-3071. http://dx.doi.org/10.1073/pnas.83.10.3068
[10]	Bakalov, B., D’Andrea, A. and Kac, V.G. (2001) Theory of Finite Pseudoalgebras. Advances in Mathematics, 162, 1-140. http://dx.doi.org/10.1006/aima.2001.1993
[11]	Bakalov, B., D’Andrea, A. and Kac, V.G. (2006) Irreducible Modules over Finite Simple Lie Pseudoalgebras. I. Primitive Pseudoalgebras of Type W and S. Advances in Mathematics, 204, 278-346. http://dx.doi.org/10.1016/j.aim.2005.07.003
[12]	Bakalov, B., D'Andrea, A. and Kac, V.G. (2013) Irreducible Modules over Finite Simple Lie Pseudoalgebras II. Primitive Pseudoalgebras of Type K. Advances in Mathematics, 232, 188-237. http://dx.doi.org/10.1016/j.aim.2012.09.012
[13]	Boyallian, C. and Liberati, J.I. (2012) On Pseudo-Bialgebras. Journal of Algebra, 372, 1-34. http://dx.doi.org/10.1016/j.jalgebra.2012.08.009
[14]	Sun, Q.X. (2012) Generalization of H-Pseudoalgebraic Structures. Journal of Mathematical Physics, 53, Article ID: 012105. http://dx.doi.org/10.1063/1.3665708
[15]	Hartwig, J., Larsson, D. and Silvestrov, S. (2006) Deformations of Lie Algebras Using σ-Derivations. Journal of Algebra, 295, 314-361. http://dx.doi.org/10.1016/j.jalgebra.2005.07.036
[16]	Larsson, D. and Silvestrov, S. (2007) Quasi-Hom-Lie Algebras, Central Extensions and 2-Cocycle-Like Identities. Journal of Algebra, 288, 321-344. http://dx.doi.org/10.1016/j.jalgebra.2005.02.032
[17]	Yau, D. (2009) Hom-Algebras and Homology. Journal of Lie Theory, 19, 409-421.
[18]	Yau, D. (2008) Enveloping Algebras of Hom-Lie Algebras. Journal of Generalized Lie Theory and Applications, 2, 95-108.
[19]	Yau, D. (2010) Hom-Bialgebras and Comodule Algebras. International Electronic Journal of Algebra, 8, 45-64.
[20]	Cheng, Y.S. and Yang, H.Y. (2010) Low-Dimensional Cohomology of q-deformed Heisenberg-Virasoro Algebra of Hom-Type. Frontiers of Mathematics in China, 5, 607-622. http://dx.doi.org/10.1007/s11464-010-0063-z
[21]	Sheng, Y.H. (2012) Representations of Hom-Lie Algebras. Algebras and Representation Theory, 15, 1081-1098. http://dx.doi.org/10.1007/s10468-011-9280-8
[22]	Sheng, Y.H. and Bai, C.M. (2014) A New Approach to Hom-Lie Bialgebras. Journal of Algebra, 399, 232-250. http://dx.doi.org/10.1016/j.jalgebra.2013.08.046
[23]	Yuan, L.M. (2012) Hom-Lie Color Algebra Structures. Communications in Algebra, 40, 575-592. http://dx.doi.org/10.1080/00927872.2010.533726
[24]	Makhlouf, A. and Silvestrov, S. (2008) Hom-Algebra Structures. Journal of Generalized Lie Theory and Applications, 3, 51-64. http://dx.doi.org/10.4303/jglta/S070206
[25]	Makhlouf, A. and Silvestrov, S. (2009) Hom-Lie Admissible Hom-Coalgebras and Hom-Hopf Algebras. In: Silvestrov, S., Paal, E., Abramov, V. and Stolin, A., Eds., Generalized Lie Theory in Mathematics, Physics and Beyond, Springer-Verlag, Berlin, 189-206.
[26]	Makhlouf, A. and Silvestrov, S. (2010) Hom-Algebras and Hom-Coalgebras. Journal of Algebra and Its Applications, 9, 553-589. http://dx.doi.org/10.1142/S0219498810004117
[27]	Caenepeel, S. and Goyvaerts, I. (2012) Monoidal Hom-Hopf Algebras. Communications in Algebra, 40, 1933-1950.
[28]	Chen, Y.Y., Wang, Z.W. and Zhang, L.Y. (2013) The FRT-Type Theorem for the Hom-Long Equation. Communications in Algebra, 41, 3931-3948. http://dx.doi.org/10.1080/00927872.2013.781614
[29]	Chen, Y.Y., Wang, Z.W. and Zhang, L.Y. (2014) Quasitriangular Hom-Hopf Algebras. Colloquium Mathematicum, 137, 67-88. http://dx.doi.org/10.4064/cm137-1-5
[30]	Gohr, A. (2010) On Hom-Algebras with Surjective Twisting. Journal of Algebra, 324, 1483-1491. http://dx.doi.org/10.1016/j.jalgebra.2010.05.003
[31]	You, M.M. and Wang, S.H. (2014) Constructing New Braided T-Categories over Monoidal Hom-Hopf Algebras. Journal of Mathematical Physics, 55, 111701. http://dx.doi.org/10.1063/1.4900824
[32]	Zhang, X.H. and Wang, S.H. (2015) Weak Hom-Hopf Algebras and Their (Co)Representations. Journal of Geometry and Physics, 94, 50-71. http://dx.doi.org/10.1016/j.geomphys.2014.11.014
[33]	Ammar, F. and Makhlouf, A. (2010) Hom-Lie Superalgebras and Hom-Lie Admissible Superalgebras. Journal of Algebra, 324, 1513-1528. http://dx.doi.org/10.1016/j.jalgebra.2010.06.014
[34]	Scheunert, M. and Zhang, R.B. (1998) Cohomology of Lie Superalgebras and Their Generalizations. Journal of Mathematical Physics, 39, 5024-5061. http://dx.doi.org/10.1063/1.532508
[35]	Sweedler, M.E. (1969) Hopf Algebras. Mathematics Lecture Notes Series. Benjamin, New York.
[36]	Bahturin, Y., Mikhalev, D., Zaicev, M. and Petrogradsky, V. (1992) Infinite Dimensional Lie Superalgebras. Walter de Gruyter Publisher, Berlin. http://dx.doi.org/10.1515/9783110851205
[37]	Kassel, C. (1995) Quantum Groups. Graduate Texts in Mathematics. Springer-Verlag, New York. http://dx.doi.org/10.1007/978-1-4612-0783-2