Non Linear Electrodynamics Contributing to a Minimum Vacuum Energy (“Cosmological Constant”) Allowed in Early Universe Cosmology ()
Received 4 November 2015; accepted 28 December 2015; published 31 December 2015

1. Introduction
We first of all cite that there exists formalism of Berry [1] as to the formation of a shortest time step consistent to FRW space-time metrics. Then we will compare the results to the derived minimum time step derivation which is consistent with Non linear Electrodynamics NLED. In doing so we will isolate what is a range of values for the vacuum energy, and “cosmological constant” dependent upon Nonlinear Electrodynamics NLED [2] inputs
Berry’s results [1] are that for a standard flat space FRW cosmology, with positive cosmological “constant”
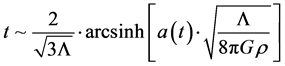 (1)
(1)
We will compare this answer with a minimum time step consistent with Non linear Electrodynamics NLED and use it to obtain a Non linear Electrodynamics NLED bound on the 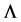 cosmological constant term.
cosmological constant term.
2. First the Non Linear Electrodynamics NLED Inputs into a Minimum Time Step
If we consider the role of an electromagnetic charge, as freely given in this presentation, we should also look at a derivation of what Zel’dovich [3] gave as far as charge and anti charge particles, in an applied E and M field could yield, which would be of the form (when  is an energy expression, and E an applied electric field of the value of)
is an energy expression, and E an applied electric field of the value of)
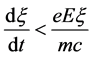 (2)
(2)
Again to generalize this Equation (2), we consider if the electric field is such that the commensurate bulk charge will have the following relationship to the given electromagnetic charge, to read as, if when 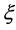 is an energy expression, with in Equation (3) below
is an energy expression, with in Equation (3) below
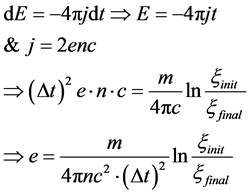 . (3)
. (3)
Picking the time variation as given by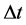 , the number of charges as given by n, and
, the number of charges as given by n, and 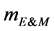 the mass of a hypothetical “magnetic” charge as, derived by the author to read as
the mass of a hypothetical “magnetic” charge as, derived by the author to read as
 (4)
(4)
Equation (3) has a deeper meaning. That that not only is there a net “magnetic” monopole charge, as given in Equation (4), that there is a minimum non zero “energy density” of the electric and magnetic field (E and M), as given by either 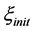 or
or 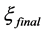 for an emergent “magnetic monopole” charge from an initial space-time configuration. This energy density value will lead to, to first order a minimum upper time step which we will characterize as [4]
for an emergent “magnetic monopole” charge from an initial space-time configuration. This energy density value will lead to, to first order a minimum upper time step which we will characterize as [4]
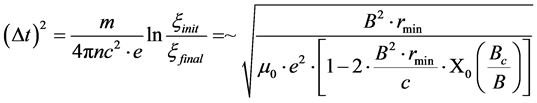 (5)
(5)
In doing so we will characterize the values to be set for Equation (4) as follows. i.e.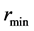 , n, B, and energy densities as given by
, n, B, and energy densities as given by 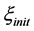 and
and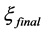 . These values come from the work of the author as interpreting [2] as well as applying the results for a minimum radial density which are implied by the minimum scale value for the initial
. These values come from the work of the author as interpreting [2] as well as applying the results for a minimum radial density which are implied by the minimum scale value for the initial
“radii” of the universe due to electro dynamics given in [3] , and of course the function ![]() given in [2] .
given in [2] .
The next section will give inputs into these values and will be used to show how non linear electrodynamics may -influence an upper bound choice for the minimum time step which may arise in the start of the evolution of the universe
2.1. Examining Inputs for rmin, n, B, and Energy Densities as given by xinit and xfinal
In this section we will lay out arguments given in [2] to their logical conclusion as well as build upon several of the procedures given in [2] [3] to fill in these quantities, so as to give an upper bound to the minimum time step as referenced in Equation (5). To do so it would be useful to look at the formalism of Zeldovich [2] very closely, and if that is done, we can assert that the nucleation life-time for an electron-positron pair is give by setting ![]() with
with ![]() defined as below
defined as below
![]() (6)
(6)
Then the numerical density of the electron-positron pair may be given as
![]() (7)
(7)
We will next begin to analyze what should be for ![]() and
and![]() . Which will in turn lead to the ‘net’ electric field, i.e. the net “E and M” field is, in reality a consequence of work in [2] -[4] which gives a minimum figure as to non linear electrodynamics and its consequence to a non zero initial radii of the universe. We go into this detail after giving order of magnitude estimates as to a minimum radii for the start of inflationary expansion.
. Which will in turn lead to the ‘net’ electric field, i.e. the net “E and M” field is, in reality a consequence of work in [2] -[4] which gives a minimum figure as to non linear electrodynamics and its consequence to a non zero initial radii of the universe. We go into this detail after giving order of magnitude estimates as to a minimum radii for the start of inflationary expansion.
2.1.1. What Are Working Values for xinit and xfinal as Well as Equation (6) n Value?
To engage on this, we use the Zeldovich [2] value for the net frequency which is given as
![]() (8)
(8)
We assume that the net frequency remains in Initial nucleation invariant, but that the initial and final volumes change, by an amount we will quantify next, i.e. start with an initial to final radii of
![]() (9)
(9)
Then
![]() (10)
(10)
This is the simplest interpretation of the consequence of varying energy density. Now for background to confirm it:
Making use of [2] [3] , we assert that the minimum radii of Equation (8) is consistent with predictions of [3] [4] , i.e. we are looking at what happens if an electron moves at a velocity of v, with
![]() (11)
(11)
Implying if c is the speed of light, and![]() , then the magnitude of the electric field should be given by
, then the magnitude of the electric field should be given by
![]() (12)
(12)
i.e. in [2] we look at a generalized density.
![]() (13)
(13)
This has a positive value only if input (E and M?) frequency ![]() is such that.
is such that.
![]() (14)
(14)
In this situation we will be setting![]() . What we are asserting is, that the very process of an existent E and M field, also, sets a non zero initial radii to the universe, i.e. in [3] there exists a scaled parameter
. What we are asserting is, that the very process of an existent E and M field, also, sets a non zero initial radii to the universe, i.e. in [3] there exists a scaled parameter![]() , and a parameter
, and a parameter ![]() which is paired with
which is paired with
![]() (15)
(15)
![]() (16)
(16)
Then if, initially, Equation (15) is large, due to a very large initial vacuum energy parameter ![]() the time, given in Equation (53) of [2] is such that we can write, most likely, that whenever one sees the coefficient like the magnetic field, with the small 0 coefficient, for large values of
the time, given in Equation (53) of [2] is such that we can write, most likely, that whenever one sees the coefficient like the magnetic field, with the small 0 coefficient, for large values of![]() , this should be the initial coefficient at the beginning of space-time which helps us make sense of the non zero but tiny minimum scale factor
, this should be the initial coefficient at the beginning of space-time which helps us make sense of the non zero but tiny minimum scale factor
![]() (17)
(17)
The above scale factor should be such that the value of Eq. (17) should be in its smallest, i.e. 480,000 times proportionately larger than a Planck length of![]() , i.e. scale
, i.e. scale ![]()
Then, we will have that
![]() (18)
(18)
![]() (19)
(19)
Equation (18) puts a strong constraint upon the frequency and magnetic field strength, whereas Equation (12) gives a strong set of values as to allowed E, so as to have, then
![]() (20)
(20)
This value for the square of the electric field should be then put into obtaining
![]() (21)
(21)
The numerical “density” of electron-positron pairs drops as when the frequency ![]() rises. Which is counter intuitive, but it meshes well with
rises. Which is counter intuitive, but it meshes well with
![]() (22)
(22)
i.e. for low frequency, we have a collapse to the Planck time frequency value, whereas, the minimum time step rises as frequency ![]() rises. Furthermore keep in mind that this result holds even if Equation (23) is formed in a way independent of a changing vacuum energy, as given by [2]
rises. Furthermore keep in mind that this result holds even if Equation (23) is formed in a way independent of a changing vacuum energy, as given by [2]
![]() (23)
(23)
Whereas this may tie into a massive graviton mass as given by the author as spin off of massive gravitons given in [5]
![]() (24)
(24)
i.e. the time step is then independent upon elementary arguments as to massive graviton mass. Furthermore, even if [6]
![]() (25)
(25)
And there is a relationship between Equation (24) and Equation (22), as well as a density functional which may relate to initially scaled mass![]() , that due to a very weak linkage between a density functional and
, that due to a very weak linkage between a density functional and![]() , and Equation (20) that there is no clear linkage between Equation (21) and Equation (20), which is also counter intuitive We will next then compare Equation (21) with Berry’s formalism [1] based on the FRW metric
, and Equation (20) that there is no clear linkage between Equation (21) and Equation (20), which is also counter intuitive We will next then compare Equation (21) with Berry’s formalism [1] based on the FRW metric
3. Minimum Time According to the FRW Metric Allows for a NLED Bound to L
The easiest case to consider is, if the ![]() is not overly large, and the initial scale factor
is not overly large, and the initial scale factor ![]() is small. Then we have
is small. Then we have
![]() (26)
(26)
Then we are looking at
![]() (27)
(27)
Here, ![]() is very small, but we are also assuming an ultra low
is very small, but we are also assuming an ultra low ![]() and
and![]() , and small m. The net effect is for a small positive
, and small m. The net effect is for a small positive ![]() as one is observing. This is different from the work done with String theory, as given in [7] below, which will be brought up in the conclusion.
as one is observing. This is different from the work done with String theory, as given in [7] below, which will be brought up in the conclusion.
![]() (28)
(28)
We will next then discuss how our work is connected to what Corda has brought up in work which is to discriminate between different cosmological models. This section is copied from the paper to be published [8] by the author and is reproduced here, due to its relevance.
4. Re-Examining Relic Gravitational Wave Models as to What Relic Gravitational Waves Could Tell Us about the Origins of the Early Universe. As Given in an Earlier Paper by the Author
Quoting from [8] we write the following. We reproduce this, because of the centrality of Equation (27) which is basic. It is very noticeable that in [9] we have that the following quote is particularly relevant to consider, in lieu of our results
“Thus, if advanced projects on the detection of GWs will improve their sensitivity allowing to perform a GWs astronomy (this is due because signals from GWs are quite weak) [1] , one will only have to look the interferometer response functions to understand if General Relativity is the definitive theory of gravity. In fact, if only the two response functions (2) and (19) will be present, we will conclude that General Relativity is definitive. If the response function (22) will be present too, we will conclude that massless Scalar- Tensor Gravity is the correct theory of gravitation. Finally, if a longitudinal response function will be present, i.e. Equation (25) for a wave propagating parallel to one interferometer arm, or its generalization to angular dependences, we will learn that the correct theory of gravity will be massive Scalar-Tensor Gravity which is equivalent to f(R) theories. In any case, such response functions will represent the definitive test for General Relativity. This is because General Relativity is the only gravity theory which admits only the two response functions (2) and (19) [2] [6] . Such response functions correspond to the two “canonical” polarizations h+ and h×. Thus, if a third polarization will be present, a third response function will be detected by GWs interferometers and this fact will rule out General Relativity like the definitive theory of gravity”.
We argue that a third polarization in Gravitational waves from the early universe may be detected, if there is proof positive that in the pre Planckian regime that the Corda conjecture [9] as given below, namely if the following analysis is part of our take on relic gravitational waves, is supported by the kinetic energy being larger than the potential energy, namely what if
“The case of massless Scalar-Tensor Gravity has been discussed in [2] [10] with a ‘bouncing photons analysis’ similar to the previous one. In this case, the line-element in the TT gauge can be extended with one more polarization, labelled with Φ (t + z), i.e. …”
This ends our recap of the section given in [8] which we think is important.
What we are arguing for is that the choice of the vacuum energy as given by Equation (27) may give conclusive proof as to satisfy the Corda conjecture and his supposition as to the existence of an additional polarization [9] . We will, in the future try to extend our results so as to determine if Equation (27) either falsifies or supports the existence of a 3rd polarization. Which will be a way to determine the final disposition of GR as THE theory of Cosmology, or open up the possibility of alternate theories. It is an issue which we think will require extreme diligence. While ending our query as to the possible existence of a third polarization we should mention what would be the supreme benefit of our upcoming analysis of Equation (27), namely how to avoid the conflating of dust, with gravitational waves, i.e. the tragic Bicep 2 mistake [10] -[13] .
5. How to Avoid the Bicep 2 Fiasco
The main agenda would be in utilization of Equation (27) to help nail down a range of admissible frequencies which would be to avoid [10] -[13] conflating the frequencies of collected Gravitational wave signals from relic cosmological conditions (or would be signals) with those connected with Dust generated Gravitational wave signals, especially from dust conflated with Galaxy formation in the early universe. More than anything else, we need to find, likely narrow (?). Frequency ranges, which would be commensurate with Equation (27), and to use advanced detector technology. Of course such a search would be hard. But it also would be a way, with due diligence as to answer questions raised by the Author in [14] . In doing so, the relative flatness of the early universe and its departure from curved space conditions would be a great way to answer the suppositions raised in [8] [9] as well.
6. Conclusions
The previously done work by the author as to graviton production invoking nonlinear electrodynamics in cosmology was re-introduced for the purpose as to density functions which are used to create an upper bound to the largest initial time step, in cosmological evolution. Counter intuitively, Equation (22) has no connection as to the scaled value of a vacuum energy as given in Equation (23), which suggests that when frequency rises, as may be connected to alternative values of cosmology, that different processes as to graviton production, as exemplified by Equation (25) still keep a sharp independence as to initial time size as we state it for Equation (22). Keep in mind, that what is being attempted is to upgrade work represented by Maggiore [5] , in what may become gravitational astronomy, once falsifiable experimental procedures are agreed upon for vetting a minimal time step. In a future article, the author intends to represent the formation of a minimum time step as a classical back drop for the formation of quantum gravity, i.e. the minimum time step will be a pre cursor to the development of quantum gravity (formation of massive gravitons).
Equation (27) and Equation (28) in themselves argue for an NLED influenced cosmological “vacuum energy”, i.e. what we observe, initially is that the above, using ![]() and Equation (28) that the value of
and Equation (28) that the value of ![]() would be greater than the present value of the cosmological constant, perhaps by 1010 - 1020, arguing that some form of quintessence is argued for. But this value of Equation (27) is far lower than the 10120 overshoot, obtained by traditional QFT methods [7] . It neatly allows for an investigation as to [14] flatness issues as well as gives credence to what is raised in [8] [9] as far as the existence, of an additional GW polarization.
would be greater than the present value of the cosmological constant, perhaps by 1010 - 1020, arguing that some form of quintessence is argued for. But this value of Equation (27) is far lower than the 10120 overshoot, obtained by traditional QFT methods [7] . It neatly allows for an investigation as to [14] flatness issues as well as gives credence to what is raised in [8] [9] as far as the existence, of an additional GW polarization.
We wish to point to an earlier paper by Beckwith, and Glinka [15] which has some of the same formalism, but which is geared toward proving inputs from a multiverse. The document so tendered now, has little of the same orientation, plus the emphasis in this paper is to vacuum energy due to conditions in the present universe, without speculation as to multiverses. In addition, [15] makes claims as to conflating gravitons with dark energy. Such speculation is beyond the scope of the document which has been submitted and which has to be proven, experimentally.
We wish to confirm, experimentally, what has been brought up as to vacuum energy, and the critical detail of stating, as done in our document that ![]() would be greater than the present value of the cosmological constant, perhaps by 1010 - 1020, arguing that some form of quintessence is argued for. After this is confirmed, speculations as to conflating gravitons, directly with dark energy may be investigated experimentally. Sadly, the author sees little hope of confirming if the multiverse construction of [15] has any chance of being experimentally tested, in the foreseeable future. Since our orientation, in this paper is the documentation of conditions which may be accessible to experimental vetting, we have to delay for the time being the interesting multiverse speculations of [15] as something which may be tested in the middle of this century, but not now.
would be greater than the present value of the cosmological constant, perhaps by 1010 - 1020, arguing that some form of quintessence is argued for. After this is confirmed, speculations as to conflating gravitons, directly with dark energy may be investigated experimentally. Sadly, the author sees little hope of confirming if the multiverse construction of [15] has any chance of being experimentally tested, in the foreseeable future. Since our orientation, in this paper is the documentation of conditions which may be accessible to experimental vetting, we have to delay for the time being the interesting multiverse speculations of [15] as something which may be tested in the middle of this century, but not now.
Acknowledgements
This work is supported in part by National Nature Science Foundation of China grant No. 11375279.