Received 6 November 2015; accepted 27 December 2015; published 30 December 2015

1. Introduction
The Choquet integral, introduced in [1] , of a nonnegative, 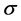 -measurable function f, based on a fuzzy measure
-measurable function f, based on a fuzzy measure 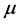 on measurable set A, is defined as
on measurable set A, is defined as
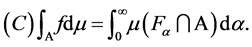
Ralescu and Adams [2] studied several equivalent definitions of fuzzy integral, while Pap [3] and Wang and Klir [4] provided an overview of fuzzy measure theory. The main properties of Choquet integral are monotonicity and positive homogeneity, see [3] [5] . Although the Choquet integral have the positive homogeneity,
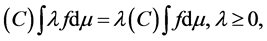
but it is generally nonlinear with respect to its integral due to the nonadditivity of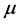 . That is, we may have
. That is, we may have
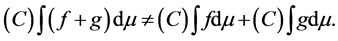
So, in some sense, the Choquet integral ia a kind of fuzzy integral. But, unlike the Sugeno integral [6] , the Choquet integral is a real generalization of the Lebesgue integral. In the special case when the monotone measure is 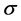 -additive, the Choquet integral coincides with the Lebesgue integral since the definition of the Choquet integral is just an equivalent definition the Lebesgue integral. The main fields for application of the Choquet integral are engineering, soft computing, social sciences, patter recognition and decision analysis [7] [8] .
-additive, the Choquet integral coincides with the Lebesgue integral since the definition of the Choquet integral is just an equivalent definition the Lebesgue integral. The main fields for application of the Choquet integral are engineering, soft computing, social sciences, patter recognition and decision analysis [7] [8] .
Integral inequalities are useful tools in several theoretical and applied fields. For more information on classical inequalities, we refer the reader to the distinguished monograph [9] [10] . Recently, Li and Sun [11] provided Hölder type inequalities for Sugeno integral. Some other classical inequalities have also been generalized to Sugeno integral by other authors (see, for example [12] [13] ). And Song have been proved the Berwald type inequality for extremal universal integrals based on 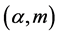 -concave function in [14] and Song also provided fuzzy algebra in triangular norm system in [15] . Recently Li and Song [16] proved Hermite-Hadamard type inequality for Sugeno integrals based on
-concave function in [14] and Song also provided fuzzy algebra in triangular norm system in [15] . Recently Li and Song [16] proved Hermite-Hadamard type inequality for Sugeno integrals based on 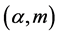 -convex function. Then Li and Song [17] proved Generalization of Liyapunov type inequality for pseudo-integrals. In [18] we proved Sandor’s type inequality for fuzzy integrals based on
-convex function. Then Li and Song [17] proved Generalization of Liyapunov type inequality for pseudo-integrals. In [18] we proved Sandor’s type inequality for fuzzy integrals based on  -Convex function.
-Convex function.
Section 2 consists of some preliminaries and notations about Choquet integral. In section 3, we prove the Hölder inequality for arbitrary fuzzy measure-based Choquet integral whenever any two of these integrated functions are comonotone. Then, we prove Minkowski inequalities and Lyapunov inequality for arbitrary fuzzy measure-based Choquet integral whenever any two of these integrated functions are comonotone. And including several examples. Finally, some conclusions are drawn.
2. Preliminaries
In this section we recall some basic definitions and previous results that will be used in the sequel.
As usual we denote by R the set of real numbers. Let X be a nonempty set, 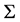 be a
be a 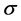 -algebra of subsets of X, and
-algebra of subsets of X, and 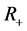 denote
denote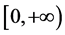 . Also, let
. Also, let 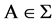 and f be a nonnegative measurable function on
and f be a nonnegative measurable function on![]() ,
, ![]() is a monotone measure.
is a monotone measure.
Definition 1. ( [11] ) A set function ![]() is called a fuzzy measure if the following properties are satisfied:
is called a fuzzy measure if the following properties are satisfied:
(FM1)![]() ;
;
(FM2) ![]() implies
implies![]() ;
;
(FM3)![]() , implies
, implies ![]()
(FM4)![]() , and
, and ![]() imply
imply ![]()
When ![]() is a fuzzy measure, then the triple
is a fuzzy measure, then the triple ![]() is called a fuzzy measure space.
is called a fuzzy measure space.
Definition 2. ( [4] ) The Choquet integral of a nonnegative measurable function f with respect to monotone measure ![]() on measurable set A, denoted by
on measurable set A, denoted by![]() , is defined by the formula
, is defined by the formula
![]()
where ![]() for
for![]() . When
. When![]() ,
, ![]() is usually written as
is usually written as![]() .
.
Since f in Definition 2 is measurable, we know that ![]() for
for ![]() and, therefore
and, therefore![]() , so
, so ![]() is well defined for all
is well defined for all![]() . Furthermore,
. Furthermore, ![]() is a class of sets that are nonincreasing with respect to
is a class of sets that are nonincreasing with respect to ![]() and so are sets in
and so are sets in![]() . Since monotone measure
. Since monotone measure ![]() is a nondecreasing set function, we know that
is a nondecreasing set function, we know that ![]() is a nondecreasing function of
is a nondecreasing function of ![]() and, therefore, the above Riemann integral makes sense. Thus, the Choquet integral of a nonnegative measurable function with respect to a monotone measure on a measurable set is well defined.
and, therefore, the above Riemann integral makes sense. Thus, the Choquet integral of a nonnegative measurable function with respect to a monotone measure on a measurable set is well defined.
The Choquet integral has some properties of the Lebesgue integral. These properties are listed in the following theorem.
Theorem 1. ( [4] ) Let f and g be nonnegative measurable functions on![]() . A and B be measurable sets, and a be a nonnegative real constant. Then,
. A and B be measurable sets, and a be a nonnegative real constant. Then,
1)![]() ;
;
2)![]() ;
;
3)![]() ;
;
4) If ![]() on A, then
on A, then![]() ;
;
5) If ![]() then,
then,![]() ;
;
6)![]() .
.
Unlike the Lebesgue integral, the Choquet integral is generally nonlinear with respect to its integrand due to the nonadditivity of![]() . That is, we may have
. That is, we may have
![]()
for some nonnegative measurable functions f and g. But when integrand f and g satisfying the properties of comonotone, then we have
![]() (1)
(1)
This is the properties of Choquet integral of comonotone additivity. Then we give the definition of two functions comonotonicity.
Definition 3. ( [11] ) Let X be a nonempty set, two functions ![]() are said to be comonotone, if for all
are said to be comonotone, if for all![]() , then
, then
![]()
Clearly, if f and g are comonotone, then for all nonnegative real numbers![]() , either
, either ![]() or
or![]() . Indeed, if this assertion does not hold, then there are
. Indeed, if this assertion does not hold, then there are ![]() and
and![]() . That is,
. That is, ![]() and
and![]() . And hence,
. And hence, ![]() , contradicting! Notice that constant function and any functions are comonotone, by (1) and Theorem 1 (2) we obtain,
, contradicting! Notice that constant function and any functions are comonotone, by (1) and Theorem 1 (2) we obtain,
![]()
3. Hölder Inequality for Choquet Integral
This section is devoted to providing Hölder inequality for Choquet integral, when there are three integrand and three weights. And these integrand satisfying the properties of comonotone additivity. Then we prove Hölder inequality for Choquet integral about a finite number of integrands and finite weights appears as its corollary.
In this paper, we suppose any two of these nonnegative measurable functions ![]() are comonotone, so we can easily obtained any two of f, g and h are comonotone.
are comonotone, so we can easily obtained any two of f, g and h are comonotone.
Theorem 2 (Hölder inequality). Let ![]() be a fuzzy measure space,
be a fuzzy measure space, ![]() , f, g and h be nonnegative
, f, g and h be nonnegative
measurable functions. When any two of f, g and h are comonotone, and![]() ,
,![]() . Then, the Hölder inequality
. Then, the Hölder inequality
![]() (2)
(2)
holds.
Proof. By Theorem 3.1 [19] the Hölder inequality about two nonnegative measurable functions and two weights
![]() (3)
(3)
holds. Let![]() , then
, then![]() ,
, ![]() When f and h are nonnegative measurable functions, then by
When f and h are nonnegative measurable functions, then by
the product of a finite number of measurable functions still can be measurable, we have fg is nonnegative measurable function. And fg and h are comonotone for any ![]() can be easily proved. Then, the inequality
can be easily proved. Then, the inequality
![]() (4)
(4)
holds. Let![]() ,
, ![]() , then
, then![]() . Then the inequality
. Then the inequality
![]() (5)
(5)
holds. Then, by the inequalities (4) and (5), we obtain
![]() (6)
(6)
This completes the proof.
Then, let us review examples illustrating the previous result.
Example 1. Let![]() ,
, ![]() ,
, ![]() ,
, ![]() for
for![]() . p = 6, q = 3 and r = 2.
. p = 6, q = 3 and r = 2. ![]() be
be
the class of all Borel sets in ![]() and
and ![]() for
for![]() , where m is the lebesgue measure. We know the
, where m is the lebesgue measure. We know the ![]() is a monotone measure on
is a monotone measure on ![]() -algebra
-algebra ![]() and f, g and h are nonnegative measurable functions on X, and any two of f, g and h are comonotone. According to Definition 2, the value of Choquet integral for fgh, f, g and h with respect to
and f, g and h are nonnegative measurable functions on X, and any two of f, g and h are comonotone. According to Definition 2, the value of Choquet integral for fgh, f, g and h with respect to ![]() are
are
![]()
In a similar manner, we calculate that
![]() ,
, ![]() , and
, and![]() .
.
By the inequality
![]()
Then, we obtain
![]() (7)
(7)
When the integrand ![]() of the integral cannot be expressed by an explicit algebraic expression of
of the integral cannot be expressed by an explicit algebraic expression of![]() , or the expression is too complex, the value of the Choquet integral has to be approximately calculated by using some numerical method (e.g., the Simpson method).
, or the expression is too complex, the value of the Choquet integral has to be approximately calculated by using some numerical method (e.g., the Simpson method).
Example 2. Let![]() ,
, ![]() ,
, ![]() and
and![]() ,
,![]() . And any two of f, g and h are comonotone, then
. And any two of f, g and h are comonotone, then
![]()
Then, we can calculate that
![]()
![]() and
and ![]()
So by the inequality
![]()
where ![]() is defined as in Example 1. Then, we obtain
is defined as in Example 1. Then, we obtain
![]() (8)
(8)
From the above two examples we can get, f, g and h be nonnegative measurable functions, when any two of f, g and h are comonotone, and![]() ,
,![]() . Then, the Hölder inequality holds.
. Then, the Hölder inequality holds.
Hölder inequality for Choquet integral about a finite number of integrands and finite weights appears in the following corollary.
Corollary 1. Let ![]() be a fuzzy measure space,
be a fuzzy measure space, ![]() ,
, ![]() and fn be nonnegative measurable
and fn be nonnegative measurable
functions. When any two of ![]() are comonotone, and
are comonotone, and![]() ,
, ![]() , then, the Hölder inequality for Choquet integral about a finite number of integrands and finite weights
, then, the Hölder inequality for Choquet integral about a finite number of integrands and finite weights
![]()
holds.
As the application of Hölder inequality for Choquet integral, we will prove Minkowski inequality. First, we prove the following lemma.
4. Minkowski Inequality for Choquet Integral
Lemma 1. Let f, g and ![]() When any two of f, g and h are comonotone, then any two of these functions
When any two of f, g and h are comonotone, then any two of these functions![]() ,
, ![]() and
and ![]() are comonotone, for any
are comonotone, for any![]() .
.
Proof. For any![]() , we first prove
, we first prove ![]() and
and ![]() are comonotone. According to Definition 3 [11] , this is equivalent to prove that
are comonotone. According to Definition 3 [11] , this is equivalent to prove that
![]() (9)
(9)
If![]() , then by any two of f, g and h are comonotone, we obtain
, then by any two of f, g and h are comonotone, we obtain![]() ,
,![]() . And by nonnegativity of f, g and h and
. And by nonnegativity of f, g and h and![]() , we get
, we get
![]()
![]()
Then, the inequality (9) holds.
The case that when![]() , the inequality (9) can be proved in a similar manner.
, the inequality (9) can be proved in a similar manner.
We have proved the inequality (9) holds, when![]() . In a same way, we prove the inequality (9) holds, when
. In a same way, we prove the inequality (9) holds, when![]() ,
,![]() . And the inequality (9) obviously holds, when
. And the inequality (9) obviously holds, when![]() ,
, ![]() and
and![]() .
.
So, we obtain the functions ![]() and
and ![]() are comonotone. In a similar manner, we get the the functions
are comonotone. In a similar manner, we get the the functions ![]() and
and ![]() are comonotone.
are comonotone.
As so far, we prove any two of these functions![]() ,
, ![]() and
and ![]() are comonotone.
are comonotone.
This completes the proof.
Then the Minkowski inequality for Choquet integral is given in the following theorem.
Theorem 3 (Minkowski inequality). Let ![]() be a fuzzy measure space and
be a fuzzy measure space and![]() , f, g and h:
, f, g and h: ![]() be measurable functions. When any two of f, g and h are comonotone, then the inequality
be measurable functions. When any two of f, g and h are comonotone, then the inequality
![]() (10)
(10)
holds for any![]() .
.
Proof. When![]() , by any two of f, g and h are comonotone, we get
, by any two of f, g and h are comonotone, we get
![]()
Obviously, the inequality (10) holds.
When![]() , there exists
, there exists![]() , such that
, such that ![]() (i.e.
(i.e.![]() ). By the Lemma 2 and Theorem 2, we obtain
). By the Lemma 2 and Theorem 2, we obtain
![]()
In the same method, we get
![]()
![]()
Hence,
![]()
![]() (11)
(11)
This completes the proof.
Example 3 Let![]() ,
, ![]() ,
, ![]() and
and![]() ,
, ![]() , when any two of f, g and h are comonotone. Where
, when any two of f, g and h are comonotone. Where ![]() is defined as in Example 1. Then
is defined as in Example 1. Then
![]()
In the same way,we calculate that
![]()
![]()
![]()
Then, we get
![]()
If there is a finite nonnegative measurable function, the Minkowski inequality for Choquet integral holds or not. First, we have to prove the following corollary.
Corollary 2. Let![]() . When any two of
. When any two of ![]() are comonotone, then any two of
are comonotone, then any two of
these functions ![]()
![]() are comonotone, for any
are comonotone, for any![]() .
.
Corollary 3. Let ![]() be a fuzzy measure space and
be a fuzzy measure space and![]() ,
, ![]() be measurable functions. When any two of
be measurable functions. When any two of ![]() are comonotone , then the inequality
are comonotone , then the inequality
![]() (12)
(12)
holds, for any![]() .
.
5. Lyapunov Inequality for Choquet Integral
Theorem 4 (Lyapunov inequality). Let ![]() be a fuzzy measure space and
be a fuzzy measure space and ![]() be a measurable set,
be a measurable set, ![]() be a measurable function. Let
be a measurable function. Let![]() ,
, ![]() , when
, when ![]() satisfies this
satisfies this
equality,![]() . Then the inequality
. Then the inequality
![]() (13)
(13)
holds.
Proof. Let![]() ,
, ![]() ,
, ![]() , then
, then![]() , for any
, for any![]() . By Theorem 2, we have
. By Theorem 2, we have
![]()
And by![]() , we get the inequality
, we get the inequality
![]() (14)
(14)
Remark 1. Let ![]() be a fuzzy measure space and
be a fuzzy measure space and ![]() be a measurable set, we have
be a measurable set, we have
![]()
for any![]() .
.
Corollary 4. Let ![]() be a fuzzy measure space,
be a fuzzy measure space, ![]() be a measurable set and
be a measurable set and ![]() be a measurable function. Let
be a measurable function. Let![]() ,
, ![]() , when
, when ![]() satisfies
satisfies
![]() , and
, and![]() . Then, we have the inequality
. Then, we have the inequality
![]() (15)
(15)
6. Conclusion
In this paper, we prove the Hölder inequalities for any arbitrary fuzzy measure based on Choquet integral whenever any two of these integrated functions f, g and h are comonotone. As its application, we also prove Minkowski inequality and Lyapunov inequality for Choquet integral. Moreover, we also obtain whenever any two of these integrated functions ![]() are comonotone, the Hölder inequality, Minkowski inequality and Lyapunov inequality hold for Choquet integral.
are comonotone, the Hölder inequality, Minkowski inequality and Lyapunov inequality hold for Choquet integral.
Acknowledgements
This work was supported by the National Natural Science Foundation of China (no. 51374199).
NOTES
![]()
*Corresponding author.