The Analysis of an SIRS Epidemic Model with Discrete Delay on Scale-Free Network ()
1. Introduction
Since the modelling of the seminal works on the scale-free network, in which the probability of  for any node with
for any node with 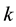 links to other nodes is distributed according to the power law
links to other nodes is distributed according to the power law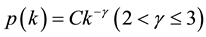 , suggested
, suggested
by Barabá and Albert [1] , it is well known that the real disease transmission networks exhibit scale-free properties (see for example [2] [3] ), and the spreading of epidemic disease on scale-free network has been studied by many researchers [4] - [21] .
Continuous time deterministic epidemic models are traditionally formulated as systems of ordinary differential equations. More realistic models should include some peat states of these systems, and ideally, a real system should be modeled by delay differential equation. Time delay plays an important role in propagation process of the epidemic, we can simulate the latent period of infectious diseases, the infections period of patients and the immunity period of recovery of the disease with time delay. Much attention has been given to the dynamical behaviors of the epidemic spreading model with time delay on homogeneous network [18] . However, up to now, compared with studies of the dynamical behaviors of the epidemic models with time delays on hetergeneous network, only a few attentions have been paid to them on heterogeneous networks. Recently, Liu and Xu presented a delay differential equation SEIRS epidemic model with discrete time delays which represent the latent period and the immune period [19] . Liu and Deng et al. discussed epidemic SIS model with discrete time delay which represents the infectious period [20] , they obtained the basic reproduction number and discussed the persistence of the disease. Wang and Wang et al. discussed an epidemic SIR model with discrete time delay which represented the latent period [21] . Motivated by these, in this paper, we will present a suitable epidemic SIRS model with discrete delay which represents the infectious period on scale-free network using functional differential equations to investigate the epidemic spreading.
The rest of this paper is organized as follows: The SIRS model on scale-free network with discrete delay is presented in Section 2. The basic reproductive number is given and dynamical behaviour of the system is analyzed in Section 3. Numerical simulations are given to demonstrate the main results in Section 4. Conclusion is finally drawn in Section 5.
2. The SIRS Model with Discrete Delay
Suppose that the size of the network is a constant N during the period of epidemic spreading, we also suppose that the degree of each degree is time invariant. Let ,
,  and
and  be the relative density of susceptible nodes, infected nodes and recovered nodes of connectivity k at time t, respectively. Obviously, the following normalization condition holds due to the fact that the number of total nodes with degree k is a constant
be the relative density of susceptible nodes, infected nodes and recovered nodes of connectivity k at time t, respectively. Obviously, the following normalization condition holds due to the fact that the number of total nodes with degree k is a constant 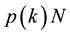 during the period of epidemic spreading.
during the period of epidemic spreading.
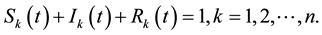
The dynamical equation for the density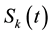 ,
,  and
and , at the mean-field level, satisfy the following system when
, at the mean-field level, satisfy the following system when :
:
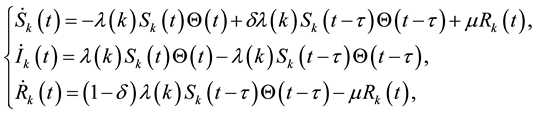 (1)
(1)
where 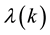 is the correlated (k-dependent) infection rate such as
is the correlated (k-dependent) infection rate such as  [5] [8] [20] ,
[5] [8] [20] , 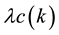 [6] and so on, and
[6] and so on, and ![]() represents the average infectious period. The term
represents the average infectious period. The term ![]() describes some infected nodes
describes some infected nodes
may become susceptible nodes because they are recovered and are not immunized, where ![]() is called incomplete cure rate, and consequently,
is called incomplete cure rate, and consequently, ![]() is cure rate. In reality, for example, some computer users find network virus and kill it after
is cure rate. In reality, for example, some computer users find network virus and kill it after ![]() due to its destruction of the user, but they do not take further action to immunize computer from the network virus, but the other users can get the technique to protect their computer and their computer cannot be infected again. The dynamics of n groups of SIRS subsystems are coupled through the function
due to its destruction of the user, but they do not take further action to immunize computer from the network virus, but the other users can get the technique to protect their computer and their computer cannot be infected again. The dynamics of n groups of SIRS subsystems are coupled through the function![]() , which represents the probability that any given link points to an infected site. Assume that the network has no degree correlations [4] , we have
, which represents the probability that any given link points to an infected site. Assume that the network has no degree correlations [4] , we have
![]() (2)
(2)
where ![]() stands for the average node degree, and
stands for the average node degree, and ![]() has many different forms, such as
has many different forms, such as ![]() in [4] [5] ,
in [4] [5] , ![]() in [7] ,
in [7] , ![]() ,
, ![]() in [8] , and
in [8] , and![]() ,
, ![]() in [9] and so on.
in [9] and so on.
The initial condition of system (1) is
![]()
where ![]() are nonnegative continuous on
are nonnegative continuous on![]() , and
, and ![]() and
and ![]() for
for![]() . C denote the Banach space
. C denote the Banach space ![]() with the norm
with the norm![]() , and
, and ![]() is Euclidean norm of
is Euclidean norm of![]() .
.
3. Dynamical Behaviors of the Model
Denote
![]()
![]() (3)
(3)
where ![]() in which
in which ![]() is a function.
is a function.
Obviously, the second equation of system (1) can be furthermore transformed into the following integral equation:
![]() (4)
(4)
Note that ![]() and
and ![]() can be replaced by
can be replaced by ![]() in the first equation of system (1). Thus we obtain the following equivalent system of system (1):
in the first equation of system (1). Thus we obtain the following equivalent system of system (1):
![]() (5)
(5)
Theorem 1. The system (5) has always a disease-free equilibrium![]() . The system (5) has a unique endemic equilibrium
. The system (5) has a unique endemic equilibrium ![]() when
when![]() .
.
Proof. Denote![]() ,
, ![]() and
and ![]() (some constants) and substitute them into (5), we have
(some constants) and substitute them into (5), we have
![]() (6)
(6)
where
![]() (7)
(7)
It yields that
![]() (8)
(8)
Substituting it into (7), we obtain the self-consistency equality
![]() (9)
(9)
Obviously, ![]() always satisfies (9), it follows that from (8) that the disease-free equilibrium
always satisfies (9), it follows that from (8) that the disease-free equilibrium ![]() of system (5) always exists. Note that
of system (5) always exists. Note that
![]()
and
![]()
Hence, if![]() , the Equation (9) has a unique positive solution, consequently, system (5) has a unique positive equilibrium
, the Equation (9) has a unique positive solution, consequently, system (5) has a unique positive equilibrium ![]() since (8) holds.
since (8) holds. ![]() is an unique endemic equilibrium.
is an unique endemic equilibrium.
Theorem 2. Consider the system (5), the following assertions hold.
(1) If![]() , the equilibrium
, the equilibrium ![]() of system (5) is globally attractive.
of system (5) is globally attractive.
(2) If![]() , the disease is uniformly persistent, i.e., there exists a positive constant
, the disease is uniformly persistent, i.e., there exists a positive constant ![]() such that
such that ![]() , and the equilibrium
, and the equilibrium ![]() of system (5) is unstable.
of system (5) is unstable.
Proof. First, According to the Equation (4), similar to the proof of Theorem 1 in [20] , we can obtain that the equilibrium ![]() of system (5) is globally attractive.
of system (5) is globally attractive.
Second, motivated by the work in [22] , we will prove that conclusion (2) in Theorem 2 holds step by step, i.e., we prove that the disease is uniformly persistent when![]() .
.
Step 1. We will prove that for any![]() , it is impossible that
, it is impossible that ![]() for
for![]() .
.
Since![]() , there exists a small enough
, there exists a small enough ![]() such that
such that
![]()
in which ![]()
Suppose ![]() for
for![]() , which implies
, which implies
![]()
for![]() . It follows from the first equation of system (5) that
. It follows from the first equation of system (5) that
![]() (10)
(10)
for![]() . Hence there exists a
. Hence there exists a ![]() such that
such that
![]() (11)
(11)
for![]() , and there a
, and there a ![]() such that
such that
![]() (12)
(12)
It follows from (2) and (12) that
![]() (13)
(13)
Set![]() . We claim that
. We claim that ![]() for
for![]() . If not, there exists a
. If not, there exists a ![]() such that
such that ![]() and
and ![]() for
for![]() . It follow that
. It follow that
![]()
which leads to![]() , contradicting
, contradicting![]() . This proves the claim.
. This proves the claim.
Choose a positive constant ![]() which satisfies
which satisfies![]() . We claim now that
. We claim now that ![]() for all
for all![]() . Note that
. Note that
![]()
If the claim is not valid, there exists a ![]() such that
such that ![]() and
and ![]() for
for![]() .
.
Thus
![]()
which leads to![]() , contradicting
, contradicting![]() . This proves the claim. By induction method, we conclude that
. This proves the claim. By induction method, we conclude that
![]()
for![]() . It follows that
. It follows that ![]() if t is sufficiently large, contradicting
if t is sufficiently large, contradicting![]() . So, for any
. So, for any![]() , it is impossible that
, it is impossible that ![]() for
for![]() .
.
Step 2. We will prove that there exists a positive constant ![]() such that
such that![]() .
.
Since it is impossible that ![]() for
for![]() . Hence, there are two cases to be considered for
. Hence, there are two cases to be considered for![]() . Hence, there are two cases to be considered for
. Hence, there are two cases to be considered for![]() .
.
Case 1: ![]() when t is sufficiently large.
when t is sufficiently large.
Case 2: ![]() is oscillates about
is oscillates about ![]() when t is sufficiently large.
when t is sufficiently large.
Suppose ![]() and
and ![]() for
for![]() , where
, where ![]() is sufficiently large such that
is sufficiently large such that ![]() holds. Consequently,
holds. Consequently, ![]() and
and ![]() for
for![]() .
. ![]() is uniformly continuous since the positive solutions of system (4) are bounded. Hence, there is a
is uniformly continuous since the positive solutions of system (4) are bounded. Hence, there is a ![]() (independent of the choice of
(independent of the choice of![]() ) such that
) such that ![]() for
for![]() . If
. If![]() , there is nothing to prove. If
, there is nothing to prove. If![]() , for
, for![]() , we have from (15) that
, we have from (15) that
![]()
Let us define![]() , then
, then ![]() for
for![]() . If
. If![]() , by similar method in step 1, we can obtain that
, by similar method in step 1, we can obtain that ![]() for
for![]() . Thus, for case 2,
. Thus, for case 2, ![]() when t is sufficiently large, and
when t is sufficiently large, and ![]() where
where![]() .
.
Hence, ![]() when t is sufficiently large, where
when t is sufficiently large, where![]() , consequently,
, consequently, ![]() , and the disease is uniformly persistent.
, and the disease is uniformly persistent.
At last, since ![]() when
when![]() , the equilibrium
, the equilibrium ![]() is unstable when
is unstable when![]() . This completes the proof of Theorem 2.
. This completes the proof of Theorem 2.
The basic reproductive number for system (4) is![]() . If
. If![]() , the disease will disappear due to the global attractivity of
, the disease will disappear due to the global attractivity of![]() . If
. If![]() , the disease will always exists due to
, the disease will always exists due to![]() .
.
Remark. When ![]() system (1) reduce to system (4) in [20] , and the results still holds.
system (1) reduce to system (4) in [20] , and the results still holds.
4. Numerical Simulations
The basic reproductive number for system (1) is
![]() (14)
(14)
Note that an epidemic always occurs on a finite networks in the real world, the maximum connectivity n of any node is related to the network age, which is measured as the number of nodes N [8] [9] :
![]() (15)
(15)
where m is the minimum connectivity of the network. It follows from (14) and (15) that![]() , which depends both N and
, which depends both N and![]() , can be approximately computed.
, can be approximately computed.
Now we present the results of numerical simulations by using MATLAB 7.0 to support the results obtained in previous sections. Since the equilibria were obtained from system (5), the simulations are based on system (5)
and a scale-free network in which the degree distribution is![]() , and C satisfies
, and C satisfies![]() . Assume the network is a finite network with
. Assume the network is a finite network with ![]() and
and ![]() are suitable assumptions. Let
are suitable assumptions. Let![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() in which
in which![]() ,
, ![]() ,
, ![]() , and
, and![]() . Figures 1-3 show the dynamic behaviors of system (5).
. Figures 1-3 show the dynamic behaviors of system (5).
![]()
Figure 1. The time series of system (5) with ![]() and
and![]() .
.
![]()
Figure 2. The time series of system (5) with ![]() and
and![]() .
.
![]()
Figure 3. The time series of system (5) with ![]() and
and![]()
From the dynamical behaviors of the SIRS model (5) shown in Figure l and Figure 2, it can be seen that if![]() , the infection eventually disappears. If
, the infection eventually disappears. If![]() , the relative density of infected nodes will tend to a positive constant, and the infection will always exist. The numerical results are consistent with the theoretical results.
, the relative density of infected nodes will tend to a positive constant, and the infection will always exist. The numerical results are consistent with the theoretical results.
According to![]() , if
, if![]() , the equilibrium
, the equilibrium ![]() is globally attractive and the disease eventually disappear. However,
is globally attractive and the disease eventually disappear. However, ![]() may hold as
may hold as ![]() increases due to the fact
increases due to the fact ![]() is proportional to
is proportional to![]() , that is to say, the equilibrium
, that is to say, the equilibrium ![]() may lose its stability when the average infection period of disease
may lose its stability when the average infection period of disease ![]() is large enough and the infection will always exist (shown in Figure 3).
is large enough and the infection will always exist (shown in Figure 3).
5. Conclusion
An SIRS model with discrete delay has been proposed for investigating the dynamical behaviors of the epidemics on scale-free networks. Through mathematical analysis, we obtained the basic reproduction number![]() . The main results reveal that when
. The main results reveal that when![]() , the disease-free equilibrium is globally attractive, while
, the disease-free equilibrium is globally attractive, while![]() , the disease-free equilibrium is unstable and the disease is uniformly persistent. In addition, numerical simulations show that the endemic equilibrium
, the disease-free equilibrium is unstable and the disease is uniformly persistent. In addition, numerical simulations show that the endemic equilibrium ![]() is globally asymptotically stable when
is globally asymptotically stable when ![]() (as shown in Figure 2). We would like to mention here that it is interesting but challenging to discuss the stability of equilibrium
(as shown in Figure 2). We would like to mention here that it is interesting but challenging to discuss the stability of equilibrium![]() , we leave this for our future work.
, we leave this for our future work.