1. Introduction
In this paper “On quasi-separative ‘semigroup’s’”, Krasilnikova Yu. I. and Novikov B.V. have studied congruences induced by certain relations on a “semigroup”. They further showed that if the “semigroup” is quasi-se- parative then the induced congruence is a semilattice congruence. In this paper we continue the study of these relations and the induced congruences. In theorem 2, we have proved that the family of all relatios  which satisfy the conditions from (1) to (3) in Def. 1 of this paper is a complete lattice. In theorem 3, we have also obtained that the family of all congruences which are induced by the relations in
which satisfy the conditions from (1) to (3) in Def. 1 of this paper is a complete lattice. In theorem 3, we have also obtained that the family of all congruences which are induced by the relations in  is a complete lattice. If S is a quasi-separative and regular “semigroup” then the necessary and sufficient condition for
is a complete lattice. If S is a quasi-separative and regular “semigroup” then the necessary and sufficient condition for 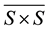 to be the smallest semilattice congruence which is denoted by h (throughout this chapter) is obtained, from which as a corollary that if S is a commutative regular “semigroup” then the congruence induced by the S × S is the smallest semilattice congruence [1] . The authors have remarked that a semilattice of weakly cancellative “semigroup’s” is weakly balanced, it is not known that “whether semilattice of weakly cancellative ‘semigroup’s’ [2] is weakly balanced”, show that the result is not true. It is also observed that every semilattice of weakly cancellative “semigroup’s”, need not be weakly balanced, for this an example is obtained.
to be the smallest semilattice congruence which is denoted by h (throughout this chapter) is obtained, from which as a corollary that if S is a commutative regular “semigroup” then the congruence induced by the S × S is the smallest semilattice congruence [1] . The authors have remarked that a semilattice of weakly cancellative “semigroup’s” is weakly balanced, it is not known that “whether semilattice of weakly cancellative ‘semigroup’s’ [2] is weakly balanced”, show that the result is not true. It is also observed that every semilattice of weakly cancellative “semigroup’s”, need not be weakly balanced, for this an example is obtained.
2. Main Content
The following definition is due to Krasilnikova Yu. I. and Novikov B. V. (see [3] ).
Def 1: Let S be a “semigroup” and Ω be a relation on S satisfying conditions.
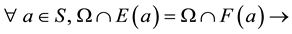 (1)
(1)
 (2)
(2)
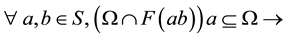 (3)
(3)
where 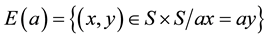 and
and 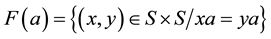
Define a relation  on S corresponding to Ω by
on S corresponding to Ω by 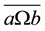 if and only if
if and only if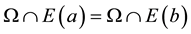 . It is also equivalent to
. It is also equivalent to  if and only if
if and only if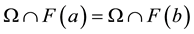 , this relation
, this relation  is a congruence on S.
is a congruence on S.
Lemma 2: Let  be the family of all relations on S which satisfy the conditions from (1) to (3) then
be the family of all relations on S which satisfy the conditions from (1) to (3) then ![]() is a complete lattice.
is a complete lattice.
Proof: Let![]() . Then clearly
. Then clearly![]() . Let
. Let ![]() be a subset of
be a subset of![]() . Then both
. Then both ![]() and
and ![]() are in
are in![]() . Therefore
. Therefore ![]() is a complete lattice.
is a complete lattice.
Lemma 3: Let ![]() then
then ![]() is a complete lattice.
is a complete lattice.
Proof: Since ![]()
![]() . Therefore
. Therefore![]() . Also
. Also ![]() is the greatest element in
is the greatest element in![]() . Let
. Let ![]() be a subset of. Then
be a subset of. Then ![]() because
because![]() .
.
Theorem 4: Let S be quasi-separative and regular “semigroup”. Then ![]() if and only if for any
if and only if for any![]() ,
,![]() .
.
Proof: Suppose S is quasi-separative and regular and Suppose![]() . Then
. Then![]() , so that it satisfies (1). Therefore
, so that it satisfies (1). Therefore ![]() if and only if
if and only if ![]() for any
for any![]() . Conversely suppose that
. Conversely suppose that![]() . Then
. Then![]() . Since S is quasi-separative
. Since S is quasi-separative ![]() is semilattice congruence and hence
is semilattice congruence and hence
![]() . Let
. Let ![]() so that
so that ![]() and hence
and hence ![]() if and ony if
if and ony if ![]() for any
for any![]() , since S is regular there exists
, since S is regular there exists ![]() such that
such that ![]() and
and![]() . Put
. Put ![]() and
and ![]() then we have
then we have ![]() and
and ![]() so that
so that ![]() hence
hence![]() . Therefore
. Therefore ![]() and similarly we have
and similarly we have![]() . Therefore if F is any filter in S then
. Therefore if F is any filter in S then ![]() if and only if
if and only if ![]() so that
so that ![]() and hence
and hence![]() .
.
Corollary 5: If S is a commutative regular “semigroup” then![]() .
.
Corollary 6: If S is a completely regular and ![]() and if
and if ![]() , for some
, for some ![]() then
then![]() .
.
The following is an example of a completely regular “semigroup” in which![]() .
.
Example 7: Let S be a left zero “semigroup” with at least two elements. If ![]() then
then ![]() then
then![]() , which is a contradiction and hence
, which is a contradiction and hence![]() .
.
Theorem 8: In a band S, ![]() if and only if S is a semilattice.
if and only if S is a semilattice.
It is natural to ask whether every semilattice congruence on “semigroup” is of the form ![]() for some
for some![]() .
.
The following example shows that it is not true.
Example 9: Consider the non modular lattice ![]() in Figure 1 and let S be the “semigroup”
in Figure 1 and let S be the “semigroup” ![]() is a semilattice. Clearly
is a semilattice. Clearly ![]() is a filter in L so that
is a filter in L so that ![]() is a congruence on S. But
is a congruence on S. But ![]() for any
for any![]() .
.
The following example shows that ![]() is a semi lattice congruence whenever
is a semi lattice congruence whenever ![]() then the “semigroup” need not be quasi-separative.
then the “semigroup” need not be quasi-separative.
Example 10: Let ![]() be two element null “semigroup”. Clearly
be two element null “semigroup”. Clearly ![]() and
and ![]() which is a semilattice ongruence. But is not a quasi-separative (since
which is a semilattice ongruence. But is not a quasi-separative (since ![]() and
and![]() ).
).
The following example shows that in non quasi-separative “semigroup’s” there exists ![]() such that
such that ![]() is a semi-lattice congruence.
is a semi-lattice congruence.
Example 11: Let S be a non quasi-separative “semigroup”, then 1s is in![]() ,
, ![]() and
and ![]() is a semi-lattice congruence.
is a semi-lattice congruence.
It is interesting to note that if S is a left or right zero “semigroup” then
![]() .
.
In paper [1] they have remarked that it is not known that whether semilattice of weakly cancellative “semigroup’s” is quasi-separative and weakly balanced. In the following we are giving an example which shows that it is not true i.e. if a “semigroup’s” is isomorphic to a semilattice of weakly cancellative “semigroup’s” then S is a quasi-separative and weakly balanced.
Example 12: Consider the “semigroup” ![]() with multiplication table as follows:
with multiplication table as follows:
Then h-classes are {a, b} and {c, d} which are right zero “semigroup’s” and hence S is a semilattice of weakly cancellative “semigroup’s”, but S is not weakly balanced since![]() ,
, ![]() , but
, but![]() .
.
The following is an example of quasi separative “semigroup”, which is not completely regular.
Example 13: Consider the “semigroup” ![]() on which “.” is defined by
on which “.” is defined by
![]()
where![]() . Clearly S is quasi separative “semigroup”, and since the inverse of (m, n) is (n, m) and
. Clearly S is quasi separative “semigroup”, and since the inverse of (m, n) is (n, m) and![]() , S is not completely regular.
, S is not completely regular.
Thorem 14: Let S be a separative “semigroup”, and ![]() such that
such that ![]() is a semilattice congruence then a Î
is a semilattice congruence then a Î![]() .
.
Proof: Let S be a separative “semigroup” and a Î S such that E(a) is a semilattice congruence. Then for any![]() ,
, ![]() , so that
, so that ![]()
![]() . Now replace “y” by “b” and “x” by “a” then
. Now replace “y” by “b” and “x” by “a” then ![]() which implies
which implies![]() . Since S is separative and
. Since S is separative and ![]() we have
we have ![]() so that
so that![]() . Since S is separative we have
. Since S is separative we have![]() . Again since E(a) is a semilattice congruence,
. Again since E(a) is a semilattice congruence, ![]() so that
so that ![]() and hence
and hence![]() .
.
Acknowledgements
We are very much thankful to the referees for their valuable suggestions.