1. Introduction
Difference equations or discrete dynamical systems are diverse field which impacts almost every branch of pure and applied mathematics. Every dynamical system  determines a difference equation and vise versa. Recently, there has been great interest in studying the system of difference equations. One of the reasons for this is a necessity for some techniques which can be used in investigating equations arising in mathematical models describing real life situations in population biology, economic, probability theory, genetics psychology, etc. The theory of difference equations occupies a central position in applicable analysis. There is no doubt that the theory of difference equations will continue to play an important role in mathematics as a whole. Nonlinear difference equations of order greater than one are of paramount importance in applications. Such equations also appear naturally as discrete analogues and as numerical solutions of differential and delay differential equations. It is very interesting to investigate the behavior of solutions of a system of nonlinear difference equations and to discuss the local asymptotic stability of their equilibrium points. Recently there has been published quite a lot of works concerning the behavior of positive solutions of systems of difference equations [1] -[8] . These results are not only valuable in their own right, but they can provide insight into their differential counterparts.
determines a difference equation and vise versa. Recently, there has been great interest in studying the system of difference equations. One of the reasons for this is a necessity for some techniques which can be used in investigating equations arising in mathematical models describing real life situations in population biology, economic, probability theory, genetics psychology, etc. The theory of difference equations occupies a central position in applicable analysis. There is no doubt that the theory of difference equations will continue to play an important role in mathematics as a whole. Nonlinear difference equations of order greater than one are of paramount importance in applications. Such equations also appear naturally as discrete analogues and as numerical solutions of differential and delay differential equations. It is very interesting to investigate the behavior of solutions of a system of nonlinear difference equations and to discuss the local asymptotic stability of their equilibrium points. Recently there has been published quite a lot of works concerning the behavior of positive solutions of systems of difference equations [1] -[8] . These results are not only valuable in their own right, but they can provide insight into their differential counterparts.
Papaschinopoulos et al. [1] investigated the global behavior for a system of the following two nonlinear difference equations.
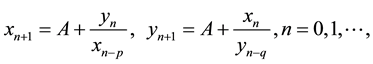
where A is a positive real number; p and q are positive integers, and  are positive real numbers.
are positive real numbers.
Clark and Kulenovic [2] [3] investigated the system of rational difference equations.
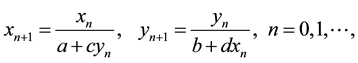
where 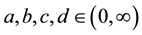 and the initial conditions
and the initial conditions 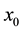 and
and 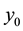 are arbitrary nonnegative numbers.
are arbitrary nonnegative numbers.
Yang [4] studied the system of high-order difference equations.
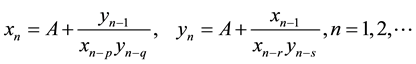
where  and initial values
and initial values 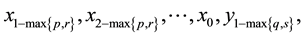
![]() are positive real numbers.
are positive real numbers.
Zhang, Yang and Liu [5] investigated the global behavior for a system of the following third order nonlinear difference equations.
![]()
where ![]() and initial values
and initial values ![]()
Zhang, Liu and Luo [6] studied dynamical behavior for third-order system of difference equations
![]()
where ![]() and initial values
and initial values ![]() are positive real numbers.
are positive real numbers.
Ibrahim [7] has obtained the positive solution of the difference equation system in the modeling competitive populations.
![]()
Din et al. [8] studied the global behavior of positive solution to the fourth-order rational difference equations
![]()
where the parameters ![]() and the initial conditions
and the initial conditions ![]() are positive real numbers.
are positive real numbers.
Although difference equations are sometimes very simple in their forms, they are extremely difficult to understand thoroughly the behavior of their solutions. In book [9] , Kocic and Ladas have studied global behavior of nonlinear difference equations of higher order. Similar nonlinear systems of difference equations were investigated (see [10] -[19] ).
Our aim in this paper is to investigate the solutions, stability character and asymptotic behavior of the system of difference equations
![]() (1)
(1)
where ![]() and initial conditions
and initial conditions![]() .
.
Clearly, if![]() , system (1) has always a positive equilibrium point
, system (1) has always a positive equilibrium point
![]()
2. Boundedness
Theorem 1. Let ![]() be a positive solution of (1), then the following statements holds:
be a positive solution of (1), then the following statements holds:
1) ![]() for each
for each ![]()
2) If![]() , then for
, then for![]() , we have
, we have
![]() (2)
(2)
Proof. Assertion 1) is obviously true. Now it only need to prove assertion 2). From (1) and in view of 1), we have, for![]() , that
, that
![]() (3)
(3)
Let ![]() be the solution of following system, respectively
be the solution of following system, respectively
![]() (4)
(4)
such that![]() .
.
We prove by induction that
![]() (5)
(5)
Suppose that (5) is true for ![]() From (3) that it follows that
From (3) that it follows that
![]() (6)
(6)
Therefore (5) is true. From (4) we have
![]() (7)
(7)
Then from (3), (5) and (6) the proof of the relation (2) follows immediately.
3. Stability
Theorem 2. Assume that![]() , then the unique positive equilibrium point
, then the unique positive equilibrium point
![]()
is locally asymptotically stable.
Proof. We can obtain easily the linearized system of (1) about the positive equilibrium ![]() is
is
![]() (8)
(8)
where
![]() (9)
(9)
Let ![]() denote the eigenvalues of matrix B, let
denote the eigenvalues of matrix B, let ![]() be a diagonal matrix, where
be a diagonal matrix, where ![]() and
and
![]()
Clearly, D is invertible. Computing matrix![]() , we obtain that
, we obtain that
![]()
From ![]() and
and![]() , it implies that
, it implies that
![]()
Furthermore
![]()
![]()
It is well known that B has the same eigenvalues as![]() , we have that
, we have that
![]()
This implies that the equilibrium ![]() of (1) is locally asymptotically stable.
of (1) is locally asymptotically stable.
Theorem 3. Assume that![]() . Then every positive solution of (1) converges to
. Then every positive solution of (1) converges to![]() .
.
Proof. Let ![]() be an arbitrary positive solution of (1). Let
be an arbitrary positive solution of (1). Let
![]()
![]()
From Theorem 2, we have ![]() This and (1) imply that
This and (1) imply that
![]()
which can derive that
![]() (10)
(10)
If ![]() and
and![]() , this implies that
, this implies that![]() , which contradict to (10). Therefore we have
, which contradict to (10). Therefore we have ![]() and
and![]() , then the
, then the ![]() and
and ![]() exist. From the uniqueness of the positive equilibrium
exist. From the uniqueness of the positive equilibrium ![]() of (1), we conclude that
of (1), we conclude that![]() ,
,![]() .
.
Combining Theorem 2 and Theorem 3, we obtain the following theorem.
Theorem 4. Assume that![]() . Then the positive equilibrium
. Then the positive equilibrium ![]() of (1) is globally asymptotically stable for all positive solutions.
of (1) is globally asymptotically stable for all positive solutions.
4. Rate of Convergence
In this section we will determine the rate of convergence of a solution that converges to the equilibrium point ![]() of the system (1). The following result gives the rate of convergence of solution of a system of difference equations
of the system (1). The following result gives the rate of convergence of solution of a system of difference equations
![]() (11)
(11)
where ![]() is a four dimensional vector,
is a four dimensional vector, ![]() is a constant matrix,
is a constant matrix, ![]() is a matrix function satisfying
is a matrix function satisfying
![]() (12)
(12)
where ![]() denotes any matrix norm which is associated with the vector norm.
denotes any matrix norm which is associated with the vector norm.
Theorem 5. [20] Assume that condition (12) hold, if Xn is a solution of (11), then either ![]() for all large n or
for all large n or
![]() (13)
(13)
or
![]() (14)
(14)
exists and is equal to the moduls of one the eigenvalues of the matrix A.
Assume that![]() , we will find a system of limiting equations for the system (1). The error terms are given as
, we will find a system of limiting equations for the system (1). The error terms are given as
![]()
Set![]() , therefore it follows that
, therefore it follows that
![]()
where
![]()
![]()
Now it is clear that
![]()
Hence, the limiting system of error terms at ![]() can be written as
can be written as
![]() (15)
(15)
where![]() , and
, and
![]()
Using Theorem 5, we have the following result.
Theorem 6. Assume that![]() , and
, and ![]() be a positive solution of the system (1). Then, the error vector
be a positive solution of the system (1). Then, the error vector ![]() of every solution of (1) satisfies both of the following asymptotic relations
of every solution of (1) satisfies both of the following asymptotic relations
![]()
where ![]() is equal to the moduls of one the eigenvalues of the Jacobian matrix evaluted at the equilibrium
is equal to the moduls of one the eigenvalues of the Jacobian matrix evaluted at the equilibrium![]() .
.
5. Numerical Examples
In order to illustrate the results of the previous sections and to support our theoretical discussions, we consider an interesting numerical example in this section.
Example 5.1. Consider the system (1) with initial conditions![]() , Moreover, choosing the parameters
, Moreover, choosing the parameters![]() . Then system (1) can be written as
. Then system (1) can be written as
![]() (16)
(16)
The plot of system (16) is shown in Figure 1.
Example 5.2. Consider the system (1) with initial conditions![]() , Moreover, choosing the parameters
, Moreover, choosing the parameters![]() . Then system (1) can be written as
. Then system (1) can be written as
![]() (17)
(17)
The plot of system (17) is shown in Figure 2.
6. Conclusions and Future Work
In this paper, the dynamical behavior of second-order discrete system is studied. It can be concluded that:
1) The positive equilibrium point ![]() is globally asymptotically stable if
is globally asymptotically stable if![]() .
.
2) The equilibrium rate of convergence is discussed. Some numerical examples are provided to support theoretical results. It is our future work to study the oscillation behavior of system (1).
Acknowledgements
The author would like to thank the Editor and the anonymous referees for their careful reading and constructive suggestions.