with 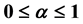 and
and  is an increasing sequence diverging to
is an increasing sequence diverging to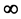 .
.
Keywords:
1. Introduction
Let 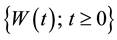 be a standard Wiener process. Suppose that
be a standard Wiener process. Suppose that 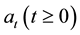 is a nondecreasing function of t such that
is a nondecreasing function of t such that  with
with 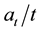 is nonincreasing and
is nonincreasing and 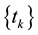 is an increasing sequence diverging to
is an increasing sequence diverging to . In [1] the following results are established.
. In [1] the following results are established.
i) If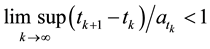 , then
, then
![]() (1)
(1)
and
![]() (2)
(2)
where ![]() and
and
![]() .
.
ii) If![]() , then
, then
![]() ,
,
where![]() ,
, ![]() and
and![]() .
.
In this paper the limit theorems on increments of a Wiener process due to [1] are developed to the case of a Gaussian process. This can be considered also as an extension of the results to Gaussian processes obtained in [2] . Throughout this paper, we shall always assume the following statements: Let ![]() be an almost
be an almost
surely continuous Gaussian process with![]() ,
, ![]() and
and![]() , where
, where
![]() is a function of
is a function of![]() . Further we assume that
. Further we assume that![]() ,
, ![]() , is a nondecreasing continuous concave, regularly varying function at exponent
, is a nondecreasing continuous concave, regularly varying function at exponent ![]() at
at ![]() (e.g., if
(e.g., if ![]() is a standard Wiener pro- cess, then
is a standard Wiener pro- cess, then![]() ).
).
Let ![]() be a nondecreasing function of t with
be a nondecreasing function of t with![]() . For large t, let us denote
. For large t, let us denote
![]()
where ![]() and
and ![]() is an increasing function of
is an increasing function of![]() .
.
We define two continuous parameter processes ![]() and
and ![]() by
by
![]()
and
![]() .
.
2. Main Results
In this section we provide the following two theorems which are the main results. We concern here with the development of the limit theorems of a Wiener process to the case of a Gaussian process under consideration the above given assumptions.
Theorem 1. Let ![]() be a nondecreasing function of t where
be a nondecreasing function of t where ![]() with the nonincreasing function
with the nonincreasing function ![]() and let
and let ![]() be any increasing sequence diverging to
be any increasing sequence diverging to ![]() such that
such that
![]() , (3)
, (3)
then
![]() (4)
(4)
and
![]() (5)
(5)
where![]() .
.
We note that ![]() for large k in case of the Wiener process. It is interesting to compare (1) and (2) with (4) and (5) respectively.
for large k in case of the Wiener process. It is interesting to compare (1) and (2) with (4) and (5) respectively.
Theorem 2. Let ![]() be a nondecreasing function of
be a nondecreasing function of ![]() where
where ![]() with the nonincreasing function
with the nonincreasing function ![]() and let
and let ![]() be an increasing sequence diverging to
be an increasing sequence diverging to ![]() such that
such that
![]() , (6)
, (6)
then
![]() (7)
(7)
and
![]() (8)
(8)
where ![]() and
and![]() .
.
3. Proofs
In order to prove Theorems 1 and 2, we need to give the following lemmas.
Lemma 1. (See [3] ). For any small ![]() there exists a positive
there exists a positive ![]() depending on
depending on ![]() such that for all
such that for all ![]()
![]() ,
,
where m is any large number and ![]() is defined above.
is defined above.
Lemma 2. (See [4] ) Let ![]() and
and ![]() be centered Gaussian processes such that
be centered Gaussian processes such that
![]() for all
for all ![]() and
and ![]() for all
for all![]() . Then for any real number u
. Then for any real number u
![]() .
.
Proof of Theorem 1. Firstly, we prove that
![]() (9)
(9)
For any ![]() with the condition (3), we define an increasing sequence
with the condition (3), we define an increasing sequence ![]() by
by
![]() .
.
For instance, let ![]() for some
for some![]() ,
,
![]() .
.
The condition (3) is satisfied, and for large k, ![]() and
and![]() . By Lemma 1, we have, for any small
. By Lemma 1, we have, for any small![]() ,
,
![]() (10)
(10)
where k is large enough and ![]() is a constant. By the definition of
is a constant. By the definition of![]() ,
,![]() .
.
We shall follow the similar proof process as in [5] . Set
![]() .
.
Since ![]() is an increasing sequence, the fact that
is an increasing sequence, the fact that ![]() implies
implies![]() . Consider the odd subse-
. Consider the odd subse-
quence ![]() of
of ![]() and define the sequence of events
and define the sequence of events ![]() in the following form
in the following form
![]() .
.
By (10), for large k we have
![]()
where ![]() is a constant. From the fact
is a constant. From the fact![]() , it is clear that
, it is clear that
![]() .
.
Since![]() , we get
, we get![]() . Also,
. Also,
![]() . (11)
. (11)
Setting
![]()
and
![]() ,
,
we have
![]() .
.
Let
![]() ,
,
and
![]() .
.
Then, by (11) and the concavity of ![]() we find that
we find that
![]()
This implies that![]() . Using Lemma 2, we obtain
. Using Lemma 2, we obtain
![]()
where![]() . It follows from the Borel-Cantelli lemma that
. It follows from the Borel-Cantelli lemma that
![]()
Also, the same result for the even subsequence ![]() of
of ![]() is easily obtained. Therefore we have (9).
is easily obtained. Therefore we have (9).
To finish the proof of Theorem 1, we need to prove
![]() (12)
(12)
The proof of (12) is similar to the provided proof in [1] . Thus the proof of Theorem 1 is complete.
Proof of Theorem 2. Firstly, we prove that
![]() (13)
(13)
According to Lemma 1, we have
![]()
provided k is large enough, where ![]() and
and![]() .
.
From the definition of![]() , it follows that
, it follows that
![]() .
.
Thus, (13) is immediate by using Borel Cantelli lemma.
To finish the proof of Theorem 2 we need to prove
![]() (14)
(14)
Let
![]() .
.
Using the well known probability inequality
![]()
(see [6] ), one can find positive constants C and K such that, for all![]() ,
,
![]()
where ![]() and
and![]() . By the definition of
. By the definition of![]() , we have
, we have
![]() .
.
The condition (6) implies that there exists ![]() such that
such that ![]() for all
for all![]() . So, using Lemma 2 and the concavity of
. So, using Lemma 2 and the concavity of![]() , we obtain
, we obtain
![]() ,
,
where ![]() and Borel-Cantelli lemma implies (14). If
and Borel-Cantelli lemma implies (14). If![]() , then Theorem 2 is immediate. Thus the proof of Theorem 2 is complete.
, then Theorem 2 is immediate. Thus the proof of Theorem 2 is complete.
4. Some Results for Partial Sums of Stationary Gaussian Sequence
In this section we obtain similar results as Theorems 1 and 2 for the case of partial sums of a stationary Gaussian sequence. Let ![]() be a stationary Gaussian sequence with
be a stationary Gaussian sequence with![]() ,
, ![]() ,
, ![]() and
and
![]() for all
for all ![]() We define
We define ![]() with
with ![]() and set
and set![]() .
.
Assume that ![]() can be extended to a continuous function
can be extended to a continuous function ![]() with
with ![]() which is nondecreasing and regularly varying with exponent
which is nondecreasing and regularly varying with exponent ![]() at
at![]() . Suppose that
. Suppose that ![]() is a nondecreasing sequence of positive integers such that
is a nondecreasing sequence of positive integers such that![]() . For large n, we define
. For large n, we define
![]() ,
,
where ![]() and
and ![]() is an increasing function of n and also we define discrete time parameter processes by
is an increasing function of n and also we define discrete time parameter processes by
![]()
and
![]() ,
,
respectively, where ![]() is an increasing sequence of positive integers diverging to
is an increasing sequence of positive integers diverging to![]() . By the same way as in the proofs of Theorems 1 and 2, we obtain the following results.
. By the same way as in the proofs of Theorems 1 and 2, we obtain the following results.
Theorem 3. Under the above statements of![]() ,
, ![]() and
and![]() , for
, for ![]() we have the following:
we have the following:
i) If![]() , then
, then
![]()
ii) If![]() , then
, then
![]()
where
![]() .
.
Example. Let ![]() be a fractional Brownian motion with the covariance function
be a fractional Brownian motion with the covariance function
![]() . Then
. Then
![]() .
.
Define random variables
![]() ,
,
![]()
![]() and.
and.
Then
![]()
and ![]() is a stationary Gaussian sequence with
is a stationary Gaussian sequence with![]() ,
, ![]() and
and ![]() for all
for all![]() . So we have Theorem 3.
. So we have Theorem 3.
In particular if![]() , then
, then ![]() is an i.i.d. Gaussian sequence with
is an i.i.d. Gaussian sequence with ![]() and
and![]() .
.
5. Conclusion
In this paper, we developed some limit theorems on increments of a Wiener process to the case of a Gaussian process. Moreover, we obtained similar results of these limit theorems for the case of partial sums of a stationary Gaussian sequence. Some obtained results can be considered as extensions of some previous given results to Gaussian processes.