Alternative Coins for Quantum Random Walk Search Optimized for a Hypercube ()
1. Introduction
The search algorithms for unstructured databases are widely used in statistical data processing for searching the maximum or minimum element or an element corresponding to specific criteria. Effective search algorithms can provide a solution for one of the Non-Deterministic Polynomial Time Complete (NPTC) problems, from which a solution can be found to any NPTC problem by an algorithm with polynomial complexity. These are the reasons for the great interest in the quantum search algorithms and their experimental implementation.
The first quantum search algorithm for unstructured databases is created by Grover [1] and is based on a quantum Fourier transformation. Quantum search on a two-qubit cavity QED database has been done by Yamaguchi et al. [2] . Quantum search on a three-qubit database with NMR has been done by Vandersypen et al. [3] . Grover’s algorithm cannot be used without knowing the exact number of solutions. To find the exact number of elements and satisfy the search criteria, the quantum counting algorithm should be used.
There are already many classical random walk algorithms that perform much better in their tasks than deterministic algorithms. Two classes of such algorithms are Las Vegas algorithms (which always end with a correct result when used for a finite time) and Monte Carlo algorithms (which depend on random input and might produce an incorrect result). Las Vegas algorithms are widely used in fields like artificial intelligence [4] , biology [5] and others. Monte Carlo algorithms are used in mathematics, condensed matter physics [6] - [8] and others.
Another type of quantum algorithms are the ones based on the quantum random walk; they are analogous to the classical random walk. There are two types of those algorithms: continuous time evolution random walk algorithms (CTRWA) and discrete time random walk algorithms (DTRWA). The CTRWA were first introduced by Farhi and Gutmann [9] . They have showed that CTRWA propagate exponentially faster through graphs [10] and can solve any black box problem like searching exponentially faster than any classical algorithm [11] . Childs has shown that the continuous time quantum random walk search algorithms (CTRWS) can find an element in a graph with dimension over 4D faster than Grover’s search algorithm [12] . DTRWA have been first proposed by Aharonov et al. [13] . Examples for DTRWA algorithms are the quantum random walk algorithms for element distinction [14] and the quantum random walk search algorithms [15] . Discrete time random walk search algorithms (DTRWSA) have been first created by Shenvi et al. [15] and are denoted as SKW. The original SKW search algorithm can find an element with probability less than 1/2. Hein has proposed a faster DTRWSA, but to be effective, the initial state of the algorithm should take into account which elements are to be searched [16] . Potocek et al. have shown that if the searched space is divided into two parts, the probability to find a solution can be increased close to 1, with large enough searched space [17] . They also have demonstrated that the probability of finding a solution can be increased if the shift operator is modified to divide the searched space. Tulsi has shown that DTRWSA is faster than Grover’s search when the searched space is two-dimensional [18] .
Grover’s search, CTRWSA and DTRWSA differ conceptually in terms of working principle. This is the reason for their different advantages and disadvantages. Grover’s search algorithm and DTRWSA can be modified to find a solution with probability close to one. Long has shown that Grover’s search can be modified so that the probability of successfully finding a solution with it to be exactly equal to one [19] , which means that the algorithm evolves from a quantum probabilistic to a quantum deterministic method. Potocek et al. have shown that a probability to find solution close to one can be obtained in DTRWSA by two different methods [17] . Grover’s search algorithm needs only one oracle call for each iteration of the algorithm and less number of qubits (as much as needed to store the searched space); let this number be denoted as n. DTRWSA needs more qubits: O(n), and two oracle calls for each iteration [20] . DTRWSA is much better than Grover’s algorithm, when there is the need to search in a register of two or more dimensions [18] [21] .
The present paper is organized as follows. In Section 2, the discrete quantum random walk is reviewed, and the quantum random walk on a line is shown as an example. In Section 3, the quantum random walk on a hyper- cube and the quantum random walk search on a hypercube are reviewed. In Section 4, a new alternative way is demonstrated for the method shown in [17] for dividing the searched space of the algorithm in two, while sustaining the hypercube topology and effectively dividing the searched space by using coins, which unequally distribute the probability of transition to adjacent nodes. In Section 4.1, Householder reflection is reviewed. In Section 4.2, the general form of specialized coins is shown; whereas their use is discussed in Section 4.3. In Section 4.4, some examples of such coins and quantum circuit for their experimental implementation in quantum random walk search algorithm are shown. The results of numerical simulations with the coins are also given. Section 5 is the conclusion of the article.
2. Classical and Discrete Quantum Random Walk, Quantum Random Walk on Line
The classic random walk on a line starts at an initial state  and at every step, a coin is tossed. There is a different probability of the possible outcomes of the toss. The sum of these probabilities is equal to one. Each of the possible outcomes of the toss is associated with a distinct direction, and the directions depend on the structure of the graph which is traveled over. For example, for a line the directions are left and right. For a square grid, the directions are left, right, up and down. The particle moves one step in the corresponding direction, according to the result of the coin toss.
and at every step, a coin is tossed. There is a different probability of the possible outcomes of the toss. The sum of these probabilities is equal to one. Each of the possible outcomes of the toss is associated with a distinct direction, and the directions depend on the structure of the graph which is traveled over. For example, for a line the directions are left and right. For a square grid, the directions are left, right, up and down. The particle moves one step in the corresponding direction, according to the result of the coin toss.
The quantum random walk algorithm is the quantum analogue of the classic random walk algorithm. HC is the Hilbert space of the quantum coin (coin space) and HS is the Hilbert space of the nodes of the structure of the graph. Again, each step of the algorithm (which is described by the operator U) has two parts. First is the coin toss. The coin flip is defined by the unitary operator of the coin C0, which acts in the coin space HC. The coin operator acts upon the Hilbert space 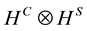 and is denoted by
and is denoted by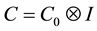 . The result of the action of the coin operator upon the coin is a chiral state [22] . This is an analogue of the classic probability. As the chiral state is a quantum state, it can be in a quantum superposition of directions. According to the toss outcome, the state of the system is changing. The exact change of the state depends on the structure. The quantum operator which represents this structure is a permutation matrix which performs controlled shift, depending on the state of the coin, and is denoted by S. The operator S acts upon the space
. The result of the action of the coin operator upon the coin is a chiral state [22] . This is an analogue of the classic probability. As the chiral state is a quantum state, it can be in a quantum superposition of directions. According to the toss outcome, the state of the system is changing. The exact change of the state depends on the structure. The quantum operator which represents this structure is a permutation matrix which performs controlled shift, depending on the state of the coin, and is denoted by S. The operator S acts upon the space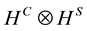 . Summarily, each step of the quantum random walk can be written as:
. Summarily, each step of the quantum random walk can be written as:
 (1)
(1)
An example for a shift operator is SL corresponding to a quantum random walk on a line [23] :
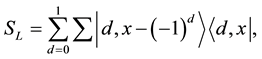 (2)
(2)
where x is the position of the particle on the line. The values of d (0 or 1) correspond to left and right directions. For the coin, a Hadamard matrix can be used:
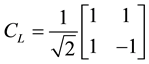 . (3)
. (3)
Summarily, a DQRW step on line can be written as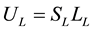 . The classic random walk spreads as a binomial distribution after each step. In DQRW, after each step of the algorithm, quantum interference occurs when more than one possible path exists to reach the respective position. The interference can be constructive or destructive, which leads to very different distribution compared to the classic random walk on a line. The variance in the number of steps t between the classic random walk and DQRW is very different. DQRW spreads as O(t); in comparison, the classic random walk spreads as
. The classic random walk spreads as a binomial distribution after each step. In DQRW, after each step of the algorithm, quantum interference occurs when more than one possible path exists to reach the respective position. The interference can be constructive or destructive, which leads to very different distribution compared to the classic random walk on a line. The variance in the number of steps t between the classic random walk and DQRW is very different. DQRW spreads as O(t); in comparison, the classic random walk spreads as  [23] . If the quantum random walk is measured at each coin flip, or after the end of each step, it will revert to the classic random walk [15] .
[23] . If the quantum random walk is measured at each coin flip, or after the end of each step, it will revert to the classic random walk [15] .
3. Quantum Random Walk and Search on a Hypercube
The hypercube is a graph with 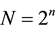 nodes and n edges between nodes. Each one of the nodes will be denoted by a n-bit string
nodes and n edges between nodes. Each one of the nodes will be denoted by a n-bit string . Two nodes of a hypercube,
. Two nodes of a hypercube,  and
and 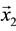 are connected only if the modulus of hamming weight of their difference is equal to one:
are connected only if the modulus of hamming weight of their difference is equal to one: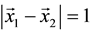 . That is why the Hilbert space of the coin is
. That is why the Hilbert space of the coin is , the Hilbert space of the nodes is
, the Hilbert space of the nodes is  and the Hilbert space of the random walk is
and the Hilbert space of the random walk is ![]() [15] . The shift operator for the hypercube SC is:
[15] . The shift operator for the hypercube SC is:
![]() (4)
(4)
where d is the direction of the motion and ![]() is the d-th basis vector of the Hypercube.
is the d-th basis vector of the Hypercube.
The Grover coin G is frequently chosen for a coin for quantum random walks on a hypercube. This coin is invariant to all permutations of the n edge directions, so it sustains the permutation symmetries of the hypercube.
![]() (5)
(5)
where I is identity operator, ![]() is an equal weight superposition of the states of all directions.
is an equal weight superposition of the states of all directions.
To make a quantum random walk search algorithm, a quantum oracle should be applied that marks the wanted element by applying a coin upon it. The oracle does this by using the function![]() , which is used to determine which coin would be applied: C0 or C1,
, which is used to determine which coin would be applied: C0 or C1,
![]() . (6)
. (6)
Summarily, the operator of the coin becomes:
![]() (7)
(7)
where C1 can be almost any unitary operator but most often it is taken![]() . The reason for this is the faster spread through the graph and the simplicity in experimental realization. Summarily, the random walk search iteration can be written as:
. The reason for this is the faster spread through the graph and the simplicity in experimental realization. Summarily, the random walk search iteration can be written as:
![]() . (8)
. (8)
The quantum circuit of the random walk search algorithm is shown in Figure 1 [15] . This circuit does not actually depend on the shape of the searched graph. When the graph is different, the shift operator S has to be changed. Summarily, the steps of the algorithm are [20] :
1) Initializing the starting state of the coin and node register in an equal weight superposition. This can be done by applying Hadamard gate on each qubit of the state![]() ;
;
2) Applying quantum random walk search iteration ![]() times [15] .
times [15] .
The steps of the quantum random walk search iteration are:
a) Applying a quantum oracle;
b) Applying an appropriate coin depending on the state of the control register;
c) Applying the quantum oracle;
d) Applying the shift operator.
Due to the symmetry of the hypercube, its nodes can always be re-labeled in such way that the marked node ![]() becomes node
becomes node ![]() [17] . The position of
[17] . The position of ![]() and the fact that the initial state is an equal weight superposition allows to project the quantum random walk on hypercube onto a quantum random walk on a line, as shown in Figure 2. The basic states of the collapsed random walk on a hypercube are:
and the fact that the initial state is an equal weight superposition allows to project the quantum random walk on hypercube onto a quantum random walk on a line, as shown in Figure 2. The basic states of the collapsed random walk on a hypercube are:
![]() , (9)
, (9)
![]() . (10)
. (10)
The shift operator in this collapsed random walk basis![]() ,
, ![]() becomes:
becomes:
![]() . (11)
. (11)
The quantum random walk on a line strongly depends on the position. In the basis![]() ,
, ![]() , the Grover coin becomes:
, the Grover coin becomes:
![]() , (12)
, (12)
where ![]() and
and![]() . The perturbed coin becomes
. The perturbed coin becomes![]() .
.
4. Algorithm with Coins from Generalized Householder Reflection
Potocek et al. have shown in [17] that if the register is divided into two subspaces―for even and for odd elements?by the shift operator, they can both evolve separately. Thus, the probability to find a solution increases twofold.
In this chapter it will be demonstrated that the same result can also be obtained by using appropriate coins.
4.1. Generalized Householder Reflection
The generalized Householder reflection![]() , is widely used in quantum information and it is given by the expression:
, is widely used in quantum information and it is given by the expression:
![]() (13)
(13)
where ![]() is a normalized N-dimensional vector, generally complex, φ is the phase, I is the identity operator. In the original SKW algorithm [15] for searching a marking coin, the operator (C1) with a minus sign is used. It can be viewed as
is a normalized N-dimensional vector, generally complex, φ is the phase, I is the identity operator. In the original SKW algorithm [15] for searching a marking coin, the operator (C1) with a minus sign is used. It can be viewed as ![]() with a phase equal to zero. For a walking coin (C0), the Grover’s coin is used which is also a Householder reflection when
with a phase equal to zero. For a walking coin (C0), the Grover’s coin is used which is also a Householder reflection when ![]() is an equal-weight superposition of all basic states and the phase is equal to π.
is an equal-weight superposition of all basic states and the phase is equal to π.
![]()
Figure 1. Quantum circuit for random walk search algorithm. The box marked as T is the random walk search iteration and shoud be repeated t times. The value of t is shown in Section 3.
![]()
Figure 2. Projecting random walk search algorithm on a hypercube to a random walk on a line. The marked state is shown with orange.
![]() (14)
(14)
In [16] , the case is discussed when C0 is again an equal superposition vector, but the phase is random. In this paper, only Householder reflection with phase equal to π will be discussed, when ![]() is different from the one used in SKW.
is different from the one used in SKW.
4.2. General Form of the Coins
Here we will view the case when C0 is a standard Grover coin, as it is in the original SKW search algorithm:
![]() , (15)
, (15)
![]() , (16)
, (16)
where ![]() is the j-th basis vector
is the j-th basis vector![]() , and
, and ![]() is the equal weight superposition vector.
is the equal weight superposition vector.
For the marking coin C1, an arbitrary Householder reflection is taken with a phase π:
![]() , (17)
, (17)
![]() , (18)
, (18)
![]() , (19)
, (19)
here aj is real and![]() .
.
The coin and the random walk step are unchanged, as in the standard SKW search algorithm:
![]() , (20)
, (20)
![]() . (21)
. (21)
The perturbed random walk coin is:
![]() (22)
(22)
![]() . (23)
. (23)
This form is too general, so in the next subsection some examples for coins will be discussed.
4.3. Algorithm
The steps of this implementation of QRWS are the same as in the SKW search algorithm. The quantum circuit of this algorithm is almost the same as in SKW and is shown in Figure 1, the difference being that the marking coin is different and an additional qubit is taken which does not need to be measured at the end of the algorithm.
4.4. Examples for Some Good Coins
Some examples of coins suitable for a random walk search are proposed in this section. These examples are probably not only the useful ones but also can be performed relatively easily in experiments. A Householder reflection can easily be done with an N-pod system.
For simplicity, a hypercube with dimension 2K, instead of a hypercube with dimension N will be reviewed.
![]() .
.
From here on, yi will denote arbitrary values, and yi may or may not be equal to yj at![]() . Also, x is an arbitrary value, the modulus of which is larger than the modulus of yi at any i. The number of yi as altogether is n − 1.
. Also, x is an arbitrary value, the modulus of which is larger than the modulus of yi at any i. The number of yi as altogether is n − 1.
One case of asymmetrical coins is when![]() ,
, ![]() , where
, where![]() , i is an integer and
, i is an integer and![]() , and r can be 0 or n − 1. These coins are designed for random walk search on a hypercube. They have an asymmetrical shape which effectively leads to division of the searched space to two (Figure 4).
, and r can be 0 or n − 1. These coins are designed for random walk search on a hypercube. They have an asymmetrical shape which effectively leads to division of the searched space to two (Figure 4).
In the first searched subspace, the coin marks the element marked by the oracle. In the second searched subspace, the marking coin effectively marks one of the adjacent nodes. The matrix chosen to be used in the marking coin defines which of the nodes is marked. The division of the searched space in two requires an additional qubit (the number of states of the register prior to the division is 2K) in order to perform the search and to have a probability of finding a solution above 80%.
Here are two examples of such coins, depending on the way of doubling the number of states by adding a qubit:
The first type of such coin is when![]() ,
, ![]() , where
, where![]() , i is an integer and
, i is an integer and![]() :
:
![]() (24)
(24)
This result can easily be explained when![]() ,
, ![]() , α = 1. The coins C1, C0,
, α = 1. The coins C1, C0, ![]() (see Equation (5)) differ from each other only by the sign of the components of their matrices, so they mark the same edges with the same amplitudes. The coin C0 marks all edges connected with the states with a plus sign. The coin
(see Equation (5)) differ from each other only by the sign of the components of their matrices, so they mark the same edges with the same amplitudes. The coin C0 marks all edges connected with the states with a plus sign. The coin ![]() marks all edges connected with the marked state with a minus sign. The coin C1 marks all edges except the last one with a plus sign, and the last one?with a minus sign (Figure 3). The first hypercube with size (K) for quantum random walk search is obtained from the marked state in such way as not to include the state marked by the coin with a minus sign (Figure 4). On the other hand, the node which is marked with a minus sign partakes in a hypercube with size (K) so that it does not include the marked state (Figure 4). This is second hypercube in the searched space divided into two.
marks all edges connected with the marked state with a minus sign. The coin C1 marks all edges except the last one with a plus sign, and the last one?with a minus sign (Figure 3). The first hypercube with size (K) for quantum random walk search is obtained from the marked state in such way as not to include the state marked by the coin with a minus sign (Figure 4). On the other hand, the node which is marked with a minus sign partakes in a hypercube with size (K) so that it does not include the marked state (Figure 4). This is second hypercube in the searched space divided into two.
The number of steps needed depends on the exact values of x and yi. The quantum circuit needed for those types of coins is shown in Figure 5.
The simulations are made by two qubit coins because of the absence of enough computational power to make simulations for coins with more qubits.
Values![]() ,
, ![]() , α = 1 are good for explanation of the working of the algorithm. For two qudit coins, when they are used, the probability for finding a solution at the 6-th iteration is 0.678, and 9 iterations are needed to obtain the maximal probability 0.859.
, α = 1 are good for explanation of the working of the algorithm. For two qudit coins, when they are used, the probability for finding a solution at the 6-th iteration is 0.678, and 9 iterations are needed to obtain the maximal probability 0.859.
With two-qubit coins, ![]() and
and ![]() the algorithm needs 6 random walk steps. The result of the numerical simulation with searched element 4 is shown in Figure 6. Simulations demonstrate that these coins can also be used when there is more than one marked state.
the algorithm needs 6 random walk steps. The result of the numerical simulation with searched element 4 is shown in Figure 6. Simulations demonstrate that these coins can also be used when there is more than one marked state.
![]()
Figure 6. Result of simulating a quantum circuit with a coin with ![]() and
and ![]() and 6 random walk steps. The searched element in the simulation is 4 and the size of the node register is 3 qubits.
and 6 random walk steps. The searched element in the simulation is 4 and the size of the node register is 3 qubits.
It has been obtained by numerical simulations that a higher probability of finding the searched element is achieved when ![]() and
and ![]() is used.
is used.
Another type of such coin is the case when![]() ,
, ![]() , i is an integer and
, i is an integer and![]() , where
, where![]() . An example for this is the coin with
. An example for this is the coin with ![]() and
and![]() . The quantum circuit for those types of coins is shown in Figure 7. The algorithm needs 6 random walk steps, when the register of the coin consists of two coin qubits and
. The quantum circuit for those types of coins is shown in Figure 7. The algorithm needs 6 random walk steps, when the register of the coin consists of two coin qubits and ![]() and
and![]() . The result of the simulation?in this case with searched element 2?is shown in Figure 8. Simulations demonstrate that these coins can also be used when there is more than one marked state.
. The result of the simulation?in this case with searched element 2?is shown in Figure 8. Simulations demonstrate that these coins can also be used when there is more than one marked state.
When ![]() and
and![]() , the algorithm also needs 9 iterations to obtain its maximal probability 0.859. A higher probability of obtaining the searched element is achieved when
, the algorithm also needs 9 iterations to obtain its maximal probability 0.859. A higher probability of obtaining the searched element is achieved when ![]() and
and![]() , by analogy with
, by analogy with ![]() and
and![]() .
.
With all other cases having this structure of the coin, when![]() ,
, ![]() , i is an integer and
, i is an integer and ![]() , where
, where![]() , r can be any number in the interval
, r can be any number in the interval![]() , the quantum circuit for obtaining the result will be more complicated or additional classical processing is needed.
, the quantum circuit for obtaining the result will be more complicated or additional classical processing is needed.
Numerical simulations show that at least when the size of the node register N = 16, there are also other coins with different shape of the vector ![]() which can be used efficiently.
which can be used efficiently.
Examples for such coins are when
![]() , (25)
, (25)
![]() , (26)
, (26)
![]()
Figure 8. Result of the simulation of the quantum circuit with a coin with ![]() and
and ![]() and 6 random walk steps. The searched element in the simulation is 2 and size of the node register is 3 qubits.
and 6 random walk steps. The searched element in the simulation is 2 and size of the node register is 3 qubits.
![]() , (27)
, (27)
and when
![]() . (28)
. (28)
An example for such coins is when the formula (28) is used with the quantum circuit shown in Figure 5. The probability for finding a solution is approximately 0.77, with w = 3.
Another example of search coin is when the formula (26) is used with the quantum circuit shown in Figure 7. The probability for finding a solution is approximately 0.77, with w = 3.
The number of steps needed for the coins showed in this section is obtained by numerical simulations and has not been found empirically yet.
5. Conclusions
A discrete quantum random walk search algorithm optimized for hypercube is discussed. A new alternative DTRWS method for dividing the searched space in two is presented. The searched space is divided effectively into two by using asymmetric coins, which distribute the probability of shifting into neighboring nodes non-un- iformly.
The advantage of this method is that it preserves the topology of the hypercube and does not divide it by modifying the shift operator. The coins are obtained by using Householder reflection, which can be easily performed in experiments by using N-pod systems.