The Weighted Mean Standard Deviation Distribution: A Geometrical Framework ()
1. Introduction
Hyperspace geometry is useful, if not essential, for the interpretation of theories involving many branches of knowledge and, in particular, general relativity (e.g., [1] ) and superstring theory (e.g., [2] ). Though further insight could be gained exploiting a geometrical framework, still pure mathematical analysis is preferred owing to a much greater difficulty in handling with hyperspace geometry.
The geometrical framework of a well known statistical problem, concerning the explicit expression of the arithmetic mean standard deviation distribution, has been considered in an earlier investigation [3] .
The current attempt is aimed at extenting the above mentioned results to the general case of the weighted mean, or in other words searching the explicit expression of the weighted mean standard deviation distribution, under the safely motivated restriction of independent measures obeying Gaussian distributions. Accordingly, the weighted mean standard deviation depends only on the uncertainties on the input data, which is not in con- tradiction with its counterpart inferred from a different approach based on the least-squares method [4] .
The extension of the procedure followed in the parent paper [3] yields results which reduce to their counter- parts therein, in the special case where the weighted mean reduces to the arithmetic mean.
The paper is organized as follows. The problem is outlined in Section 2 together with three steps towards the solution. The first, second, third step are exploited in Sections 3, 4, 5, respectively. In addition, three different attempts are exploited in Section 4. The solution of the problem is shown in Section 6, where a number of (well known) related parameters are also inferred for sake of completeness. The conclusion is drawn in Section 7. Further details are shown in the Appendix, including extension of analytic geometry formulation to 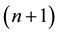 - spaces, Jacobi formulae, reduction of the results to ordinary geometry, and a corrigendum to the parent paper [3] .
- spaces, Jacobi formulae, reduction of the results to ordinary geometry, and a corrigendum to the parent paper [3] .
2. The Problem
Let 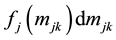 be distributions related to assigned measure methods,
be distributions related to assigned measure methods, 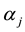 ,
, 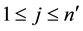 , and a specified
, and a specified
statistical system, where the occurrence of the events,  , has been designed by the value of the random var- iables,
, has been designed by the value of the random var- iables, 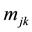 ,
,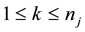 . The special case of Gaussian distributions, which well holds for independent measures, reads:
. The special case of Gaussian distributions, which well holds for independent measures, reads:
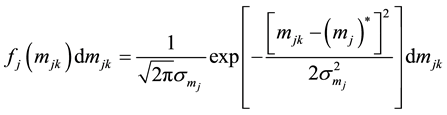 (1)
(1)
where 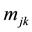 is a generic measure related to the method,
is a generic measure related to the method, 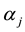 , and
, and ,
, 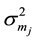 ,
, ![]() , are the expected value, the variance, the rms error, respectively, of the distribution. Expected values and rms error estimators are known to be the arithmetic mean and the standard deviation, respectively, and the results of an earlier study [3] well apply to each method,
, are the expected value, the variance, the rms error, respectively, of the distribution. Expected values and rms error estimators are known to be the arithmetic mean and the standard deviation, respectively, and the results of an earlier study [3] well apply to each method, ![]() , taken separately.
, taken separately.
Let ![]() be the distribution related to the whole set of the above mentioned measure methods and statistical system, where the occurrence of the event,
be the distribution related to the whole set of the above mentioned measure methods and statistical system, where the occurrence of the event, ![]() , has been designed by the value of the random variable,
, has been designed by the value of the random variable, ![]() , which is a linear combination of the above mentioned random variables, for sake of simplicity denoted hereafter as
, which is a linear combination of the above mentioned random variables, for sake of simplicity denoted hereafter as![]() , via the coefficients,
, via the coefficients, ![]() ,
, ![]() ,
,![]() . Owing to a theorem of statistics (e.g., [5] , Chapter 8; [6] , Chapter 2), the explicit expression reads:
. Owing to a theorem of statistics (e.g., [5] , Chapter 8; [6] , Chapter 2), the explicit expression reads:
![]() (2)
(2)
![]() (3)
(3)
![]() (4)
(4)
where ![]() is related to a generic
is related to a generic ![]() collection and
collection and![]() ,
, ![]() ,
, ![]() , are the expected value, the variance, the rms error, respectively, of the distribution.
, are the expected value, the variance, the rms error, respectively, of the distribution.
The additional condition on the coefficients:
![]() (5)
(5)
ensures that in the special case of distributions with equal expected values, which is the one under consideration,
![]() ,
, ![]() via Equation (3), using a theorem of statistics (e.g., [5] , Chapter 8; [6] , Chapter 2).
via Equation (3), using a theorem of statistics (e.g., [5] , Chapter 8; [6] , Chapter 2).
The further condition that the variance, defined by Equation (4), has to be minimum for fixed data, implies for the coefficients, ![]() , the following expression (e.g., [6] , Chapter 4):
, the following expression (e.g., [6] , Chapter 4):
![]() (6)
(6)
and Equations (2), (3), (4), (6), reduce to:
![]() (7)
(7)
![]() (8)
(8)
![]() (9)
(9)
![]() (10)
(10)
where ![]() is a random variable and
is a random variable and![]() ,
, ![]() ,
, ![]() , are the expected value, the variance, the rms error, respectively, of the distribution.
, are the expected value, the variance, the rms error, respectively, of the distribution.
Expected value and rms error estimators are known to be the weighted mean, ![]() , expressed by Equation (8) where
, expressed by Equation (8) where ![]() are measured values, and the standard deviation,
are measured values, and the standard deviation, ![]() , respectively, which reads:
, respectively, which reads:
![]() (11)
(11)
![]() (12)
(12)
where ![]() is the deviation from the weighted mean. It is worth emphasizing the tilde over
is the deviation from the weighted mean. It is worth emphasizing the tilde over ![]() and
and ![]()
means the rms error and the deviation relate to the weighted mean: neither ![]() nor
nor![]() , in itself, is a weighted mean. In addition, the following relations hold:
, in itself, is a weighted mean. In addition, the following relations hold:
![]() (13)
(13)
where ![]() has to be intended in statistical sense, according to Bernoulli’s theorem (e.g., [5] , Chapter 2,
has to be intended in statistical sense, according to Bernoulli’s theorem (e.g., [5] , Chapter 2, ![]() 13; [6] , Chapter 2); [7] , Chapter 3,
13; [6] , Chapter 2); [7] , Chapter 3, ![]() 3) and
3) and ![]() is the standard deviation inferred from data related to the meas- ure method,
is the standard deviation inferred from data related to the meas- ure method, ![]() ,
, ![]() (e.g., [3] ).
(e.g., [3] ).
The substitution of Equation (8) into (12) yields the explicit expression of the deviation in terms of the measures, ![]() , as:
, as:
![]() (14)
(14)
where ![]() is the Kronecker symbol.
is the Kronecker symbol.
Using a theorem of statistics (e.g., [5] , Chapter 8; [6] , Chapter 2), the deviation distribution reads:
![]() (15)
(15)
![]() (16)
(16)
by use of Equation (10).
With regard to Equation (11), the weighted mean standard deviation distribution reads:
![]() (17)
(17)
where the random variables, ![]() ,
, ![]() , are no longer independent via Equation (12),
, are no longer independent via Equation (12), ![]() is a normalizing constant and the integration domain,
is a normalizing constant and the integration domain, ![]() , is made of the whole amount of
, is made of the whole amount of ![]() -tuples,
-tuples, ![]() which, via Equations (11), (12), define an interval, centered on
which, via Equations (11), (12), define an interval, centered on![]() , of infinitesimal amplitude equal to
, of infinitesimal amplitude equal to![]() .
.
The deviations, ![]() , are dependent random variables owing to Equation (12). Conversely, any deviation is a function of the measures,
, are dependent random variables owing to Equation (12). Conversely, any deviation is a function of the measures, ![]() , as shown by Equation (14). Accordingly, the weighted mean standard deviation,
, as shown by Equation (14). Accordingly, the weighted mean standard deviation, ![]() , has to be expressed in terms of independent random variables.
, has to be expressed in terms of independent random variables.
Aiming to calculate the multiple integral on the right-hand side of Equation (17), three steps shall be per- formed as in the parent paper [3] , where the weighted mean, ![]() , the weighted mean rms error,
, the weighted mean rms error, ![]() , the weight-
, the weight-
ed mean standard deviation, ![]() , the weighted mean standard deviation distribution,
, the weighted mean standard deviation distribution, ![]() , are to be con- sidered in place of their counterparts related to the arithmetic mean.
, are to be con- sidered in place of their counterparts related to the arithmetic mean.
In dealing with the geometrical framework, for sake of simplicity, the formalism has to be specified as in the parent paper [3] , to which an interested reader is addressed. The extension of useful formulation of analytic geometry to ![]() -spaces, which shall be needed in the following, is outlined in Appendix A.
-spaces, which shall be needed in the following, is outlined in Appendix A.
3. Expression of ![]() in Terms of
in Terms of ![]() and Related Geometrical Framework
and Related Geometrical Framework
The generic deviation, ![]() , in terms of the errors,
, in terms of the errors, ![]() , can be expressed as:
, can be expressed as:
![]() (18)
(18)
![]() (19)
(19)
![]() (20)
(20)
according to the general definition of error. It is apparent the error of the weighted mean equals the weighted mean of the errors. The substitution of Equation (20) into (18) yields:
![]() (21)
(21)
which shows the deviation of a measure from the weighted mean of the measures equals the deviation of the related error from the weighted mean of the errors. The right-hand side relation appearing in Equation (21)
represents a ![]() -plane passing through the origin within a
-plane passing through the origin within a ![]() -space described by the reference frame,
-space described by the reference frame,![]() .
.
The substitution of Equation (21) into (11) after some algebra yields:
![]() (22)
(22)
which represents a one-sheet ![]() -hyperboloid where the polar axis coincides with the coordinate axis,
-hyperboloid where the polar axis coincides with the coordinate axis, ![]() , the equatorial semiaxes and the polar semiaxis read:
, the equatorial semiaxes and the polar semiaxis read:
![]() (23)
(23)
respectively1, and the equator is the intersection between the ![]() -hyperboloid and the principal
-hyperboloid and the principal ![]() -plane,
-plane,![]() .
.
The asymptotes of the ![]() -hyperboloid are generatrixes of a
-hyperboloid are generatrixes of a ![]() -cone where the axis coincides with the coordinate axis,
-cone where the axis coincides with the coordinate axis, ![]() , the vertex coincides with the origin,
, the vertex coincides with the origin, ![]() , and the lateral
, and the lateral ![]() -surface reads:
-surface reads:
![]() (24)
(24)
which may be considered as the equation of the ![]() -cone.
-cone.
The generatrixes lying on the principal plane, ![]() , are expressed as:
, are expressed as:
![]() (25)
(25)
which can be extended to a generic direction, ![]() , by replacing
, by replacing ![]() with
with ![]() .
.
Using general formulation of analytic geometry extended to ![]() -spaces, Equations (108) and (111), it can be ascertained if the angle,
-spaces, Equations (108) and (111), it can be ascertained if the angle, ![]() , formed by the coordinate axis,
, formed by the coordinate axis, ![]() , and the
, and the ![]() -plane, expressed by Equation (21), lies between the minimum and the maximum angle,
-plane, expressed by Equation (21), lies between the minimum and the maximum angle, ![]() ,
, ![]() , formed by the coordinate axis,
, formed by the coordinate axis, ![]() , and related generatrixes of the
, and related generatrixes of the ![]() -cone, expressed by Equation (25). To this respect, a necessary and sufficient condition is that the
-cone, expressed by Equation (25). To this respect, a necessary and sufficient condition is that the ![]() -plane, expressed by Equation (21), is tangent to the
-plane, expressed by Equation (21), is tangent to the ![]() -cone, expressed by Equa- tion (24), along a generatrix,
-cone, expressed by Equa- tion (24), along a generatrix, ![]() , which can be determined via the condition that the generic generatrix,
, which can be determined via the condition that the generic generatrix, ![]() , lies on the
, lies on the ![]() -plane, expressed by Equation (21).
-plane, expressed by Equation (21).
Keeping in mind the ![]() -cone has vertex on the origin and axis coinciding with the coordinate axis,
-cone has vertex on the origin and axis coinciding with the coordinate axis, ![]() , the equation of the generic generatrix reads:
, the equation of the generic generatrix reads:
![]() (26)
(26)
which implies![]() . Accordingly, Equation (24) reduces to:
. Accordingly, Equation (24) reduces to:
![]() (27)
(27)
that is equivalent to:
![]() (28)
(28)
where, in the case under discussion of generatrixes, the square coefficient, ![]() , equals the weighted mean of the square coefficients,
, equals the weighted mean of the square coefficients,![]() . Finally, the substitution of Equation (28) into (26) yields:
. Finally, the substitution of Equation (28) into (26) yields:
![]() (29)
(29)
![]()
1In the special case of the arithmetic mean, ![]() ,
, ![]() , Equation (23) reduces to:
, Equation (23) reduces to:![]() ,
,![]() ;
;![]() , respectively. Then Equation (18) appearing in an earlier investigation [3] has to be related to the polar semiaxis instead of the equatorial semiaxis, see corrigendum in Appendix D.
, respectively. Then Equation (18) appearing in an earlier investigation [3] has to be related to the polar semiaxis instead of the equatorial semiaxis, see corrigendum in Appendix D.
and the condition of parallelism between ![]() and the
and the ![]() -plane, expressed by Equation (21), selects a special generatrix,
-plane, expressed by Equation (21), selects a special generatrix, ![]() , where the above mentioned
, where the above mentioned ![]() -plane is tangent to the
-plane is tangent to the ![]() -cone, expressed by Equation (24).
-cone, expressed by Equation (24).
Owing to Equation (113), the result is:
![]() (30)
(30)
where, in the case under discussion of the generatrix, ![]() , the coefficient,
, the coefficient, ![]() , equals the weighted mean of the coefficients,
, equals the weighted mean of the coefficients,![]() . The further condition, expressed by Equation (28), necessarily implies
. The further condition, expressed by Equation (28), necessarily implies ![]() , as
, as ![]() is needed to define a straight line in the
is needed to define a straight line in the ![]() -space under the validity of Equations (28) and (30).
-space under the validity of Equations (28) and (30).
Accordingly, the generatrix of the ![]() -cone, defined by Equation (24), where the
-cone, defined by Equation (24), where the ![]() -plane, defined by Equation (21), is tangent, can be expressed as:
-plane, defined by Equation (21), is tangent, can be expressed as:
![]() (31)
(31)
which is the ![]() -sector2 of the first and
-sector2 of the first and ![]() th
th ![]() -ant3 of the reference frame,
-ant3 of the reference frame,![]() .
.
Let ![]() be the projection of
be the projection of ![]() on the principal
on the principal ![]() -plane,
-plane,![]() . An explicit expression can be ob- tained erasing the additional coordinate,
. An explicit expression can be ob- tained erasing the additional coordinate, ![]() , from the definition of
, from the definition of![]() , Equation (31). The result is:
, Equation (31). The result is:
![]() (32)
(32)
which is the ![]() -sector of the first and
-sector of the first and ![]() th
th ![]() -ant of the reference frame,
-ant of the reference frame,![]() .
.
Let ![]() be the straight
be the straight ![]() -line, intersection between the
-line, intersection between the ![]() -plane,
-plane, ![]() , defined by Equation (21), and the principal
, defined by Equation (21), and the principal ![]() -plane,
-plane,![]() . The expression of
. The expression of ![]() can be obtained by erasing the additional coordinate,
can be obtained by erasing the additional coordinate, ![]() , from Equation (21). The result is:
, from Equation (21). The result is:
![]() (33)
(33)
where ![]() passes through the origin, as expected.
passes through the origin, as expected.
Let![]() ,
, ![]() , be the angle formed by the straight line,
, be the angle formed by the straight line, ![]() , and the straight
, and the straight ![]() -line,
-line,![]() . The particularization of Equation (107) to the case under discussion
. The particularization of Equation (107) to the case under discussion
![]() yields:
yields:
![]() (34)
(34)
which implies ![]() is normal to
is normal to ![]() if and only if
if and only if![]() ,
, ![]() , to avoid contradiction with Equation (5), or in other words the weighted mean reduces to the arithmetic mean.
, to avoid contradiction with Equation (5), or in other words the weighted mean reduces to the arithmetic mean.
In summary, the weighted mean standard deviation, ![]() , can be expressed as a function of the errors,
, can be expressed as a function of the errors, ![]() , and their weighted mean,
, and their weighted mean, ![]() , via Equation (22), which represents a one-sheet
, via Equation (22), which represents a one-sheet ![]() -hyperboloid
-hyperboloid
where the polar axis coincides with the coordinate axis, ![]() , and the asymptotes are the generatrixes of a
, and the asymptotes are the generatrixes of a ![]() - cone, expressed by Equation (24), with vertex on the origin of the reference frame,
- cone, expressed by Equation (24), with vertex on the origin of the reference frame,![]() . The condi-
. The condi-
tion that the weighted mean of deviations is null, Equation (21), defines a ![]() -plane,
-plane, ![]() , passing through the origin, which is tangent to the above mentioned
, passing through the origin, which is tangent to the above mentioned ![]() -cone at the generatrix,
-cone at the generatrix, ![]() , coinciding with the
, coinciding with the ![]() - sector of the first and
- sector of the first and ![]() th
th ![]() -ant, according to Equation (31). The straight
-ant, according to Equation (31). The straight ![]() -line,
-line, ![]() , intersection between the
, intersection between the ![]() -plane,
-plane, ![]() , and the principal
, and the principal ![]() -plane,
-plane, ![]() , is normal to the projection,
, is normal to the projection, ![]() , of the generatrix,
, of the generatrix, ![]() , on the principal
, on the principal ![]() -plane,
-plane, ![]() , if and only if the weighted mean reduces to the arithmetic mean,
, if and only if the weighted mean reduces to the arithmetic mean, ![]() ,
, ![]() , according to Equation (34). In this limit, the results of the current section reduce to their counterparts in the parent paper [3] .
, according to Equation (34). In this limit, the results of the current section reduce to their counterparts in the parent paper [3] .
4. Expression of ![]() in Terms of
in Terms of![]() , and Related Geometrical Framework
, and Related Geometrical Framework
According to the above results, (i) the points, ![]() , related to a fixed value of the weighted mean standard deviation,
, related to a fixed value of the weighted mean standard deviation, ![]() , lie on a one-sheet
, lie on a one-sheet ![]() -hyperboloid, defined by Equation (22), and (ii) the points,
-hyperboloid, defined by Equation (22), and (ii) the points,
![]() , for which the weighted mean of deviations from the weighted mean is null, lie on a
, for which the weighted mean of deviations from the weighted mean is null, lie on a ![]() -plane, defined by Equation (21). The combination of Equations (20)-(22), yields:
-plane, defined by Equation (21). The combination of Equations (20)-(22), yields:
![]() (35)
(35)
where the polar semiaxis of the ![]() -hyperboloid,
-hyperboloid, ![]() , is defined by Equation (23).
, is defined by Equation (23).
In terms of the errors, ![]() , Equation (35) represents the intersection between the above mentioned
, Equation (35) represents the intersection between the above mentioned ![]() -hyperboloid and
-hyperboloid and ![]() -plane, projected on the principal plane,
-plane, projected on the principal plane, ![]() , as:
, as:
![]() (36)
(36)
where, with regard to the middle side, the single sum is made of ![]() square terms and the double sum of
square terms and the double sum of ![]() mixed products. The
mixed products. The ![]() -line,
-line, ![]() , is the domain of the distribution,
, is the domain of the distribution, ![]() , depending on the weighted mean standard deviation,
, depending on the weighted mean standard deviation, ![]() , via the errors,
, via the errors,![]() .
.
After performing some algebra, Equation (36) may be cast under the equivalent form [6] , Chapter 4:
![]() (37)
(37)
which is a ![]() -quadric where the coefficients of the first-degree terms are null and the axis coincides with the
-quadric where the coefficients of the first-degree terms are null and the axis coincides with the ![]() -sector,
-sector, ![]() , defined by Equation (32). In addition, the quadratic form on the left-hand side of Equation (37) is clearly positive definite.
, defined by Equation (32). In addition, the quadratic form on the left-hand side of Equation (37) is clearly positive definite.
The canonical form of the above mentioned ![]() -quadric can be attained passing from the starting re- ference frame,
-quadric can be attained passing from the starting re- ference frame, ![]() , to the resulting reference frame,
, to the resulting reference frame, ![]() , via rigid rotation around the origin, where the resulting coordinate axes,
, via rigid rotation around the origin, where the resulting coordinate axes, ![]() , coincide with the principal axes of the
, coincide with the principal axes of the ![]() -surface bounded by the
-surface bounded by the ![]() -quadric and, without loss of generality,
-quadric and, without loss of generality, ![]() may be chosen as polar axis. To this aim, the direction cosines must be determined where, in general,
may be chosen as polar axis. To this aim, the direction cosines must be determined where, in general, ![]() is the cosine of the angle formed by the resulting coordinate axis,
is the cosine of the angle formed by the resulting coordinate axis, ![]() , and the starting coordinate axis,
, and the starting coordinate axis, ![]() , within the starting reference frame;
, within the starting reference frame; ![]() is the cosine of the angle formed by the starting coordinate axis,
is the cosine of the angle formed by the starting coordinate axis, ![]() , and the resulting coordinate axis,
, and the resulting coordinate axis, ![]() , within the resulting reference frame; by definition,
, within the resulting reference frame; by definition, ![]() ,
, ![]() ,
,![]() .
.
Following the procedure outlined in the parent paper [3] , the direction cosines, ![]() , related to the coordinate axis,
, related to the coordinate axis, ![]() , read:
, read:
![]() (38)
(38)
conformly to Equation (32). The remaining coordinate axes, ![]() , can be arbitrarily selected in that they are related to the
, can be arbitrarily selected in that they are related to the ![]() principal axes of the
principal axes of the ![]() -ellipse, centered on the origin and normal to the coordinate axis,
-ellipse, centered on the origin and normal to the coordinate axis,![]() . For this reason, the starting and the resulting reference frame are not needed to be con-
. For this reason, the starting and the resulting reference frame are not needed to be con-
gruent provided the Jacobian determinant is orthogonal,![]() .
.
The term, ![]() , appearing in Equation (37), within the resulting reference frame can be expressed as:
, appearing in Equation (37), within the resulting reference frame can be expressed as:
![]() (39)
(39)
which is independent of the coordinate, ![]() , related to the polar axis of the
, related to the polar axis of the ![]() -quadric, as
-quadric, as![]() , owing to Equation (38). The explicit expression of the table of direction cosines,
, owing to Equation (38). The explicit expression of the table of direction cosines, ![]() , reads [6] , Chapter 4:
, reads [6] , Chapter 4:
![]() (40)
(40)
where its counterpart related to ![]() -planes,
-planes, ![]() , can be obtained from
, can be obtained from ![]() along the following steps: (i) erase the
along the following steps: (i) erase the ![]() -th line and the
-th line and the ![]() -th column; and (ii) change all the elements of the first column from
-th column; and (ii) change all the elements of the first column from ![]() to
to![]() .
.
The ![]() -quadric, expressed by Equation (37), may be cast under the equivalent form:
-quadric, expressed by Equation (37), may be cast under the equivalent form:
![]() (41)
(41)
where the contribution related to the coordinate axis, ![]() , has been written separately. The substitution of Equation (39) into (41) yields the equation of the
, has been written separately. The substitution of Equation (39) into (41) yields the equation of the ![]() -quadric in the resulting reference frame,
-quadric in the resulting reference frame, ![]() , as:
, as:
![]() (42)
(42)
where the coefficients, ![]() ,
, ![]() ,
, ![]() , have the same formal espression as their counterparts,
, have the same formal espression as their counterparts, ![]() ,
, ![]() , related to the
, related to the ![]() -quadric in the reference frame,
-quadric in the reference frame, ![]() , but different numerical value due to the presence of the coefficients,
, but different numerical value due to the presence of the coefficients, ![]() , conditioned by Equation (5).
, conditioned by Equation (5).
The coordinate, ![]() , does not appear in Equation (42), which necessarily implies the
, does not appear in Equation (42), which necessarily implies the ![]() -quadric is the orthogonal section of a
-quadric is the orthogonal section of a ![]() -cylinder, normal to the polar axis,
-cylinder, normal to the polar axis,![]() . The presence of mixed products,
. The presence of mixed products, ![]() , in Equation (42), necessarily implies the principal axes of the orthogonal section are not coincident with the coordinate axes,
, in Equation (42), necessarily implies the principal axes of the orthogonal section are not coincident with the coordinate axes,![]() . Then a rigid rotation of the reference frame,
. Then a rigid rotation of the reference frame, ![]() , around the coordinate axis,
, around the coordinate axis, ![]() , is needed to make the principal axes of the
, is needed to make the principal axes of the ![]() -ellipse coincide with the remaining coordinate axes. To this aim, three different attempts shall be exploited. In the special case where the weighted mean reduces to the arithmetic mean, the
-ellipse coincide with the remaining coordinate axes. To this aim, three different attempts shall be exploited. In the special case where the weighted mean reduces to the arithmetic mean, the ![]() -ellipse reduces to a
-ellipse reduces to a ![]() -circle and a simpler procedure can be used [3] .
-circle and a simpler procedure can be used [3] .
4.1. First Attempt
The explicit expression of the coefficients, ![]() ,
,![]() ;
;![]() ,
,![]() ; appearing in Equation (42), may be deter- mined along the following steps [6] , Chapter 4.
; appearing in Equation (42), may be deter- mined along the following steps [6] , Chapter 4.
Particularize Equations (39) and (40) to the special case, ![]() ,
, ![]() , and express the differences,
, and express the differences, ![]() , in terms of direction cosines,
, in terms of direction cosines, ![]() ,
, ![]() , and resulting coordinates,
, and resulting coordinates, ![]() ,
,![]() .
.
Express the square differences, ![]() , by writing the terms in
, by writing the terms in ![]() separately from the remaining ones.
separately from the remaining ones.
Substitute the above expressions into the second term on the right-hand side of Equation (41) particularized to the case under discussion,![]() .
.
Substitute the result into the right-hand side of Equation (42) particularized to the case under discussion, ![]() , and compare with the middle side, term by term in
, and compare with the middle side, term by term in ![]() and
and![]() .
.
The result is [6] , Chapter 4:
![]() (43a)
(43a)
![]() (43b)
(43b)
![]() (43c)
(43c)
![]() (43d)
(43d)
and the recursive application of Equations (43a) and (43c), with due account taken of (43b) and (43d), after some algebra yields:
![]() (44a)
(44a)
![]() (44b)
(44b)
with regard to ![]() -spaces, and:
-spaces, and:
![]() (45a)
(45a)
![]() (45b)
(45b)
with regard to ![]() -planes.
-planes.
Finally, the symmetry relations:
![]() (46)
(46)
are due to the appearence of related coefficients in the expression of a quadratic form on the middle side of Equation (42).
With regard to the ![]() -quadric, expressed by Equation (42), the above results do not allow finding a table of direction cosines, counterpart of Equation (40), related to a rigid rotation of the reference frame,
-quadric, expressed by Equation (42), the above results do not allow finding a table of direction cosines, counterpart of Equation (40), related to a rigid rotation of the reference frame, ![]() , around the coordinate axis,
, around the coordinate axis, ![]() , yielding a new reference frame where the
, yielding a new reference frame where the ![]() -quadric is expressed in canonical form. To this aim, general transformations of the reference frame have to be considered, including orthogonal transformations as special cases. That is the case of linear transformations, which imply matrix operations (e.g., [8] , Tome III, Part I, Chapter II).
-quadric is expressed in canonical form. To this aim, general transformations of the reference frame have to be considered, including orthogonal transformations as special cases. That is the case of linear transformations, which imply matrix operations (e.g., [8] , Tome III, Part I, Chapter II).
4.2. Second Attempt
With regard to the ![]() -quadric, expressed by Equation (36), the matrix of the quadratic form on the left- hand side reads:
-quadric, expressed by Equation (36), the matrix of the quadratic form on the left- hand side reads:
![]() (47)
(47)
where the determinant, ![]() , is the discriminant of the quadratic form, and the elements are real numbers in
, is the discriminant of the quadratic form, and the elements are real numbers in
the case under consideration. Due to the symmetry of the elements of quadratic forms, ![]() concerning Equation (36), the matrix of the quadratic form coincides with the transpose matrix,
concerning Equation (36), the matrix of the quadratic form coincides with the transpose matrix,![]() .
.
Let ![]() be a matrix of order,
be a matrix of order, ![]() , related to an assigned linear transformation from a starting reference frame,
, related to an assigned linear transformation from a starting reference frame, ![]() , to a resulting reference frame,
, to a resulting reference frame, ![]() , as:
, as:
![]() (48)
(48)
where the coordinates define the same vector in the appropriate reference frame.
The linear transformation reduces to an orthogonal transformation if and only if the elements of the matrix, ![]() , satisfy the orthogonality conditions (e.g., [8] , Tome III, Part I, Chapter II):
, satisfy the orthogonality conditions (e.g., [8] , Tome III, Part I, Chapter II):
![]() (49)
(49)
concerning lines and columns, respectively.
According to the theory of linear transformations, the expression of the quadratic form on the left-hand side of Equation (36), in canonical form, takes place via the determination of an orthogonal matrix, ![]() , which makes the product matrix,
, which makes the product matrix, ![]() ,
, ![]() inverse matrix of
inverse matrix of![]() , be diagonal. Then
, be diagonal. Then ![]() is the matrix of the linear transformation of interest. The above procedure implies the solution of an eigenvalue problem (e.g., [8] , Tome III, Part I, Chapter II).
is the matrix of the linear transformation of interest. The above procedure implies the solution of an eigenvalue problem (e.g., [8] , Tome III, Part I, Chapter II).
With regard to the ![]() -quadric, expressed by Equation (36), the eigenvalue equation is:
-quadric, expressed by Equation (36), the eigenvalue equation is:
![]() (50)
(50)
where the matrix, ![]() , is defined by Equation (47); the matrix,
, is defined by Equation (47); the matrix, ![]() , reads
, reads![]() ,
, ![]() unit matrix of order,
unit matrix of order,![]() ; and
; and ![]() is the variable. After a lot of determinant algebra, Equation (50) may be cast under the explicit form [6] , Chapter 4:
is the variable. After a lot of determinant algebra, Equation (50) may be cast under the explicit form [6] , Chapter 4:
![]() (51a)
(51a)
![]() (51b)
(51b)
which is an algebraic equation of degree, ![]() , as [6] , Chapter 4:
, as [6] , Chapter 4:
![]() (52)
(52)
where the coefficients, regardless of an inessential multiplicative constant, may be expressed as [6] , Chapter 4:
![]() (53)
(53)
and, in particular:
![]() (54)
(54)
where the last relation comes from Equation (47) after summation of all lines or columns on a selected line or column which, via Equation (5), yields a line or column made of null elements implying, in turn, a null deter- minant.
With regard to Equation (51a), the existence of ![]() solutions (eigenvalues) is ensured by the fundamental theorem of algebra, where all the solutions are real in the case under discussion, as linear orthogonal transfor- mations are involved. In particular, the appearence of a null solution, let it be
solutions (eigenvalues) is ensured by the fundamental theorem of algebra, where all the solutions are real in the case under discussion, as linear orthogonal transfor- mations are involved. In particular, the appearence of a null solution, let it be![]() , implies the
, implies the ![]() - quadric, expressed by Equation (36), is degenerate to the lateral
- quadric, expressed by Equation (36), is degenerate to the lateral ![]() -surface of a
-surface of a ![]() -cylinder.
-cylinder.
According to the general theory (e.g., [8] , Tome III, Part I, Chapter II; [9] , Vol. I, Chapter 10), a generic quadratic form where the coefficients are real, as:
![]() (55)
(55)
can be reduced to the canonical form:
![]() (56)
(56)
by use of a convenient linear orthogonal transformation, where![]() , are the eigenvalues of the matrix of the quadratic form.
, are the eigenvalues of the matrix of the quadratic form.
In the case under discussion, with regard to Equations (23), (47), (51a), Equation (56) reduces to:
![]() (57)
(57)
which defines a ![]() -ellipse, orthogonal section of a
-ellipse, orthogonal section of a ![]() -cylinder, where the coordinate axes,
-cylinder, where the coordinate axes, ![]() , coincide with the principal axes of the
, coincide with the principal axes of the ![]() -ellipse, and the coordinate axis,
-ellipse, and the coordinate axis, ![]() , coincides with the axis of the
, coincides with the axis of the ![]() -cylinder4.
-cylinder4.
The semiaxes of the ![]() -ellipse are:
-ellipse are:
![]() (58a)
(58a)
![]() (58b)
(58b)
accordingly, the features of the ![]() -ellipse depend on the eigenvalues only. In particular, the axis ratios,
-ellipse depend on the eigenvalues only. In particular, the axis ratios, ![]() ,
, ![]() , depend on the eigenvalues only, which implies different
, depend on the eigenvalues only, which implies different ![]() -ellipses, defined by Equation (57), are homotetic.
-ellipses, defined by Equation (57), are homotetic.
It is apparent from Equation (51a) that the determination of eigenvalues is not an easy matter in the general case,![]() . For this reason, a different attempt has to be exploited, where a canonical expression of the quadratic form is inferred regardless of the eigenvalues. To this aim, general linear transformations shall be used, which reduce to orthogonal linear transformations as a special case.
. For this reason, a different attempt has to be exploited, where a canonical expression of the quadratic form is inferred regardless of the eigenvalues. To this aim, general linear transformations shall be used, which reduce to orthogonal linear transformations as a special case.
4.3. Third Attempt
![]()
4For sake of convenience, the polar axis here is denoted as ![]() instead of
instead of ![]() as done earlier in the current section.
as done earlier in the current section.
With regard to the quadratic form, expressed by Equation (55), let ![]() real linear forms be considered, among which
real linear forms be considered, among which ![]() are linearly independent,
are linearly independent, ![]() , as:
, as:
![]() (59)
(59)
where![]() ,
, ![]() , are coefficients satisfying the relation:
, are coefficients satisfying the relation:
![]() (60)
(60)
where, in turn, ![]() ,
, ![]() , are coefficients characterized by the following properties.
, are coefficients characterized by the following properties.
(1) The number of positive, ![]() , and negative,
, and negative, ![]() , coefficients, equals the rank of the matrix of the quadratic form,
, coefficients, equals the rank of the matrix of the quadratic form,![]() .
.
(2) With regard to the whole set of linear transformations, defined by Equation (60), the number of negative
coefficients, ![]() , positive coefficients,
, positive coefficients, ![]() , and null coefficients,
, and null coefficients, ![]() , remains unchanged
, remains unchanged
for any element of the set, according to the theorem of inertia of quadratic forms (e.g., [8] , Tome III, Part I, Chapter II; [9] , Vol. I, Chapter 10).
Further considerations shall be restricted to linear transformations defined by Jacobi formulae. For additional details, an interested reader is addressed to Appendix B. Using the Jacobi formulae, Equation (60) reduces to:
![]() (61)
(61)
which is valid in general.
In the case of interest, ![]() , the coefficients of the quadratic form, defined by Equation (55), take the explicit form:
, the coefficients of the quadratic form, defined by Equation (55), take the explicit form:
![]() (62)
(62)
according to Equation (47), and Equation (115) reduces to (47). Finally, Equation (114) reads:
![]() (63)
(63)
and Equations (116) take the explicit form [6] , Chapter 4:
![]() (64a)
(64a)
![]() (64b)
(64b)
in addition, Equation (64a) may be expressed via a recursive formula, as:
![]() (65a)
(65a)
![]() (65b)
(65b)
where inequalities are due to the constraints, ![]() ,
, ![]() , conformly to Equation (5).
, conformly to Equation (5).
Let ![]() be a reference frame where the quadratic form on the left-hand side of Equation (36) can be expressed as a linear combination of pure quadratic terms, i.e. the coefficients of mixed products are null. The particularization of Equation (117) to the case under discussion yields:
be a reference frame where the quadratic form on the left-hand side of Equation (36) can be expressed as a linear combination of pure quadratic terms, i.e. the coefficients of mixed products are null. The particularization of Equation (117) to the case under discussion yields:
![]() (66a)
(66a)
![]() (66b)
(66b)
where the rank of the matrix, ![]() , defined by Equation (47), is
, defined by Equation (47), is![]() , conformly to Equations (53) and (54), which implies the appearence of
, conformly to Equations (53) and (54), which implies the appearence of ![]() independent quadratic terms,
independent quadratic terms, ![]() ,
, ![]() , in the expression of the above mentioned quadratic form.
, in the expression of the above mentioned quadratic form.
By use of Jacobi formulae, Equations (118), the ![]() -quadric, expressed by Equations (36) and (23), may be cast under the canonical form:
-quadric, expressed by Equations (36) and (23), may be cast under the canonical form:
![]() (67)
(67)
which defines a ![]() -ellipse, orthogonal section of a
-ellipse, orthogonal section of a ![]() -cylinder, where the coordinate axes,
-cylinder, where the coordinate axes, ![]() , coincide with the principal axes of the
, coincide with the principal axes of the ![]() -ellipse, and the coordinate axis,
-ellipse, and the coordinate axis, ![]() , coincides with the axis of the
, coincides with the axis of the ![]() -cylinder.
-cylinder.
4.4. Explicit Expressions
With regard to the ![]() -quadric, expressed by Equations (36) and (23), the canonical form in terms of eigen- values, Equation (57), can be equated to its counterpart inferred from Jacobi formulae, Equation (67), as:
-quadric, expressed by Equations (36) and (23), the canonical form in terms of eigen- values, Equation (57), can be equated to its counterpart inferred from Jacobi formulae, Equation (67), as:
![]() (68)
(68)
where the resulting coordinates, ![]() , depend on the starting coordinates,
, depend on the starting coordinates, ![]() , via an orthogonal trans- formation:
, via an orthogonal trans- formation:
![]() (69)
(69)
which implies a rigid rotation around the origin in a ![]() -space, together with a possible change in chirality, according to the sign of the Jacobian determinant.
-space, together with a possible change in chirality, according to the sign of the Jacobian determinant.
Let ![]() a matrix of order,
a matrix of order, ![]() , whose elements are
, whose elements are![]() ,
, ![]() ,
,![]() . Accordingly, the matrix of the orthogonal transformation, expressed by Equation (69), is:
. Accordingly, the matrix of the orthogonal transformation, expressed by Equation (69), is:
![]() (70)
(70)
in terms of eigenvalues of the matrix, ![]() , of the quadratic form on the left-hand side of Equation (36).
, of the quadratic form on the left-hand side of Equation (36).
The semiaxes of the ![]() -ellipse, orthogonal section of the
-ellipse, orthogonal section of the ![]() -cylinder, defined by Equation (57), via Equation (67) can be expressed as:
-cylinder, defined by Equation (57), via Equation (67) can be expressed as:
![]() (71a)
(71a)
![]() (71b)
(71b)
where the leading principal minors, ![]() , are positive according to Equations (65) which, in addition, implies semiaxis inequalities.
, are positive according to Equations (65) which, in addition, implies semiaxis inequalities.
In the special case where the weighted mean reduces to the arithmetic mean, ![]() ,
, ![]() , conformly to Equation (6), Equations (64) reduce to:
, conformly to Equation (6), Equations (64) reduce to:
![]() (72)
(72)
and the substitution of Equation (72) into (71a) yields:
![]() (73a)
(73a)
![]() (73b)
(73b)
which shows the current procedure is conceptually different from its counterpart related to the arithmetic mean, where the orthogonal section of the ![]() -cylinder corresponds to a
-cylinder corresponds to a ![]() -circle [3] .
-circle [3] .
The apparent discrepancy can be explained in the following way. Jacobi formulae are grounded on non orthogonal linear transformations which, in themselves, imply changes in metric relations. On the other hand, eigenvalue equations are grounded on orthogonal linear transformations which, in themselves, imply conserva- tion of metric relations.
According to the general theory of linear transformations and quadratic forms (e.g., [8] , Tome III, Part I, Chapter II; [9] , Vol. I, Chapter 10), the coefficients of the eigenvalue equation, expressed by Equations (51)-(52), regardless of an inessential multiplicative constant, coincide with the invariants of the matrix, ![]() , expressed by Equation (47), with regard to the quadratic form on the left-hand side of Equation (36). The above mentioned
, expressed by Equation (47), with regard to the quadratic form on the left-hand side of Equation (36). The above mentioned
invariants, ![]() ,
, ![]() , remain unchanged for any matrix inferred from
, remain unchanged for any matrix inferred from ![]() via a similarity transfor- mation,
via a similarity transfor- mation, ![]() ,
, ![]() ,
, ![]() nonsingular matrix. The matrix,
nonsingular matrix. The matrix, ![]() , is symmetric in that it relates to a quadratic form where the coefficients, in the case under consideration, are real, which implies the matrix,
, is symmetric in that it relates to a quadratic form where the coefficients, in the case under consideration, are real, which implies the matrix, ![]() , or
, or![]() , is necessarily orthogonal.
, is necessarily orthogonal.
Let![]() ,
, ![]() , be singular matrixes defined as:
, be singular matrixes defined as:
![]() (74)
(74)
![]() (75)
(75)
where![]() , are starting coordinates and
, are starting coordinates and![]() , are resulting coordinates of a reference frame where the matrix of the quadratic form is diagonal via an orthogonal transformation using the matrix,
, are resulting coordinates of a reference frame where the matrix of the quadratic form is diagonal via an orthogonal transformation using the matrix,![]() . Ac- cordingly, the following relations hold:
. Ac- cordingly, the following relations hold:
![]() (76)
(76)
where the elements, ![]() , of the matrix,
, of the matrix, ![]() , can be determined by solving
, can be determined by solving ![]() systems of equations, extracted from the condition that the matrix,
systems of equations, extracted from the condition that the matrix, ![]() , is diagonal, and from the knowledge of the eigenvalues.
, is diagonal, and from the knowledge of the eigenvalues.
According to the general theory of linear transformations and quadratic forms (e.g., [8] , Tome III, Part I, Chapter II; [9] , Vol. I, Chapter 10), the above mentioned systems of equations read:
![]() (77)
(77)
where ![]() are elements of the matrix,
are elements of the matrix, ![]() , and
, and ![]() is the
is the ![]() -th eigenvalue on a total of
-th eigenvalue on a total of![]() :
:![]() .
.
The validity of the eigenvalue equation, expressed by Equation (51), necessarily implies the determinant of the system, defined by Equation (77), is null in the case of interest or, in other words, the existence of infinite solutions. On the other hand, the condition of unit norm:
![]() (78)
(78)
allows the selection of a special solution (regardless of the sign) among infinite others.
The particularization of the system, defined by Equation (77), to the case of interest, ![]() , and to the eigenvalue,
, and to the eigenvalue, ![]() , reads:
, reads:
![]() (79)
(79)
which is equivalent to:
![]() (80)
(80)
accordingly, the following relations hold:
![]() (81)
(81)
and the substitution of Equation (81) into (78), particularized to![]() , yields:
, yields:
![]() (82)
(82)
where the positive sign relates to Equation (38).
Using the definition of matrix product, Equation (76) takes the explicit form:
![]() (83a)
(83a)
![]() (83b)
(83b)
where, in the case under discussion of orthogonal linear transformations, the inverse matrix, ![]() , is inferred from the matrix,
, is inferred from the matrix, ![]() , simply by exchanging lines with columns and vice versa. With regard to the coordinate axis,
, simply by exchanging lines with columns and vice versa. With regard to the coordinate axis, ![]() , the combination of Equations (82) and (83a) yields:
, the combination of Equations (82) and (83a) yields:
![]() (84)
(84)
which is related to the eigenvalue, ![]() , then the coordinate axis,
, then the coordinate axis, ![]() , coincides with the axis of the
, coincides with the axis of the ![]() - cylinder, defined by Equation (56).
- cylinder, defined by Equation (56).
In summary, the weighted mean standard deviation distribution can be expressed as a function of the errors, ![]() , as:
, as:
![]() (85)
(85)
where ![]() is a normalizing constant,
is a normalizing constant, ![]() the integration domain, expressed by Equation (36) which, turned into canonical expression, Equation (56), represents an infinitely thin
the integration domain, expressed by Equation (36) which, turned into canonical expression, Equation (56), represents an infinitely thin ![]() -cylindrical corona of infinite height, axis coinciding with the coordinate axis,
-cylindrical corona of infinite height, axis coinciding with the coordinate axis, ![]() , orthogonal section expressed by Equation (57), that is an in- finitely thin, homotetic
, orthogonal section expressed by Equation (57), that is an in- finitely thin, homotetic ![]() -elliptical corona whose semiaxes are defined by Equation (71).
-elliptical corona whose semiaxes are defined by Equation (71).
Finally, ![]() ,
, ![]() , are error distributions expressed via Equation (1) as:
, are error distributions expressed via Equation (1) as:
![]() (86)
(86)
where ![]() is the error of the generic measure,
is the error of the generic measure, ![]() , and
, and ![]() is the rms error of the related distribution.
is the rms error of the related distribution.
5. Expression of ![]() in Terms of
in Terms of![]() ,
, ![]() , and Related Geometrical Framework
, and Related Geometrical Framework
The substitution of Equations (10) and (86) into (85), after little algebra yields:
![]() (87)
(87)
the special case, ![]() , is shown in Figure 1 [6] , Chapter 4.
, is shown in Figure 1 [6] , Chapter 4.
With regard to the resulting reference frame, ![]() , the substitution of Equation (83b) into (84) yields:
, the substitution of Equation (83b) into (84) yields:
![]() (88)
(88)
which necessarily implies the following:
![]() (89)
(89)
conformly to Equation (82).
The weighted mean of the errors, expressed by Equation (20), by use of Equations (83b) and (89), after little algebra may be cast under the equivalent form:
![]() (90)
(90)
and the substitution of Equations (23) and (90) into (35) yields:
![]() (91)
(91)
in terms of a single resulting coordinate,![]() .
.
![]()
Figure 1. The integration domain, ![]() , with regard to the reference frame,
, with regard to the reference frame, ![]() , in the special case,
, in the special case,![]() . The straight lines,
. The straight lines, ![]() ,
, ![]() , are the axes of infinitely thin bands, whose bounderies are defined by the straight lines,
, are the axes of infinitely thin bands, whose bounderies are defined by the straight lines,![]() ;
;![]() . The integration domain,
. The integration domain, ![]() , is defined by the above mentioned bands.
, is defined by the above mentioned bands.
The transformation, ![]() , appearing in Equation (76), is orthogonal with unit norm, which implies the squared Jacobian of the transformation also equals unity,
, appearing in Equation (76), is orthogonal with unit norm, which implies the squared Jacobian of the transformation also equals unity, ![]() , conformly to Equation (76).
, conformly to Equation (76).
With regard to the resulting reference frame, ![]() , after a change of variables (e.g., [8] , Chapter III,
, after a change of variables (e.g., [8] , Chapter III, ![]() 4.10; [6] , Chapter 4) by use of Equation (91) keeping in mind
4.10; [6] , Chapter 4) by use of Equation (91) keeping in mind![]() , Equation (87) translates into5
, Equation (87) translates into5
![]() (92)
(92)
where the integration domain, ![]() , is an infinitely thin, homotetic
, is an infinitely thin, homotetic ![]() -cylindrical corona with axis,
-cylindrical corona with axis, ![]() , and semiaxes defined by Equation (71). The special case,
, and semiaxes defined by Equation (71). The special case, ![]() , is shown in Figure 2 [6] , Chapter 4.
, is shown in Figure 2 [6] , Chapter 4.
With regard to the principal ![]() -plane,
-plane, ![]() , the
, the ![]() -surface of the
-surface of the ![]() -ellipse of semiaxes,
-ellipse of semiaxes, ![]() ,
, ![]() , is:
, is:
![]() (93a)
(93a)
![]() (93b)
(93b)
![]()
5A negative Jacobian determinant, ![]() , relates to a resulting reference frame,
, relates to a resulting reference frame, ![]() , which is not congruent to the starting reference frame,
, which is not congruent to the starting reference frame, ![]() , and the negative sign is compensated by an even number of sign changes within the integrals appearing in Equation (87).
, and the negative sign is compensated by an even number of sign changes within the integrals appearing in Equation (87).
where ![]() is the Euler Gamma function, which satisfies the following relations (e.g., [10] , Chapter 39,
is the Euler Gamma function, which satisfies the following relations (e.g., [10] , Chapter 39, ![]() 39.6):
39.6):
![]()
Figure 2. The integration domain, ![]() , with regard to the reference frame,
, with regard to the reference frame, ![]() , in the special case,
, in the special case,![]() . The straight lines,
. The straight lines, ![]() ,
, ![]() , are the axes of infinitely thin bands, whose boundaries are defined by the straight lines,
, are the axes of infinitely thin bands, whose boundaries are defined by the straight lines,![]() ;
;![]() ; according to Equations (51) and (57). The integration domain,
; according to Equations (51) and (57). The integration domain, ![]() , is defined by the above mentioned bands.
, is defined by the above mentioned bands.
![]() (94a)
(94a)
![]() (94b)
(94b)
![]() (94c)
(94c)
![]() (94d)
(94d)
![]() (94e)
(94e)
and the particularization of Equation (93a) to the simplest cases, ![]() yields:
yields:
![]() (95)
(95)
with regard to points, segments, ellipses, ellipsoids, respectively.
In particular, ![]() implies a single deviation from the mean,
implies a single deviation from the mean, ![]() conformly to Equation (12), then
conformly to Equation (12), then ![]() via Equations (11) and (23), and the semiaxes of the 0-ellipse cannot be defined using Equation (58).
via Equations (11) and (23), and the semiaxes of the 0-ellipse cannot be defined using Equation (58).
Accordingly, the 0-ellipse coincides with the origin of the reference frame, ![]() , the 0-surface of which is clearly null. For this reason, the undetermined expression,
, the 0-surface of which is clearly null. For this reason, the undetermined expression, ![]() as
as![]() , appearing in Equation (93a),
, appearing in Equation (93a),
may safely be put equal to 0, hence![]() , in agreement with Equation (95). On the other hand, Equations (22), (24), (25), lose their validity for
, in agreement with Equation (95). On the other hand, Equations (22), (24), (25), lose their validity for![]() .
.
The ![]() -surface of an infinitely thin
-surface of an infinitely thin ![]() -elliptical corona can be determined by differentiating both sides of Equation (93a). The result is:
-elliptical corona can be determined by differentiating both sides of Equation (93a). The result is:
![]() (96)
(96)
which is independent of the reference frame.
In summary, the weighted mean standard deviation distribution, ![]() , may be expressed as a multiple integral where the integration domain,
, may be expressed as a multiple integral where the integration domain, ![]() , is an infinitely thin
, is an infinitely thin ![]() -cylindrical corona where the axis coincides with the coordinate axis,
-cylindrical corona where the axis coincides with the coordinate axis, ![]() , and the semiaxes of the
, and the semiaxes of the ![]() -elliptical orthogonal section are defined by Equation (58). The result, expressed by Equation (92), after some algebra takes the form:
-elliptical orthogonal section are defined by Equation (58). The result, expressed by Equation (92), after some algebra takes the form:
![]() (97)
(97)
where the integration domain of the ordinary and the multiple integral are the axis and the ![]() -elliptical orthogonal section, respectively, of the
-elliptical orthogonal section, respectively, of the ![]() -cylindrical corona, hence
-cylindrical corona, hence![]() .
.
6. The Solution
The substitution of Equations (58) and (96) into (97), after long but stimulating algebra yields [6] , Chapter 4:
![]() (98)
(98)
where due account has been paid to Equations (94a), (94c), together with the normalizing condition:
![]() (99)
(99)
which, after integration as outlined above via Equation (97), is equivalent to:
![]() (100)
(100)
according to Equation (98) that, in addition, can be related to a chi square distribution with ![]() degrees of freedom.
degrees of freedom.
The distribution, ![]() , expressed by Equation (98), is formally identical to its counterpart related to the arithmetic mean,
, expressed by Equation (98), is formally identical to its counterpart related to the arithmetic mean, ![]() , expressed in an earlier attempt [3] . Accordingly, related results can be extended to the weighted mean provided the random variable,
, expressed in an earlier attempt [3] . Accordingly, related results can be extended to the weighted mean provided the random variable, ![]() , and the rms error,
, and the rms error, ![]() , are replaced by
, are replaced by ![]() and
and![]() , respectively.
, respectively.
The expected values, ![]() ,
, ![]() , take the expression:
, take the expression:
![]() (101)
(101)
![]() (102)
(102)
and the rms error of the weighted mean standard deviation distribution, ![]() , takes the expression:
, takes the expression:
![]() (103)
(103)
which, for![]() , is approximated by the asymptotic formula:
, is approximated by the asymptotic formula:
![]() (104)
(104)
where the ratio, ![]() , is understimated [3] .
, is understimated [3] .
In summary, the weighted mean standard deviation distribution is explicitly expressed by Equation (98) and related expectation values, ![]() ,
, ![]() , and rms error,
, and rms error, ![]() , are expressed by Equations (101)-(103), respec-
, are expressed by Equations (101)-(103), respec-
tively. Finally, an asymptotic formula involving ![]() is shown by Equation (104), where the value is unders- timated. The above mentioned results, Equations (101)-(104), have the same formal expression with respect to their counterparts in the special case where the weighted mean reduces to the arithmetic mean [3] .
is shown by Equation (104), where the value is unders- timated. The above mentioned results, Equations (101)-(104), have the same formal expression with respect to their counterparts in the special case where the weighted mean reduces to the arithmetic mean [3] .
7. Conclusions
![]()
6In ordinary plane and space, ellipses and triaxial ellipsoids are referred to as having biaxial and triplanar symmetry, respectively. By extension to ![]() -planes and
-planes and ![]() -spaces,
-spaces, ![]() -ellipses and
-ellipses and ![]() -ellipsoids can be referred to as having
-ellipsoids can be referred to as having ![]() -axial and
-axial and ![]() -planar symmetry, respectively.
-planar symmetry, respectively.
The weighted mean standard deviation distribution and related parameters have been determined following a procedure where the geometrical framework is clearly shown using typical formulation generalized to ![]() -spaces. After exploiting three different attempts, the integration has been performed via a change of reference frame, where the integration domain turns out to be an infinitely thin, homotetic
-spaces. After exploiting three different attempts, the integration has been performed via a change of reference frame, where the integration domain turns out to be an infinitely thin, homotetic ![]() -cylindrical corona that is
-cylindrical corona that is ![]() - axially symmetric6 with respect to a coordinate axis.
- axially symmetric6 with respect to a coordinate axis.
In the special case where the weighted mean reduces to the arithmetic mean, the results of the current paper reduce to their counterparts (where present) in the parent paper [3] . In particular, the integration domain reduces to an infinitely thin ![]() -cylindrical corona where the symmetry axis coincides with a coordinate axis and the orthogonal section is a
-cylindrical corona where the symmetry axis coincides with a coordinate axis and the orthogonal section is a ![]() -circle. Accordingly,
-circle. Accordingly, ![]() eigenvalues are coincident and the remaining one is null.
eigenvalues are coincident and the remaining one is null.
Acknowledgements
A more extended version of the current attempt appears in a specific textbook [6] by the author.
Appendix
A. Analytic Geometry Formulation Extended to ![]() -Spaces
-Spaces
Analytic geometry formulation extended to ![]() -spaces, used throughout the text, is outlined below. It shall be intended, but not explicitly mentioned where unnecessary, that the reference frame is
-spaces, used throughout the text, is outlined below. It shall be intended, but not explicitly mentioned where unnecessary, that the reference frame is![]() .
.
Let ![]() and
and ![]() be a generic straight line and
be a generic straight line and ![]() -plane, respectively, defined as:
-plane, respectively, defined as:
![]() (105)
(105)
![]() (106)
(106)
where ![]() is a fixed point belonging to
is a fixed point belonging to ![]() and
and![]() ,
,![]() ;
;![]() ,
, ![]() , A; are specified coefficients.
, A; are specified coefficients.
Let![]() ,
, ![]() , be the angle formed by the straight line and the
, be the angle formed by the straight line and the ![]() -plane. Related trigonometric functions can be inferred from the explicit expression of the sine, as:
-plane. Related trigonometric functions can be inferred from the explicit expression of the sine, as:
![]() (107)
(107)
which, in the case of interest![]() , reduces to:
, reduces to:
![]() (108)
(108)
where ![]() coincides with the coordinate axis,
coincides with the coordinate axis, ![]() , and
, and ![]() passes through the origin.
passes through the origin.
Let ![]() be a generic straight line, defined as:
be a generic straight line, defined as:
![]() (109)
(109)
where ![]() is a fixed point belonging to
is a fixed point belonging to ![]() and
and![]() ,
, ![]() , are specified coeffi- cients.
, are specified coeffi- cients.
Let![]() ,
, ![]() , be the angle formed by the straight lines,
, be the angle formed by the straight lines, ![]() and
and![]() . Related trigonometric functions can be inferred from the explicit expression of the cosine, as:
. Related trigonometric functions can be inferred from the explicit expression of the cosine, as:
![]() (110)
(110)
which, in the case of interest (![]() ,
,![]() ;
;![]() ;
;![]() ;
;![]() ,
,![]() ;
;![]() ), reduces to:
), reduces to:
![]() (111)
(111)
where ![]() is the generatrix, lying on the principal plane,
is the generatrix, lying on the principal plane, ![]() , of the
, of the ![]() -cone, defined by Equation (24), and
-cone, defined by Equation (24), and ![]() coincides with the coordinate axis,
coincides with the coordinate axis,![]() .
.
The condition of parallelism between the straight line, ![]() , and the
, and the ![]() -plane,
-plane, ![]() , reads:
, reads:
![]() (112)
(112)
which, in the case of interest![]() , reduces to:
, reduces to:
![]() (113)
(113)
where ![]() is the
is the ![]() -plane, defined by Equation (21).
-plane, defined by Equation (21).
B. Jacobi Formulae
With regard to the quadratic form, defined by Equation (51), let the following linear forms be defined:
![]() (114)
(114)
where ![]() are elements of the matrix of the quadratic form:
are elements of the matrix of the quadratic form:
![]() (115)
(115)
and let the leading principal minors of the discriminant of the quadratic form, ![]() , be defined as:
, be defined as:
![]() (116a)
(116a)
![]() (116b)
(116b)
where ![]() relates to an empty (i.e. with no element) minor, whose value is conventionally assumed equal to unity. Finally, let the following linear forms be defined as:
relates to an empty (i.e. with no element) minor, whose value is conventionally assumed equal to unity. Finally, let the following linear forms be defined as:
![]() (117a)
(117a)
![]() (117b)
(117b)
and let ![]() be the rank of the matrix,
be the rank of the matrix, ![]() , where
, where![]() ,
, ![]() ,
, ![]() ,
,![]() . Then, according to the theory of the quadratic forms, the linear forms,
. Then, according to the theory of the quadratic forms, the linear forms, ![]() ,
, ![]() , are linearly independent and the Jacobi formulae hold:
, are linearly independent and the Jacobi formulae hold:
![]() (118a)
(118a)
![]() (118b)
(118b)
which allow an explicit expression of Equation (60).
For further details, an interested reader is addredded to specific textbooks (e.g., [8] , Tome III, Part I, Chapter II; [9] , Vol. I, Chapter 10).
C. Reduction to Ordinary Geometry
Aiming to get further insight on the general results related to ![]() -spaces, the reduction to ordinary geometry, i.e. ordinary spaces,
-spaces, the reduction to ordinary geometry, i.e. ordinary spaces, ![]() , and ordinary planes,
, and ordinary planes, ![]() , shall be considered in detail.
, shall be considered in detail.
C.1. Ordinary Planes
In the special case, ![]() , the quadratic form on the left-hand side of Equation (37) reduces to:
, the quadratic form on the left-hand side of Equation (37) reduces to:
![]() (119)
(119)
and the related eigenvalue equation, expressed by Equation (51), reduces to:
![]() (120)
(120)
where eigenvalues can be calculated by equating each factor to zero, using Equation (5). The result is:
![]() (121)
(121)
The canonical equation of the quadratic form, expressed by Equations (56)-(57), reduces to:
![]() (122)
(122)
and the related orthogonal transformation, defined by Equation (69), reduces to:
![]() (123)
(123)
finally, the system of equations, expressed by Equation (77), reduces to:
![]() (124)
(124)
and the substitution of Equation (121) into (124), using Equation (5), after some algebra yields:
![]() (125)
(125)
On the other hand, the condition of unit norm, expressed by Equation (78), reduces to:
![]() (126)
(126)
and the combination of Equations (125) and (126) yields:
![]() (127)
(127)
finally, the substitution of Equation (127) into (123) produces:
![]() (128)
(128)
where, in addition, the substitution of Equation (128) into (122) yields Equation (120), as expected.
The coefficients and the linear forms appearing in Jacobi formulae, expressed by Equations (64) and (66), respectively, using Equation (5), reduce to:
![]() (129)
(129)
![]() (130)
(130)
and the canonical expression of the quadratic form, appearing on the left-hand side of Equation (67), reduces to:
![]() (131)
(131)
where, in addition, the substitution of Equation (130) into (131) yields Equation (119), as expected.
The equivalence of alternative canonical formulations, expressed by Equation (68), reduces to:
![]() (132)
(132)
and the substitution of Equation (121) into (132) yields:
![]() (133)
(133)
which shows the connection between coordinates, ![]() and
and![]() , with regard to the nonzero eigenvalue.
, with regard to the nonzero eigenvalue.
The canonical equation of the ![]() -quadric, lateral
-quadric, lateral ![]() -surface of the
-surface of the ![]() -cylinder, expressed by Equa- tions (36) and (23), reduces to:
-cylinder, expressed by Equa- tions (36) and (23), reduces to:
![]() (134)
(134)
where the substitution of Equation (121) into (134) yields:
![]() (135)
(135)
with regard to eigenvalue equation and, using Equation (132):
![]() (136)
(136)
which is equivalent to:
![]() (137)
(137)
with regard to Jacobi formulae. In any case, the ![]() -cylinder reduces to a band where the axis coincides with a coordinate axis.
-cylinder reduces to a band where the axis coincides with a coordinate axis.
C.2. Ordinary Spaces
In the special case, ![]() , the quadratic form on the left-hand side of Equation (37) reduces to:
, the quadratic form on the left-hand side of Equation (37) reduces to:
![]() (138)
(138)
and the related eigenvalue equation, expressed by Equation (51), reduces to:
![]() (139)
(139)
where eigenvalues can be calculated by equating each factor to zero, using Equation (5). The result is:
![]() (140a)
(140a)
![]() (140b)
(140b)
![]() (140c)
(140c)
![]() (140d)
(140d)
where the discriminant, ![]() , has necessarily to be nonnegative in that eigenvalues are real in the case under discussion.
, has necessarily to be nonnegative in that eigenvalues are real in the case under discussion.
The canonical equation of the quadratic form, expressed by Equations (56) and (57), reduces to:
![]() (141)
(141)
and the related orthogonal transformation, defined by Equation (69), reduces to:
![]() (142)
(142)
finally, the system of equations, expressed by Equation (77), reduces to:
![]() (143)
(143)
and the substitution of Equations (140) into (143), using Equation (5), after some algebra yields:
![]() (144)
(144)
On the other hand, the condition of unit norm, expressed by Equation (78), reduces to:
![]() (145)
(145)
and the combination of Equations (144) and (145) yields:
![]() (146)
(146)
where ![]() can be expressed in terms of
can be expressed in terms of ![]() as:
as:
![]() (147)
(147)
and the substitution of Equation (147) into (144) after some algebra yields:
![]() (148)
(148)
according to the condition of unit norm.
The system of equations, expressed by Equation (143), ![]() , can be solved by substitution of Equations (147) and (148) into (143), but related formulation is extremely cumbersome and direct numerical computations would be preferred [6] , Chapter 4.
, can be solved by substitution of Equations (147) and (148) into (143), but related formulation is extremely cumbersome and direct numerical computations would be preferred [6] , Chapter 4.
Concerning the special case, ![]() , the solutions of the system of equations, expressed by Equation (143), are:
, the solutions of the system of equations, expressed by Equation (143), are:
![]() (149)
(149)
where the condition of unit norm selects the solution:
![]() (150)
(150)
which completely specifies the orthogonal transformation, defined by Equation (142).
The substitution of Equation (142) into (141), via comparison with Equation (138) term by term, after some algebra yields [6] , Chapter 4:
![]() (151a)
(151a)
![]() (151b)
(151b)
![]() (151c)
(151c)
![]() (151d)
(151d)
![]() (151e)
(151e)
![]() (151f)
(151f)
which have been cheched numerically for assigned choices of the input parameters, ![]() ,
, ![]() ,
,![]() .
.
The coefficients and the linear forms appearing in Jacobi formulae, expressed by Equations (64) and (66), respectively, using Equation (5), reduce to:
![]() (152)
(152)
![]() (153)
(153)
![]() (154)
(154)
and the canonical expression of the quadratic form, appearing on the left-hand side of Equation (67), reduces to:
![]() (155)
(155)
where, in addition, the substitution of Equations (153)-(154) into (155) yields Equation (138), as expected.
The equivalence of alternative canonical formulations, expressed by Equation (68), reduces to:
![]() (156)
(156)
![]() (157)
(157)
and the substitution of Equations (140a), (140b), into (156), (157), respectively, after some algebra yields:
![]() (158)
(158)
![]() (159)
(159)
which shows the connection between coordinates, ![]() and
and![]() , with regard to nonzero eigenvalues.
, with regard to nonzero eigenvalues.
The canonical equation of the ![]() -quadric, lateral
-quadric, lateral ![]() -surface of a
-surface of a ![]() -cylinder, expressed by Equa- tions (36) and (23), reduces to:
-cylinder, expressed by Equa- tions (36) and (23), reduces to:
![]() (160)
(160)
where the substitution of Equations (140a)-(140b), into (160) yields:
![]() (161)
(161)
with regard to eigenvalue equation and, using Equations (156)-(157):
![]() (162)
(162)
with regard to Jacobi formulae. In any case, the ![]() -cylinder reduces to an ordinary cylinder where the axis coin- cides with a coordinate axis, and the orthogonal section is an ellipse whose semiaxes read:
-cylinder reduces to an ordinary cylinder where the axis coin- cides with a coordinate axis, and the orthogonal section is an ellipse whose semiaxes read:
![]() (163a)
(163a)
![]() (163b)
(163b)
with regard to eigenvalue equation, and:
![]() (164a)
(164a)
![]() (164b)
(164b)
with regard to Jacobi formulae.
D. Corrigendum [3]
Additional help to an interested reader could arise from the following corrigendum to a quoted earlier attempt devoted to the arithmetic mean [3] .
p. 2, right column: Equations (9) and (10) are inferred via Equation (8) instead of Equation (6).
p. 3, left column: the extension of usual formulation of analytic geometry has to be intended to ![]() - spaces instead of
- spaces instead of ![]() -spaces.
-spaces.
p. 3, right column: Equation (18) relates to the polar intead of equatorial semiaxis.
p. 4, right column: Equation (30) relates to a ![]() -hyperboloid intead of a
-hyperboloid intead of a ![]() -hyperboloid, where
-hyperboloid, where ![]() - hyperboloids are to be intended as
- hyperboloids are to be intended as ![]() -surfaces instead of
-surfaces instead of ![]() -volumes.
-volumes.
p. 4, right column: Equation (30) relates to the polar intead of equatorial semiaxis.
p. 4, right column: the intersection, ![]() , expressed by Equation (31), is to be conceived as a
, expressed by Equation (31), is to be conceived as a ![]() -line intead of a
-line intead of a ![]() -surface.
-surface.
p. 5, left column:![]() , are to be conceived as principal axes of a
, are to be conceived as principal axes of a ![]() -surface instead of a
-surface instead of a ![]() - volume.
- volume.
p. 5, left column: to avoid ambiguity, the direction cosines should be defined as ![]() with regard to the starting reference frame,
with regard to the starting reference frame,![]() ;
; ![]() with regard to the resulting reference frame,
with regard to the resulting reference frame,![]() . Accordingly,
. Accordingly, ![]() has to be replaced by
has to be replaced by ![]() in Equations (32), (33), (36), (37), and
in Equations (32), (33), (36), (37), and ![]() has to be replaced by
has to be replaced by ![]() in Equation (37). Finally,
in Equation (37). Finally, ![]() has to be replaced by
has to be replaced by ![]() everywhere in Equation (38).
everywhere in Equation (38).
p. 6, left column: the integration domain, ![]() , represents an infinitely thin
, represents an infinitely thin ![]() -cylindrical corona instead of a
-cylindrical corona instead of a ![]() -cylinder.
-cylinder.
p. 8, left column: ![]() has to be considered instead of
has to be considered instead of![]() .
.
p. 8, left column: Equation (49) has to be considered instead of (18) for determining Equation (60).
NOTES
2In particular, 2-sector reads bisector, 3-sector reads trisector, 4-sector reads quadrisector, and so on.
3In particular, 2-ant reads versant, 4-ant reads quadrant, 8-ant reads octant, and so on.