Stratified Convexity & Concavity of Gradient Flows on Manifolds with Boundary ()
1. Introduction
This paper is the first in a series that investigates the Morse Theory and gradient flows on smooth compact manifolds with boundary, a special case of the well-developed Morse theory on stratified spaces (see [2] -[4] ). For us, however, the starting point and the source of inspiration is the 1929 paper of Morse [1] .
We intend to present to the reader a version of the Morse Theory in which the critical points remain behind the scene, while shaping the geometry of the boundary! Some of the concepts that animate our approach can be found in [5] , where they are adopted to the special environment a 3 D-gradient flows. These notions include stratified convexity or concavity of traversing flows in connection to the boundary of the manifold. That concavity serves as a measure of intrinsic complexity of a given manifold 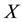 with respect to any traversing flow. Both convexity and concavity have strong topological implications.
with respect to any traversing flow. Both convexity and concavity have strong topological implications.
Another central theme that will make its first brief appearance in this paper is the holographic properties of traversing flows on manifolds with boundary. The ultimate aim here is to reconstruct (perhaps, only partially) the bulk of the manifold and the dynamics of the flow on it from some residual structures on the boundary, thus the name “holography”.
In Section 2, for so-called boundary generic fields  on
on  (see Definition 2.1), we explore the Morse stratification
(see Definition 2.1), we explore the Morse stratification  of the boundary
of the boundary ![]() (see Formula (1) and [1] ), induced by the vector field
(see Formula (1) and [1] ), induced by the vector field ![]() on
on![]() .
.
In Section 3, we investigate the degrees of freedom to change this stratification by deforming a given vector field within the space of gradient-like fields (Theorem 3.2, Corollary 3.2, and Corollary 3.3).
In Section 4, for vector fields on compact manifolds, we introduce the pivotal notion of boundary ![]() -convexity/
-convexity/![]() -concavity,
-concavity, ![]() (see Definition 4.1). Then we explore some topological implications of the existence of a boundary
(see Definition 4.1). Then we explore some topological implications of the existence of a boundary ![]() -convex/
-convex/![]() -concave traversing field on
-concave traversing field on ![]() (see Lemma 4.2, Corollary 4.2, Corollary 4.3, and Corollary 4.4).
(see Lemma 4.2, Corollary 4.2, Corollary 4.3, and Corollary 4.4).
Let ![]() denote the orthogonal projection of the field
denote the orthogonal projection of the field ![]() on the bundle
on the bundle ![]() tangent to the boundary. Occasionally, we can determine whether a given field
tangent to the boundary. Occasionally, we can determine whether a given field ![]() is convex/concave just by observing the behavior of the
is convex/concave just by observing the behavior of the ![]() -trajectories on the boundary
-trajectories on the boundary ![]() (Theorem 4.1, Theorem 4.2). We view the possibility of such determination as an instance of a more general phenomenon, which we call “holography”. This phenomenon will occupy us fully in a different paper.
(Theorem 4.1, Theorem 4.2). We view the possibility of such determination as an instance of a more general phenomenon, which we call “holography”. This phenomenon will occupy us fully in a different paper.
The Eliashberg surgery theory of folding maps [6] [7] helps us to describe the patterns of Morse stratifications for traversing 3-concave and 3-convex fields (Theorem 5.1, Conjecture 5.1, and Corollary 5.1).
2. The Morse Stratification ![]()
Inspired by [1] , we start by introducing some basic notions and constructions that describe the way in which generic vector fields on a compact smooth manifold interact with its boundary.
Let ![]() be a compact smooth
be a compact smooth ![]() -dimensional manifold with a boundary
-dimensional manifold with a boundary![]() . Let v be a smooth vec- tor field on
. Let v be a smooth vec- tor field on ![]() which does not vanish on the boundary
which does not vanish on the boundary![]() . As a rule, we assume that X is properly contained in a
. As a rule, we assume that X is properly contained in a ![]() -dimensional manifold
-dimensional manifold ![]() and that the field v extends to a field
and that the field v extends to a field ![]() on
on ![]() so that
so that![]() . In fact, we always treat the pair
. In fact, we always treat the pair ![]() as a germ of a space and a field in the vicinity of the given pair
as a germ of a space and a field in the vicinity of the given pair![]() .
.
Often we will consider vector fields only with the isolated Morse-type singularities (zeros) located away from the boundary. This means that v, viewed as a section of the tangent bundle![]() , is transversal its zero section. In other words, in the vicinity of each singular point, there is a local system of coordinates
, is transversal its zero section. In other words, in the vicinity of each singular point, there is a local system of coordinates ![]() such that the field v can be represented as
such that the field v can be represented as![]() , where all
, where all![]() .
.
To achieve some uniformity in our notations, let ![]() and
and![]() .
.
The vector field v gives rise to a partition ![]() of the boundary
of the boundary ![]() into two sets: the locus
into two sets: the locus![]() , where the field is directed inward of
, where the field is directed inward of![]() , and
, and![]() , where it is directed outwards. We assume that
, where it is directed outwards. We assume that![]() , viewed as a section of the quotient line bundle
, viewed as a section of the quotient line bundle ![]() over
over![]() , is transversal to its zero section. This assumption implies that both sets
, is transversal to its zero section. This assumption implies that both sets ![]() are compact manifolds which share a common boundary
are compact manifolds which share a common boundary ![]() . Evidently,
. Evidently, ![]() is the locus where
is the locus where ![]() is tangent to the boundary
is tangent to the boundary![]() .
.
Morse has noticed that, for a generic vector field ν, the tangent locus ![]() inherits a similar structure in con- nection to
inherits a similar structure in con- nection to![]() , as
, as ![]() has in connection to
has in connection to ![]() (see [1] ). That is,
(see [1] ). That is, ![]() gives rise to a partition
gives rise to a partition ![]() of
of ![]() into two sets: the locus
into two sets: the locus![]() , where the field is directed inward of
, where the field is directed inward of![]() , and
, and![]() , where it is directed outward of
, where it is directed outward of![]() . Again, let us assume that v, viewed as a section of the quotient line bundle
. Again, let us assume that v, viewed as a section of the quotient line bundle ![]() over
over![]() , is transversal to its zero section.
, is transversal to its zero section.
For generic fields, this structure replicates itself: the cuspidal locus ![]() is defined as the locus where v is tangent to
is defined as the locus where v is tangent to![]() ;
; ![]() is divided into two manifolds,
is divided into two manifolds, ![]() and
and![]() . In
. In![]() , the field is directed inward of
, the field is directed inward of![]() , in
, in![]() , outward of
, outward of![]() . We can repeat this construction until we reach the zero-dimensional stratum
. We can repeat this construction until we reach the zero-dimensional stratum ![]() (see Figure 1 and Figure 2 which depict these strata for fields in
(see Figure 1 and Figure 2 which depict these strata for fields in![]() ).
).
These considerations motivate
Definition 2.1 We say that a smooth field v on ![]() is boundary generic if:
is boundary generic if:
・ ![]() ,
,
・ ![]() , viewed as a section of the tangent bundle
, viewed as a section of the tangent bundle![]() , is transversal to its zero section,
, is transversal to its zero section,
・ for each![]() , the
, the ![]() -generated stratum
-generated stratum ![]() is a smooth submanifold of
is a smooth submanifold of![]() ,
,
・ the field![]() , viewed as section of the quotient 1-bundle
, viewed as section of the quotient 1-bundle
![]() ,
,
is transversal to the zero section of ![]() for all
for all![]() .
.
We denote the space of smooth boundarygeneric vector fields on ![]() by the symbol
by the symbol![]() .
. ![]()
Thus a boundary generic vector field ![]() on
on ![]() gives rise to two stratifications:
gives rise to two stratifications:
![]() (1)
(1)
, the first one by closed submanifolds, the second one―by compact ones. Here ![]() .
.
For simplicity, the notations “![]() ” do not reflect the dependence of these strata on the vector field
” do not reflect the dependence of these strata on the vector field![]() . When the field varies, we use a more accurate notation “
. When the field varies, we use a more accurate notation “![]() ”.
”.
Remark 2.1. Replacing ![]() with
with ![]() affects the Morse stratification according to the formula:
affects the Morse stratification according to the formula:
![]()
where ![]() when
when![]() , and
, and ![]() otherwise.
otherwise. ![]()
We will postpone the proof of the theorem below until the second paper in this series of articles (see [8] , Theorem 3.4, an extension of Theorem 2.1 below). There we will develop the needed analytical tools.
Theorem 2.1 Boundary generic vector fields form an open and dense subset ![]() in the space
in the space ![]() of all smooth fields on
of all smooth fields on![]() .
. ![]()
Definition 2.2 We say that a smooth vector field ![]() on
on ![]() is of the gradient type (or gradient-like) for a smooth function
is of the gradient type (or gradient-like) for a smooth function ![]() if:
if:
・ the differential ![]() and the field
and the field ![]() vanish on the same locus
vanish on the same locus![]() ,
,
・ the function ![]() in
in![]() ,
,
・ in the vicinity of![]() , there exist a Rimannian metric
, there exist a Rimannian metric ![]() on
on ![]() so that
so that![]() , the gradient field of
, the gradient field of ![]() in the metric
in the metric![]() .
. ![]()
Definition 2.3 A smooth function ![]() is called Morse function if its differential
is called Morse function if its differential![]() , viewed as a section of the cotangent bundle
, viewed as a section of the cotangent bundle![]() , is transversal to the zero section.
, is transversal to the zero section. ![]()
Recall that, for a Morse function ![]() on a compact
on a compact ![]() -manifold X, the critical set
-manifold X, the critical set ![]()
is finite, and each point ![]() has special local coordinates
has special local coordinates ![]() such that
such that![]() , where
, where
![]() for all
for all ![]() (for example, see [9] ).
(for example, see [9] ).
Definition 2.4 Let ![]() be a smooth function and
be a smooth function and ![]() its gradient-like vector field. We say that the pair
its gradient-like vector field. We say that the pair ![]() is boundary generic if the field
is boundary generic if the field ![]() is boundary generic in the sense of Definition 2.1 and the res- trictions of
is boundary generic in the sense of Definition 2.1 and the res- trictions of ![]() to each stratum
to each stratum ![]() are Morse functions for all
are Morse functions for all![]() .
. ![]()
Lemma 2.1 Let ![]() be a compact smooth manifold, and
be a compact smooth manifold, and ![]() a smooth manifold which is stratified by sub- manifolds
a smooth manifold which is stratified by sub- manifolds![]() . Let
. Let ![]() be the space of smooth maps
be the space of smooth maps ![]() which are transversal to each stratum
which are transversal to each stratum![]() . Put
. Put![]() . Next consider the space
. Next consider the space ![]() of pairs
of pairs ![]() such that
such that ![]()
and ![]() has the property:
has the property: ![]() are Morse functions for all
are Morse functions for all![]() . Then
. Then ![]() is open and dense in the
is open and dense in the
space![]() .
.
Proof. Consider the space![]() , where
, where ![]() denotes the cotangent bundle of
denotes the cotangent bundle of![]() . The property
. The property ![]() is equivalent to the property of the section
is equivalent to the property of the section ![]() of the bundle
of the bundle
![]()
to be transversal to each (transversal) intersection of the ![]() -graph
-graph ![]() with each stratum
with each stratum![]() . The latter property defines a open set in
. The latter property defines a open set in![]() .
.
In order to validate density of ![]() in
in![]() , we first perturb a given map
, we first perturb a given map ![]() to make it transversal to each stratum
to make it transversal to each stratum![]() , and then perturb a given function
, and then perturb a given function ![]() to make the section
to make the section ![]() of
of ![]() transversal to each manifold
transversal to each manifold![]() .
. ![]()
Theorem 2.2 The boundary generic1 Morse pairs ![]() on a compact manifold
on a compact manifold ![]() form an open and dense subset in the space of all smooth functions
form an open and dense subset in the space of all smooth functions ![]() and their gradient-like fields
and their gradient-like fields![]() .
.
Proof. By Theorem 2.1, the boundary generic fields ![]() form an open and dense set in the space of all fields.
form an open and dense set in the space of all fields.
Let ![]() be a complete flag in
be a complete flag in![]() , formed by subspaces
, formed by subspaces ![]() of codimension j. In the proof of Theorem 3.4 [8] , for every field
of codimension j. In the proof of Theorem 3.4 [8] , for every field![]() , we will construct a smooth map
, we will construct a smooth map ![]() such that
such that![]() . Moreover,
. Moreover, ![]() is transversal to each
is transversal to each![]() , if and only if,
, if and only if, ![]() is a boundary generic field. The construction of the map
is a boundary generic field. The construction of the map ![]() utilizes high order Lie derivatives
utilizes high order Lie derivatives ![]() of an auxiliary function
of an auxiliary function ![]() as in Lemma 3.1 [8] .
as in Lemma 3.1 [8] .
Now the property of boundary generic Morse pairs ![]() to be open and dense in the space of all pairs follows from Lemma 2.1: just let
to be open and dense in the space of all pairs follows from Lemma 2.1: just let![]() ,
, ![]() ,
, ![]() , and
, and ![]() in that lemma.
in that lemma.
For the reader convenience, let us sketch now an alternative argument that establishes just the density of boundary generic Morse pairs ![]() in the space of all pairs. It does not rely on the construction of the map
in the space of all pairs. It does not rely on the construction of the map ![]() from [8] .
from [8] .
We start with a pair ![]() where
where ![]() and
and ![]() at the points of the set where
at the points of the set where![]() . By a small perturbation of
. By a small perturbation of![]() , we can assume the
, we can assume the ![]() is a Morse function on
is a Morse function on ![]() and
and ![]() its gradient-like field.
its gradient-like field.
Let ![]() be a compact regular neighborhood of
be a compact regular neighborhood of ![]() in
in ![]() so small that
so small that![]() . By Theorem 2.1, we can perturb
. By Theorem 2.1, we can perturb ![]() to a new field
to a new field ![]() so that
so that ![]() is boundary generic in the sense of Definition 2.1 and still
is boundary generic in the sense of Definition 2.1 and still![]() .
.
For a given![]() , the condition
, the condition ![]() defines an open cone in the space of all fields
defines an open cone in the space of all fields![]() , subject to the constraint
, subject to the constraint![]() . Therefore
. Therefore ![]() can be chosen both boundary generic and gradient-like for
can be chosen both boundary generic and gradient-like for![]() . When
. When ![]() is fixed, so are the stratifications
is fixed, so are the stratifications![]() .
.
Next, with ![]() being fixed, we perturb
being fixed, we perturb ![]() again to a new function
again to a new function ![]() so that
so that ![]() and
and
![]() are Morse functions for all j. The perturbation will be supported in the compact
are Morse functions for all j. The perturbation will be supported in the compact![]() . We start
. We start
constructing ![]() inductively first from adjusting it on the 1-manifold
inductively first from adjusting it on the 1-manifold ![]() and then moving sequentially to the strata
and then moving sequentially to the strata ![]() with lower indices j. We pick each perturbation
with lower indices j. We pick each perturbation ![]() so small that the open condition
so small that the open condition ![]() is not violated. The existence of the desired
is not violated. The existence of the desired ![]() -th perturbation is based on the fact that Morse functions on a compact manifold
-th perturbation is based on the fact that Morse functions on a compact manifold ![]() (in this case, on
(in this case, on![]() ) form an open and dense subset in
) form an open and dense subset in![]() , the space of all smooth functions on
, the space of all smooth functions on![]() , being equipped with the Whitney topology. Note that since
, being equipped with the Whitney topology. Note that since ![]() is tangent
is tangent
to ![]() along
along ![]() and
and![]() , the restriction
, the restriction ![]() has no critical points in the vi-
has no critical points in the vi-
cinity of![]() . Thus we need to perturb
. Thus we need to perturb ![]() only on a compact subset
only on a compact subset ![]() which has an
which has an
empty intersection with![]() . This perturbation extends smoothly from
. This perturbation extends smoothly from ![]() to
to![]() . Eventually, we reach the upper stratum
. Eventually, we reach the upper stratum![]() , thus constructing a boundary generic approximation of the given pair
, thus constructing a boundary generic approximation of the given pair![]() .
.
All the changes ![]() of
of![]() , but the first one, we have introduced so far are supported in
, but the first one, we have introduced so far are supported in![]() , where
, where
![]() and
and![]() . This proves that the boundary generic pairs form a dense set in the space of all pairs
. This proves that the boundary generic pairs form a dense set in the space of all pairs![]() , where
, where ![]() being a
being a ![]() -gradient-like field, subject to the constraints:
-gradient-like field, subject to the constraints:![]() , and
, and ![]() being a Morse function.
being a Morse function. ![]()
For a given Morse pair![]() , we denote by
, we denote by ![]() the set of critical points of the function
the set of critical points of the function![]() . For a boundary generic Morse pair
. For a boundary generic Morse pair![]() , the finite critical set
, the finite critical set ![]() is divided into two complementary sets: the set
is divided into two complementary sets: the set ![]() of positive critical points and the set
of positive critical points and the set ![]() of negative ones (see Figure 3).
of negative ones (see Figure 3).
Remark 2.2. Note that when![]() , it may happen that
, it may happen that![]() . However, if a component
. However, if a component ![]() of
of ![]() is a closed manifold, then
is a closed manifold, then ![]() must have local extrema, in which case
must have local extrema, in which case![]() .
. ![]()
Consider a generic field ![]() and a Riemannian metric
and a Riemannian metric ![]() on
on![]() . We denote by
. We denote by ![]() the orthogonal projection of the field
the orthogonal projection of the field ![]() on the tangent space
on the tangent space![]() . Note that if
. Note that if ![]() is a gradient field for a function
is a gradient field for a function ![]() in metric
in metric![]() , then
, then ![]() is automatically a gradient field for the restrictions
is automatically a gradient field for the restrictions ![]() and
and![]() .
.
Take a smooth vector field ![]() on a compact
on a compact ![]() -manifold
-manifold ![]() with isolated singularities
with isolated singularities
![]() . We denote by
. We denote by ![]() the localized index of
the localized index of ![]() at its typical singular point
at its typical singular point![]() . In a
. In a
local chart, ![]() is defined as the degree of a map
is defined as the degree of a map ![]() from a small
from a small ![]() -centered
-centered ![]() -sphere
-sphere
to the unit ![]() -sphere. The map takes each point
-sphere. The map takes each point ![]() to the point
to the point![]() .
.
We define the “global” index ![]() as the sum
as the sum![]() .
.
For a generic field ![]() and a Riemannian metric
and a Riemannian metric ![]() on
on![]() , we form the fields
, we form the fields ![]() on
on ![]() and define the global index of
and define the global index of ![]() by the formula:
by the formula:
![]()
Figure 3. Positive (the left diagram) and negative (the right diagram) singularities of ![]() on the boundary of a solid
on the boundary of a solid![]() .
.
![]()
Let us revisit the beautiful Morse formulas [1] :
Theorem 2.3 (The Morse Law of Vector Fields) For a boundary generic vector field ![]() and a Riemannian metric on a
and a Riemannian metric on a ![]() -manifold
-manifold![]() , such that the singularities of the fields
, such that the singularities of the fields ![]() are isolated for all
are isolated for all![]() , the following two equivalent sets of formulas hold:
, the following two equivalent sets of formulas hold:
![]()
![]() (2)
(2)
where ![]() stands for the Euler number of the appropriate space2.
stands for the Euler number of the appropriate space2. ![]()
For vector fields with symmetry, the Morse Law of Vector Fields has an equivariant generalization [10] . Here is its brief description: for a compact Lie group ![]() acting on a compact manifold
acting on a compact manifold![]() , equipped with a
, equipped with a ![]()
-equivariant field![]() , we prove that the invariants
, we prove that the invariants ![]() can be interpreted as taking values in the
can be interpreted as taking values in the
Burnside ring ![]() of the group
of the group ![]() (see [11] for the definitions). With this interpretation in place, the appearance of Formula (2) does not change.
(see [11] for the definitions). With this interpretation in place, the appearance of Formula (2) does not change.
Morse Formula (2) has an instant, but significant implication:
Corollary 2.1 Let ![]() be a smooth neighborhood of the zero set of a vector field
be a smooth neighborhood of the zero set of a vector field ![]() on a compact
on a compact ![]() -manifold
-manifold![]() . Assume that
. Assume that ![]() is boundary generic with respect to both boundaries,
is boundary generic with respect to both boundaries, ![]() and
and![]() . Then
. Then
![]()
Remark 2.3. Therefore, the numbers
![]()
can serve as “more and less localized” definitions of the index invariant![]() .
. ![]()
An interesting discussion, connected to Theorem 2.3, its topological and geometrical implications, can be found in the paper of Gotlieb [12] . The “Topological Gauss-Bonnet Theorem” below is a sample of these re- sults.
Theorem 2.4 (Gotlieb) Let ![]() be a compact smooth
be a compact smooth ![]() -dimensional manifold and
-dimensional manifold and ![]() a smooth map which is a immersion in the vicinity of the boundary
a smooth map which is a immersion in the vicinity of the boundary![]() . Let
. Let ![]() be a Riemannian metric on
be a Riemannian metric on ![]() which, in the vicinity of
which, in the vicinity of![]() , is the pull-back
, is the pull-back ![]() of the Euclidean metric on
of the Euclidean metric on![]() . Consider a generic linear function
. Consider a generic linear function ![]() such that the composite function
such that the composite function ![]() has only isolated singularities in the interior of
has only isolated singularities in the interior of![]() . Let
. Let ![]() be the gradient field of
be the gradient field of ![]() 3. Assume that
3. Assume that ![]() is boundary generic.
is boundary generic.
Then the degree of the Gauss map
![]()
can be calculated either by integrating over ![]() the normal curvature
the normal curvature ![]() (in the metric
(in the metric![]() ) of the hyper- surface
) of the hyper- surface![]() , or in terms of the
, or in terms of the ![]() -induced stratification
-induced stratification
![]()
by the formula
![]() (3)4
(3)4
Example 2.1. Let X be an orientable surface of genus g with a single boundary component. Let ![]() be an immersion, and let
be an immersion, and let![]() ,
, ![]() and
and ![]() be as in Theorem 2.4.
be as in Theorem 2.4.
Since Ф is an immersion everywhere (and not only in the vicinity of ∂X as Theorem 2.4 presumes), we get that![]() . Thus
. Thus![]() . Then Theorem 2.4 claims that the degree of the Gauss map
. Then Theorem 2.4 claims that the degree of the Gauss map ![]() is equal to
is equal to
![]()
Thus, the topological Gauss-Bonnet theorem, for immersions![]() , reduces to the equation
, reduces to the equation
![]() .
.
So the number of ![]() -trajectories
-trajectories ![]() in
in ![]() that are tangent to
that are tangent to![]() , but are not singletons (they correspond to points of
, but are not singletons (they correspond to points of![]() ), as a function of genus
), as a function of genus![]() , grows at least as fast as
, grows at least as fast as![]() .
.
On the other hand, by the Whitney index formula [13] , the degree of ![]() can be also calculated as
can be also calculated as![]() , where
, where ![]() denotes the number of positive/negative self-intersections of the curve
denotes the number of positive/negative self-intersections of the curve![]() , and
, and![]() .
.
By a theorem of L. Guth [14] , the total number of self-intersections![]() . Moreover, this lower bound is realized by an immersion
. Moreover, this lower bound is realized by an immersion![]() ! Therefore, for any immersion
! Therefore, for any immersion![]() , the total number of self-intersections of the curve
, the total number of self-intersections of the curve ![]() can be estimated in terms of the boundary-tangent
can be estimated in terms of the boundary-tangent ![]() -trajectories:
-trajectories:
![]()
and for some special immersion![]() , we get
, we get
![]()
Corollary 2.2 Let ![]() be a compact
be a compact ![]() -manifold with boundary, which is properly contained in an
-manifold with boundary, which is properly contained in an
open ![]() -manifold
-manifold![]() . Let
. Let ![]() be a smooth map which is a immersion in the vicinity of the
be a smooth map which is a immersion in the vicinity of the
boundary![]() . Let
. Let ![]() be a Riemannian metric on
be a Riemannian metric on ![]() which, in the vicinity of
which, in the vicinity of![]() , is the pull-back
, is the pull-back ![]() of the Euclidean metric on
of the Euclidean metric on![]() .
.
Let ![]() be a linear function, and
be a linear function, and ![]() its composition with the map
its composition with the map![]() . Form the gradient field
. Form the gradient field ![]() in
in![]() . Assume that the pair
. Assume that the pair ![]() is boundary generic in the sense of Definition 2.4.
is boundary generic in the sense of Definition 2.4.
For each![]() , consider a
, consider a ![]() -small tubular neighborhood
-small tubular neighborhood ![]() of the manifold
of the manifold ![]() in
in![]() . Then
. Then ![]() is an immersion. This setting gives rise to the Gauss map
is an immersion. This setting gives rise to the Gauss map![]() , defined by the
, defined by the
formula![]() , where
, where ![]() and
and ![]() is the unit vector inward normal to
is the unit vector inward normal to ![]() at
at![]() .
.
Then the degree of the Gauss map ![]() can be calculated either by integrating (with respect to the
can be calculated either by integrating (with respect to the ![]()
-measure![]() ) over
) over ![]() the normal curvature
the normal curvature ![]() of the hypersurface
of the hypersurface![]() , or in terms of the
, or in terms of the ![]()
-induced stratum![]() :
:
![]() (4)
(4)
Proof. We will apply Theorem 2.4 to the field ![]() in
in ![]() to conclude that
to conclude that
![]()
Since ![]() in
in![]() ,
, ![]() , and the last term of this equation reduces to
, and the last term of this equation reduces to![]() .
. ![]()
Remark 2.4. Of course, for an odd-dimensional![]() , the Euler number
, the Euler number![]() , and so is
, and so is![]() . When
. When ![]() is even-dimensional (i.e.,
is even-dimensional (i.e.,![]() ), the integral in Equation (4) can be expressed in terms of intrinsic Riemannian geometry of the manifold
), the integral in Equation (4) can be expressed in terms of intrinsic Riemannian geometry of the manifold![]() , namely, in terms of the Pfaffian
, namely, in terms of the Pfaffian![]() . The Pfafian is a
. The Pfafian is a ![]() -differential form, whose construction utilizes the curvature tensor on the manifold (see [15] ). So, when
-differential form, whose construction utilizes the curvature tensor on the manifold (see [15] ). So, when![]() ,
,
![]()
Given a boundary generic field ![]() on
on![]() , we introduce a sequence of basic degree-type invariants
, we introduce a sequence of basic degree-type invariants ![]()
which are intimately linked, via the Morse Formula (2), to the invariants![]() .
.
We use a Riemannian metric ![]() on
on ![]() to produce the orthogonal projection
to produce the orthogonal projection ![]() of the field
of the field ![]() on the tangent subspace
on the tangent subspace![]() .
.
Let ![]() be the bundle of unit
be the bundle of unit ![]() -spheres associated with the tangent bundle of the manifold
-spheres associated with the tangent bundle of the manifold![]() . We denote by
. We denote by ![]() the restriction of the bundle
the restriction of the bundle ![]() to the subspace
to the subspace![]() .
.
For each k, consider two fields, the inward normal field ![]() to
to ![]() in
in ![]() and
and![]() , as sections of the sphere bundle
, as sections of the sphere bundle ![]() (remember,
(remember, ![]() is tangent to
is tangent to ![]() along
along ![]() so that
so that ![]() along
along![]() !). Assume that the sections ν and
!). Assume that the sections ν and ![]() are transversal in the space
are transversal in the space![]() . This transversality can be achieved by a perturbation of
. This transversality can be achieved by a perturbation of ![]() (equivalently, by a perturbation of the metric
(equivalently, by a perturbation of the metric![]() ), supported in the vicinity of the singularity locus
), supported in the vicinity of the singularity locus![]() . Indeed, the intersections occur where the field
. Indeed, the intersections occur where the field ![]() is positively proportional to
is positively proportional to![]() , that is, where
, that is, where![]() . The later locus is exactly the locus
. The later locus is exactly the locus![]() . The perturbation does not affect the stratification
. The perturbation does not affect the stratification![]() . Assuming the transversality of the intersection, the locus
. Assuming the transversality of the intersection, the locus ![]() is zero- dimensional.
is zero- dimensional.
We define the integer ![]() as the algebraic intersection number of two
as the algebraic intersection number of two ![]() -cycles,
-cycles, ![]() and
and![]() , in the ambient manifold
, in the ambient manifold ![]() of dimension
of dimension![]() .
.
Lemma 2.2 For a boundary generic field ![]() on a Riemannian manifold
on a Riemannian manifold![]() , the following formula holds:
, the following formula holds:
![]()
Proof. We already have noticed that the intersection set ![]() projects bijectively under the map
projects bijectively under the map ![]() onto the locus
onto the locus![]() , where the component
, where the component ![]() of
of ![]() vanishes and
vanishes and ![]() points in- ward of
points in- ward of![]() . It takes more work to see that the sign attached to the transversal intersection point
. It takes more work to see that the sign attached to the transversal intersection point ![]() is
is![]() , where
, where ![]() is the index (the localized degree) of the field
is the index (the localized degree) of the field ![]() in the vicinity of its singularity
in the vicinity of its singularity![]() . Thus
. Thus![]() . By the Morse Formula (2), the claim of the lemma follows.
. By the Morse Formula (2), the claim of the lemma follows. ![]()
Corollary 2.3 The integer ![]() depends only on the singular locus
depends only on the singular locus ![]() of
of ![]() and on the local indices of its points.
and on the local indices of its points. ![]()
Question 2.1. How to compute ![]() in the terms of Riemannian geometry and in the spirit of Theorem 2.4 and Corollary 2.2?
in the terms of Riemannian geometry and in the spirit of Theorem 2.4 and Corollary 2.2? ![]()
For a boundary generic field ![]() and a fixed metric
and a fixed metric ![]() on
on![]() , each manifold
, each manifold ![]() comes equipped with a preferred normal framing
comes equipped with a preferred normal framing ![]() of the normal bundle
of the normal bundle![]() : just consider the unitary inward normal field
: just consider the unitary inward normal field ![]() of
of ![]() in
in![]() , then the unitary inward normal field
, then the unitary inward normal field ![]() of
of ![]() in
in![]() , being restricted to
, being restricted to![]() , then the unitary inward normal field
, then the unitary inward normal field ![]() of
of ![]() in
in![]() , being restricted to
, being restricted to![]() , and so on.
, and so on.
Via the Pontryagin construction [16] , this framing ![]() generates a continuous map
generates a continuous map![]() . Its homotopy class
. Its homotopy class ![]() is an element of the cohomotopy set
is an element of the cohomotopy set![]() . If
. If![]() , then we define
, then we define ![]() to be the trivial map that takes
to be the trivial map that takes ![]() to the base point in
to the base point in![]() .
.
Unfortunately, as we will see soon,![]() ! However, when
! However, when![]() , each of the two loci
, each of the two loci ![]() is a closed manifold. Then we can apply the Pontryagin construction only to, say,
is a closed manifold. Then we can apply the Pontryagin construction only to, say, ![]() to get a map
to get a map![]() . This application leads directly to the following proposition.
. This application leads directly to the following proposition.
Corollary 2.4 Consider a boundary generic vector field ![]() such that
such that ![]() and a metric
and a metric![]() , defined in the vicinity of
, defined in the vicinity of ![]() in
in![]() . Then these data give rise to continuous map
. Then these data give rise to continuous map![]() .
.
The homotopy class ![]() is independent of the choice of
is independent of the choice of ![]() and a homotopy of
and a homotopy of ![]()
within the open subspace of![]() , defined by the constraint
, defined by the constraint![]() .
.
In particular, when![]() , we get an element
, we get an element
![]()
and when![]() , an element
, an element
![]()
If![]() , we can interpret
, we can interpret ![]() also as an element of the homotopy group
also as an element of the homotopy group![]() .
. ![]()
The elements ![]() and
and ![]() have another classical interpretation as elements of oriented framed
have another classical interpretation as elements of oriented framed
cobordism set![]() . In fact, the pair
. In fact, the pair ![]() defines the trivial element in
defines the trivial element in![]() . In
. In
contrast, if![]() , then the bordism class
, then the bordism class ![]() may be nontrivial.
may be nontrivial.
Let us recall the definition of framed cobordisms (for example, see [17] ). Let ![]() be oriented closed smooth
be oriented closed smooth ![]() -dimensional submanifolds of a compact
-dimensional submanifolds of a compact ![]() -manifold
-manifold![]() , whose normal bundles
, whose normal bundles ![]() and
and ![]() are equipped with framings
are equipped with framings ![]() and
and![]() , respectively.
, respectively.
We say that two pairs ![]() and
and ![]() define the same element in
define the same element in![]() , if there is a compact
, if there is a compact ![]() -dimensional oriented submanifold
-dimensional oriented submanifold ![]() whose normal bundle
whose normal bundle ![]() admits a framing
admits a framing ![]() so that:
so that:
1)![]() ,
,
2) the restriction of ![]() to
to ![]() coincides with
coincides with![]() , and the restriction of
, and the restriction of ![]() to
to ![]() coincides with
coincides with![]() .
.
Then the Pontryagin construction establishes a bijection![]() , where
, where![]() . If
. If ![]() both sets admit a structure of abelian groups and the bijection
both sets admit a structure of abelian groups and the bijection ![]() becomes a group isomorphism.
becomes a group isomorphism.
Now we are in position to explain why![]() . Consider the obvious embedding
. Consider the obvious embedding
![]()
We can isotop ![]() in
in ![]() to a regular embedding
to a regular embedding
![]()
such that:
1)![]() , and
, and
2) the inward normal field ![]() is parallel to the factor
is parallel to the factor ![]() in the product
in the product![]() .
.
Note that for![]() , all the normal fields
, all the normal fields ![]() are preserved under the imbedding
are preserved under the imbedding![]() . So,
. So,
for any![]() , the normal framing
, the normal framing ![]() of
of ![]() in
in ![]() extends to a normal framing
extends to a normal framing ![]()
of ![]() in
in![]() . Therefore
. Therefore ![]() as an element of the framed bordisms of
as an element of the framed bordisms of![]() . As a
. As a
result, when![]() , we get
, we get ![]() in
in ![]() (equivalently, in
(equivalently, in![]() ).
).
3. Deforming the Morse Stratification
Let ![]() be a smooth compact
be a smooth compact ![]() -manifold with boundary
-manifold with boundary![]() . A boundary generic field
. A boundary generic field ![]() (see Definition 2.1) gives rise to two stratifications (1).
(see Definition 2.1) gives rise to two stratifications (1).
We are going to investigate how the stratification ![]() changes as a result of deforming the vector field
changes as a result of deforming the vector field![]() .
.
Lemma 3.1 Let ![]() be a closed submanifold of a manifold
be a closed submanifold of a manifold ![]() and
and ![]() a closed manifold. Consider a family of maps
a closed manifold. Consider a family of maps ![]() such that each
such that each ![]() is transversal to
is transversal to![]() . All the manifolds, maps, and families of maps are assumed to be smooth.
. All the manifolds, maps, and families of maps are assumed to be smooth.
Then all the submanifolds ![]() are isotopic in
are isotopic in![]() . In particular, the intersections
. In particular, the intersections ![]() and
and ![]() are diffeomorphic.
are diffeomorphic.
Proof. Let ![]() be the map defined by the family
be the map defined by the family![]() . Thanks to the transversality hypothesis,
. Thanks to the transversality hypothesis, ![]() is transversal to
is transversal to ![]() and
and ![]() is a submanifold of
is a submanifold of ![]() whose boundary is
whose boundary is
![]()
Let ![]() be a vector field on
be a vector field on![]() , normal to each codimension 1 submanifold
, normal to each codimension 1 submanifold ![]() in
in![]() . In the construction of
. In the construction of![]() , we evidently rely on the property of each
, we evidently rely on the property of each ![]() being transversal to
being transversal to![]() . Since
. Since ![]() and
and![]() , each w-trajectory that originates at a point of
, each w-trajectory that originates at a point of ![]() must reach
must reach ![]() in finite time. Therefore, employing the w-flow,
in finite time. Therefore, employing the w-flow, ![]() is diffeomorphic to
is diffeomorphic to![]() , and the
, and the ![]() -image of that product structure in
-image of that product structure in ![]() defines a smooth isotopy between
defines a smooth isotopy between ![]() and
and ![]() in
in![]() . This isotopy extends to an ambient isotopy of
. This isotopy extends to an ambient isotopy of ![]() itself [18] .
itself [18] .
Note that these arguments fail in general if ether M or N have boundaries. However, under additional assumptions (such as![]() being t-independent and
being t-independent and![]() ), the relative versions of the lemma are valid.
), the relative versions of the lemma are valid. ![]()
Theorem 3.1 The diffeomorphism type of each stratum ![]() is constant within each path-connected component of the space
is constant within each path-connected component of the space ![]() of boundary generic fields.
of boundary generic fields.
Proof. If two generic fields, ![]() and
and![]() , are connected by a continuous path
, are connected by a continuous path![]() , then they can be connected by a path
, then they can be connected by a path ![]() such that the dependence of the field
such that the dependence of the field ![]() on
on ![]() is smooth. The argument is based on the property of generic fields to form an open set in the space of all fields (Theorem 2.1), the smooth partition of unity technique (which utilizes the compactness of manifold
is smooth. The argument is based on the property of generic fields to form an open set in the space of all fields (Theorem 2.1), the smooth partition of unity technique (which utilizes the compactness of manifold![]() ), and the standard techniques of approximating continuos functions with the smooth ones.
), and the standard techniques of approximating continuos functions with the smooth ones.
Thus it suffices to consider a smooth 1-parameter family of vector fields![]() , connecting
, connecting ![]() to
to![]() . Since any generic field
. Since any generic field![]() , viewed as a section of the vector bundle
, viewed as a section of the vector bundle![]() , is transversal its zero section, we may apply Lemma 3.1 (with
, is transversal its zero section, we may apply Lemma 3.1 (with![]() ,
, ![]() being the zero section of
being the zero section of![]() ,
, ![]() , and
, and![]() ) to conclude that all the submanifolds
) to conclude that all the submanifolds ![]() are isotopic in
are isotopic in![]() .
.
Since each ![]() divides
divides ![]() into a pair of complementary domains,
into a pair of complementary domains, ![]() and
and![]() , and since their polarity ±is determined by the inward/outward direction of
, and since their polarity ±is determined by the inward/outward direction of![]() , which changes continuously with
, which changes continuously with![]() , the ambient isotopy of
, the ambient isotopy of ![]() (which takes
(which takes ![]() to
to![]() ) must take
) must take ![]() to
to![]() . The isotopy
. The isotopy ![]() extends to an isotopy
extends to an isotopy![]() .
.
A similar argument applies to lower strata![]() . Indeed, with the isotopy
. Indeed, with the isotopy ![]() that takes
that takes ![]() to
to ![]() in place, consider the two sections,
in place, consider the two sections, ![]() and
and![]() , of the bundle
, of the bundle
![]() , both sections being transversal to the zero section of
, both sections being transversal to the zero section of![]() . Applying again Lemma 3.1, we conclude that the loci
. Applying again Lemma 3.1, we conclude that the loci ![]() and
and ![]() are isotopic in
are isotopic in ![]() (recall that these
(recall that these
loci are exactly the transversal intersections of two sections ![]() and
and ![]() of
of ![]() with its zero section).
with its zero section).
Again, an isotopy ![]() that takes
that takes ![]() to
to ![]() must take
must take ![]() to
to![]() . The isotopy
. The isotopy ![]() extends to an isotopy
extends to an isotopy ![]() which preserves the pair
which preserves the pair![]() . So, the pairs
. So, the pairs ![]() and
and ![]() are diffeomorphic via the composite isotopy
are diffeomorphic via the composite isotopy![]() .
.
This reasoning can be recycled to prove that all the pairs ![]() and
and ![]() are diffeomorphic via a single isotopy of
are diffeomorphic via a single isotopy of![]() . This argument is carried explicitly in the proof of Theorem 3.4 from [8] .
. This argument is carried explicitly in the proof of Theorem 3.4 from [8] . ![]()
Corollary 3.1 Let ![]() be a
be a ![]() -dimensional compact smooth manifold with boundary.
-dimensional compact smooth manifold with boundary.
Within each path-connected component of the space ![]() of generic fields, the numbers
of generic fields, the numbers![]() , as
, as
well as the numbers![]() , are constant.
, are constant.
Proof. The claim follows instantly from Theorem 3.1 and Lemma 2.2. ![]()
For a manifold ![]() with nonempty boundary, by deforming any given function
with nonempty boundary, by deforming any given function ![]() and its gradient-like field
and its gradient-like field![]() , we can expel the isolated
, we can expel the isolated ![]() -singularities from
-singularities from![]() . This can be achieved by the appropriate “finger moves” which originate at points of the boundary
. This can be achieved by the appropriate “finger moves” which originate at points of the boundary ![]() and engulf the isolated singularities of
and engulf the isolated singularities of![]() . The result of these manipulations leads to
. The result of these manipulations leads to
Lemma 3.2 Any connected ![]() -manifold
-manifold ![]() with a non-empty boundary admits a Morse function
with a non-empty boundary admits a Morse function ![]() with no critical points in the interior of
with no critical points in the interior of ![]() and such that
and such that ![]() is a Morse function. Such functions form an open nonempty set in the space
is a Morse function. Such functions form an open nonempty set in the space ![]() of all smooth functions on
of all smooth functions on![]() .
.
As a result, the gradient-like vector fields ![]() on
on ![]() form an open nonempty set in the space
form an open nonempty set in the space ![]() of all vector fields on
of all vector fields on![]() .
.
Proof. Let us sketch the main idea of the argument. Start with a Morse function![]() . Connect each critical point in the interior of
. Connect each critical point in the interior of ![]() by a smooth path to a point on the boundary in such a way that a system of non-intersecting paths is generated. Then delete from
by a smooth path to a point on the boundary in such a way that a system of non-intersecting paths is generated. Then delete from ![]() small regular neighborhoods of those paths (“dig a system of dead-end tunnels”) and restrict
small regular neighborhoods of those paths (“dig a system of dead-end tunnels”) and restrict ![]() to the remaining portion
to the remaining portion ![]() of
of![]() . Smoothen the entrances of the tunnels so that the boundary of
. Smoothen the entrances of the tunnels so that the boundary of ![]() will be a smooth manifold which is diffeomorphic to
will be a smooth manifold which is diffeomorphic to![]() . We got a nonsingular function
. We got a nonsingular function ![]() on
on![]() . A slight perturbation of
. A slight perturbation of ![]() on
on ![]() will not introduce critical points in the interior of
will not introduce critical points in the interior of ![]() and will deliver a Morse function on its boundary. Indeed, recall that the sets of Morse functions on
and will deliver a Morse function on its boundary. Indeed, recall that the sets of Morse functions on ![]() and
and ![]() are open and dense in the spaces
are open and dense in the spaces ![]() and
and ![]() of all smooth functions, respectively (for example, see [9] ). Of course
of all smooth functions, respectively (for example, see [9] ). Of course ![]() is an open condition imposed on a vector field on a compact manifold. On the other hand, if
is an open condition imposed on a vector field on a compact manifold. On the other hand, if![]() , then any field
, then any field![]() , sufficiently close to
, sufficiently close to![]() , will have the property
, will have the property![]() . The previous arguments show that the set of gradient-like non-vanishing fields is nonempty. So it is an open nonempty subspace in the space
. The previous arguments show that the set of gradient-like non-vanishing fields is nonempty. So it is an open nonempty subspace in the space ![]() of all all vector fields on
of all all vector fields on![]() .
. ![]()
Eliminating isolated critical points of a given function ![]() on a manifold with boundary is not “a free lunch”: the elimination introduces new critical points of the restricted function
on a manifold with boundary is not “a free lunch”: the elimination introduces new critical points of the restricted function![]() . This is a persistent theme throughout our program:
. This is a persistent theme throughout our program:
Expelling critical points of gradient flows from a manifold ![]() leaves crucial residual geometry on its boundary.
leaves crucial residual geometry on its boundary.
This boundary-confined geometry allows for a reconstruction of the topology of![]() .
.
Ideas like these will be developed in the future papers from this series. Meanwhile, the following lemma gives a taste of things to come.
Lemma 3.3 Let ![]() be a Morse function with no local extrema in the interior of a
be a Morse function with no local extrema in the interior of a ![]() -manifold
-manifold![]() . Then an elimination by a finger move5 of each
. Then an elimination by a finger move5 of each ![]() -critical point
-critical point ![]() of the Morse index
of the Morse index ![]() results in the introduction of
results in the introduction of ![]() new critical points of positive type and
new critical points of positive type and ![]() new critical points of negative type for the modified function
new critical points of negative type for the modified function![]() .
.
Proof. Let ![]() be a Morse singularity of
be a Morse singularity of ![]() in the interior of
in the interior of![]() . Denote by
. Denote by ![]() a sphere which bounds a
a sphere which bounds a
small disk ![]() centered on
centered on ![]() and such that
and such that ![]() is a Morse function. Without loss of generality, we can
is a Morse function. Without loss of generality, we can
assume that, in the Morse coordinates![]() ,
, ![]() is given by
is given by![]() , while
, while ![]() with all the
with all the ![]() being distinct. Then
being distinct. Then ![]() has only Morse-type singularities at the points where the coordinate axes pierce the sphere
has only Morse-type singularities at the points where the coordinate axes pierce the sphere![]() . With respect to the pair
. With respect to the pair![]() , these points come in two flavors: positive and negative. The two types are separated by the hypersurface of the cone
, these points come in two flavors: positive and negative. The two types are separated by the hypersurface of the cone
![]()
In the vicinity of![]() , the intersection
, the intersection ![]() is exactly the locus
is exactly the locus
![]()
so that the ![]() -gradient field
-gradient field ![]() (tangent to
(tangent to ![]() along
along![]() ) is transversal to
) is transversal to![]() , the product of two spheres. Therefore, in the vicinity of
, the product of two spheres. Therefore, in the vicinity of![]() ,
,![]() !
!
The function ![]() has exactly
has exactly ![]() critical points of the positive type and exactly
critical points of the positive type and exactly ![]()
critical points of the negative type. We shall denote these sets by ![]() and the two domains in which
and the two domains in which ![]() divides
divides![]() ―by
―by![]() .
.
Let ![]() be a local maximum of
be a local maximum of![]() . Note that it is possible to connect
. Note that it is possible to connect ![]() to a non-singular (for
to a non-singular (for![]() ) point
) point ![]() by a smooth path
by a smooth path ![]() along which f is increasing. Indeed, any non-extendable path
along which f is increasing. Indeed, any non-extendable path ![]() such that
such that ![]() either approaches a critical point or reaches the boundary
either approaches a critical point or reaches the boundary![]() . By a small perturbation, we can insure that
. By a small perturbation, we can insure that ![]() avoids all the (hyperbolic) critical points in the interior of X (by the hypothesis, f has no local maxima/minima in the interior of X). Thus
avoids all the (hyperbolic) critical points in the interior of X (by the hypothesis, f has no local maxima/minima in the interior of X). Thus ![]() can be extended until it reaches the boundary
can be extended until it reaches the boundary ![]() at a point
at a point![]() .
.
Drilling a narrow tunnel![]() , diffeomorphic to the product
, diffeomorphic to the product![]() , along
, along ![]() does not change the topology of X; the function
does not change the topology of X; the function ![]() retains almost the same list of singularities at the boundary as the function
retains almost the same list of singularities at the boundary as the function ![]() has: more accurately, the local maximum at
has: more accurately, the local maximum at ![]() disappears in
disappears in ![]() and a negative critical point of index 1 of
and a negative critical point of index 1 of ![]() appears near the
appears near the ![]() -end of the tunnel
-end of the tunnel![]() . Thus we have modified f and have eliminated the critical point
. Thus we have modified f and have eliminated the critical point ![]() in the interior of X at the cost of introducing on the boundary
in the interior of X at the cost of introducing on the boundary ![]() critical points of positive type and
critical points of positive type and ![]() critical points of negative type.
critical points of negative type. ![]()
Soon, motivated by Lemma 3.2, we will restrict our attention to nonsingular functions ![]() and their gradient-like fields
and their gradient-like fields![]() ―an open subset in the space of all gradient-like pairs
―an open subset in the space of all gradient-like pairs![]() ; but for now, let us investigate a more general case.
; but for now, let us investigate a more general case.
Consider Morse data![]() , where the field
, where the field ![]() is nonsingular along the boundary
is nonsingular along the boundary![]() . Extend
. Extend ![]() to
to ![]() and
and![]() , where C is some external collar of
, where C is some external collar of ![]() so that the extension
so that the extension ![]() is nonsingular in C. At each point
is nonsingular in C. At each point![]() , the
, the ![]() -flow defines a projection
-flow defines a projection ![]() of the germ of
of the germ of ![]() into the germ of the hypersurface
into the germ of the hypersurface![]() .
.
Let ![]() and
and ![]() denote the pure strata
denote the pure strata ![]() and
and![]() , respectively. At the points
, respectively. At the points![]() ,
, ![]() is a surjection; at the points of
is a surjection; at the points of![]() , it is a folding map; at the points
, it is a folding map; at the points![]() , it is a cuspidal map. Often we will refer to points
, it is a cuspidal map. Often we will refer to points ![]() by the smooth types of their
by the smooth types of their ![]() -projections.
-projections.
As the theorem and the corollary below testify, for a given function![]() , we enjoy a considerable freedom in changing the given Morse stratification
, we enjoy a considerable freedom in changing the given Morse stratification ![]() by deforming the
by deforming the ![]() -gradient-like field
-gradient-like field ![]() (cf. Section 3 in [5] ).
(cf. Section 3 in [5] ).
Theorem 3.2 Let ![]() be a compact smooth
be a compact smooth ![]() -manifold with nonempty boundary. Take a smooth function
-manifold with nonempty boundary. Take a smooth function ![]() with no singularities along
with no singularities along![]() , and let
, and let ![]() be its gradient-like field. Consider a stratification
be its gradient-like field. Consider a stratification
![]()
of X by compact smooth manifolds![]() , and let
, and let ![]() and
and ![]() denote the critical sets of the restrictions
denote the critical sets of the restrictions ![]() and
and![]() , respectively. Assume that the following properties are satisfied:
, respectively. Assume that the following properties are satisfied:
・ ![]() ,
,
・ ![]() and
and ![]() are regular embeddings for all
are regular embeddings for all![]() ,
,
・ for each ![]() the functions
the functions ![]() and
and ![]() have Morse-type critical points at the loci
have Morse-type critical points at the loci ![]() and
and![]() , respectively,
, respectively,
・ at the points of![]() ,
, ![]() and, at the points of
and, at the points of![]() ,
, ![]() , where
, where ![]() is the inward normal to
is the inward normal to ![]() in
in ![]() 6.
6.
Then, within the space of ![]() -gradient-like fields, there is a deformation of
-gradient-like fields, there is a deformation of ![]() into a new boundary generic gradient-like field
into a new boundary generic gradient-like field![]() , such that the stratification
, such that the stratification![]() , defined by
, defined by![]() , coincides with the given
, coincides with the given
stratification![]() .
.
Proof. We pick a Riemannian metric g in a collar U of ![]() in X so that
in X so that ![]() becomes the gradient field of f. Consider auxiliary vector fields
becomes the gradient field of f. Consider auxiliary vector fields![]() , where
, where ![]() denotes the orthogonal projection of
denotes the orthogonal projection of ![]() on the tangent spaces of closed manifold
on the tangent spaces of closed manifold![]() .
.
The construction of the desired field ![]() is inductive in nature, the induction being executed in increasing values of the index
is inductive in nature, the induction being executed in increasing values of the index![]() . Figure 4 illustrates a typical inductive step.
. Figure 4 illustrates a typical inductive step.
Assume that ![]() has been already constructed so that
has been already constructed so that ![]() and
and ![]() for all
for all![]() . This assumption implies that
. This assumption implies that ![]() is tangent to
is tangent to ![]() exactly along its boundary
exactly along its boundary ![]() for all
for all![]() . Along
. Along
![]() (and thus along
(and thus along![]() ), we decompose
), we decompose ![]() as
as![]() , where
, where
![]() .
.
The idea is to modify ![]() in the direction normal to
in the direction normal to ![]() in
in![]() , while keeping the rest of its components
, while keeping the rest of its components ![]() unchanged.
unchanged.
Denote by ![]() the tangent space of
the tangent space of ![]() at
at![]() . Let
. Let ![]() be the open half-space of
be the open half-space of ![]() positively
positively
spanned by the vectors that point inside of![]() . Let
. Let ![]() be half of the tangent space
be half of the tangent space![]() , defined by
, defined by![]() , where
, where![]() . We introduce the complementary to
. We introduce the complementary to ![]() and
and ![]() open half-spaces
open half-spaces ![]() and
and![]() .
.
At each point![]() , consider the open cone
, consider the open cone ![]() and, at each point
and, at each point![]() , the open cone
, the open cone ![]() (see Figure 4). These cones are non-empty, except perhaps at the points of
(see Figure 4). These cones are non-empty, except perhaps at the points of![]() , where
, where ![]() is anti-parallel to the inward normal
is anti-parallel to the inward normal ![]() of
of![]() . However, at
. However, at![]() ,
, ![]() , and at
, and at![]() ,
, ![]() due to the last bullet in the hypotheses of the theorem. Thus, for each
due to the last bullet in the hypotheses of the theorem. Thus, for each![]() , there is a number h so that the vector
, there is a number h so that the vector ![]() (this conclusion uses the the property
(this conclusion uses the the property ![]() on the set
on the set![]() ). Similarly, for each
). Similarly, for each![]() , there is a number h so that
, there is a number h so that![]() . By the partition of unity argument, which employes convexity of the cones
. By the partition of unity argument, which employes convexity of the cones![]() , there is a smooth function
, there is a smooth function ![]() which delivers the desired field uk along
which delivers the desired field uk along![]() . In order to insure the continuity of h and
. In order to insure the continuity of h and ![]() across the boundary
across the boundary![]() , we require
, we require![]() . Thus
. Thus ![]() on
on![]() .
.
Put![]() . Now,
. Now, ![]() for all
for all ![]() (these strata depend on the
(these strata depend on the![]() ’s only),
’s only),
and ![]() by the construction of
by the construction of![]() . Moreover,
. Moreover, ![]() for all
for all![]() . In fact,
. In fact, ![]() is tangent to
is tangent to ![]() along
along![]() . Note that this inductive argument should be modified for
. Note that this inductive argument should be modified for ![]() since
since ![]() is 0-dimensional.
is 0-dimensional.
We smoothly extend ![]() into a regular neighborhood V of
into a regular neighborhood V of ![]() in X. Abusing notations, we denote this extension by
in X. Abusing notations, we denote this extension by ![]() as well. The neighborhood
as well. The neighborhood ![]() is chosen so that there
is chosen so that there![]() .
.
To complete the proof of the inductive step![]() , we form the field
, we form the field![]() , where the functions
, where the functions ![]() deliver a smooth partition of unity subordinate to the cover
deliver a smooth partition of unity subordinate to the cover ![]() of X. Since
of X. Since ![]() defines a convex cone in the space of vector fields,
defines a convex cone in the space of vector fields, ![]() is a f-gradient-like field with the desired Morse stratification.
is a f-gradient-like field with the desired Morse stratification. ![]()
Theorem 3.2 has an immediate implication:
Corollary 3.2 Let ![]() be a Morse function and
be a Morse function and ![]() its boundary generic gradient-like field with the
its boundary generic gradient-like field with the
Morse stratification![]() . Assume that compact codimension zero submanifolds
. Assume that compact codimension zero submanifolds
![]() are chosen so that, for each
are chosen so that, for each![]() ,
, ![]() and
and![]() .
.
Then, within the space of f-gradient-like fields, it is possible to deform ![]() into a new gradient-like boundary generic field
into a new gradient-like boundary generic field![]() , such that the stratification
, such that the stratification ![]() coincides with the given stratification
coincides with the given stratification![]() .
.
Moreover,![]() .
.
In particular, if![]() , the claim is valid for any stratification
, the claim is valid for any stratification ![]() as above that terminates with
as above that terminates with![]() .
. ![]()
The next proposition (based on Corollary 3.2) shows that, for a given Morse function![]() , by an appropriate choice of gradient-like field
, by an appropriate choice of gradient-like field![]() , the Morse stratification
, the Morse stratification ![]() can be made topologically very simple and regular: namely, each stratum
can be made topologically very simple and regular: namely, each stratum ![]() is a disjoint union of
is a disjoint union of ![]() -dimensional disks. Moreover, when the boundary
-dimensional disks. Moreover, when the boundary ![]() is connected and
is connected and![]() , each stratum
, each stratum ![]() is a just a single disk.
is a just a single disk.
Corollary 3.3 Let ![]() be a Morse function on a compact
be a Morse function on a compact ![]() -manifold
-manifold![]() ,
, ![]() being nonsingular along the boundary
being nonsingular along the boundary![]() . We divide the connected components
. We divide the connected components ![]() of the boundary into two types, A and B. By definition, for type A, the singularity set
of the boundary into two types, A and B. By definition, for type A, the singularity set![]() , and for type B,
, and for type B,![]() .
.
Then any f-gradient-like field ![]() can be deformed, within the space of f-gradient-like fields, into a boundary generic field
can be deformed, within the space of f-gradient-like fields, into a boundary generic field ![]() so that, for each component
so that, for each component ![]() of type A and all
of type A and all![]() , the stratum
, the stratum ![]() is diffeomorphic to a disk
is diffeomorphic to a disk![]() . At the same time, for the components of type
. At the same time, for the components of type ![]() and all
and all![]() , the stratum
, the stratum![]() .
.
For the components of type![]() , in contrast, the 1-dimensional stratum
, in contrast, the 1-dimensional stratum ![]() is a finite union of arcs residing in the circle
is a finite union of arcs residing in the circle![]() . Moreover,
. Moreover, ![]() , the number or arcs in
, the number or arcs in![]() , and the number of points in
, and the number of points in ![]() are linked via the formula
are linked via the formula
![]()
, where![]() is the index of the field
is the index of the field![]() , and
, and ![]() is the number of boundary components of type
is the number of boundary components of type![]() .
.
Proof. If![]() , for each type A connected component
, for each type A connected component ![]() of
of![]() , the singularity set
, the singularity set ![]() can be included in a disk
can be included in a disk![]() . By Corollary 3.2, we can deform
. By Corollary 3.2, we can deform ![]() to a new
to a new ![]() -gradient-like field
-gradient-like field![]() , so that the new stratum
, so that the new stratum![]() . If
. If![]() , then the singularity set
, then the singularity set ![]() can be incapsulated in a disk
can be incapsulated in a disk![]() . By the same token, after still another deformation
. By the same token, after still another deformation ![]() of
of![]() , we can arrange for
, we can arrange for ![]() and
and![]() . This process repeats itself, unless the dimension of
. This process repeats itself, unless the dimension of ![]() becomes one. At its final stage,
becomes one. At its final stage, ![]() consists of several arcs which are contained in the circle
consists of several arcs which are contained in the circle![]() .
.
For each type ![]() connected component
connected component ![]() of
of![]() , by a similar reasoning, we can arrange for
, by a similar reasoning, we can arrange for![]() . Thus,
. Thus, ![]() for all
for all ![]() and
and![]() .
.
Therefore, letting ![]() for all
for all ![]() in Corollary 3.2, we have established all the claims of the corollary, but the last one.
in Corollary 3.2, we have established all the claims of the corollary, but the last one.
Since ![]() and
and ![]() both are the gradient-like fields for the same Morse function
both are the gradient-like fields for the same Morse function![]() , their indexes,
, their indexes, ![]() and
and![]() , are equal. Thus we get
, are equal. Thus we get
![]()
, where ![]() is the contribution of all the disk-shaped strata
is the contribution of all the disk-shaped strata ![]() to the Morse Formula (2).
to the Morse Formula (2).
Recall that, by Corollary 4.4 [5] , for any 3-fold ![]() and a boundary generic field
and a boundary generic field ![]() on it, we get
on it, we get![]() , provided
, provided![]() . Thus, as a positive
. Thus, as a positive ![]() increases, the boundary of the disk
increases, the boundary of the disk ![]() becomes more “wavily”.
becomes more “wavily”.
For example, by attaching many 2-handles to the boundary of a 2-disk, we get a 2-fold X with a big value ![]() of
of![]() . On such
. On such![]() ,
, ![]() for any gradient-like field
for any gradient-like field ![]() such that
such that![]() .
.
These examples motivate the following question.
Question 3.1 For boundary generic gradient-like fields ![]() with a fixed value
with a fixed value ![]() of the index
of the index ![]() and a
and a
disk-shaped stratification ![]() as in Corollary 3.3, what is the minimum
as in Corollary 3.3, what is the minimum ![]() of
of![]() ?
? ![]()
Evidently, such number ![]() is an invariant of the diffeomorphism type of
is an invariant of the diffeomorphism type of![]() . It seems that
. It seems that ![]() is semi-additive under the connected sum operation: that is,
is semi-additive under the connected sum operation: that is,
![]()
4. Boundary Convexity and Concavity of Vector Fields
We are ready to introduce pivotal concepts of the stratified convexity and concavity for smooth vector fields on manifolds with boundary.
Definition 4.1 Given a boundary generic vector field ![]() (see Definition 2.1), we say that
(see Definition 2.1), we say that ![]() is boundary
is boundary ![]() -convex, if
-convex, if![]() . In particular, if
. In particular, if![]() , we say that
, we say that ![]() is boundary
is boundary ![]() -convex, or just boundary convex.
-convex, or just boundary convex.
We say that ![]() is boundary s-concave, if
is boundary s-concave, if![]() . In particular, if
. In particular, if![]() , we say that
, we say that ![]() is boundary 2-concave, or just boundary concave.
is boundary 2-concave, or just boundary concave. ![]()
Example 4.1. Assume that a compact manifold X is defined as a 0-dimensional submanifold in the interior of a Riemannian manifold![]() , given by an inequality
, given by an inequality![]() , where
, where ![]() is a smooth function with 0 being a regular value. Then the boundary convexity of a gradient field
is a smooth function with 0 being a regular value. Then the boundary convexity of a gradient field ![]() in X can be expressed in terms of the Hessian matrix
in X can be expressed in terms of the Hessian matrix ![]() by the inequality
by the inequality
![]()
at all points![]() , where
, where ![]() is tangent to
is tangent to![]() . If
. If
![]()
, where ![]() is tangent to
is tangent to![]() , then the field
, then the field ![]() is boundary concave.
is boundary concave. ![]()
Example 4.2. According to the argument in Lemma 3.3, the complement to a small convex (in the Morse coordinates) disk, centered at a Morse type ![]() -critical point, is boundary concave with respect to the gradient field
-critical point, is boundary concave with respect to the gradient field![]() . In fact, the field
. In fact, the field ![]() is both boundary 3-concave and 3-convex! So, if
is both boundary 3-concave and 3-convex! So, if ![]() is a Morse function on a closed manifold Y with a critical set
is a Morse function on a closed manifold Y with a critical set![]() , then the complement X in Y to a small locally convex neighborhood of
, then the complement X in Y to a small locally convex neighborhood of ![]() admits a boundary concave
admits a boundary concave ![]() -gradient-like field (with
-gradient-like field (with![]() )!
)! ![]()
Theorem 4.1 below belongs to a family of results which we call “holographic” (see also and Theorem 4.2). The intension in such results is to reconstruct some structures on the “bulk” ![]() (or even the space
(or even the space ![]() itself) from the appropriate flow-generated structures (“observables”) on its boundary
itself) from the appropriate flow-generated structures (“observables”) on its boundary![]() . A paper from this series will be devoted entirely to the phenomenon of holography for nonsingular gradient flows.
. A paper from this series will be devoted entirely to the phenomenon of holography for nonsingular gradient flows.
In Theorem 4.1, we describe how some boundary-confined interactions between the critical points of a given function ![]() of opposite polarities can serve as an indicator of the convexity/concavity of the gradient field
of opposite polarities can serve as an indicator of the convexity/concavity of the gradient field ![]() in X (recall that the convexity/concavity properties of the
in X (recall that the convexity/concavity properties of the ![]() -flow do require knowing the field in the vicinity of
-flow do require knowing the field in the vicinity of ![]() in X!).
in X!).
Theorem 4.1 Let![]() ,
, ![]() be Morse functions and
be Morse functions and ![]() and
and ![]() their gradient fields with respect to a Riemannian metric
their gradient fields with respect to a Riemannian metric ![]() on X and its restriction to
on X and its restriction to![]() , respectively. Assume that
, respectively. Assume that ![]() is boundary generic.
is boundary generic.
If![]() , then there is no ascending
, then there is no ascending ![]() -trajectory
-trajectory![]() , such that
, such that
![]()
(both critical sets ![]() depend only on
depend only on![]() ).
).
Conversely, if for a given ![]() -gradient pair
-gradient pair![]() , no such
, no such ![]() -trajectory
-trajectory ![]() exists, then one can deform
exists, then one can deform ![]() to a new boundary generic pair
to a new boundary generic pair ![]() of the
of the ![]() -gradient type so that
-gradient type so that![]() . Moreover, the fields
. Moreover, the fields ![]() and
and ![]() on
on ![]() can be chosen to be arbitrary close in the
can be chosen to be arbitrary close in the ![]() -topology.
-topology.
In particular, if ![]() (as sets), then X admits a boundary generic and convex f-gradient-like field
(as sets), then X admits a boundary generic and convex f-gradient-like field
![]() ; similarly, if
; similarly, if![]() , then X admits a boundary generic and concave f-gradient-like field
, then X admits a boundary generic and concave f-gradient-like field![]() .
.
Proof. First consider the convex case, that is, the relation between the property ![]() and the absence of an ascending
and the absence of an ascending ![]() -trajectory
-trajectory ![]() which connects
which connects ![]() to
to![]() .
.
Consider the function![]() , defined via the formula
, defined via the formula![]() , where
, where ![]() denotes a unitary field inward normal to
denotes a unitary field inward normal to ![]() in
in![]() . Since
. Since ![]() is boundary generic, zero is a regular value of
is boundary generic, zero is a regular value of![]() . Then
. Then
![]()
and![]() .
.
If an ascending ![]() -trajectory
-trajectory![]() , which links
, which links ![]() with
with![]() , does exist, it must cross somewhere the boundary
, does exist, it must cross somewhere the boundary ![]() of
of![]() . Since the field
. Since the field ![]() is an orthogonal projection of
is an orthogonal projection of ![]() on
on![]() , the two fields must agree at any point
, the two fields must agree at any point![]() ―the locus where
―the locus where ![]() is tangent to
is tangent to![]() . Thus,
. Thus, ![]() is the gradient of
is the gradient of ![]() at
at![]() . Therefore, as
. Therefore, as ![]() crosses from
crosses from ![]() into
into ![]() at
at![]() , in its vicinity, the arc
, in its vicinity, the arc ![]() lies below the arc
lies below the arc ![]() (see Figure 5). By the definition of the locus
(see Figure 5). By the definition of the locus![]() , such crossing
, such crossing ![]() belongs to
belongs to![]() . Therefore,
. Therefore, ![]() , contrary to the theorem hypothesis.
, contrary to the theorem hypothesis.
On the other hand, if no such ![]() -trajectory
-trajectory ![]() exists, then we claim the existence of a codimension one closed submanifold
exists, then we claim the existence of a codimension one closed submanifold![]() , which separates
, which separates ![]() in two manifolds,
in two manifolds, ![]() and
and ![]()
![]() , such that the vector field
, such that the vector field![]() , or rather its perturbation
, or rather its perturbation![]() , is transversal to
, is transversal to ![]() and points outward of
and points outward of![]() . Indeed, for each critical point
. Indeed, for each critical point![]() , in the local Morse coordinates
, in the local Morse coordinates ![]() on
on![]() , consider a small closed
, consider a small closed ![]()
-disk ![]() centered on the critical point x. Denote by
centered on the critical point x. Denote by ![]() the closure of the union of downward trajectories of the
the closure of the union of downward trajectories of the ![]() -flow passing through the points of
-flow passing through the points of ![]() (see Figure 6, the left diagram). Let
(see Figure 6, the left diagram). Let ![]() be the union
be the union ![]() (see Figure 6, the right diagram).
(see Figure 6, the right diagram).
Since we assume that no descending ![]() -trajectory
-trajectory ![]() links a point of
links a point of ![]() to a point of
to a point of![]() , we can choose
, we can choose
the disks ![]() so small that the set
so small that the set ![]() belongs to the complement
belongs to the complement![]() .
.
For each![]() , the zero cone
, the zero cone ![]() of the Morse function
of the Morse function ![]() separates the sphere
separates the sphere
![]() into two handles,
into two handles, ![]() and
and ![]() (each being a product of a sphere with a disk). We denote by
(each being a product of a sphere with a disk). We denote by ![]() the handle in
the handle in ![]() whose spherical core is formed by the intersection of the unstable disk through
whose spherical core is formed by the intersection of the unstable disk through ![]() with the sphere
with the sphere![]() . Then, by definition, the set
. Then, by definition, the set ![]() is a collection of downward trajectories through the points of
is a collection of downward trajectories through the points of ![]() union with
union with![]() . Note that the downward trajectories from a different set
. Note that the downward trajectories from a different set ![]() could enter the disk
could enter the disk ![]() only through the complementary handle
only through the complementary handle ![]() in its boundary. As a result,
in its boundary. As a result, ![]() is a manifold whose piecewise smooth boundary could have corners (see Figure 6, the right diagram). Similarly,
is a manifold whose piecewise smooth boundary could have corners (see Figure 6, the right diagram). Similarly, ![]() is a domain in
is a domain in ![]() whose boundary is piecewise smooth manifold with corners.
whose boundary is piecewise smooth manifold with corners.
Since ![]() consists of the downward trajectories of
consists of the downward trajectories of![]() , if
, if![]() , then any point
, then any point ![]() which can be reached from
which can be reached from ![]() following the field
following the field ![]() (for short, “is below
(for short, “is below![]() ”) belongs to
”) belongs to ![]() as well. Therefore the boundary
as well. Therefore the boundary ![]() is assembled either from downward trajectories or from singletons; the singletons are contributed by some portions of
is assembled either from downward trajectories or from singletons; the singletons are contributed by some portions of ![]() where
where ![]() points outside of the relevant disk
points outside of the relevant disk![]() . Thus either
. Thus either ![]() is tangent to
is tangent to![]() , or it points outside
, or it points outside![]() .
.
![]()
Figure 6. The set ![]() (the left diagram) and the set
(the left diagram) and the set ![]() (the right diagram).
(the right diagram).
Away from![]() ,
, ![]() is of the
is of the ![]() -gradient type. Thus, in each tangent space
-gradient type. Thus, in each tangent space![]() , where
, where![]() , there is an open cone
, there is an open cone ![]() comprised of
comprised of ![]() -gradient type vectors, and
-gradient type vectors, and![]() . Therefore, in the vicinity of
. Therefore, in the vicinity of![]() , we can perturb
, we can perturb ![]() to a new field
to a new field ![]() of the
of the ![]() -gradient type, so that
-gradient type, so that ![]() points strictly outside
points strictly outside ![]() and still
and still ![]() for all
for all![]() . It is possible to smoothen the boundary
. It is possible to smoothen the boundary ![]() so that, with respect to a new smooth boundary
so that, with respect to a new smooth boundary![]() , the field
, the field ![]() still points outside
still points outside![]() , the new domain bounded by
, the new domain bounded by![]() , and
, and ![]() for all
for all![]() .
.
Note that if![]() , then
, then ![]() can serve as a separator.
can serve as a separator.
Let ![]() and
and![]() . With the separator
. With the separator ![]() in place, consider a smooth function
in place, consider a smooth function ![]() with the properties:
with the properties:
1) zero is a regular value of![]() , and
, and![]() ,
,
2)![]() ,
, ![]() ,
,
3) ![]() in a neighborhood of
in a neighborhood of![]() ,
,
4)![]() , where
, where ![]() is the inward normal to
is the inward normal to ![]() in
in![]() .
.
Note that the field ![]() points inside of
points inside of ![]() along
along ![]() and outside of
and outside of ![]() along
along![]() . It also points outside of
. It also points outside of ![]() along
along![]() . As a result, we conclude that
. As a result, we conclude that ![]() and
and![]() ; in other words,
; in other words, ![]() is boundary convex. Note that
is boundary convex. Note that ![]() can be chosen arbitrary close to
can be chosen arbitrary close to![]() . Ineeded, employing Theorem 3.2, we can perturb
. Ineeded, employing Theorem 3.2, we can perturb ![]() to insure its genericity with respect to the pair
to insure its genericity with respect to the pair![]() , and thus the boundary genericity of
, and thus the boundary genericity of ![]() itself.
itself.
The argument in the concave case, which deals with the relation between the property ![]() and the absence of an ascending
and the absence of an ascending ![]() -trajectory
-trajectory![]() , connecting
, connecting ![]() to
to![]() , is analogous. We just need to switch the polarities of the relevant sets.
, is analogous. We just need to switch the polarities of the relevant sets. ![]()
Now we need to introduce a number basic notions to which we will return on many occasions in the future.
Definition 4.2 Let ![]() be a differential
be a differential ![]() -form on a manifold
-form on a manifold![]() .
.
We say that a path ![]() is
is ![]() -positive (
-positive (![]() -negative), if,
-negative), if, ![]() for all values of the parameter
for all values of the parameter![]() .
. ![]()
Definition 4.3 Let ![]() be a closed differential 1-form on a manifold
be a closed differential 1-form on a manifold![]() , equipped with a Riemannian metric
, equipped with a Riemannian metric![]() . We say that a vector field
. We say that a vector field ![]() on
on ![]() is the gradient of
is the gradient of ![]() (and denote it “
(and denote it “![]() ”), if
”), if ![]() for any vector field
for any vector field ![]() on
on![]() .
. ![]()
Definition 4.4 Let ![]() be a differential 1-form on a manifold Y and let
be a differential 1-form on a manifold Y and let ![]() be the set of points
be the set of points![]() , where
, where ![]() is the zero map. Assume that
is the zero map. Assume that ![]() for some smooth function
for some smooth function ![]() in the vicinity of
in the vicinity of![]() .
.
We say that a vector field ![]() is of
is of ![]() -gradient type if
-gradient type if ![]() on
on ![]() and
and ![]() in the vicinity of
in the vicinity of![]() . Here
. Here ![]() is some Riemannian metric in the vicinity of
is some Riemannian metric in the vicinity of ![]() (cf. Definition 2.2).
(cf. Definition 2.2). ![]()
We are in position to formulate a generalization of Theorem 4.1 for closed differential ![]() -forms―another instance of somewhat weaker “holographic phenomenon”, this time for fields which may not be gradient-like globally.
-forms―another instance of somewhat weaker “holographic phenomenon”, this time for fields which may not be gradient-like globally.
Theorem 4.2 Let ![]() be a closed 1-form on a compact manifold
be a closed 1-form on a compact manifold![]() , equipped with a Riemannian metric
, equipped with a Riemannian metric![]() . Assume that
. Assume that ![]() and
and ![]() have only Morse-type singularities. Let the gradient
have only Morse-type singularities. Let the gradient ![]() be a boundary
be a boundary
generic field, and let![]() .
.
If![]() , then there is no
, then there is no ![]() -ascending
-ascending ![]() -trajectory
-trajectory![]() , such that
, such that
![]()
Assume that there exists a codimension one submanifold![]() , which separates
, which separates ![]() and
and ![]() and such that the field
and such that the field ![]() is transversal to
is transversal to ![]() and points outwards/inwards of the domain in
and points outwards/inwards of the domain in ![]() that is bounded by
that is bounded by ![]() and contains
and contains![]() . Then one can deform the
. Then one can deform the ![]() -gradient vector fields
-gradient vector fields ![]() to a new boundary generic pair
to a new boundary generic pair ![]() of the
of the ![]() -gradient type so that
-gradient type so that![]() .
.
Proof. The ![]() -gradient fields
-gradient fields ![]() on
on ![]() are characterized by the property
are characterized by the property![]() , valid on the
, valid on the
locus where![]() . Usually, in this setting, we do not have a natural choice for the wall
. Usually, in this setting, we do not have a natural choice for the wall ![]() which would separate the singularities of opposite polarities
which would separate the singularities of opposite polarities ![]() and
and ![]() and such that the field
and such that the field ![]() would be transversal to N. It seems unlikely that the absence of an ascending
would be transversal to N. It seems unlikely that the absence of an ascending ![]() -trajectory which links
-trajectory which links ![]() with
with ![]() is sufficient to guarantee the existence of a separator N. However, in the presence of such separator N, the arguments are identical with the ones employed in the proof of Theorem 4.1.
is sufficient to guarantee the existence of a separator N. However, in the presence of such separator N, the arguments are identical with the ones employed in the proof of Theorem 4.1. ![]()
Remark 4.1. In Theorem 4.1 and Theorem 4.2, the partition ![]() of the singular set
of the singular set ![]() must satisfy some basic relations:
must satisfy some basic relations:
![]()
![]()
These relations reflect the fact that ![]() when
when![]() , and
, and ![]() when
when![]() .
. ![]()
Given a metric ![]() on a Riemannian
on a Riemannian ![]() -manifold
-manifold![]() , let us recall a definition of the Hodge Star Operator
, let us recall a definition of the Hodge Star Operator ![]() (see [19] ).
(see [19] ).
Pick a local basis ![]() of 1-forms in
of 1-forms in ![]() and consider the associated basis
and consider the associated basis
![]()
of![]() , where
, where ![]() and the symbol “
and the symbol “![]() “ stands for omitting the
“ stands for omitting the ![]() -th form
-th form ![]() from the product
from the product![]() .
.
Assume that, in the dual to ![]() basis
basis ![]() of
of![]() , the metric
, the metric ![]() is locally given by a matrix
is locally given by a matrix![]() . Then the matrix
. Then the matrix ![]() of the
of the ![]() -operator in the bases
-operator in the bases![]() ,
, ![]() is given by the formula
is given by the formula
![]() (5)
(5)
whence![]() .
.
Definition 4.5 A closed differential 1-form ![]() on a compact manifold Y is called intrinsically harmonic if there exists a Riemannian metric g on Y such that the form
on a compact manifold Y is called intrinsically harmonic if there exists a Riemannian metric g on Y such that the form ![]() is closed.
is closed. ![]()
Example 4.3. Let ![]() be a closed smooth manifold and
be a closed smooth manifold and ![]() a smooth map with isolated Morse-type singularities. Consider the closed
a smooth map with isolated Morse-type singularities. Consider the closed ![]() -form
-form![]() , the pull-back of the canonic 1-form
, the pull-back of the canonic 1-form ![]() on the circle
on the circle![]() . Assume that one of the
. Assume that one of the ![]() -fibers,
-fibers, ![]() , is connected. Then
, is connected. Then ![]() is intrinsically harmonic [20] .
is intrinsically harmonic [20] . ![]()
Let ![]() denote the singularity set of a closed 1-form
denote the singularity set of a closed 1-form ![]() on a compact manifold Y. We assume that
on a compact manifold Y. We assume that![]() .
.
By Calabi’s Proposition 1 [19] , ![]() is intrinsically harmonic if and only if through every point
is intrinsically harmonic if and only if through every point ![]() there is a
there is a ![]() -positive path
-positive path ![]() which either is a loop, or which starts and terminates at the boundary
which either is a loop, or which starts and terminates at the boundary![]() .
.
Theorem 4.3 Let ![]() be a closed
be a closed ![]() -form on a Riemannian manifold
-form on a Riemannian manifold![]() , such that
, such that![]() . Assume that
. Assume that![]() , the restriction of
, the restriction of ![]() to
to![]() , is a harmonic form7.
, is a harmonic form7.
Then the gradient field ![]() is not boundary convex or boundary concave (that is,
is not boundary convex or boundary concave (that is, ![]() and
and![]() ). Thus, if
). Thus, if ![]() is connected, then
is connected, then![]() .
.
Proof. We abbreviate ![]() to
to ![]() and
and ![]() to
to![]() . Here
. Here ![]() is the *-operator on the boundary of X
is the *-operator on the boundary of X
with respect to the given Riemannian metric ![]() on
on![]() .
.
If ![]() is a closed
is a closed ![]() -form on
-form on![]() , then by the Stokes Theorem,
, then by the Stokes Theorem,
![]()
However, for a concave/convex gradient field![]() , the
, the ![]() -form
-form![]() , being restricted to
, being restricted to![]() , is proportional to the volume form of
, is proportional to the volume form of ![]() with negative/positive functional coefficient. Indeed, at the points of
with negative/positive functional coefficient. Indeed, at the points of![]() , the angle between
, the angle between ![]() and the normal
and the normal ![]() to
to ![]() in
in ![]() is acute, while it is obtuse at the
is acute, while it is obtuse at the
points of![]() . Therefore,
. Therefore, ![]() when either
when either ![]() or
or![]() . The resulting contradic-
. The resulting contradic-
tion proves that ![]() and
and![]() .
.
Therefore, when ![]() is connected, then
is connected, then ![]() and
and ![]() must share the common nonempty boundary
must share the common nonempty boundary![]() ―the gradient field
―the gradient field ![]() must have cuspidal points.
must have cuspidal points. ![]()
Example 4.4. Let ![]() be a compact smooth manifold and
be a compact smooth manifold and ![]() a smooth map with isolated Morse- type singularities. Consider the closed
a smooth map with isolated Morse- type singularities. Consider the closed ![]() -form
-form![]() , the pull-back of the canonic 1-form
, the pull-back of the canonic 1-form ![]() on the circle
on the circle![]() . Assume that one of the fibers of the map
. Assume that one of the fibers of the map ![]() is connected. Then there exists a metric
is connected. Then there exists a metric ![]()
on ![]() such that the form
such that the form ![]() is harmonic ([19] [20] ). Consider the gradient field
is harmonic ([19] [20] ). Consider the gradient field![]() .
.
Then by Theorem 4.3, ![]() and
and ![]() for any metric
for any metric ![]() that “harmonizes”
that “harmonizes”![]() .
. ![]()
Definition 4.6 A non-vanishing vector field ![]() on a compact manifold
on a compact manifold ![]() is called traversing if each
is called traversing if each ![]() -trajectory is either a closed segment or a singleton which belongs to
-trajectory is either a closed segment or a singleton which belongs to![]() .
. ![]()
Remark 4.2. The definition excludes fields with zeros in ![]() (they will generate trajectories that are homeomorphic to open or semi-open intervals) and fields with closed trajectories. Note that all gradient-like fields of nonsingular functions are traversing, but the gradient-like fields of nonsingular closed 1-forms may not be traversing!
(they will generate trajectories that are homeomorphic to open or semi-open intervals) and fields with closed trajectories. Note that all gradient-like fields of nonsingular functions are traversing, but the gradient-like fields of nonsingular closed 1-forms may not be traversing! ![]()
Lemma 4.1 Any traversing vector field is of the gradient type.
Proof. Let ![]() be a traversing field on X. We extend the pair
be a traversing field on X. We extend the pair ![]() to a pair
to a pair ![]() so that X is properly contained in
so that X is properly contained in ![]() and
and![]() .
.
Then every ![]() -trajectory
-trajectory ![]() has a local transversal compact section
has a local transversal compact section ![]() of the
of the ![]() -flow. We can choose
-flow. We can choose ![]() to be diffeomorphic to a n-dimensional ball with its center at the singleton
to be diffeomorphic to a n-dimensional ball with its center at the singleton![]() . We denote by
. We denote by ![]() the union of
the union of ![]() -trajectories through
-trajectories through![]() .
.
For each ![]() -trajectory
-trajectory![]() , there exists a section
, there exists a section ![]() so that the set
so that the set ![]() contains a compact cylinder
contains a compact cylinder
![]() , where
, where ![]() are positive constants (which depend on
are positive constants (which depend on![]() ), with the properties:
), with the properties:
1)![]() ,
,
2) for any ![]() -trajectory
-trajectory ![]() through
through![]() , the intersection
, the intersection ![]() is a segment,
is a segment,
3) the point ![]() belongs to the interior of segment
belongs to the interior of segment![]() .
.
Then the collection ![]() forms a cover of
forms a cover of![]() . Since
. Since ![]() is compact, we can choose
is compact, we can choose
a finite subcover ![]() of
of![]() .
.
For each ![]() and the corresponding section
and the corresponding section![]() , we produce a smooth function
, we produce a smooth function ![]() by integrating the vector field
by integrating the vector field ![]() and using
and using ![]() as the initial location for the integration.
as the initial location for the integration.
More accurately, let
![]()
be the parametrization of a typical trajectory ![]() , such that
, such that
![]()
for all ![]() and
and ![]() . This bijective parametrization introduces a smooth product structure
. This bijective parametrization introduces a smooth product structure
![]()
by the formula ![]() .
.
We define a smooth function ![]() by the formula
by the formula ![]() and denote it (quite appropriately)
and denote it (quite appropriately)
by the symbol ![]() .
.
Let ![]() be a smooth non-negative function that vanishes only on the boundary
be a smooth non-negative function that vanishes only on the boundary ![]() . Let
. Let
![]() denote the composition of the
denote the composition of the ![]() -directed projection
-directed projection ![]() with the function
with the function
![]() . Since
. Since ![]() vanishes on
vanishes on ![]() , the function extends smoothly on X to produce a smooth function
, the function extends smoothly on X to produce a smooth function
![]() with the support in
with the support in ![]() .
.
Now consider the smooth function
![]() (6)
(6)
It is well-defined on X. Let us compute its![]() -directional derivative:
-directional derivative:
![]() (7)
(7)
Let us explain Formula (7). By the very definition of ![]() , it is constant on each
, it is constant on each![]() -trajectory, so that
-trajectory, so that
![]() . Also,
. Also, ![]() in
in ![]() . At the same time,
. At the same time, ![]() , since
, since ![]() and
and
![]() increases in the direction of
increases in the direction of ![]() . Finally, each
. Finally, each ![]() belongs to the interior of some set
belongs to the interior of some set ![]() .
.
Therefore, ![]() , so that
, so that ![]() is a gradient-like field for
is a gradient-like field for ![]() .
. ![]()
Corollary 4.1 Let X be a smooth compact manifold with boundary. Then ![]() ―the space of traversing vector fields on X―is nonempty and coincides with the intersection
―the space of traversing vector fields on X―is nonempty and coincides with the intersection ![]() , where
, where ![]() denotes the space of gradient-like fields, and
denotes the space of gradient-like fields, and ![]() the space of all non-vanishing fields on X.
the space of all non-vanishing fields on X.
Proof. By definition, any traversing field ![]() on X does not vanish. By Lemma 4.1,
on X does not vanish. By Lemma 4.1, ![]() must be of the gradient type. Thus
must be of the gradient type. Thus
![]()
On the other hand, for a compact X with a gradient-like ![]() , each
, each ![]() -trajectory
-trajectory ![]() through
through ![]() must reach the boundary in both finite positive and negative times (since it is controlled by some Lyapunov function f).
must reach the boundary in both finite positive and negative times (since it is controlled by some Lyapunov function f).
As a result,
![]()
It remains to show that ![]() . By Lemma 3.2,
. By Lemma 3.2, ![]() , which implies that
, which implies that ![]() .
. ![]()
There are simple topological obstructions to boundary convexity of any gradient-like nonvanishing field on a given manifold X. The next lemma testifies that the existence of boundary convex traversing fields ![]() imposes severe restrictions on the topology of the manifold X.
imposes severe restrictions on the topology of the manifold X.
Lemma 4.2 A connected ![]() -manifold X admits a boundary convex traversing8 field
-manifold X admits a boundary convex traversing8 field ![]() , if and only if, X is diffeomorphic to a product of a connected compact n-manifold and a segment, the corners of the product being smoothly rounded.
, if and only if, X is diffeomorphic to a product of a connected compact n-manifold and a segment, the corners of the product being smoothly rounded.
Proof. Indeed, if such convex ![]() exists,
exists, ![]() must be a deformation retract of X: just use the down flow to produce the retraction. Therefore, when
must be a deformation retract of X: just use the down flow to produce the retraction. Therefore, when ![]() , then X is homeomorphic to the quotient space
, then X is homeomorphic to the quotient space
![]() , where the equivalence relation “
, where the equivalence relation “![]() ” is defined by collapsing each segment
” is defined by collapsing each segment ![]()
to a point. If we round the corners generated in the collapse, we will get a diffeomorphism between X and the “lens” ![]() (see Figure 7).
(see Figure 7).
On the other hand, any product ![]() , whose conners
, whose conners ![]() being rounded, admits a field of the desired boundary convex type.
being rounded, admits a field of the desired boundary convex type. ![]()
Corollary 4.2 For all ![]() , any smooth compact contractible
, any smooth compact contractible ![]() -manifold X, which admits a boundary convex traversing field, is diffeomorphic to the standard
-manifold X, which admits a boundary convex traversing field, is diffeomorphic to the standard ![]() -disk.
-disk.
![]()
Figure 7. The existence of a traversing boundary convex field ![]() (the constant vertical field) on a (n + 1)-manifold X (the ellipsoid-bounded solid) implies that topologically it is a product of a compact n-manifold Y (the elliptical shadow) with an interval.
(the constant vertical field) on a (n + 1)-manifold X (the ellipsoid-bounded solid) implies that topologically it is a product of a compact n-manifold Y (the elliptical shadow) with an interval.
Proof. By Lemma 4.2, X is diffeomorphic to a product of a fake ![]() -disk Y with
-disk Y with ![]() , the corners of the product being rounded.
, the corners of the product being rounded.
For ![]() , by Perelman’s results [21] [22] , Y is diffeomorphic to the standard 3-disk. Thus X is diffeomorphic to the standart 4-disk.
, by Perelman’s results [21] [22] , Y is diffeomorphic to the standard 3-disk. Thus X is diffeomorphic to the standart 4-disk.
For ![]() , we do not know whether Y is a standard 4-disk.
, we do not know whether Y is a standard 4-disk.
For ![]() , the h-cobordism theorem [23] implies that any fake n-disk is diffeomorphic to the standard disk.
, the h-cobordism theorem [23] implies that any fake n-disk is diffeomorphic to the standard disk.
This leaves only the case of 5-dimensional X wide open. ![]()
We notice that ![]() is an obstruction to finding boundary convex traversing
is an obstruction to finding boundary convex traversing ![]() on a
on a ![]() -dimensional manifold
-dimensional manifold ![]() with a connected boundary.
with a connected boundary.
Corollary 4.3 Let X be a smooth connected compact ![]() -manifold with boundary, which admits a boundary convex traversing field.
-manifold with boundary, which admits a boundary convex traversing field.
If ![]() , then X is diffeomorphic to the product
, then X is diffeomorphic to the product ![]() , where Y is a closed manifold.
, where Y is a closed manifold.
In particular, no connected X with boundary ![]() , whose number of connected components differs from two, and with the property
, whose number of connected components differs from two, and with the property ![]() admits a boundary convex traversing field.
admits a boundary convex traversing field.
Proof. If such boundary convex traversing field ![]() exists,
exists, ![]() must be a deformation retract of X. Therefore, for a connected X,
must be a deformation retract of X. Therefore, for a connected X, ![]() must be connected as well.
must be connected as well.
On the other hand, if ![]() , then the connected
, then the connected ![]() must be of a homotopy type of a
must be of a homotopy type of a ![]() -dimensional complex. In such a case, the groups
-dimensional complex. In such a case, the groups ![]() must vanish.
must vanish.
Thus when ![]() and
and ![]() is boundary convex, the only remaining option is
is boundary convex, the only remaining option is ![]() , which implies that
, which implies that ![]() ―the manifold
―the manifold ![]() is closed. In such a case, X is a product of a connected closed
is closed. In such a case, X is a product of a connected closed ![]() -manifold with an interval; so the boundary
-manifold with an interval; so the boundary ![]() must be the union of two diffeomorphic components.
must be the union of two diffeomorphic components. ![]()
As with the boundary convex traversing fields, perhaps, there are topological obstructions to the existence of a boundary concave traversing field on a given manifold? At the present time, the contours of the universe of such obstructions are murky. We know only that the disk ![]() does not admit a non-vanishing boundary concave field (see Example 4.4).
does not admit a non-vanishing boundary concave field (see Example 4.4).
Lemma 4.3 If a boundary generic vector field ![]() on an even-dimensional compact orientable manifold
on an even-dimensional compact orientable manifold ![]() is boundary concave, then its index
is boundary concave, then its index
![]()
If a boundary generic vector field ![]() on an odd-dimensional compact orientable manifold X is boundary concave, then its index
on an odd-dimensional compact orientable manifold X is boundary concave, then its index
![]()
Thus, for all boundary concave fields ![]() with a fixed value of index
with a fixed value of index ![]() , the Euler number
, the Euler number ![]() is a topological invariant.
is a topological invariant.
Proof. For a boundary concave field ![]() ,
, ![]() . Therefore, the Morse Formula (2) reduces to the equation
. Therefore, the Morse Formula (2) reduces to the equation
![]() (8)
(8)
Recall that, for any orientable odd-dimensional manifold Y, ![]() . Therefore, when
. Therefore, when ![]() , we get
, we get ![]() . Thus formula (8) transforms into
. Thus formula (8) transforms into
![]()
For an odd-dimensional X, the closed manifold ![]() is odd-dimensional, so
is odd-dimensional, so ![]() . Therefore
. Therefore
![]() .
. ![]()
Corollary 4.4 Let X be a ![]() -dimensional oriented smooth and compact manifold with boundary.
-dimensional oriented smooth and compact manifold with boundary.
If ![]() , then for any boundary generic concave vector field
, then for any boundary generic concave vector field ![]() on X of index 0, the locus
on X of index 0, the locus ![]() contains at least
contains at least ![]() two-dimensional spheres.
two-dimensional spheres.
Proof. Since ![]() is a closed orientable 2-manifold, its Euler number is positive only if
is a closed orientable 2-manifold, its Euler number is positive only if ![]() contains sufficiently many 2-spheres. By Lemma 4.3,
contains sufficiently many 2-spheres. By Lemma 4.3, ![]() . Therefore
. Therefore ![]() contains at least
contains at least ![]() two-dimensional spheres.
two-dimensional spheres. ![]()
Example 4.4. Let ![]() , the 2-dimensional ball. If
, the 2-dimensional ball. If ![]() on X, then by the Morse formula,
on X, then by the Morse formula,
![]()
If ![]() consists of k arcs, then by this formula,
consists of k arcs, then by this formula, ![]() . At the same time,
. At the same time, ![]() . Therefore,
. Therefore, ![]() . So we conclude that
. So we conclude that ![]() does not admit a non-vanishing field with
does not admit a non-vanishing field with ![]() , that is, a boundary concave field.
, that is, a boundary concave field.
At the same time, if we delete any number of disjoint open disks from ![]() , the remaining surface X admits a concave non-vanishing gradient-like field: indeed, consider the radial field in an annulus A and delete from A any non-negative number of small round disks. The radial field
, the remaining surface X admits a concave non-vanishing gradient-like field: indeed, consider the radial field in an annulus A and delete from A any non-negative number of small round disks. The radial field ![]() on A, being restricted to X, is evidently of the gradient type and concave with respect to
on A, being restricted to X, is evidently of the gradient type and concave with respect to ![]() .
.
Note that, if a connected compact surface X admits a generic traversing concave field ![]() , then X is homeomorphic either to a thickening of a finite graph
, then X is homeomorphic either to a thickening of a finite graph ![]() whose vertexes all have valency 3, or to an annulus.
whose vertexes all have valency 3, or to an annulus. ![]()
In the previous example, we have seen that the disk ![]() does not admit a non-vanishing concave field. In contrast,
does not admit a non-vanishing concave field. In contrast, ![]() does admit a boundary generic concave non-vanishing field: just consider the restriction of the Hopf field
does admit a boundary generic concave non-vanishing field: just consider the restriction of the Hopf field ![]() on
on ![]() to the northern hemisphere
to the northern hemisphere ![]() . For the unitary disk
. For the unitary disk ![]() centered at the origin, informally, we can describe
centered at the origin, informally, we can describe ![]() as the sum of the velocity field of the solid
as the sum of the velocity field of the solid ![]() , spinning around the
, spinning around the ![]() -axis, with the solenoidal field of the loop
-axis, with the solenoidal field of the loop ![]() . However, this field
. However, this field ![]() is not of the traversing type: it has closed trajectories (residing in the solid torus
is not of the traversing type: it has closed trajectories (residing in the solid torus ![]() ).
).
These observations encourage us to formulate
Conjecture 4.1 The standard ![]() -disk
-disk![]() does not admit a traversing boundary concave vector field
does not admit a traversing boundary concave vector field![]() .
.
The construction of a boundary concave field on a 2-disk with holes (see Example 4.4) admits a simple generalization.
Example 4.5. Consider a closed ![]() -manifold Y. Let
-manifold Y. Let ![]() be compact submanifolds also of dimension
be compact submanifolds also of dimension ![]() . Let
. Let ![]() . We pick
. We pick ![]() disjointed close intervals
disjointed close intervals ![]() in the interval
in the interval ![]() . Then we form the product
. Then we form the product ![]() . By rounding the corners of
. By rounding the corners of ![]() , we get a
, we get a ![]() -manifold
-manifold ![]() so that each segment
so that each segment ![]() , where
, where ![]() , hits
, hits ![]() along a closed segment, and each segment
along a closed segment, and each segment ![]() , where
, where ![]() , hits
, hits ![]() along a singleton.
along a singleton.
Form the manifold ![]() . Its boundary consists of two copies of Y together with the disjoint union of
. Its boundary consists of two copies of Y together with the disjoint union of ![]() (they are the doubles of
(they are the doubles of ![]() ’s). The obvious vertical field
’s). The obvious vertical field ![]() on W, being restricted to X, is boundary
on W, being restricted to X, is boundary
concave. In fact, ![]() , where
, where ![]() , and
, and ![]() .
. ![]()
These examples lead to few interesting questions:
Question 4.1. Which compact manifolds admit boundary concave non-vanishing vector fields? Which compact manifolds admit boundary concave non-vanishing gradient-like fields? ![]()
Despite the “natural” flavor of these questions, we have a limited understanding of the general answers. Nevertheless, feeling a bit adventurous, let us state briefly what kind of answer one might anticipate. This anticipation is based on a better understanding of boundary concave traversing fields on 3-folds (see [5] [24] ).
We conjecture that an ![]() -dimensional X admits a traversing concave field
-dimensional X admits a traversing concave field ![]() such that
such that
![]() if (perhaps, if and only if) X has a “special trivalent” simple
if (perhaps, if and only if) X has a “special trivalent” simple ![]() -dimensional spine
-dimensional spine![]() , where
, where ![]() denotes a smooth triangulation of X (see [25] for the definitions of simple spines and for the description of their local topology). Here “special trivalent” means that each
denotes a smooth triangulation of X (see [25] for the definitions of simple spines and for the description of their local topology). Here “special trivalent” means that each ![]() -simplex from the singular set SK of K is adjacent to exactly three
-simplex from the singular set SK of K is adjacent to exactly three ![]() -simplexes from K. Moreover, the vicinity of SK in K admits an oriented branching as in [24] .
-simplexes from K. Moreover, the vicinity of SK in K admits an oriented branching as in [24] .
When the ![]() -manifold in question is specially manufactured from a closed
-manifold in question is specially manufactured from a closed ![]() -manifold by removing a number of
-manifold by removing a number of ![]() -disks, another paper from this series will provide us with a wast gallery of manifolds which admit traversing concave fields.
-disks, another paper from this series will provide us with a wast gallery of manifolds which admit traversing concave fields.
5. Morse Stratifications of the Boundary 3-Convex and 3-Concave Fields
We have seen that the boundary 2-convexity of traversing fields on X has strong implications for the topology of X (for example, see Lemmas 4.2 - 4.3, and Corollaries 4.2 - 4.4).
By itself, the boundary 3-convexity and 3-concavity of traversing fields has no topological significance for the topology of 3-folds: we have proved in Theorem 9.5 from [5] that, for every 3-fold X, any boundary generic ![]() of the gradient type can be deformed into new such field
of the gradient type can be deformed into new such field ![]() with
with![]() . However, in conjunction with certain topological constraints on
. However, in conjunction with certain topological constraints on ![]() (like being connected), the boundary 3-convexity has topological implications (see [5] , Corollary 2.3 and Corollary 2.5).
(like being connected), the boundary 3-convexity has topological implications (see [5] , Corollary 2.3 and Corollary 2.5).
These observations suggest two general questions:
Question 5.1.
・ Given a manifold X, which patterns of the stratifications ![]() are realizable by boundary generic traversing fields
are realizable by boundary generic traversing fields ![]() on X?9
on X?9
・ Given two such fields, ![]() and
and![]() , can we find a linking path
, can we find a linking path ![]() in the space
in the space ![]() that avoids certain types of singularities?10 Specifically, if for some
that avoids certain types of singularities?10 Specifically, if for some![]() ,
, ![]() , is there a linking path so that
, is there a linking path so that ![]() for all
for all![]() ?
? ![]()
Remark 5.1. The property of the field ![]() in Question 5.1 being traversing (equivalently, boundary generic and of the gradient type) is the essence of the question. For just boundary generic fields, there are no known restrictions on the patterns of
in Question 5.1 being traversing (equivalently, boundary generic and of the gradient type) is the essence of the question. For just boundary generic fields, there are no known restrictions on the patterns of![]() .
.
Let us illustrate this remark for the fields ![]() such that
such that![]() . We divide the boundary
. We divide the boundary ![]() into two complementary domains,
into two complementary domains, ![]() and
and![]() , which share a common boundary
, which share a common boundary![]() ―a closed manifold of dimension
―a closed manifold of dimension![]() . It may have several connected components. Next, we divide the manifold
. It may have several connected components. Next, we divide the manifold ![]() into two com- plementary closed manifolds
into two com- plementary closed manifolds ![]() and
and![]() .
.
We claim that it is possible to find a boundary generic field ![]() with the properties:
with the properties:![]() ,
, ![]() , and
, and![]() . The construction of such
. The construction of such ![]() is quite familiar (see the arguments in Theorem 3.2).
is quite familiar (see the arguments in Theorem 3.2).
We start with a field ![]() which is normal to
which is normal to ![]() and points outside of
and points outside of ![]() along
along ![]() and inside of
and inside of ![]() along
along![]() . We extend
. We extend ![]() to a field
to a field ![]() tangent to the boundary
tangent to the boundary ![]() so that
so that ![]() has only isolated zeros. Let
has only isolated zeros. Let ![]() be the outward normal field of
be the outward normal field of ![]() in X and
in X and ![]() a smooth function such that 0 is its regular value and
a smooth function such that 0 is its regular value and
![]()
Along![]() , form the field
, form the field ![]() and extend it to a field
and extend it to a field ![]() on X with isolated singularities in
on X with isolated singularities in![]() . By its construction,
. By its construction, ![]() has all the desired properties. Note that here we do not insist on the property
has all the desired properties. Note that here we do not insist on the property![]() .
. ![]()
In our inquiry, we are inspired by the Eliashberg surgery theory of folding maps [6] [7] . In many cases, Eliashberg’s results give criteria for realizing given patterns of![]() , provided that
, provided that![]() , thus answering Question 5.1. Let us state one such result, Theorem 5.3 from [7] .
, thus answering Question 5.1. Let us state one such result, Theorem 5.3 from [7] .
Theorem 5.1 (Eliashberg) Let![]() ,
, ![]() , be a compact connected smooth submanifold of dimension
, be a compact connected smooth submanifold of dimension![]() . Consider two disjoint closed and nonempty
. Consider two disjoint closed and nonempty ![]() -submanifolds
-submanifolds ![]() and
and ![]() of
of ![]() whose union separates
whose union separates ![]() into two complementary
into two complementary ![]() -manifolds,
-manifolds, ![]() and
and![]() . Let
. Let ![]() be the outward normal field of
be the outward normal field of ![]() in X, and denote by
in X, and denote by ![]() the degree of the Gauss map
the degree of the Gauss map![]() . Let
. Let ![]() be a linear surjection.
be a linear surjection.
Then the topological constraints
・ ![]() , when
, when ![]()
・ ![]() , when
, when ![]()
are necessary and sufficient for the existence of an orientation-preserving diffeomorphism ![]() with the following properties:
with the following properties:
・ ![]() is the fold locus of the map
is the fold locus of the map![]() ,
,
・ ![]() , being restricted to
, being restricted to![]() , is a immersion, and the image
, is a immersion, and the image ![]() has only transversal self-intersections in
has only transversal self-intersections in![]() ,
,
・ the differential ![]() takes the normal field
takes the normal field ![]() to the field inward normal to
to the field inward normal to ![]() in
in![]() ,
,
・ the differential ![]() takes the normal field
takes the normal field ![]() to the field outward normal to
to the field outward normal to ![]() in
in![]() .
. ![]()
Considering a traversing field ![]() which is tangent to the fibers of the map
which is tangent to the fibers of the map ![]() from Theorem 5.1, leads instantly to
from Theorem 5.1, leads instantly to
Corollary 5.1 Under the hypotheses and notations from Theorem 5.1, there exists a boundary generic traversing field ![]() on
on ![]() so that:
so that:
・ ![]() ,
,
・ ![]() ,
,
・ ![]() .
. ![]()
Thus, at least for smooth domains ![]() and for boundary generic traversing fields
and for boundary generic traversing fields![]() , which are both 3-convex and 3-concave, the patterns for the strata
, which are both 3-convex and 3-concave, the patterns for the strata
![]()
are indeed very flexible. However, the requirement that both ![]() and
and ![]() puts breaks on any applcation of Corollary 5.1 to boundary concave and boundary convex traversing fields on
puts breaks on any applcation of Corollary 5.1 to boundary concave and boundary convex traversing fields on![]() !
!
Example 5.1. Let us illustrate how non-trivial the conclusions of Theorem 5.1 and Corollary 5.1 are. Let![]() ,
,![]() . When
. When ![]() is odd, take any codimension one submanifold
is odd, take any codimension one submanifold ![]() such that
such that![]() ,
, ![]() , and
, and![]() . Then
. Then ![]() admits a boundary generic traversing field
admits a boundary generic traversing field ![]() such that
such that ![]() and
and![]() .
.
For instance, ![]() admits a a boundary concave traversing field
admits a a boundary concave traversing field ![]() such that
such that![]() , the orientable surface of genus 2, and
, the orientable surface of genus 2, and![]() , the 2-torus.
, the 2-torus.
When ![]() is even, take any codimension one submanifold
is even, take any codimension one submanifold ![]() such that
such that![]() ,
, ![]() , and
, and![]() . Then
. Then ![]() admits a boundary generic traversing field
admits a boundary generic traversing field ![]() such that
such that ![]() and
and![]() .
.
For example, for any collection of loops![]() ,
, ![]() ,
, ![]() , the disk
, the disk ![]() admits a boun-
admits a boun-
dary generic traversing field ![]() such that
such that ![]() and
and![]() .
. ![]()
We suspect that an important for our program generalization of Theorem 5.1 is valid and can be established by the methods as in [6] [7] .
Conjecture 5.1 Let ![]() be a compact connected smooth manifold of dimension
be a compact connected smooth manifold of dimension![]() , equipped with a traversing vector field
, equipped with a traversing vector field![]() . Let
. Let ![]() and
and ![]() be two disjoint closed and nonempty
be two disjoint closed and nonempty ![]() -submanifolds of
-submanifolds of ![]() whose union separates
whose union separates ![]() into two
into two ![]() -manifolds,
-manifolds, ![]() and
and![]() .
.
Then the topological constraints
![]() (9)
(9)
![]() (10)
(10)
are necessary and sufficient for the existence of an orientation-preserving diffeomorphism ![]() with the following properties:
with the following properties:
・ the restriction of ![]() to the image
to the image ![]() is boundary generic in the sense of Definition 2.111,
is boundary generic in the sense of Definition 2.111,
・ ![]() ,
,
・ ![]() ,
,
・ ![]() .
.
Moreover, in a given collar ![]() of
of ![]() in
in![]() , there is a
, there is a ![]() -supported diffeomorphism
-supported diffeomorphism ![]() as above which is arbitrary close in the
as above which is arbitrary close in the ![]() -topology to the identity map.
-topology to the identity map. ![]()
To prove the necessity of the topological constraints (9) and (10) is straightforward. By the Morse Formula (2) (see also Corollary 5.1), a necessary condition for the existence of a diffeomorphism ![]() with the desired properties, described in the bullets, is the constraint
with the desired properties, described in the bullets, is the constraint
![]()
Since ![]() is a homeomorphism, this equation is equivalent to
is a homeomorphism, this equation is equivalent to
![]() (11)
(11)
If![]() , then
, then
![]()
Therefore, using Formula (11), the constraint becomes![]() ―Formula (10).
―Formula (10).
When![]() , since
, since ![]() are closed odd-dimensional manifolds,
are closed odd-dimensional manifolds, ![]() , Formula (11) reduces to
, Formula (11) reduces to![]() ―Formula (9).
―Formula (9).
Therefore the topological constraints (9) and (10) imposed on the “candidates”![]() ,
, ![]() and
and ![]() and
and ![]() are necessary for the existence of the desired diffeomorphism
are necessary for the existence of the desired diffeomorphism![]() .
.
To prove the sufficiency of these conditions may require a clever application of the ![]() -principle in the spirit of [6] [7] .
-principle in the spirit of [6] [7] .
Corollary 5.2 Assuming the validity of Conjecture 5.1, any compact smooth manifold ![]() with boundary admits a boundary generic traversing field
with boundary admits a boundary generic traversing field ![]() with the property
with the property![]() .
. ![]()
Proof. By Corollary 4.1, ![]() , we can start with a traversing field
, we can start with a traversing field ![]() and apply Conjecture 5.1 to it to get the pull-back field
and apply Conjecture 5.1 to it to get the pull-back field ![]() with the desired properties.
with the desired properties.
Conjecture 5.2 Given two vector fields ![]() and
and ![]() as in Corollary 5.2, there is a 1-parameter family of traversing fields
as in Corollary 5.2, there is a 1-parameter family of traversing fields ![]() which connects
which connects ![]() to
to ![]() and such that only for finitely many instances
and such that only for finitely many instances![]() ,
,![]() . For those exceptional
. For those exceptional![]() ’s,
’s,![]() .
. ![]()
NOTES
1in the sense of Definition 2.4
![]()
2 By definition, ![]() and
and![]() .
.
3Thus ![]() is a transfer by
is a transfer by ![]() of the constant field
of the constant field![]() .
.
![]()
4Recall that![]() .
.
![]()
5as in the proof of Lemma 3.2.
![]()
6This condition is metric-independent: it does not depend on the choice of![]() .
.
![]()
7This assumption implies that![]() , provided
, provided![]() .
.
![]()
8equivalently, a non-vanishing gradient-like field.
![]()
9Theorem 5.1 and Corollary 5.1 below give just a taste of a possible answer.
10When![]() , Theorem 9.5 in [5] addresses some of these questions.
, Theorem 9.5 in [5] addresses some of these questions.
![]()
11and even traversally generic in the sense of Definition 3.2 from [8]