1. Introduction
The purpose of this paper is to calculate the binding energy of the simplest bound nucleus, the deuteron 2H, with only fundamental laws (electromagnetics with Schrödinger equation) and associated constants. Up to now, mainstream nuclear physics is unable to obtain a single nuclear binding energy by applying fundamental laws and constants. The so-called “LQCD fundamental approaches”, with “ab initio predictions of observables”, have no quantitative fundamental laws, only phenomenological models, thus qualitative.
1.1. History of the Nuclear Underlying Force
After one century of nuclear physics, “it is an open secret that the underlying force remains a puzzle” [1] . The parameters of the potential are still determined by fitting to experimental data [2] . Up to now no fundamental law of the nuclear interaction has been discovered. The electric and magnetic forces are known qualitatively since two millenaries, when the Greeks discovered the properties of amber (elektron) and magnetite (from mount Magnetos). The electromagnetic laws were discovered by Coulomb [3] and Poisson [4] two centuries ago, unified by Maxwell [5] . Born [6] noticed that “From Newton’s law, one can derive that the binding energy of two massive bodies is inversely proportional to the distance between them.” Unfortunately he believed that the neutron was an uncharged particle, thus needing “forces of a different type (...) restricted to a very short range”. The “strong force” hypothesis originated from the idea that the protons would repel one another and the nucleus should therefore fly apart. The attraction between a proton and a neutron seems still to be ignored although the discovery by Bloch [7] of the magnetic moment of the neutron showed its electric charges with no net charge.
1.2. State of the Art
The main phenomenological assumptions in nuclear physics are:
a) The forces between nucleons are almost the same according to the assumption of charge independence: NN ≈ pp ≈ nn ≈ np. The so-called “Coulomb force”, repulsive between protons, is the only recognized electromagnetic interaction in a nucleus. The attraction between a proton and a neutron as well as the magnetic moments of the nucleons is ignored.
b) The nuclides have an approximate spherical shape, as for the deuteron (see Figure 1).
c) “The Standard Model has a disturbingly large number of parameters whose numerical values are not explained; many aspects of the model seem unnatural” [8] .
d) “In contrast with the situation with atoms, the nucleus contains no massive central body which can act as a force center. This deficiency is circumvented by the bold assumption that each nucleon experiences a central attractive force” [9] . Moreover, fundamental laws cannot be obtained from magic numbers.
e) Innumerable nuclear forces have been imagined from “Strong force” to LQCD: none has fundamental laws. The “Strong force” is assumed to have a coupling constant of 1 (how come?), thus 137 times the electromagnetic interaction. In fact the fundamental laws of the nuclear interaction are unknown. The “strong force” will disappear as the phlogiston, thanks to Lavoisier, and the Aether, thanks to Einstein.
A completely different approach based on known fundamental laws is necessary.
2. Method
We shall apply, in the Schrödinger equation, the Coulomb [3] and Poisson [4] fundamental laws with the associated fundamental constants. The nucleon radius, not fundamental, is not used here.
2.1. Electromagnetic Interactions between Nucleons
Every child knows that a rubbed plastic pen attracts small neutral pieces of paper. The same attraction arises between the electric charge of the proton and a nearby “not so neutral neutron”. This attraction, able to create a deuteron, is equilibrated by the repulsion between the collinear and opposite magnetic moments of the proton and the neutron in the deuteron. The dipole and polarizability formulas being invalid in a non-uniform electric field, the exact induced dipole formula has to be used here [10] . No need of a binomial expansion, the exact dipole formula is simple and precise. The following calculations will show that the magnetic repulsion equilibrates statically the electric attraction (Figure 1), giving the binding energy of the deuteron.
2.2. Fundamental Constants
The physical constants used are: elementary electric charge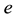 , neutron and proton magnetic moments
, neutron and proton magnetic moments 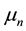 and
and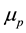 , magnetic permeability
, magnetic permeability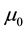 , vacuum electric permittivity
, vacuum electric permittivity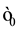 , light speed c or, equivalent nuclear fundamental constants, fine structure constant
, light speed c or, equivalent nuclear fundamental constants, fine structure constant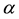 , proton mass
, proton mass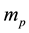 , neutron and proton Landé factors
, neutron and proton Landé factors ,
, 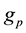 and the proton Compton radius
and the proton Compton radius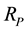 . The formulas in the appendix show the conversion from classical electromagnetic formulas to nuclear physics formulas. The usual fundamental constants of the Coulomb electromagnetic potential are replaced by the rigorously equivalent nuclear fundamental constants shown in the appendix. The formula
. The formulas in the appendix show the conversion from classical electromagnetic formulas to nuclear physics formulas. The usual fundamental constants of the Coulomb electromagnetic potential are replaced by the rigorously equivalent nuclear fundamental constants shown in the appendix. The formula 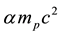 (3) is not “an arbitrary manipulation of the fine structure constant
(3) is not “an arbitrary manipulation of the fine structure constant ![]() together with the proton mass”: it shows an interesting similarity with the Hartree constant (twice the Rydberg constant),
together with the proton mass”: it shows an interesting similarity with the Hartree constant (twice the Rydberg constant), ![]() , characterizing the chemical energy.
, characterizing the chemical energy.
2.3. Electromagnetic Potential Energy
The total electromagnetic potential energy ![]() is the sum of the electrostatic interaction energy
is the sum of the electrostatic interaction energy ![]()
and the magnetostatic interaction energy ![]() between two point-like electric charges
between two point-like electric charges ![]() and
and![]() :
:
![]() (1)
(1)
The electrostatic interaction energy ![]() between particles
between particles ![]() and
and ![]() is due to the electric charges
is due to the electric charges ![]() and
and![]() . The magnetostatic interaction energy
. The magnetostatic interaction energy ![]() is due to the magnetic dipoles
is due to the magnetic dipoles ![]() and
and![]() . The general formula of the electric and magnetic interactions between two particles
. The general formula of the electric and magnetic interactions between two particles ![]() and
and ![]() is [5] [7] [11] -[13] :
is [5] [7] [11] -[13] :
![]() (2)
(2)
where ![]() (Figure 1) is the distance between the centers of the point-like particles and
(Figure 1) is the distance between the centers of the point-like particles and ![]() the corresponding internucleon vector. Formula (2) shows that the Coulomb potential is attractive or repulsive depending on the sign of the product of the electric charges and on the orientation and position of the magnetic moments. An alternative presentation of formula (2) is
the corresponding internucleon vector. Formula (2) shows that the Coulomb potential is attractive or repulsive depending on the sign of the product of the electric charges and on the orientation and position of the magnetic moments. An alternative presentation of formula (2) is
![]() (3)
(3)
where the tensor operator is [14] :
![]() (4)
(4)
![]() is the Landé factor of the
is the Landé factor of the ![]() particle.
particle. ![]() is the mass.
is the mass. ![]() and
and ![]() are the Compton radii of the electron and the proton.
are the Compton radii of the electron and the proton. ![]() is positive for magnetic repulsion and negative for magnetic attraction, depending on the relative orientation and position of the magnetic moments of the nucleons. Formulas (3) and (4) are rigorously equivalent to (2), with equivalent fundamental constants.
is positive for magnetic repulsion and negative for magnetic attraction, depending on the relative orientation and position of the magnetic moments of the nucleons. Formulas (3) and (4) are rigorously equivalent to (2), with equivalent fundamental constants.
2.4. Schrödinger Equation after Heitler [15]
The Schrödinger equation writes [9] :
![]() (5)
(5)
where ![]() is the wave function,
is the wave function, ![]() the mass,
the mass, ![]() the reduced Planck constant, E the fundamental state binding energy, V the potential energy.
the reduced Planck constant, E the fundamental state binding energy, V the potential energy. ![]() is the kinetic energy, always positive. Without kinetic energy, the Schrödinger equation reduces to the Laplace equation
is the kinetic energy, always positive. Without kinetic energy, the Schrödinger equation reduces to the Laplace equation![]() . For a spherically symmetrical potential, one has:
. For a spherically symmetrical potential, one has:
![]() (6)
(6)
Heitler [15] has calculated the binding energy of the ![]() atom with the exponential wave function,
atom with the exponential wave function, ![]() where
where ![]() is the range of the wavefunction. For
is the range of the wavefunction. For ![]() or
or ![]() at the center, the wavefunction is 1. Here we don’t need normalization of the wave function, only the binding energy:
at the center, the wavefunction is 1. Here we don’t need normalization of the wave function, only the binding energy:
![]() (7)
(7)
![]() (8)
(8)
Replacing these expressions in the Schrödinger equation we obtain:
![]() (9)
(9)
Simplifying by![]() , the kinetic energy becomes:
, the kinetic energy becomes:
![]() (10)
(10)
which is positive only for ![]() meaning that there is no kinetic energy for
meaning that there is no kinetic energy for![]() .
.
Formula (10) will be used for both hydrogen atom and heavy hydrogen nucleus. The atomic potential is electrostatic, equilibrated by the centrifugal force. The nuclear potential is electrostatic, equilibrated by the magnetic repulsion. The concept of eigenfunction is useless for the fundamental state [15] , the only state known in the deuteron.
2.5. Schrödinger Equation of the Hydrogen Atom 1H Fundamental State
From formula (10), using the attractive Coulomb potential, the fundamental state potential of the hydrogen atom is:
![]() (11)
(11)
where ![]() is the attractive potential between the proton and the electron. The first term corresponds to
is the attractive potential between the proton and the electron. The first term corresponds to
the quantized centrifugal movement, the same as in the Bohr model but obtained with the Schrödinger equation. This expression will be identically null if the constant and variable terms in 1/r are identically nullified, giving two equations:
![]() (12)
(12)
The well known formula for the hydrogen atom fundamental state energy is thus [15] :
![]() (13)
(13)
where ![]() is the fine structure constant and
is the fine structure constant and ![]() the electron mass.
the electron mass.
2.6. Schrödinger Equation of the Deuteron 2H Fundamental State
In the deuteron, the negative charge of the neutron is attracted by the proton positive charge; its positive charge is repelled farther away. The result is a net attractive electrostatic force.
The magnetic moments being opposite and collinear, the magnetic energy is repulsive (14). The nuclear electromagnetic potential ![]() is the sum of the Coulomb electric and Poisson magnetic potentials [10] :
is the sum of the Coulomb electric and Poisson magnetic potentials [10] :
![]() (14)
(14)
2a is the distance between the positive and negative electric charges of the neutron and r the distance between the centers of the nucleons. Although this potential is not really spherical we may use it because we need only the forces along the neutron-proton axis. The kinetic energy, always positive, represented by the first term of Equation (15) below, is repulsive, needing the condition![]() , as for the H atom but with the proton mass. The binding energy
, as for the H atom but with the proton mass. The binding energy ![]() being defined as the minimum of the potential energy, the Schrödinger equation of the deuteron becomes, according to (10) and (14):
being defined as the minimum of the potential energy, the Schrödinger equation of the deuteron becomes, according to (10) and (14):
![]() (15)
(15)
Numerically, the energy is given in MeV and the distances in fm:
![]() (16)
(16)
The potential energy having three variables ![]() we have to find their values to obtain the potential minimum. This equation was solved by trial and error until the horizontal inflection point attains a minimum by varying a and b as shown on Figure 2 (not to be confused with an ad hoc adjustment). The lowest horizontal inflection point (saddle point) of the thick curve is only a relative minimum of the potential energy
we have to find their values to obtain the potential minimum. This equation was solved by trial and error until the horizontal inflection point attains a minimum by varying a and b as shown on Figure 2 (not to be confused with an ad hoc adjustment). The lowest horizontal inflection point (saddle point) of the thick curve is only a relative minimum of the potential energy ![]() due to the Coulomb singularity in
due to the Coulomb singularity in ![]() (Figure 2). On the other hand, b cannot decrease towards zero, giving a repulsion, larger than the experimental binding energy, attainable only for b infinite, practically
(Figure 2). On the other hand, b cannot decrease towards zero, giving a repulsion, larger than the experimental binding energy, attainable only for b infinite, practically ![]() annihilating the wave mechanics term, representing the repulsive kinetic energy:
annihilating the wave mechanics term, representing the repulsive kinetic energy:
![]() (17)
(17)
The lowest saddle point coincides with the deuteron measured binding energy, ![]() , with a 5% precision (Figure 2). Only electromagnetic forces, without kinetic energy
, with a 5% precision (Figure 2). Only electromagnetic forces, without kinetic energy ![]() [10] are needed. The distance
[10] are needed. The distance ![]() is the distance between the centers of the nucleons, not the radius of the deuteron, around 2 fm. This approach is confirmed for 4He however with a lower precision due to the approximations used [16] .
is the distance between the centers of the nucleons, not the radius of the deuteron, around 2 fm. This approach is confirmed for 4He however with a lower precision due to the approximations used [16] .
To check the graphical result, let us ignore the magnetic repulsion. The distance between the positive charge of the proton and the negative charge of the neutron is![]() . To have equidistance of the positive charges with the negative charge, we have the condition
. To have equidistance of the positive charges with the negative charge, we have the condition ![]() thus
thus![]() . Using the graphical solution,
. Using the graphical solution, ![]() gives
gives![]() , without magnetic repulsion, not to too far from the result of the complete graphical calculation,
, without magnetic repulsion, not to too far from the result of the complete graphical calculation,![]() . The magnetic repulsive potential (in
. The magnetic repulsive potential (in![]() ) is small but its force (in
) is small but its force (in![]() ) is important for the equilibrium.
) is important for the equilibrium.
3. Nuclear and Chemical Energies
The energy needed to separate an electron from a proton is given by the Rydberg constant![]() , half the Hartree energy, according to the Bohr formula:
, half the Hartree energy, according to the Bohr formula:
![]() (18)
(18)
where ![]() is the electron mass,
is the electron mass, ![]() the fine structure constant and
the fine structure constant and ![]() the velocity of light. The energy needed to separate the neutron from the proton of a deuteron had been obtained with an analytical formula [17] :
the velocity of light. The energy needed to separate the neutron from the proton of a deuteron had been obtained with an analytical formula [17] :
![]() (19)
(19)
where![]() ,
, ![]() ,
, ![]() ,
, ![]() are respectively, the proton Compton radius, proton mass, neutron and proton Landé factors. This calculated deuteron binding energy value is 30% weaker than the experimental value,
are respectively, the proton Compton radius, proton mass, neutron and proton Landé factors. This calculated deuteron binding energy value is 30% weaker than the experimental value, ![]() due to the approximation used, neglecting the positive charge of the neutron repulsed farther away from the proton. The order of magnitude of the nuclear/chemical energy ratio may be characterized by one quarter of a million:
due to the approximation used, neglecting the positive charge of the neutron repulsed farther away from the proton. The order of magnitude of the nuclear/chemical energy ratio may be characterized by one quarter of a million:
![]() (20)
(20)
The experimental binding energies per nucleon vary from ![]() for 7H to almost
for 7H to almost ![]() for Fe, corresponding to a nuclear to chemical binding energy ratio varying from 44,000 to 662,000, coherent with the above calculated value and the usual estimation of the order of one million for the nuclear/chemical energy ratio. The binding energy per nucleon of any nuclide is given by
for Fe, corresponding to a nuclear to chemical binding energy ratio varying from 44,000 to 662,000, coherent with the above calculated value and the usual estimation of the order of one million for the nuclear/chemical energy ratio. The binding energy per nucleon of any nuclide is given by ![]() multiplied by a coefficient depending only on the electromagnetic structure of the nucleus. This is not numerology, it results from the bare application of Coulomb electric and Poisson magnetic laws in the Schrödinger equation or static electromagnetism alone [10] .
multiplied by a coefficient depending only on the electromagnetic structure of the nucleus. This is not numerology, it results from the bare application of Coulomb electric and Poisson magnetic laws in the Schrödinger equation or static electromagnetism alone [10] .
4. Results
The Schrödinger equation of the deuteron has been solved using only electric and magnetic interactions. The results obtained confirm the validity of the static approach, simplified with an analytical formula [17] or, with a better precision due to a more complete electric potential needing a graphical solution [16] . The ratio between nuclear and chemical energies is found to be 250,000 with the analytical formula [17] usually assumed to be of the order of one million. No phenomenological or empirical theory is able to obtain similar results without ad hoc fitting.
5. Discussion
In the deuteron, the magnetic moments of the proton and the neutron are opposite and collinear (not antiparallel![]() , creating an unphysical angular momentum). Their algebraic sum gives, approximately, with an error of 20%, the deuteron magnetic moment. The proton and the neutron rotate around their common axis, stabilized by the gyroscopic effect due to the nucleon spin. It is well known that the deuteron is weakly bound, still unexplained. A simple quantitative explanation is that the deuteron has only 1/2 neutron-proton bond per nucleon. The
, creating an unphysical angular momentum). Their algebraic sum gives, approximately, with an error of 20%, the deuteron magnetic moment. The proton and the neutron rotate around their common axis, stabilized by the gyroscopic effect due to the nucleon spin. It is well known that the deuteron is weakly bound, still unexplained. A simple quantitative explanation is that the deuteron has only 1/2 neutron-proton bond per nucleon. The ![]() particle, having 2 neutron-proton bonds per proton, is 4 times stronger than the deuteron [16] [18] . The magnetic repulsion is smaller in 4He than in 2H, due to the inclination of the magnetic moments at
particle, having 2 neutron-proton bonds per proton, is 4 times stronger than the deuteron [16] [18] . The magnetic repulsion is smaller in 4He than in 2H, due to the inclination of the magnetic moments at![]() . The magnetic repulsion being smaller, it increases by 50% the energy (2) thus multiplying by 6 the 4He binding energy to give
. The magnetic repulsion being smaller, it increases by 50% the energy (2) thus multiplying by 6 the 4He binding energy to give ![]() instead of
instead of![]() , not too bad (a graphical solution gives a better precision).
, not too bad (a graphical solution gives a better precision).
The electromagnetic potential has a real minimum when the positive charge of the neutron is neglected [17] . The advantage is that the binding energy is given by an analytical formula. The inconvenience is an error of 30%. When both the positive and negative charges of the neutron are taken into account, the error is less than 5% but needs a graphical solution. The other inconvenience is that the potential has no real minimum, only a horizontal inflection point due to the Coulomb singularity. The calculation of the binding energy of the deuteron, combined with the electric and magnetic interactions between the nucleons shows that the quantum mechanics
term ![]() of the Schrödinger equation has to be very small, e.g. zero or, in other words, there is no
of the Schrödinger equation has to be very small, e.g. zero or, in other words, there is no
kinetic energy in the nucleus, contradicting the mainstream belief that the nucleons orbit like the electrons in an atom. With centrifugal force, the result would be incorrect, differing from the experimental value. The centrifugal force in the atom is replaced in the nucleus by the magnetic repulsion between nucleons.
6. Conclusion
The nuclear interaction is phenomenologically called “strong force”, “LQCD” or other denominations. Unfortunately, after one century of nuclear physics, the fundamental laws of the nuclear interaction remain unknown, still needing empirical formulas fitted to experiment. The Schrödinger equation with the Coulomb and Poisson formulas alone is able to provide the nuclear binding energy of the simplest bound nucleus, the deuteron 2H and also of 4He [16] , without fitting parameters. The result obtained here is the same with or without the Schrödinger equation because the nucleons don’t move. The physical nature of the nuclear interaction is electromagnetic and static.
Appendix: Fundamental Constants Used [19]
・ Light velocity:
![]()
・ Proton-electron mass ratio:
![]()
・ Fine structure or coupling constant:
![]()
・ Proton mass:
![]()
・ Proton Compton radius:
![]()
・ Nuclear magneton:
![]()
・ Landé factors of the neutron and the proton:
Neutron: ![]()
Proton: ![]()
Magnetic moments: ![]() where i means n or p
where i means n or p
・ Relation between vacuum dielectric permittivity and magnetic permeability:
![]()
・ Electrostatic energy constant:
![]()
This fundamental constant, 4% weaker than the ![]() particle binding energy per nucleon
particle binding energy per nucleon![]() , is the nuclear equivalent of the Hartree energy.
, is the nuclear equivalent of the Hartree energy.
・ Magnetic energy constant:
![]()
.