Weierstrass’ Elliptic Function Solution to the Autonomous Limit of the String Equation of Type (2,5)* ()
1. Introduction
1.1. The String Equation of Type (2,5)
Put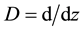 . Consider the commutator equation of ordinary differential operators
. Consider the commutator equation of ordinary differential operators
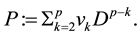
We call it the string equation (or Douglas equation) of type , which appears in the string theory or the theory of quantum gravity in 2D [1] -[9] . In the followings, we set
, which appears in the string theory or the theory of quantum gravity in 2D [1] -[9] . In the followings, we set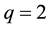 ,
,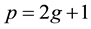 .
.
In the case where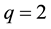 ,
, 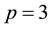 , the string equation is written as an ODE satisfied by the potential w of Sturm-Liouville operator
, the string equation is written as an ODE satisfied by the potential w of Sturm-Liouville operator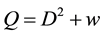 , and then, by a fractional linear transformation, it is reduced to the first Painlevé equation [10] [11]
, and then, by a fractional linear transformation, it is reduced to the first Painlevé equation [10] [11]
 , (PI)
, (PI)
which is equivalent to the Hamiltonian system:

In the case where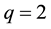 ,
,  ,
,  yields
yields
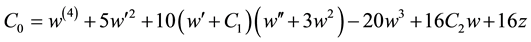 ,
,
where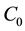 ,
, 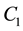 ,
, ![]() are integral constants. By the fractional linear transformation
are integral constants. By the fractional linear transformation![]() ,
, ![]() ,
,
![]()
and putting![]() , the string equation is reduced to
, the string equation is reduced to
![]() , (S)
, (S)
We also call it the string equation of type (2,5). Note that (S) coincides the 4th order equation of the first Painlevé hierarchy [12] -[15]
![]() , (2nPI)
, (2nPI)
for![]() , where
, where ![]() is an expression of a given meromorphic function w defined by
is an expression of a given meromorphic function w defined by ![]() and
and![]() .
.
1.2. Degenerated Garnier System
Equation (S) is also obtained as follows. Consider a 2D degenerated Garnier system [16] [17] :
![]() (dG9/2)
(dG9/2)
![]()
which is a 2D analogue of (PI) in the theory of isomonodromic deformations. If we fix one of the independent variables![]() , we get a Hamiltonian system with only one independent variable
, we get a Hamiltonian system with only one independent variable ![]() as follows:
as follows:
![]()
From the above system, eliminating![]() ,
, ![]() ,
, ![]() and putting
and putting![]() , we obtain (S). So, Equation (S) is 4th order analogue of (PI) in the double sences.
, we obtain (S). So, Equation (S) is 4th order analogue of (PI) in the double sences.
It is already known by Shimomura [18] that every solution to (S) is meromorphic on![]() , and that every pole of every solution is double one with its residue 0.
, and that every pole of every solution is double one with its residue 0.
1.3. Autonomous Limit of the First Painlevé Equation
The first Painlevé equation (PI) has the autonomous limit [11] . Replacing ![]() by
by ![]() with a constant
with a constant![]() , and taking limit
, and taking limit![]() , we obtain
, we obtain ![]() which is solved by the Weierstrass’ elliptic function [10] [11] . The relation between the fundamental 2-form before and after the replacement is
which is solved by the Weierstrass’ elliptic function [10] [11] . The relation between the fundamental 2-form before and after the replacement is
![]() .
.
1.4. Results
It is quite natural to think that:
Conjecture. Each equation of the first Painlevé hierarchy has the autonomous limit, and which is satisfied by the Weierstrass’ elliptic function.
For![]() , the statement is valid, i.e.
, the statement is valid, i.e.
Theorem A. Replacing ![]() by
by![]() , or replacing
, or replacing ![]() by
by ![]() with a constant
with a constant![]() , and taking limit
, and taking limit![]() , we obtain the autonomous limit of the 4th order equation of the first Painlevé hierarchy (S). Moreover, the relation between the fundamental 2-form before and after the replacement is
, we obtain the autonomous limit of the 4th order equation of the first Painlevé hierarchy (S). Moreover, the relation between the fundamental 2-form before and after the replacement is
![]() .
.
It is easy to show the above. The autonomous limit is given by
![]() , (A)
, (A)
Theorem B. The autonomous limit Equation (A) has a solution concretely described by the Weierstrass’ elliptic function as
![]() ,
,
where![]() .
.
Remark. Modulus of the elliptic function is determined by the constants a and b. g2 and g3 in the elliptic function theory are as follows:
![]() .
.
The next section is devoted to give the proof of Theorem B.
2. Proof of Theorem B
Put![]() , i.e.
, i.e.
![]() . (1)
. (1)
Multiplying both sides of ![]() by
by![]() , and integrating it, we obtain a first integral of (A)
, and integrating it, we obtain a first integral of (A)
![]() (2)
(2)
In order to find the elliptic function solution, let w satisfy the relation:
![]() . (3)
. (3)
Substituting (3), ![]() and
and ![]() into (2), we have
into (2), we have
![]()
So, if we take
![]()
then solutions of (3) satisfy (2). Now, in order to reduce ![]() to
to![]() , we use the scale transformation
, we use the scale transformation![]() ,
,![]() . Immediately we obtain
. Immediately we obtain![]() , and also
, and also![]() ,
,![]() .
. ![]()
NOTES
*Dedicated to Professor Masafumi Yoshino on the occasion of his 60th birthday.