Some Properties of Analytic Functions with the Fixed Second Coefficients ()
1. Introduction
Let  be the set of functions
be the set of functions  that are analytic in
that are analytic in  and normalized by
and normalized by

And let  be the class of all functions
be the class of all functions  that are analytic and have positive real part in
that are analytic and have positive real part in  with
with .
.
Nunokawa investigated some properties of analytic functions which are not Caratheodory, that is, which are not in . Furthermore, he has found the order of strongly starlikeness of strongly convex functions in
. Furthermore, he has found the order of strongly starlikeness of strongly convex functions in  (see: [1] [2] ).
(see: [1] [2] ).
Now, for a fixed , let
, let  consist of functions
consist of functions  of the form
of the form
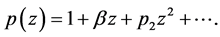
And let 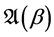 consist of analytic functions
consist of analytic functions  of the form
of the form

where the second coefficient  is fixed constant.
is fixed constant.
In [3] [4] , Ali et al. have extended the theory of differential subordination developed by Miller and Mocanu [5] , to the functions with fixed second coefficients. And Lee et al. [6] and Nagpal et al. [7] have applied the results, to obtain several extensions of properties for univalent functions with fixed second coefficients.
In this paper, we investigate some argument properties for analytic functions  with fixed second coefficients and positive real part. And we apply our results to the normalized univalent functions with fixed second coefficients.
with fixed second coefficients and positive real part. And we apply our results to the normalized univalent functions with fixed second coefficients.
We need the following Lemma for functions with fixed initial coefficient.
Lemma 1 [3] Let 
 and
and  be continuous in
be continuous in , analytic in
, analytic in  with
with  and
and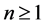 . If
. If

then
 and
and 
where
 (1)
(1)
Here, we note that the inequality (1) implies that

since .
.
2. Lemmas
Lemma 2 Let  be analytic in
be analytic in  and
and  in
in . Suppose that there exists a point
. Suppose that there exists a point  such that
such that
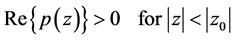
and
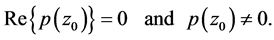
Then
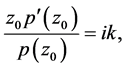
where  and
and .
.
Proof. Let us put
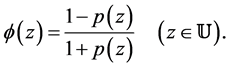
Then ,
,  for
for  and
and . And we note that
. And we note that

By Lemma 1, we have
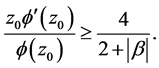
Hence
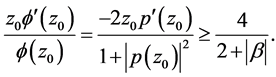
And this inequality implies  is a negative real number which satisfies
is a negative real number which satisfies

Now, we put . For the case
. For the case ,
,
 (2)
(2)
For the case ,
,
 (3)
(3)
Hence, by (2) and (3),
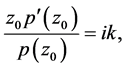
where  and
and
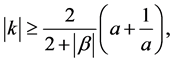
Hence the proof of Lemma 2 is completed.
Lemma 3 Let  be analytic in
be analytic in  and
and  in
in . Suppose that there exists a
. Suppose that there exists a  such that
such that

and
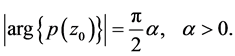
Then
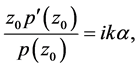
where
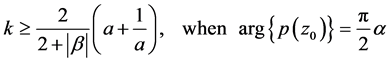
and

with

Proof. Let us put

Then
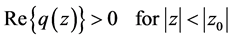
and
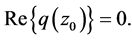
Let us put
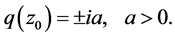
Applying Lemma 2, we get
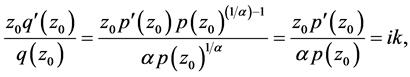
where  with
with
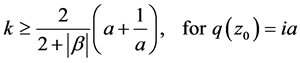
and
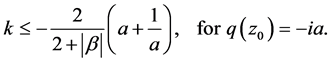
3. Argument Estimates for Functions with Fixed Second Coefficient
Theorem 4 Let 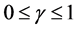 and
and  satisfy
satisfy

Then

where
 (4)
(4)
Proof. Suppose that there exists a point  such that
such that

and
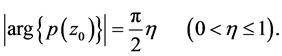
By Lemma 3, we can obtain that
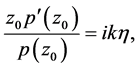
where
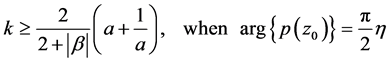
and
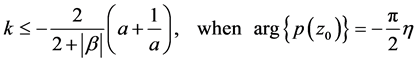
with . For the case
. For the case ,
,
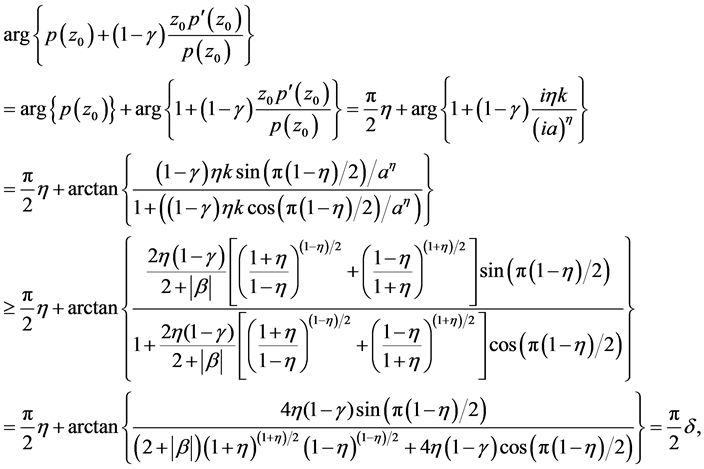
which is a contradiction to the assumption. For the case , using the same method, we can obtain a contradiction to the assumption.
, using the same method, we can obtain a contradiction to the assumption.
Remark 5 If , then Theorem 4 reduces the result in [[8] , Theorem 3].
, then Theorem 4 reduces the result in [[8] , Theorem 3].
Theorem 6 Let  and
and  satisfy
satisfy
 (5)
(5)
Then
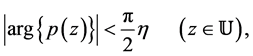
where
 (6)
(6)
Proof. If there exists a point  such that
such that
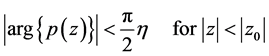
and
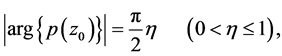
then Lemma 3 gives us that

If , then we have
, then we have . Therefore, we see that
. Therefore, we see that

with
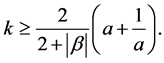
Hence
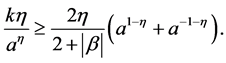
Now, we define a function  by
by
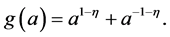
Then
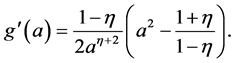
Hence  takes the minimum value at
takes the minimum value at . Therefore,
. Therefore,

Thus we have
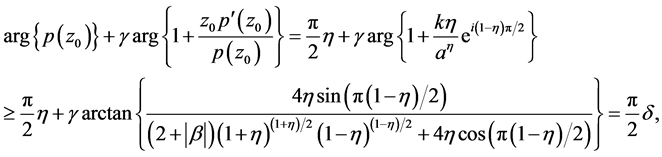
which contradicts the condition (5). And if , applying the same method we have
, applying the same method we have
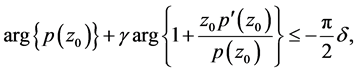
which contradicts the condition (5). And this completes the proof of the Theorem 6.
Remark 7 If , then Theorem 6 reduces the result in [[8] , Theorem 1].
, then Theorem 6 reduces the result in [[8] , Theorem 1].
Theorem 8 Let  and
and
 (7)
(7)
for some ,
, 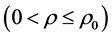
 , where
, where 
 is given by
is given by

Then

Proof. Suppose that there exists a  such that
such that

and
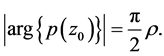
By Lemma 3, we can obtain that
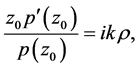
where  with
with

and

For the case ,
,
 (8)
(8)
since
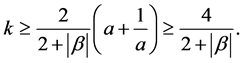
Now, we define

Then
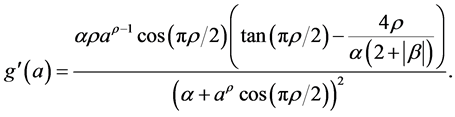
Define
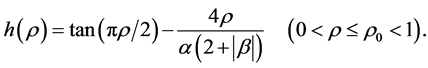
Then  and
and . Furthermore,
. Furthermore,

for all . Hence
. Hence  for all
for all . And
. And
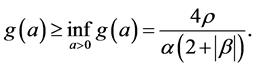
By (8), we have
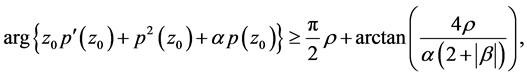
which is a contradiction to the hypothesis. For the case 
 , using the same method, we can obtain a contradiction to the assumption.
, using the same method, we can obtain a contradiction to the assumption.
Remark 9 If , then Theorem 8 reduces the result in [[9] , Theorem 2.1].
, then Theorem 8 reduces the result in [[9] , Theorem 2.1].
4. Corollaries
For a function ,
,  is called strongly starlike of order
is called strongly starlike of order ,
,  , if
, if
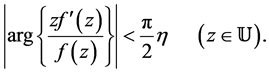
And  is called strongly convex of order
is called strongly convex of order ,
,  , if
, if
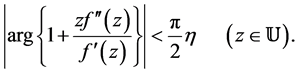
Using these definitions and Theorem in Section 3, we can obtain the following corollaries.
Corollary 10 Let  and
and
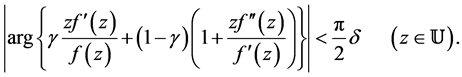
Then  is strongly starlike of order
is strongly starlike of order , where
, where  is given by (4).
is given by (4).
Putting  in Corollary 1, we can obtain the following Corollary.
in Corollary 1, we can obtain the following Corollary.
Corollary 11 Let  be a strongly convex function of order
be a strongly convex function of order . Then
. Then  is a strongly starlike function of order
is a strongly starlike function of order , where
, where  is given by
is given by

Corollary 12 Let  and
and  satisfy
satisfy
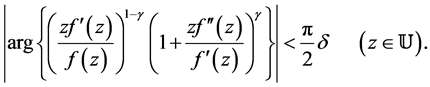
Then  is strongly starlike of order
is strongly starlike of order , where
, where  is given by (6).
is given by (6).
Corollary 13 Let  and
and

where  is given by (7). Then
is given by (7). Then  is strongly starlike of order
is strongly starlike of order .
.