On the Generalization of Hilbert’s 17th Problem and Pythagorean Fields ()
1. Introduction
In the latter half of the twentieth century, a consideration for the generalization of pythagorean fields has been made by many researchers, e.g., Elman and Lam [1], Becker [2], Koziol, Szymiczek, and Yucas [3-6], Kijima and Nishi [7], and so on.
Throughout the paper, let 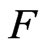 be a field of characteristic different from 2 and
be a field of characteristic different from 2 and  be the multiplicative group of
be the multiplicative group of . A field
. A field  is said to be pythagorean if
is said to be pythagorean if . For a quadratic form
. For a quadratic form  over
over , we put
, we put  and
and . Witt [8] defined a round quadratic form
. Witt [8] defined a round quadratic form  as
as . Recall that Pfister forms are round ([8], Satz 4. (c)).
. Recall that Pfister forms are round ([8], Satz 4. (c)).
The class of fields with the following property  has been proposed by Elman and Lam [1]:
has been proposed by Elman and Lam [1]:
 : Any torsion n-fold Pfister form over F is hyperbolic.
: Any torsion n-fold Pfister form over F is hyperbolic.
Furthermore, they made a hypothesis that if a field  satisfies the property
satisfies the property , then the ideal
, then the ideal  is torsionfree, where
is torsionfree, where  is the ideal of even dimensional forms in the Witt ring
is the ideal of even dimensional forms in the Witt ring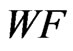 . Szymiczek [5] replaced this hypothesis with a problem of rigid elements that if
. Szymiczek [5] replaced this hypothesis with a problem of rigid elements that if , then
, then , and had studied this problem for amenable fields, linked fields, abstract Witt rings of elementary type, and so on. When a field
, and had studied this problem for amenable fields, linked fields, abstract Witt rings of elementary type, and so on. When a field  satisfies the property
satisfies the property , it is clear that
, it is clear that  also satisfies the property
also satisfies the property .
.
We denote by  the set of n-fold Pfister forms over
the set of n-fold Pfister forms over  and by
and by  the nth radical of
the nth radical of , which is given by
, which is given by . This radical defined by Yucas [6] shows a generalization of Kaplansky’s radical
. This radical defined by Yucas [6] shows a generalization of Kaplansky’s radical  [9].
[9].
Later, Koziol [3] has proposed the class of npythagorean fields with the following property as every n-fold Pfister form represents all sums of squares over , that is,
, that is, .
.
Pythagorean fields are  -pythagorean and the class of 1-pythagorean fields is the same as the class of quasipythagorean fields defined by Kijima and Nishi [7]. In fact, the class of n-pythagorean fields is the same as the class of fields which satisfy the property
-pythagorean and the class of 1-pythagorean fields is the same as the class of quasipythagorean fields defined by Kijima and Nishi [7]. In fact, the class of n-pythagorean fields is the same as the class of fields which satisfy the property  ([3], Proposition 2.3).
([3], Proposition 2.3).
On the other hand, a generalization of Hilbert’s 17th Problem has been accomplished by Artin [10]. Later, an interpretation of this generalization has been made by Pfister [11], who has proposed the class of  -fields with the following property as for any
-fields with the following property as for any ,
,  holds, where
holds, where
 .
.
Furthermore, he showed implicitly in ([11], chapter 6, Theorem 3.5) that if a field  is a
is a  -field, then
-field, then  is n-pythagorean.
is n-pythagorean.
Unexplained notation and terminology refer to [12,13].
2. Preorderings and Round Quadratic Forms
Pfister [11] has derived upper bounds for the number of squares on Hilbert’s 17th Problem. Hence, the following can be shown by results of Artin [10] and Pfister ([11], chapter 6, Corollary 3.4).
Theorem 1. Let  be the rational function field in n variables over a real closed field R and
be the rational function field in n variables over a real closed field R and  be an element of
be an element of . Then the following statements are equivalent:
. Then the following statements are equivalent:
1)  for all
for all  where
where  is defined.
is defined.
2)  holds.
holds.
3)  is a totally positive element.
is a totally positive element.
We shall prove some results by use of the notions of preorderings (Serre [14]) and round quadratic forms. By Proposition 2.3 in [3] and Lemma 3.1 in [15], the following can be shown.
Theorem 2. (([16], Proposition 1), ([17], Proposition 2.1)). For a field F, the following statements are equivalent:
1) F is n-pythagorean.
2)  holds for all
holds for all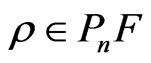 .
.
In particular, if F is formally real, these statements are further equivalent to the condition.
3) The nth radical  is a preordering.
is a preordering.
If a field  is n-pythagorean, then
is n-pythagorean, then  . Thus, the following can be obtained.
. Thus, the following can be obtained.
Corollary 3. (cf. [13], Corollary 11.4.11). For any formally real n-pythagorean field F, every totally positive element of F is a sum of  squares.
squares.
Remark 4. Corollary 3 shows a generalization of Hilbert’s 17th Problem. The notion of preordering and nth radical play an important role for this Problem. A typical example of n-pythagorean field is a field of transcendence degree n over a real closed field. Many examples of n-pythagorean fields are known. For example, n-Hilbert fields are so in [4]. Also, Kijima [18] has constructed many such examples by use of some results of Kula [19].
Next, we shall discuss about the generalization of pythagorean fields. The following result is well-known.
Theorem 5. (cf. [8], Satz 3. (g)). Let  be a field and l
be a field and l  be an odd integer. Then the following statements are equivalent:
be an odd integer. Then the following statements are equivalent:
1) The form  is round.
is round.
2)  is pythagorean.
is pythagorean.
In particular, if the form  is anisotropic, then
is anisotropic, then  is a formally real field.
is a formally real field.
Proposition 6. ([16, Proposition 3]). Let  be an n-fold Pfister form over F and l
be an n-fold Pfister form over F and l  be an odd integer. If
be an odd integer. If  is a round quadratic form, then
is a round quadratic form, then  holds.
holds.
Proof. For any , it is sufficient to show that
, it is sufficient to show that . The round form
. The round form  means that
means that . Since
. Since  is an odd integer, we put
is an odd integer, we put  for some integer
for some integer . Hence it follows that
. Hence it follows that . On the other hand, since
. On the other hand, since  is a Pfister form,
is a Pfister form,  holds and then
holds and then . Thus
. Thus  follows from Witt’s Cancellation Theorem. This implies that
follows from Witt’s Cancellation Theorem. This implies that .
.
Corollary 7. ([16, Proposition 3]). If there exist an integer 
 and an odd integer
and an odd integer 
 such that the form
such that the form  is anisotropic round, then F is formally real.
is anisotropic round, then F is formally real.
Proof. Since the form  is round, it follows from Proposition 6 that
is round, it follows from Proposition 6 that . If a field
. If a field  is non-real, then
is non-real, then . This contradicts the assumption that
. This contradicts the assumption that  is anisotropic.
is anisotropic.
As a characterization of an n-pythagorean property, the following generalization of pythagorean fields can be presented.
Theorem 8. ([16], Proposition 3). For a field F, the following statements are equivalent:
1) F is n-pythagorean.
2) For any , there exists an odd integer
, there exists an odd integer  such that
such that  is a round quadratic form.
is a round quadratic form.
Proof. (1) => (2): If a field  is n-pythagorean, then
is n-pythagorean, then  is a round quadratic form for any
is a round quadratic form for any , any positive integer
, any positive integer  and any
and any .
.
(2) => (1): This follows from Theorem 2 and Proposition 6.
Theorem 9. The n-pythagorean field is the generalization of pythagorean field and the Pythagoras number of this field is at most .
.
Proof. If a field  is m-pythagorean, then
is m-pythagorean, then  is (m + 1)-pythagorean. Thus, it follows from Theorem 5 and Theorem 8.
is (m + 1)-pythagorean. Thus, it follows from Theorem 5 and Theorem 8.
Finally, the main result of this paper has been established as follows.
Theorem 10. The generalization of pythagorean fields coincides with the generalization of Hilbert’s 17th Problem.
Proof. If a field  is non-real, then
is non-real, then  has no ordering and moreover
has no ordering and moreover  holds. Therefore, Hilbert’s 17th Problem results in a problem that if a field
holds. Therefore, Hilbert’s 17th Problem results in a problem that if a field  is non-real, then does an equality
is non-real, then does an equality  hold? Thus, the required result can be established by use of Corollary 3 and Theorem 9.
hold? Thus, the required result can be established by use of Corollary 3 and Theorem 9.
Incidentally, the notion of round quadratic forms is connected with the torsion-freeness of the ideal . We shall extend Proposition 2.3 in [7] to an npythagorean field.
. We shall extend Proposition 2.3 in [7] to an npythagorean field.
Proposition 11. ([17, Proposition 3.1]). Let  be an integer
be an integer . If F is an n-pythagorean field, then the following statements hold.
. If F is an n-pythagorean field, then the following statements hold.
1) , where
, where  is the maximal torsion subgroup of
is the maximal torsion subgroup of .
.
2)  is torsion-free.
is torsion-free.
Proof. 1) For any element  of
of , there exists an element
, there exists an element  of
of  such that
such that . By ([20], Satz 22), we can find
. By ([20], Satz 22), we can find  and
and 
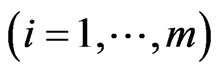 such that
such that
 in
in . Because of
. Because of
 , the Pfister form
, the Pfister form  is universal and round. Hence
is universal and round. Hence  and
and  in
in .
.
2) For any element  of
of , it is sufficient to show that p = 0. Now there exists an element
, it is sufficient to show that p = 0. Now there exists an element  of
of  such that
such that . Since
. Since , it follows from 1) that
, it follows from 1) that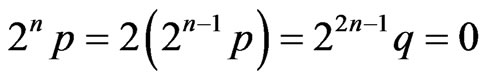 . Therefore
. Therefore  and then
and then  for some element
for some element  from 1). Since
from 1). Since  is an element of
is an element of , it follows from ([13], Hauptsatz 10.5.1) that p = 0.
, it follows from ([13], Hauptsatz 10.5.1) that p = 0.
Remark 12. In case of 1-pythagorean fields, statements 1) and 2) of Proposition 11 are equivalent (see Remark 2.4 in [7]). As a characterization of 1-pythagorean fields, Corollary 4.4 of Krawczyk [21] is beautiful and can be extended to  -pythagorean fields. This will be given in the forthcoming paper [22].
-pythagorean fields. This will be given in the forthcoming paper [22].
3. Concluding Remark
Becker [2] has used the terminology of n-pythagorean fields  as
as . Therefore, for the field with the property
. Therefore, for the field with the property  defined by Elman and Lam [1], the following name shall be recommended as Hilbert-Pythagoras field of level n.
defined by Elman and Lam [1], the following name shall be recommended as Hilbert-Pythagoras field of level n.
4. Acknowledgements
The author would like to express his deep appreciation to the late Professor M. Nishi for leading to the quadratic form theory. Also, he is very grateful to Professor S. Kageyama and the late Dr. T. Iwakami for their valuable advices.