General Boundary Value Problems for Nonlinear Uniformly Elliptic Equations in Multiply Connected Infinite Domains

Guochun Wen; Yanhui Zhang; Dechang Chen

doi:10.4236/ijmnta.2013.23024

Home > Journals > Engineering | Physics & Mathematics > IJMNTA

International Journal of Modern Nonlinea... > Vol.2 No.3, September 2013

General Boundary Value Problems for Nonlinear Uniformly Elliptic Equations in Multiply Connected Infinite Domains ()

Guochun Wen, Yanhui Zhang, Dechang Chen

Mathematic Department, Beijing Technology and Business University, Beijing, China.
School of Mathematical Sciences, Peking University, Beijing, China.
Uniformed Services University of the Health Sciences, Bethesda, USA.

DOI: 10.4236/ijmnta.2013.23024 PDF HTML 2,570 Downloads 4,250 Views

Abstract

This article discusses the general boundary value problem for the nonlinear uniformly elliptic equation of second order in D (0.1) and the boundary condition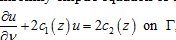,(0.2) in a multiply connected infinite domain D with the boundary T. The above boundary value problem is called Problem G. Problem G extends the work [8] in which the equation (0.1) includes a nonlinear lower term and the boundary condition (0.2) is more general. If the complex equation (0.1) and the boundary condition (0.2) meet certain assumptions, some solvability results for Problem G can be obtained. By using reduction to absurdity, we first discuss a priori estimates of solutions and solvability for a modified problem. Then we present results on solvability of Problem G.

Keywords

Boundary Value Problems; Nonlinear Elliptic Equations; Multiply Connected Infinite Domains

Share and Cite:

G. Wen, Y. Zhang and D. Chen, "General Boundary Value Problems for Nonlinear Uniformly Elliptic Equations in Multiply Connected Infinite Domains," International Journal of Modern Nonlinear Theory and Application, Vol. 2 No. 3, 2013, pp. 170-175. doi: 10.4236/ijmnta.2013.23024.

Conflicts of Interest

The authors declare no conflicts of interest.

References

[1]	I. N. Vekua, “Generalized Analytic Functions,” Pergamon, Oxford, 1962.
[2]	G. C. Wen, “Linear and Nonlinear Elliptic Complex Equations,” Shanghai Scientific and Technical Publishers, Shanghai, 1986. (in Chinese)
[3]	G. C. Wen, “Conformal Mappings and Boundary Value Problems,” American Mathematical Society, Providence, 1992.
[4]	G. C. Wen, “Approximate Methods and Numerical Analysis for Elliptic Complex Equations,” Gordon and Breach, Amsterdam, 1999.
[5]	G. C. Wen, D. C. Chen and Z. L. Xu, “Nonlinear Complex Analysis and its Applications, Mathematics Monograph Series 12,” Science Press, Beijing, 2008.
[6]	G. C. Wen, “Recent Progress in Theory and Applications of Modern Complex Analysis,” Science Press, Beijing, 2010.
[7]	G. C. Wen and C. C. Yang, “On General Boundary Value Problems for Nonlinear Elliptic Equations of Second Order in a Multiply Connected Domain,” Acta Applicandae Mathematicae, Vol. 43 No. 2, 1996, pp. 169-189. doi:10.1007/BF00047923
[8]	G. C. Wen, “Irregular Oblique Derivative Problems for Second Order Nonlinear Elliptic Equations on Infinite Domains,” Electronic Journal of Differential Equations, Vol. 2012, No. 142, 2012, pp. 1-8.