Pulse Soliton Solutions of the Modified KdV and Born-Infeld Equations ()
1. Introduction
Dynamics of physical phenomena are more often described analytically by nonlinear partial differential equations (NPDEs). These equations are varied and are met in various branches of the Physics, notably in Classical Mechanics, Quantum Mechanics, Fluid Mechanics, Electrodynamics, and so on. These NPDEs are not always easy to solve. Knowing that all analytic solutions of a NPDE always bring supplementary information, many researchers develop every day the means to solve these NPDEs or to improve some existing solutions. It is in this optics that several methods and techniques facilitating the resolution of NPDEs have been proposed [1-3]. From all these equations, those which contain the scattering terms and nonlinear terms create a particular interest, because they generally admit some soliton solutions; the soliton being a futuristic concept which imposes itself progressively in the world of Physics and especially as alternative in the modern telecommunications. This propensity is translated besides by all recent works that have been published [4-7].
Among these numerous equations, two have drawn our attention in this work. It is precisely the equation of Born-Infeld developed by Born and Infeld in the years 1930 and admits numerous applications in physics [8-15]. The second is the equation of KdV. This equation which is the first that describes the dynamics of the navy waves has been also the object of several investigations and modifications [16-22].
Abdul-Majid Wazwaz in one of his recent works demonstrated that the modified KdV equation admitted several types of solutions notably the solitons, the peakons and the cuspons [23]. In this work, we follow the same logic to look for all solutions of the shape Sechn- of the modified KdV equation. We are also going to determine all solutions Sechn- of the equation of Born-Infeld. The problem we want to solve has been motivated in the following manner: We know that the equation of KdV under its initial shape admits a solution in Sechn- (for ) but we don’t know what happens precisely when
) but we don’t know what happens precisely when . Thus, we at first look for the equation of KdV under its initial shape then admit a solution of shape Sechnwith
. Thus, we at first look for the equation of KdV under its initial shape then admit a solution of shape Sechnwith . But it is not the first objective of this work, we want to test the efficiency of the method rightly by using on a simplest case. The goal of work is to look for all pulse solutions of the shape Sechn- in the modified KdV and Born-Infeld equations by the Bogning-Djeumen Tchaho-Kofané method (BDKm) [24-28].
. But it is not the first objective of this work, we want to test the efficiency of the method rightly by using on a simplest case. The goal of work is to look for all pulse solutions of the shape Sechn- in the modified KdV and Born-Infeld equations by the Bogning-Djeumen Tchaho-Kofané method (BDKm) [24-28].
This work is organized as follows: in Section 2, we verify if the solution in Sechn- of the initial KdV equation is only obtained for . Section 3 is devoted in research of all solutions in Sechn- of the modified KdV equation. The same analysis is made in Section 4 for equation of Born-Infeld. Finally, in Section 5, we conclude our work.
. Section 3 is devoted in research of all solutions in Sechn- of the modified KdV equation. The same analysis is made in Section 4 for equation of Born-Infeld. Finally, in Section 5, we conclude our work.
2. Sechn- Soliton Solution of the Initial KdV Equation
The KdV equation in its initial form is given by
 , (1)
, (1)
where  represents the amplitude of navy wave,
represents the amplitude of navy wave, 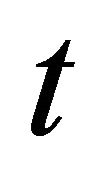 is the time and
is the time and  the spatial variable. The problem here is to construct the solution of Equation (1) in the form
the spatial variable. The problem here is to construct the solution of Equation (1) in the form
 , (2)
, (2)
where  is a constant and
is a constant and  a real number which is different of two. When we introduce the Equation (2) in the Equation (1) we obtain an equation of which the use of the different transformations linked to BDKm [24-28] permits to write it under the form
a real number which is different of two. When we introduce the Equation (2) in the Equation (1) we obtain an equation of which the use of the different transformations linked to BDKm [24-28] permits to write it under the form
 (3)
(3)
called equation of ranges where ,
,  ,
,  ,
,  and
and  are functions of the coefficients
are functions of the coefficients 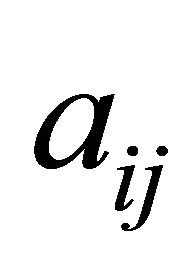 to determine and
to determine and  are positive whole integers.
are positive whole integers.
These transformations concern the terms obtain when we introduce Equation (2) in Equation (1) and they are given by the following equations
 , (4)
, (4)
 , (5)
, (5)
 (6)
(6)
 (7)
(7)
 , (8)
, (8)
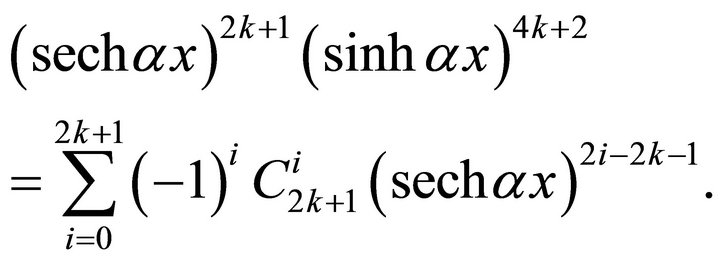 (9)
(9)
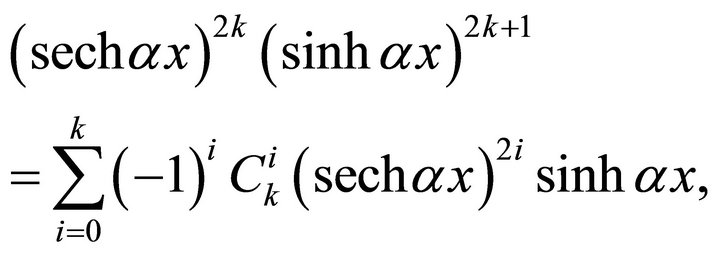 (10)
(10)
 (11)
(11)
 (12)
(12)
 (13)
(13)
where ,
, and
and  are fixed integers such that
are fixed integers such that . Thus, substituting Equation (2) in Equation (1), we obtain with the help of the preceding transformations the general equation
. Thus, substituting Equation (2) in Equation (1), we obtain with the help of the preceding transformations the general equation
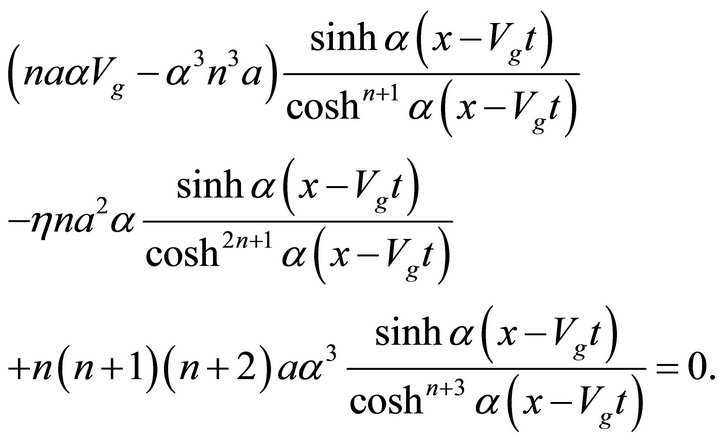 (14)
(14)
After haven gotten the Equation (13), the principle of analysis is as follows: before passing to the identification of coefficients of the terms in
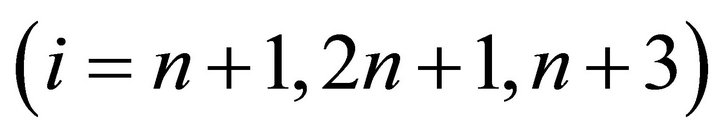
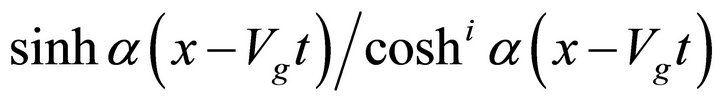
of Equation (14), we verify if there exist some values of  for which some terms of the Equation (14) have the same factor
for which some terms of the Equation (14) have the same factor ? So for the Equation (14), the factors
? So for the Equation (14), the factors
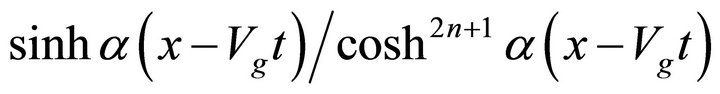
and

are identical for  (it is sufficient to solve the equation
(it is sufficient to solve the equation ). We also notice that the factors
). We also notice that the factors

and
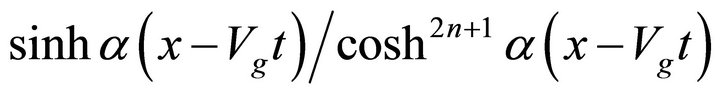
are identical for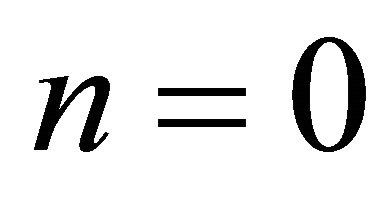 . But for
. But for , the Equation (14) leads to a trivial solution of the type
, the Equation (14) leads to a trivial solution of the type  (constant) that is not interesting. For n = 2, Equation (14) is written as
(constant) that is not interesting. For n = 2, Equation (14) is written as
 (15)
(15)
From Equation (15), we obtain the following ranges of equations
 , (16)
, (16)
and
 . (17)
. (17)
The resolution of Equation (16) and Equation (17)
permits to have  and
and . While reporting these values obtained in the expression (2), we at first find a solution considered like particular solution of the Equation (1) and given by
. While reporting these values obtained in the expression (2), we at first find a solution considered like particular solution of the Equation (1) and given by
 , (18)
, (18)
with 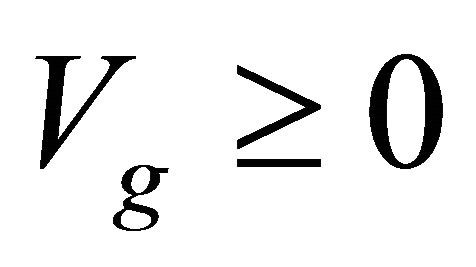 and
and . The particular cases
. The particular cases  and
and  being studied, we now look at the case where
being studied, we now look at the case where  and
and . So for
. So for  and
and , the Equation (14) doesn’t present more terms that can merge themselves. At this level, the only acceptable solution is the trivial solution
, the Equation (14) doesn’t present more terms that can merge themselves. At this level, the only acceptable solution is the trivial solution  which doesn’t have importance. In conclusion, the equation of KdV as considered above admits non trivial solutions only for
which doesn’t have importance. In conclusion, the equation of KdV as considered above admits non trivial solutions only for  and Equation (18) also gives the general solution of the KdV equation considered under its initial shape given by Equation (1).
and Equation (18) also gives the general solution of the KdV equation considered under its initial shape given by Equation (1).
3. Sechn- Soliton Solution of the Modified KdV Equation
The modified KdV equation that we are going to use here is in the form [23]
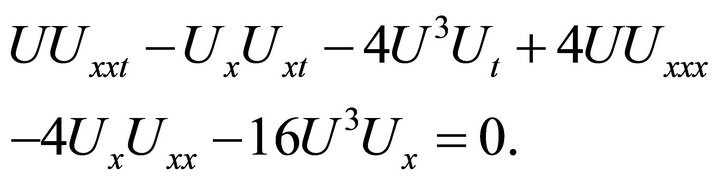 (19)
(19)
We look for the solutions of Equation (19) under the shape
 , (20)
, (20)
where  and
and  are constants to be determined,
are constants to be determined,  a known constant and
a known constant and  a real number to determine. Thus, taking into account Equation (20) into Equation (19) gives with the help of transformations (4), (5),···, (13) the following ranges equation
a real number to determine. Thus, taking into account Equation (20) into Equation (19) gives with the help of transformations (4), (5),···, (13) the following ranges equation
 (21)
(21)
The two terms of the Equation (21) merge themselves for  (it is sufficient to solve the equation
(it is sufficient to solve the equation ). So from the Equation (21), we obtain
). So from the Equation (21), we obtain
 , (22)
, (22)
with . Equation (22) can also be written as
. Equation (22) can also be written as
 (23)
(23)
with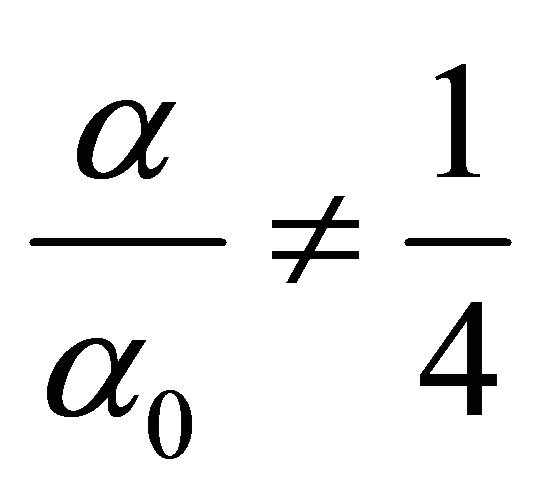 . By setting
. By setting 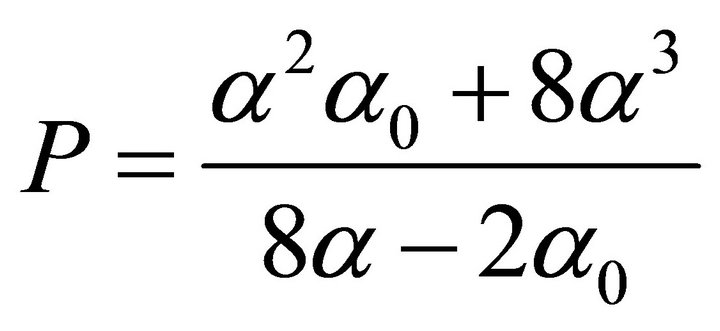 and
and , Equation (23) becomes
, Equation (23) becomes
 . (24)
. (24)
By using the Cardano’s method, we set  leads to the relations
leads to the relations
 , (25)
, (25)
and
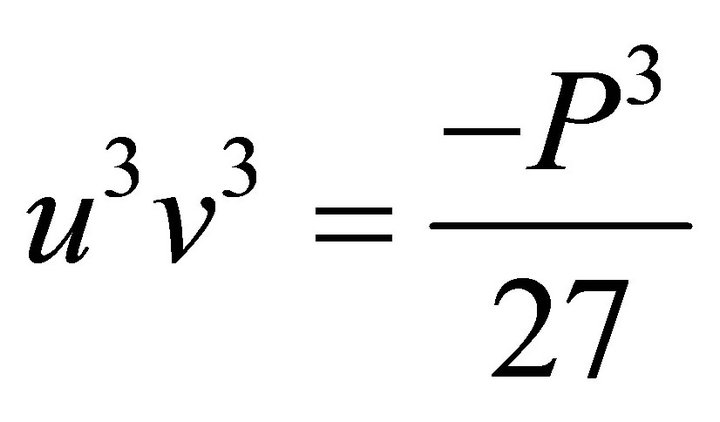
 . (26)
. (26)
The resolution of the coupled system (25) and (26) gives
 . (27)
. (27)
Therefore the solution obtained for  is given by
is given by
 (28)
(28)
For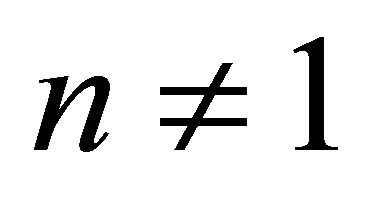 , Equation (21) leads to the following relations:
, Equation (21) leads to the following relations:
Term in ,
,
 . (29)
. (29)
Term in ,
,
 . (30)
. (30)
Then for  and
and , the solution of the modified KdV equation is given by
, the solution of the modified KdV equation is given by
 . (31)
. (31)
In the following section, we are going to make an analogous survey for the equation of Born-Infeld.
4. Sechn- Solution of the Born-Infeld’s Equation
The Born-Infeld’s equation that we want to analyze in this section is given by
 . (32)
. (32)
Like in the preceeding section, we look for the solution of Equation (31) in the form of Equation (20). With the help of the transformations (4), (5),···, (13), we obtain
 (33)
(33)
As in the previous cases, we first look for the values of  for which the terms which constituted Equation (33) merge themselves. Thus, the terms in
for which the terms which constituted Equation (33) merge themselves. Thus, the terms in
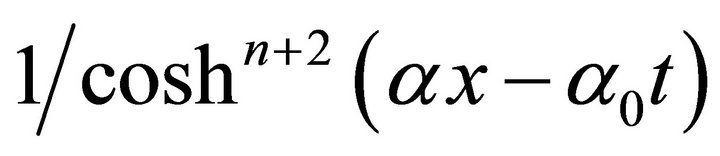 and
and  merge for
merge for  as well as the terms in
as well as the terms in  and
and
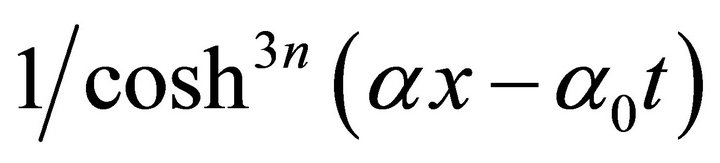 . The terms in
. The terms in 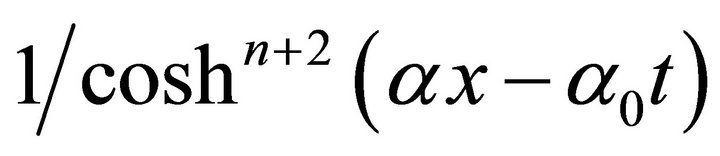
and  as well as the terms in
as well as the terms in
 and
and  merge for
merge for . The terms in
. The terms in  and
and
 merge for
merge for . The terms in
. The terms in
 and
and  merge for
merge for . Then, for
. Then, for , the Equation (33) contains the terms which merge. In the continuation we are going to study in detail the Equation (33) when
, the Equation (33) contains the terms which merge. In the continuation we are going to study in detail the Equation (33) when  is equal to these found values.
is equal to these found values.
• For , the resolution of Equation (33) gives
, the resolution of Equation (33) gives  and
and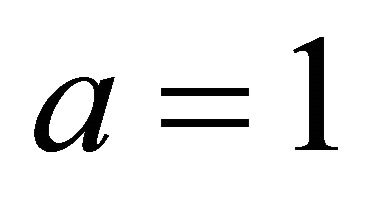 . The solution of Equation (32) in this case is given by
. The solution of Equation (32) in this case is given by
 (34)
(34)
• For , the resolution of Equation (33) gives
, the resolution of Equation (33) gives  and
and . The solution of Equation (32) in this case is given by
. The solution of Equation (32) in this case is given by
 (35)
(35)
• For , we obtain
, we obtain 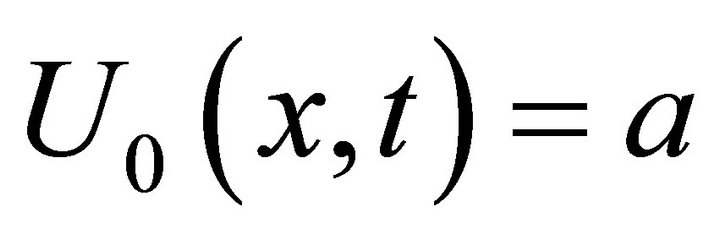 which is a constant.
which is a constant.
• For , the Equation (33) becomes
, the Equation (33) becomes
 (36)
(36)
While equating the terms of the Equation (36) to be zero we obtain ;
; 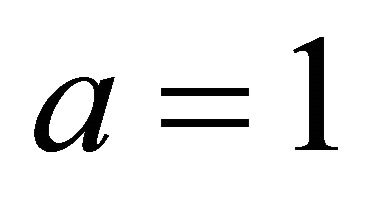 and the solution of the Equation (32) is
and the solution of the Equation (32) is
 . (37)
. (37)
In the case where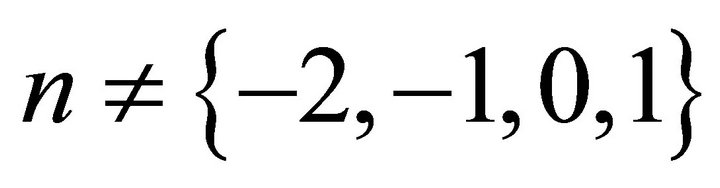 , we identify the terms of Equation (32) to zero and look for the non trivial solutions
, we identify the terms of Equation (32) to zero and look for the non trivial solutions  which give
which give 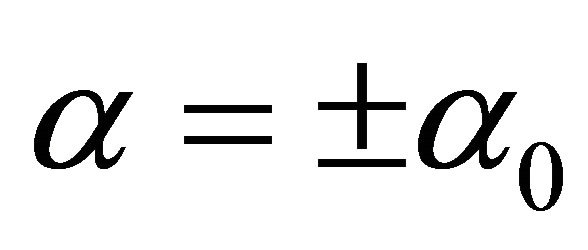 and
and . So we can affirm without hesitation that the general solution of the equation of Born-Infeld is
. So we can affirm without hesitation that the general solution of the equation of Born-Infeld is
 (38)
(38)
5. Conclusion
In this work, we constructed with success the solutions of shape Sechnof the KdV and Born-Infeld equations. Our survey has been guided by the fact that, we already know that by a direct integration, the initial KdV equation admits a solution in Sechn-  and we don’t know what happens precisely when
and we don’t know what happens precisely when . To this effect, we supposed that its solution is under the shape Sechn- to arrive at the conclusion
. To this effect, we supposed that its solution is under the shape Sechn- to arrive at the conclusion . The survey has been extended to the case of the modified KdV and Born-Infeld equations. The solutions of shape
. The survey has been extended to the case of the modified KdV and Born-Infeld equations. The solutions of shape
 for
for ,
,  and
and ,
,
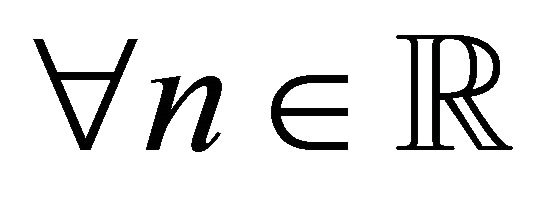 , have been obtained respectively. The success of this survey is due to the BDKm whose mastery permits to push the analysis as far as possible in the equations that present an elevated nonlinearity as seen in the two equations studied scrupulously.
, have been obtained respectively. The success of this survey is due to the BDKm whose mastery permits to push the analysis as far as possible in the equations that present an elevated nonlinearity as seen in the two equations studied scrupulously.
6. Acknowledgements
I acknowledge support from the ministry of Higher Education of Cameroon through its program of support to Research, which enabled me to carry out this work. I also thank Mr Tsapgou Jean Jeremie for some corrections after the reading of the work.