Quantum Entanglement: Where Dark Energy and Negative Gravity plus Accelerated Expansion of the Universe Comes from ()
1. Introduction
We utilize the wave-particle duality [1,2] and quantum entanglement [2-8] as fundamental features of quantum mechanics [1,8] as well as classical field, quantum set theory [3] and transfinite quantization [4-44] to develop an effective quantum gravity energy theory [1-8] that can convincingly resolve the problem of the accelerated rate of cosmic expansion and the missing dark energy of the cosmos and at the same time agrees with the accurate WMAP and Supernova cosmic energy density measurements and analysis [9-18]. It turns out that ordinary energy is intimately related to the position energy of a generic quantum particle [9,20] in five dimensional KaluzaKlein spacetime [2,4,6] and amounts to

where E is the energy, m is the mass, c is the speed of light,  is Hardy’s quantum entanglement and
is Hardy’s quantum entanglement and  [12,17,18] (see Figure 1). Dark energy on the other hand was found to be a hitherto unknown and unsuspected halo-like feature of a generic quantum probability wave with a negative kinetic energy inducing negative gravity (see Figure 2). Thus a certain broken symmetry governs particles and waves from a set theoretical perspective [1,8]. In short the quantum particle is the ultimate source of ordinary energy and contributes to
[12,17,18] (see Figure 1). Dark energy on the other hand was found to be a hitherto unknown and unsuspected halo-like feature of a generic quantum probability wave with a negative kinetic energy inducing negative gravity (see Figure 2). Thus a certain broken symmetry governs particles and waves from a set theoretical perspective [1,8]. In short the quantum particle is the ultimate source of ordinary energy and contributes to
 (a)
(a) (b)
(b)
Figure 1. (a) The set theoretical ordinary energy-dark energy duality; (b) The quintessence of dark energy from the topology of the cantor set.
about  percent of the total energy of the cosmos [18,19] (see Figures 1-12). In turn the quantum particle is modeled by the zero set represented dimensionally by von Neumann-Connes dimensional function [8,44] of a Penrose quasicrystal fractal tiling space [21-23]. The broken mirror image of the quantum particle is the halo-like quantum probability wave which is the source of a negative energy anti-gravity (see Figures 9 and 11) stemming from modeling the quantum wave via the empty set of the same Penrose quasicrystals fractal tiling [21-23,30-34,44]. Subsequently the energy of the quantum wave was found to be [12,18]
percent of the total energy of the cosmos [18,19] (see Figures 1-12). In turn the quantum particle is modeled by the zero set represented dimensionally by von Neumann-Connes dimensional function [8,44] of a Penrose quasicrystal fractal tiling space [21-23]. The broken mirror image of the quantum particle is the halo-like quantum probability wave which is the source of a negative energy anti-gravity (see Figures 9 and 11) stemming from modeling the quantum wave via the empty set of the same Penrose quasicrystals fractal tiling [21-23,30-34,44]. Subsequently the energy of the quantum wave was found to be [12,18]

It was then found to the Author’s delight that the sum of ordinary energy of the particle and absolute value of the negative dark energy of the wave added together is exactly the energy predicted by Einstein’s formula [2,3, 17]

Our most important conclusion in this work is that Einstein’s  gives the correct total energy formula for an infinitely large Cantor set-like clopen i.e. closed and open universe [24-29] but it does not distinguish between ordinary energy and negative dark energy of a basically fractal cosmos. Noting that measurement leads to quantum wave collapse, it is completely normal that we cannot measure dark energy using present day technology. In the following sections we will attempt to explain more accurately but concisely the points transacted above. For a quick overview, the entire analysis and results are summarized in Figures 1-3, while valuable details and additional information are presented in Figures 4-12. In Figures 13 and 14 we give a naive yet very instructive geometrical visualization and interpretation of the wave-particle duality in five dimensional Kaluza-Klein spacetime.
gives the correct total energy formula for an infinitely large Cantor set-like clopen i.e. closed and open universe [24-29] but it does not distinguish between ordinary energy and negative dark energy of a basically fractal cosmos. Noting that measurement leads to quantum wave collapse, it is completely normal that we cannot measure dark energy using present day technology. In the following sections we will attempt to explain more accurately but concisely the points transacted above. For a quick overview, the entire analysis and results are summarized in Figures 1-3, while valuable details and additional information are presented in Figures 4-12. In Figures 13 and 14 we give a naive yet very instructive geometrical visualization and interpretation of the wave-particle duality in five dimensional Kaluza-Klein spacetime.
2. Basic Concept
2.1 Ordinary Energy, the Zero Set and the Quantum Particle
Ordinary energy of the universe is directly proportional to the fractal nature of spacetime [4-12,30-34]. More accurately it is the multiplicative hyper Hausdorff measure or volume of the zero set i.e. the quantum particle in a five dimensional Kaluza-Klein universe . The zero set which possessrs the cardinality of the continuum is fixed using the Bi-dimensions formula [8]
. The zero set which possessrs the cardinality of the continuum is fixed using the Bi-dimensions formula [8]
 (1a)
(1a)
i.e. the topological dimension  and the Hausdorff dimension
and the Hausdorff dimension  so that we find
so that we find
 (1b)
(1b)
where  as in von Neumann-Connes’ non-commutative geometry [8,22,33] and its well known dimen dimensional function when applied to Penrose fractal clopen i.e. closed and open universe tiling [21,44].
as in von Neumann-Connes’ non-commutative geometry [8,22,33] and its well known dimen dimensional function when applied to Penrose fractal clopen i.e. closed and open universe tiling [21,44].
2.2. Dark Energy, the Empty Set and the Quantum Wave
Dark energy on the other hand is given by the dual additive hyper Hausdorff measure or volume of the empty set i.e. quantum wave in  Kaluza-Klein spacetime [4,6]. Thus we start from
Kaluza-Klein spacetime [4,6]. Thus we start from  which is the empty set given by [8]
which is the empty set given by [8]
 (2)
(2)
i.e.  [8,44].
[8,44].
2.3. The Hausdorff “Volume” of the Zero Set in Five Dimensional Space and the Quantum Particle
For the hyper Hausdorff volume of the fractal zero set of ordinary energy [8], we apply the intersection rule of sets in 5 Kaluza-Klein dimensions and find (see Figures 1(a) and (b))
 (3)
(3)
In a sense this Hausdorff measure is the “volume” or the energy density of a quantum particle [8].
The volume  could be naively interpreted geometrically as the generalization of the volume of a cube to a pseudo 5D cube with each side being of a length equal to
could be naively interpreted geometrically as the generalization of the volume of a cube to a pseudo 5D cube with each side being of a length equal to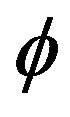 . (See Figures 13 and 14)
. (See Figures 13 and 14)
2.4. The Hausdorff “Volume” of the Empty Set in Five Dimensional Space and the Quantum Wave
On the other hand for the additive Hausdorff “volume” which is equal the length of the empty set [22,33] of dark energy we have the addition or union rule of sets in 5 K-K dimension and one finds (see Figures 1(a) and (b))
 (4)
(4)
We used here the union of sets rather than the intersection because of the duality between particle and wave [2,8] (see Figures 13 and 14). Consequently the Hausdorff measure of the empty set in K-K spacetime represents the energy density of a quantum wave which does not exist in the usual conventional quantum interpretation because a conventional quantum wave in orthodox quantum mechanics is a probability wave and has no
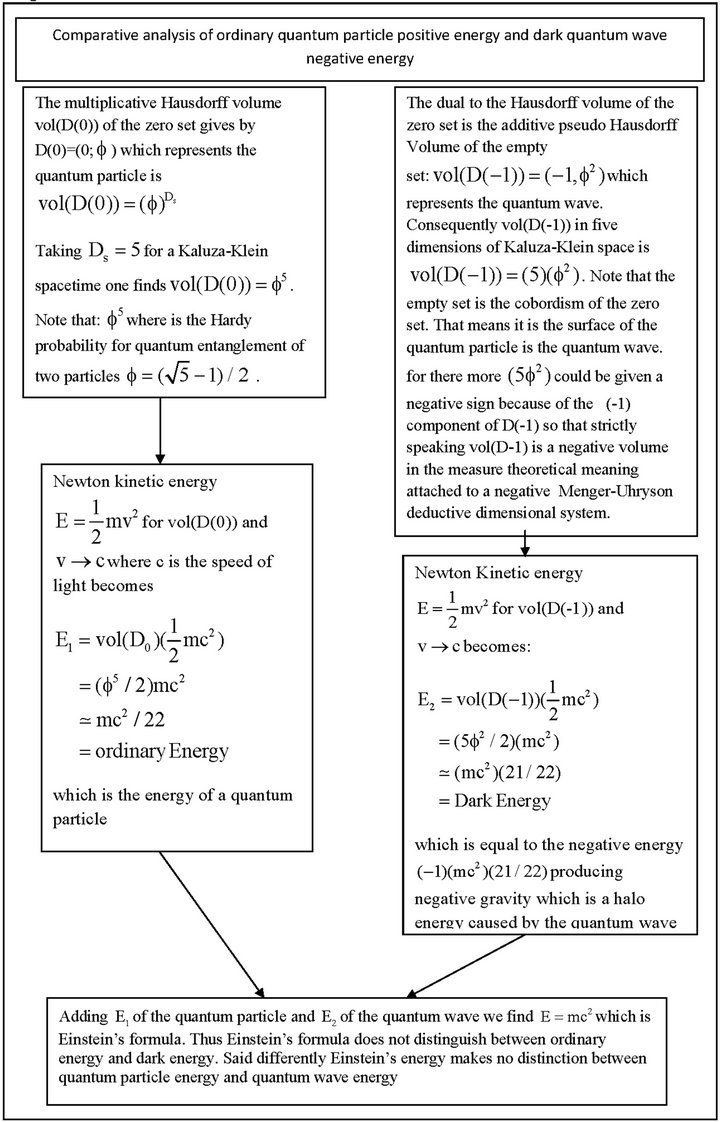
Figure 3. Comparative analysis of ordinary quantum particle positive energy and dark quantum wave negative energy.

Figure 4. The universe as a fractal in infinity space.

Figure 5. Special relativity with extra dimensional twist or all roads lead to Rome and all theories lead to .
.

Figure 6. Yang-Mills for dark energy. Deriving  from quantum field theory in curved spacetime.
from quantum field theory in curved spacetime.

Figure 7. A Beautiful mind for dark energy. A flow chart starting from Nash embedding and Witten’s M-Theory explaining the logical connections and sequential deduction using Einstein relativity and leading to quantum relativity energy .
.

Figure 8. Bosonic strings interpretation of 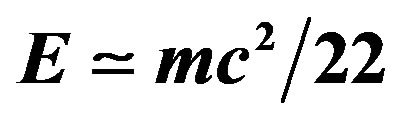 and varying speed of Light Theory
and varying speed of Light Theory .
.
ordinary energy and collapses at measurement [1,2]. and collapse at measurement [1,2]. Again a possible naïvegeometrical interpretation of  is being a one dimensional “surface” i.e. the circumference of a pentagon with each of the 5 sides being of a length equal to
is being a one dimensional “surface” i.e. the circumference of a pentagon with each of the 5 sides being of a length equal to 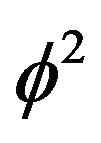 (see Figure 13). In particular the quantum wave determines the most probable location of a quantum particle but vanishes for a classically large object which is the contrary effect of negative gravity induced by the same wave i.e. anti gravity vanishes locally and exists only by accumulation at cosmic scales.
(see Figure 13). In particular the quantum wave determines the most probable location of a quantum particle but vanishes for a classically large object which is the contrary effect of negative gravity induced by the same wave i.e. anti gravity vanishes locally and exists only by accumulation at cosmic scales.
2.5. The Average Hausdorff Volume of Quantum Particle and Wave as Total Relativity Energy
From the above points (3) and (4) we see that the total geometrical density or pseudo Hausdorff “volume” is the sum of both the fractal part of spacetime  and the voids “empty” part of spacetime
and the voids “empty” part of spacetime . This means (see Figures 1(a) and (b) as well as Figures 13 and 14)
. This means (see Figures 1(a) and (b) as well as Figures 13 and 14)
 (5)
(5)
This is an extremely important result because an arithmetic average geometrical density or Hausdorff “dimensional volume” would give us
 (6)
(6)
On the other hand the measure or “volume” or geometrical density of the unitarity set  is
is  and leads directly to Einstein’s celebrated energy density formula [2,5,17] (see Figures 1 and 2)
and leads directly to Einstein’s celebrated energy density formula [2,5,17] (see Figures 1 and 2)
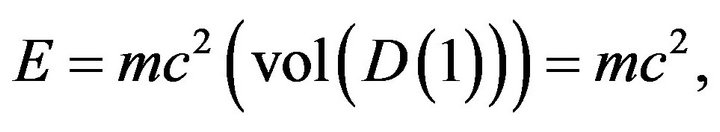 (7)
(7)
where E is the energy, m is the mass and c is the speed of light [2,3,12,17].
 (a)
(a) (b)
(b)
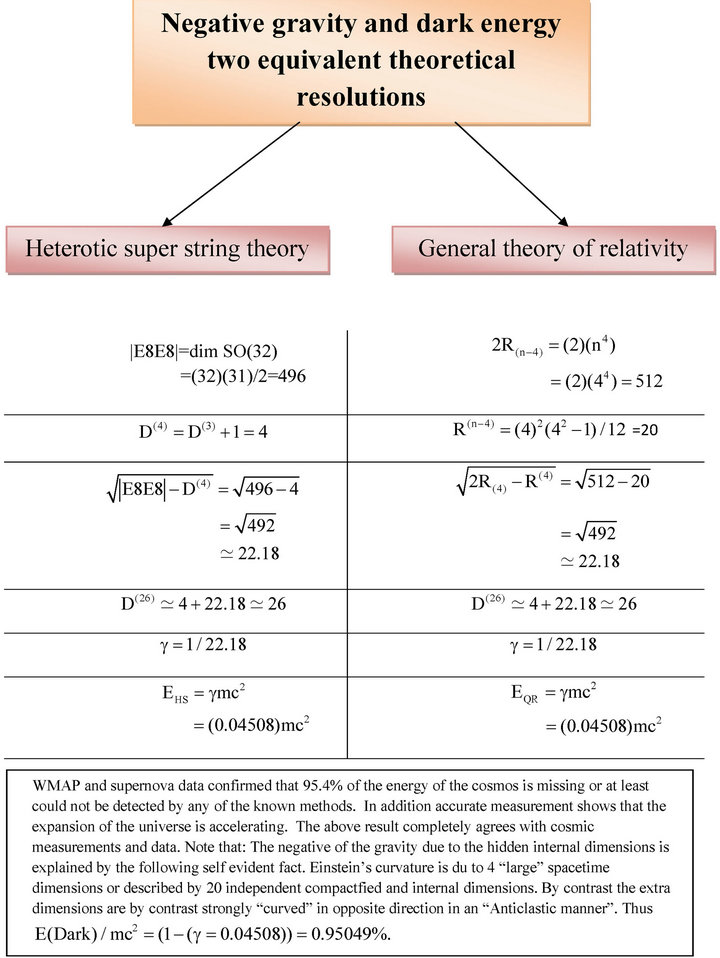
Figure 9. The deriving force behind gravity and negative gravity.
The fractal meaning of the constancy of the speed of light is explained in Figure 11 to round up the discussion and stress the fundamental role played by fractal geometry of spacetime in determining the duality of ordinary and dark energy [12-14,22,33].
3. Analysis
3.1. The Speed of Light, Positive Energy, Negative Energy and Einstein’s Energy
Noting the result of point (2.5) we see that Einstein’s equation may be viewed as based on an arithmetic average density or “volume” of 5D Kaluza-Klein space which does not differentiate between fractals due to voids and the voids in spacetime themselves and consequently Einstein’s energy density is simply given by the famous equation based on continuous geometry and unit hyper volume equal to one [12,26]:
 (8)
(8)
On the other hand ignoring the density of the voids and considering only the fractal part of spacetime represented by the five dimensional zero set, we then find the quantum relativity formula of ordinary energy density validated by direct cosmic measurement namely [12,17, 18] (see Figure 2)
 (9)
(9)
Note that division by 2 in the above equation is due to arithmetic averaging and not due to using Newton’s kinetic energy because in this particular interpretation velocity tends to be the velocity of light without multiplying it with  as in unit interval physics using Sigalotti’s critical value [28,29]. For an alternative derivation of
as in unit interval physics using Sigalotti’s critical value [28,29]. For an alternative derivation of  see Figures 3-8 as well as Figures 13 and 14.
see Figures 3-8 as well as Figures 13 and 14.
By contrast if we consider spacetime voids only as represented by the quantum wave i.e. the empty set in 5D Kaluza-Klein theory (which means that it is the so-called dark energy of the cosmos), then we have the following complementary quantum relativity energy density formula: (see Figure 2 as well as Figures 13 and 14)
 (10)
(10)
 (a)
(a) (b)
(b)
Figure 10. (a) Complexity of maximal entropy and minimum entropy are equal; (b) Negative dimension and negative Kelvin temperature.

Figure 11. An overview regarding the constancy of the speed of light beeing a fractal mean value at the classical-quantum interface and thus constant.
where

[22,34]
3.2. Einstein’s Energy Formula and a New Point of View
From points discussed in the preceding sections we can conclude that Einstein’s formula  is blind to the difference between ordinary energy i.e. quantum particle energy and dark energy i.e. the quantum wave energy because it presupposes a continuous smooth spacetime and never addresses any questions related to fractality or sparseness of spacetime which is the cause of the constancy of the speed of light at the classical interface being a fractal average (see Figure 11) [8,12,13,30]. However there are more profound consequences of the
is blind to the difference between ordinary energy i.e. quantum particle energy and dark energy i.e. the quantum wave energy because it presupposes a continuous smooth spacetime and never addresses any questions related to fractality or sparseness of spacetime which is the cause of the constancy of the speed of light at the classical interface being a fractal average (see Figure 11) [8,12,13,30]. However there are more profound consequences of the

Figure 12. An overview regarding Gödel’s universe.

Figure 13. A naive geometrical interpretation of geometrical density or Hausdorff measure  which decide upon the magnitude of dark energy of the quantum wave
which decide upon the magnitude of dark energy of the quantum wave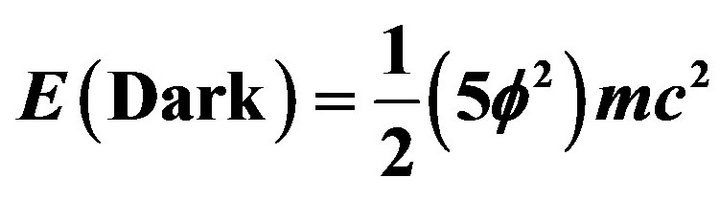 .
.
spacetime voids than that which will be discussed in the next paragraph. For the moment we have only to recall that the wave is the cobordism i.e. the surface of the quantum particle and thus particle and wave are inseparable [8].
3.3. The Negative Sign of the Quantum Wave Energy and Anti Gravity
If we consider the effect of the two topological dimensions of the zero set and the empty set on our average pseudo Hausdorff Kaluza-Klein hyper volume or geometrical density then we could work out the following modified negative arithmetic average [8] (see Figures 1 and 2)
 (11)
(11)
Consequently dark energy acquires in this case the

Figure 14.A naive geometrical interpretation of  of the ordinary energy of the quantum particle
of the ordinary energy of the quantum particle .
.
negative sign of the topological dimension of the empty set corresponding to the basic compactification equation of bosonic string theory [2,6,17]
 (12)
(12)
This negative sign (see Figure 9) is therefore an additional indication that dark energy or what is equivalently the energy of the quantum wave i.e. the empty set differs fundamentally from ordinary energy for being negative and represents effectively negative gravity pushing in the opposite direction of classical gravity and causing the cosmic expansion of the Universe to accelerate rather than de-accelerate in agreement with relatively recent accurate observations [9-17].
4. Intermediate Discussion
We conclude that while fractals i.e. the zero set or phenomenologically the quantum particle is behind ordinary energy, voids in spacetime corresponding to the empty set i.e. the quantum wave i.e. the halo of the quantum particle [8,23,33,44] are not only behind dark energy but also are the cause of negative gravity and the increased rate of cosmic expansion [9-11,15,16]. Negative gravity is essentially due to negative curvature of spacetime. If we see gravity as the Riemannian curvature of  spacetime, then negative curvature [9] is related to
spacetime, then negative curvature [9] is related to  and is akin to the anticlastic curvature (see Figure 9) known from the behavior of elastic materials and theories of elasticity and plasticity [35-37]. A torsion tensor like in Cosserat micropolar media and similar classical field theories may actually simulate something similar to Cartan relativity and admit negative curvature and antigravity [2]. Thus we have here the intuitive phenomenological explanation arising from the effect of Poisson’s ratio that when a long thick beam [35-37] is curved in the longitudinal direction then cross section curves in the opposite perpendicular direction [35]. Consequently, we could say that
and is akin to the anticlastic curvature (see Figure 9) known from the behavior of elastic materials and theories of elasticity and plasticity [35-37]. A torsion tensor like in Cosserat micropolar media and similar classical field theories may actually simulate something similar to Cartan relativity and admit negative curvature and antigravity [2]. Thus we have here the intuitive phenomenological explanation arising from the effect of Poisson’s ratio that when a long thick beam [35-37] is curved in the longitudinal direction then cross section curves in the opposite perpendicular direction [35]. Consequently, we could say that  has the effect of an anti-curvature producing anti-gravity [9] due to negative energy of the halo [9] of the quantum particle i.e. the quantum wave which we have dubbed dark energy for want of a better physical word communicating the deep meaning [9-18]. We can go even further still and claim that negative absolute temperature is another physical manifestation of negative dimensions and the empty set (see Figure 10) and therefore negative energy and consequently dark energy [41]. The epistemological question of how a wave devoid of ordinary energy could induce a measurable negative gravity effect is essentially the other side of the fact that this same wave decides on the location of a quantum particle as confirmed by theory and experiment [1-3]. In other words the quantum probability wave possesses an ontological quality. Said differently we know that measurement collapses the quantum wave by converting an empty set particle to zero set wave. Consequently cosmic measurement collapses the Hawking-Hartle wave solution of the Wheeler-DeWitt Schrödinger equation of the universe and therefore we cannot see or measure any energy of this wave unless we develop quantum nondemolition measurement instrument [1].
has the effect of an anti-curvature producing anti-gravity [9] due to negative energy of the halo [9] of the quantum particle i.e. the quantum wave which we have dubbed dark energy for want of a better physical word communicating the deep meaning [9-18]. We can go even further still and claim that negative absolute temperature is another physical manifestation of negative dimensions and the empty set (see Figure 10) and therefore negative energy and consequently dark energy [41]. The epistemological question of how a wave devoid of ordinary energy could induce a measurable negative gravity effect is essentially the other side of the fact that this same wave decides on the location of a quantum particle as confirmed by theory and experiment [1-3]. In other words the quantum probability wave possesses an ontological quality. Said differently we know that measurement collapses the quantum wave by converting an empty set particle to zero set wave. Consequently cosmic measurement collapses the Hawking-Hartle wave solution of the Wheeler-DeWitt Schrödinger equation of the universe and therefore we cannot see or measure any energy of this wave unless we develop quantum nondemolition measurement instrument [1].
5. Various Interpretations and Duality in Five Dimensional Kaluza-Klein Spacetime
Ordinary energy density and dark energy density are two dual yet complementary faces to a one common energy given by the sum of both which is equal to Einstein’s famous equation  [2]. Unlike ordinary energy which is entirely rooted in the zero set describing a generic quantum particle state, dark energy is solely due to the quantum wave aspect of the quantum particle as modeled by the empty set [8]. Nothing could show this symmetric duality better than writing both types of energies in the Magueijo-Smolin formalism [5,26] and their elegant energy expression (see Figure 3):
[2]. Unlike ordinary energy which is entirely rooted in the zero set describing a generic quantum particle state, dark energy is solely due to the quantum wave aspect of the quantum particle as modeled by the empty set [8]. Nothing could show this symmetric duality better than writing both types of energies in the Magueijo-Smolin formalism [5,26] and their elegant energy expression (see Figure 3):
 (13)
(13)
Here m is the five dimensional Kaluza-Klein mass [38,39], c is the speed of light and  is the Planck energy. Using the unit interval set theoretical value for
is the Planck energy. Using the unit interval set theoretical value for  it was reasoned earlier on that we must have:
it was reasoned earlier on that we must have:  and inserting in E we find that [12,17] (see Figure 3)
and inserting in E we find that [12,17] (see Figure 3)
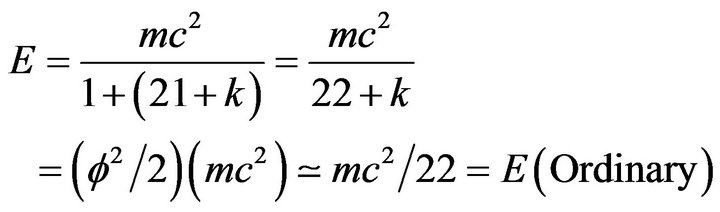 (14)
(14)
exactly as should be. However using Witten’s T-duality [2,4,6,40] (see Figure 3):
 (15)
(15)
and inserting in E one finds
 (16)
(16)
where  [22,33].
[22,33].
Thus we have demonstrated in the present work and presumably for the first time that dark energy is essentially the absolute value of the negative energy density of the Hawking-Hartle quantum wave solution of Feynman-Wheeler-DeWitt Schrödinger equation of the entire universe and as such it induces the hitherto unknown phenomena of negative gravity which we and others consider to be the cause of the observed increased rate of cosmic expansion [9-18]. This physical interpretation makes a great deal of sense because the set theoretical definition of the quantum wave is the empty set represented by  whose topological dimension is minus one (−1). Consequently the accurate way to write down the quantum wave energy density is [8,9,32]
whose topological dimension is minus one (−1). Consequently the accurate way to write down the quantum wave energy density is [8,9,32]
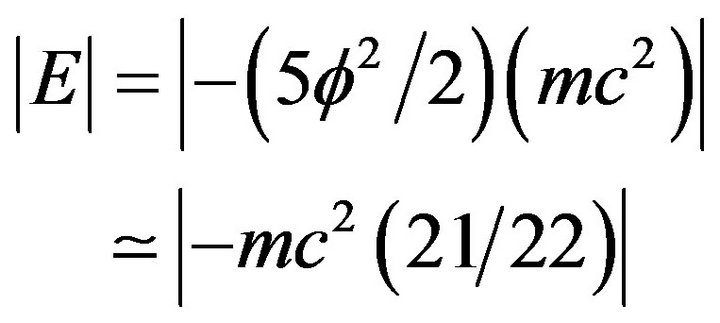 (17)
(17)
where 5 is the dimension of Kaluza-Klein spacetime [42, 43]. To gain an even deeper understanding of the broken symmetry and duality between particle and wave and consequently between ordinary energy and negative or dark energy, let us start this time from Newton’s classical expression for the kinetic energy [2]
 (18)
(18)
Recalling the set theoretical duality between the quantum particle zero set and the quantum wave empty set [8]
 and
and  (19)
(19)
as well as the dual transformation given for particle and wave as in the following box:
Box No. 1. Particle-wave duality transformation.
| For particle |
For wave |
| 
|

|
Then inserting in  one finds the following particle and wave energy as per the next box:
one finds the following particle and wave energy as per the next box:
Box No.2. Quantum particle ordinary energy and quantum wave dark energy.
| For particle |
For wave |
| 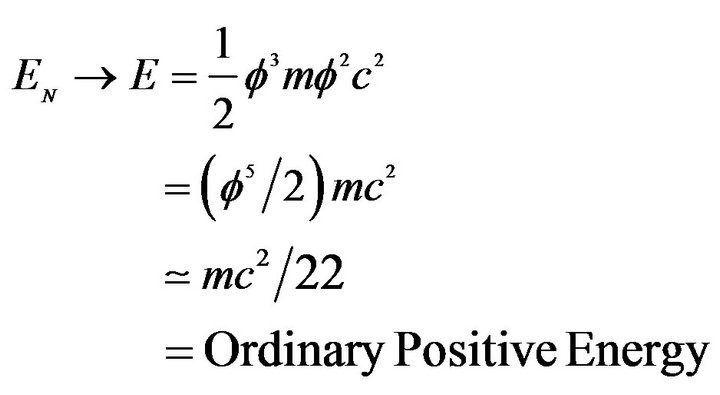
|

|
Let us contemplate the meaning of  in
in  and 5 in 5m as well as
and 5 in 5m as well as  in
in . The simplest way to explain it in the present context is
. The simplest way to explain it in the present context is  in
in . This stems from Sigalotti’s critical speed given by
. This stems from Sigalotti’s critical speed given by  The
The 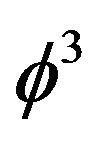 on the other hand is
on the other hand is  where
where  is the Hausdorff dimension of a four dimensional set given by
is the Hausdorff dimension of a four dimensional set given by  and representing the core of fractal-Cantorian spacetime [21,22]. At the same time
and representing the core of fractal-Cantorian spacetime [21,22]. At the same time  is the global quantum entanglement probability or what some call the counter factual effect of the spacetime surrounding a quantum particle [32]. It is numerically equal to the Unruh dimensionless thermal temperature [2] and together with
is the global quantum entanglement probability or what some call the counter factual effect of the spacetime surrounding a quantum particle [32]. It is numerically equal to the Unruh dimensionless thermal temperature [2] and together with  gives
gives  which is glement of two quantum particles [37]. On the other hand the appearance of 5 in 5m maybe seen as the five dimensionality of a Kaluza-Klein spacetime [2,4,6]. This is an ordinary 4D-Einstien space with a single additional cyclical pipe-like compactified extra dimension joining electro magnetism to gravity [38,39,42,43] (see Figure 4). It could be also seen as an extra spin half fermionic dimension [22]. However like the bosonic fractal dimension
which is glement of two quantum particles [37]. On the other hand the appearance of 5 in 5m maybe seen as the five dimensionality of a Kaluza-Klein spacetime [2,4,6]. This is an ordinary 4D-Einstien space with a single additional cyclical pipe-like compactified extra dimension joining electro magnetism to gravity [38,39,42,43] (see Figure 4). It could be also seen as an extra spin half fermionic dimension [22]. However like the bosonic fractal dimension  enters into the energy equation of the quantum particle in the inverse form
enters into the energy equation of the quantum particle in the inverse form , the non-fractal five dimensional fermionic K-K spacetime [38,39] enters into the energy equation of the quantum wave or dark energy directly simply as factor 5. For the sake of completeness let us see what would result from considering a fractal i.e. a transfinitely quantized KleinKaluza spacetime developed by the Author [38,39, 42,43] with
, the non-fractal five dimensional fermionic K-K spacetime [38,39] enters into the energy equation of the quantum wave or dark energy directly simply as factor 5. For the sake of completeness let us see what would result from considering a fractal i.e. a transfinitely quantized KleinKaluza spacetime developed by the Author [38,39, 42,43] with  which means (see Figure 4)
which means (see Figure 4)
 (20)
(20)
In this case  would mislead us into wrongly thinking that dark energy is equal to Einstein’s energy (see Figures 1 and 4)
would mislead us into wrongly thinking that dark energy is equal to Einstein’s energy (see Figures 1 and 4)
 (21)
(21)
This is initially a surprising result but it is a misunderstanding as well because it shows that E(Einstein) is obtainable from a fractal five-dimension Kaluza-Klein spacetime while dark energy is the child of a mere fivedimensional non-fractal Kaluza-Klein spacetime. However the difference is namely that  is the factor responsible for the additional energy which is nothing but the ordinary energy which when added to non-fractal 5D Klein Kaluza [38,39] i.e. to dark energy gives the total energy, namely that of Einstein’s energy. This confirms all of our earlier results namely that
is the factor responsible for the additional energy which is nothing but the ordinary energy which when added to non-fractal 5D Klein Kaluza [38,39] i.e. to dark energy gives the total energy, namely that of Einstein’s energy. This confirms all of our earlier results namely that  could be obtained via either a smooth 4D spacetime or via a delicate analysis using a fractal
could be obtained via either a smooth 4D spacetime or via a delicate analysis using a fractal 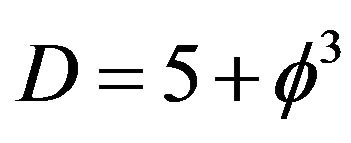 five dimension spacetime (see Figure 4). Seen that way
five dimension spacetime (see Figure 4). Seen that way  which is related to the fractal 11-D theory via
which is related to the fractal 11-D theory via  is a more fundamental theory than Einstein’s
is a more fundamental theory than Einstein’s 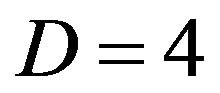 spacetime theory. In fact we could deduce
spacetime theory. In fact we could deduce  from
from  by dividing by 2 and subtracting the number weight of 1 photon [20] namely
by dividing by 2 and subtracting the number weight of 1 photon [20] namely  so that one finds (see Figure 4)
so that one finds (see Figure 4)
 (22)
(22)
In other words we can either interpret  in
in  as a hyper volume or Hausdorff measure and division by 2 as averaging or we see it as the transformation
as a hyper volume or Hausdorff measure and division by 2 as averaging or we see it as the transformation  and
and  and division by 2 as coming from using Newton’s kinetic energy.
and division by 2 as coming from using Newton’s kinetic energy.
We conclude this section by stressing that there are three and not only one Kaluza-Klein theories. The first is the classical 5D theory of Kaluza [41]. The second is the quantized 5D theory of Klein [41,42]. The third is the transfinitely quantized  theory developed by the author [38,39,43].
theory developed by the author [38,39,43].
6. Conclusions
Dark energy is a non-classical form of negative energy density stemming from the quantum wave empty set characteristic and we found it quantitatively to be given by the absolute value of
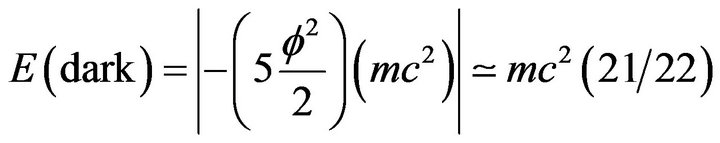 (23)
(23)
where  could be interpreted as the local Hardy’s type quantum entanglement of two particles or the Hausdorff dimension of the empty set and
could be interpreted as the local Hardy’s type quantum entanglement of two particles or the Hausdorff dimension of the empty set and .
.
Ordinary energy on the other hand is given by the positive energy of the zero set describing the quantum particle and found in the present work to be equal [12]
 (24)
(24)
where 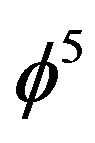 is the famous golden mean Hardy’s quantum entanglement [2,31].
is the famous golden mean Hardy’s quantum entanglement [2,31].
There are numerous other methods and theories which lead to essentially the same conclusion regarding ordinary positive energy density being equal to Einstein’s energy divided by 22 which are summarized in Figures 5-8. In particular Figure 7 shows how to derive 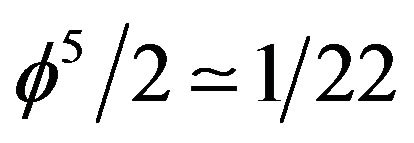 from Nash’s embedding plus the notion of spacetime sparseness and Figure 8 gives an idea about the role of bosonic strings dimension.
from Nash’s embedding plus the notion of spacetime sparseness and Figure 8 gives an idea about the role of bosonic strings dimension.
A particular tantalizing result is that the sum of both energies, dark energy and ordinary energy, is a neat confirmation of Einstein’s famous relativity formula because
 (25)
(25)
The negatives of the dark energy on the other hand confirm earlier string theoretical conjectures that negative gravity is due to negative energy of the compactified 22 dimensions which produce negative curvature as per the bosonic string dimensional equation [2,4,12]
 (26)
(26)
Following the same reasoning one can write the  factor of dark energy as
factor of dark energy as
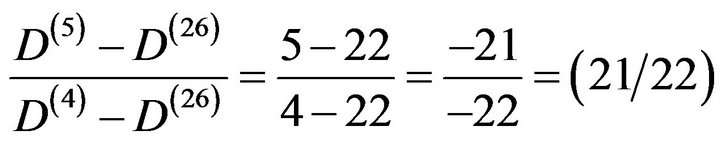 (27)
(27)
which proves that  of ordinary energy and
of ordinary energy and  of dark energy have opposite signs in any event. In fact the present theory strongly suggests the physical reality of Kaluza-Klein extra fifth dimension [42,43] as well as the fractality of spacetime and ontological reality of the quantum wave [8,13,14,20,43,44] all apart from the reality of dark energy which we cannot see or measure because measurement collapses the quantum wave.
of dark energy have opposite signs in any event. In fact the present theory strongly suggests the physical reality of Kaluza-Klein extra fifth dimension [42,43] as well as the fractality of spacetime and ontological reality of the quantum wave [8,13,14,20,43,44] all apart from the reality of dark energy which we cannot see or measure because measurement collapses the quantum wave.
For a different view of negative gravity via general relativity and comparison with Heterotic string theory see Figure 9 and commentary therein.
It is worth noting that negative dimensions are behind the experimentally observed negative Kelvin absolute temperature [41] and may thus be looked upon as another physical interpretation of dark energy (see Figures 10(a) and (b)). Furthermore if the building blocks of spacetime are random Cantor sets as the present work suggests, then our cosmos is clopen i.e. opened and closed because Cantor sets are clopen.
We could understand the repelling effect of dark energy on a cosmic scale as a phenomenon basically due to the fact that the motion of quantum objects and its spatial location is determined completely by the quantum wave following prescription [1-3]. It is a matter of speculation that the spinning of Gödel’s universe (see Figure 12) [40] is related to dark energy and repelling gravity. Finally the constancy of the speed of light as a fractal average is explained in some detail in Figure 11.