Groupoid Approach to Ergodic Dynamical System of Commutative von Neumann Algebra ()
1. Preliminaries
Assuming the preliminary materials of [1] which form the background of this work, we are motivated to explore an alternative approach to the representation of the dynamical system of the commutative algebra
on a commutative von Neumann algebra using groupoid framework.
This work uses the more complex and profound analytic method, according to [2] , for operator algebra. In place of the usual tool of polar decomposition of linear forms, we employ the inherent decomposition of measures within the measure groupoid. This enables the use of groupoid equivalence to present the relations existing between the von Neumann algebra and its commutants. Hence, the groupoid representations relate the predual of the algebra to the Hilbert space constituting its domain.
The starting point for the analytical method is the relationship between the closure of a space or subspace with the boundedness of the functions defined on it. Because the measurability of functions imposes a very little restriction on the space, according to Connes [3] , which translates to closure of the space or subspace supporting the function; the space must be a closed interval
or a standard Borel space X for measurability to be granted. Hence, measurability of X is a structure defined on X by measurable functions. This measure structure is invariant under
the transformations of X. (cf. [3] ). The definition of a Borel measure as a positive operator valued set map by [4] connects these structures to operator algebra.
The connection is based on the positivity and completeness of the Hilbert space of square integrable functions
, and the fact that the
Radon-Nikodym Theorem asserts that the derivatives
are measurable
functions f on X. It follows that while the Borel structure on X gives rise to the Hilbert space
and von Neumann algebra, the topological structure defined by continuous functions
gives rise to the Banach algebra
with norm
. This good interaction between the two
structures is established using the Borel measures on X, whose mutual derivatives
define measurable functions, and define linear forms
on
and bilinear/sesquilinear forms or inner product
on
, with
.
Spectral consideration is used to identify the algebra of these measures as von Neumann algebra of operators on
. For the spectrum of a self-adjoint operator
can be analysed using polynomials
which which are constitutive of the maximal ideals
and the geometric structure of the algebra
. Every polynomial
on X defines an operator as a measurable function, and there is always a sequence of polynomials uniformly approximating a measurable function by Weierstrass approximation theorem. Thus, the continuous extensions of any continuous function vanishing at a point
to a function vanishing in some closed subsets containing x define the spectrum of the resulting partially ordered operators. Cf. [4] .
These continuous extensions are captured by the uniformly convergent
sequences or nets, such that the sequence
means
for all
; which also gives rise to weak convergence
,
. A net defines a unique measure class
on X supported on the closed (compact) interval
such that
.
This interval is related to the spectral radius of the operator T in a von Neumann algebra
which can be defined as follows.
Definition 1.1 (Cf. [3] ) A commutative von Neumann algebra
is the algebra of operators on
of the form
for some bounded Borel function f. This is called the von Neumann algebra generated by the operator T.
Hence,
is made up of operators with the same symmetries as T. This means that they commute with all unitary operators (
, commuting with T. Thus, given that
, then
, where
for some Borel function f. The commutative von Neumann algebra
is naturally isomorphic to
-the algebra of bounded measurable functions on
that is equal
-a.e.
Because proper actions relate directly to slice theorem used in the cohomogeneity-one G-space analysis as in [5] [6] [7] , some of the main results of the paper also relate to slice theorem.
2. The Algebra and the Generalized Space
According to [8] , the time evolution of dynamical systems modelled by measure-preserving actions of integers
or real numbers
which represent passage of time are generalized by measure-preserving actions of lattices which are usually “subgroup” of Lie groups. The two basic constituents of the commutative algebra
: the Borel group of units
, and the maximal ideals
, are used to model the above in the action of
. The dynamical system defined by the Borel group
of units on the geometric point
is the ergodic action of the (lattice) algebra
on the generalized space
of nonnegative Radon measures on the space X; and the maximal ideals
are the (projective) modules which characterize and encode the symmetries of measurable functions vanishing on the neighbourhoods of each point of X.
These symmetries are represented by z-ultrafilters
of zero sets (affine algebraic varieties of
) converging to each
. The complements of these algebraic sets constitute the open neighbourhood base of points of X. The ultrafilter
convergence of closed sets to x has associated nets of polynomials or measurable functions converging to a function f defined on x. Given a net
of contractions in the complete metric space X, as in [9] , it follows that
such that
. All these are represented on the generalized space
with ergodic action of
in form of ergodic groupoid.
The idea of a generalized space
of Radon measures on X which is conceived as the state space (cf. [8] ) is a direct extension of Mackey’s conception of a measure class C as a generalized subset. At the centre of this extension is the focus on i) measure preserving transformations of the compact metric space X, and ii) the Dirac measures
as generalized or geometric points embedding the points of X in the generalized space. The role assigned to the ergodic transformations by Mackey, is to translate along time in such a way as to ensure the invariance of measure or state.
Every measure preserving continuous linear transformation
decomposes into ergodic components. This is based on the fact that the “noncommutative spaces” replacing the “phase space” are basically quotient spaces determined by ergodic actions of a Borel group. Hence, X is embedded in the generalized space as its geometric or generalized points; that is, the
-space
. Within this quotient setting, it is clear that non-ergodic transformations are constituted by ergodic components which are considered limits of nets of the former. This makes ergodic sense of what is said above on the operator T, and its transforms
constituting the von Neumann algebra
, and the commutants
made up of unitary operators
leaving them invariant. This is given as follows.
Proposition 2.1 The algebra
defines an ergodic and equivariant transformations
by its Borel group
on X and on the generalized space
.
Proof. The homothety
defined by
, is a transformation of balls
centred at x. It is measure preserving since the push forward of a Radon measure
under the map is given as
,
. The measure class is preserved because
for all
,
.
According to [8] , ergodic theorems express a relationship between averages taken along the orbit of a point under the iteration of a measure-preserving map or transformation. The iteration of the transformations
on X which induces
on the generalized points
represents passage of time,
and its invariance in both spaces
constitutes limits of nets of transformations involving the maximal filter convergence
and the convergence of net of tangent measures of
. These
represent averages over time.
The induced iteration on the generalized space
with respect to some invariant measure
(or measure class
) represents
invariance over states. The ergodicity represented by average over space or states (averages taken over the classes of measures) is given by nets of invariant nonergodic measures converging to an ergodic limit. It is also the convergence of operators to an ergodic operator in a von Neumann algebra. Cf. [4] . These two averages are given by the invariance or stability of
and the measure
classes
under
-actions.
Remark 2.2 From the proposition, we see that the restriction of
to the generalized points
gives a transformation of
defined as
, such that for any
, we have
This shows that the set
of the generalized points can be continuously and affinely extended to the generalized space
.
Subsequently, the generalized points
can generate the generalized space
and the generalized subspaces
or the measure classes. This is stated in the following result.
Proposition 2.3 The generalized space
of Radon measures on X is an affine and continuous extension of the geometric points
.
Proof. The coincidence of zero sets of
with null sets of
establishes the existence of nets
of non-ergodic Radon measures related to
which converge to the Dirac measures
as the z-ultrafilter
converges
. Since the elements of
vanish at x, its
-action is transferred to fibre of measure classes (or tangent measures to
) via
(see [1] ). □
From this result, the dynamism defined by the transformations
is encoded in the symmetry of the measures classes contributing to the convergent nets. Hence, the connection between ergodic theory and the dynamics defined by continuous transformations on compact metric spaces is encoded by the closure of the resulting convex set of non-ergodic
-invariant measures with ergodic measures as boundary. Cf. [8] .
3. The Principal Groupoid and its Action
In what follows, we present the action of the commutative algebra
using the groupoid equivalence. The
-action is determined at each point
by the maximal ideal
and the Borel group
. The maximal ideal is a module of the (lattice) algebra
and a
-space at every point
. Hence, there is a trivialization of an action groupoid on X which we will now explore in order to describe the
dynamical system on X and on the generalized space
.
The two algebraic objects
and
aid in the understanding of the dynamics associated to the commutative algebra
at each
. Their employment also associates a z-ultrafilter related to a maximal ideal
to the dynamical system. Thus, given the zero map
, the family of closed sets
is a closed cover for X. Cf. [10] . An open cover for X can be constructed from their complements, a countable number of
, such that
is an open covering for X and each inverse image
is fibrewise homeomorphic to
. These give a system of homeomorphisms
forming the transition functions
These transition functions also form unitary group if the Borel measures defined on the closed subsets are given the following characterisation.
Definition 3.1 [4] A positive operator valued measure is a triple
, where X is a set,
is a ring (or σ-algebra) of subsets of X, and
is an operator valued set function on
with the following properties
1)
is positive, i.e.
for each
.
2)
is additive, i.e.
whenever
in
.
3)
is continuous in the sense that
if
is an increasing sequence of sets in
whose union M is also in
. So
is called positive operator-valued measure on X or
. It is monotone on
if
.
These conditions are satisfied by the complements of ultra filters of zero sets
of the maximal ideals
of
[10] . Given a maximal filter
, we have
, where
; it follows that
is an increasing sequence of Hermitian operators, with
.
Proposition 3.2 The group of automorphisms or transformations
of X constitutes the unitary group
of the space of operators
.
Proof. That
is positive operator-valued implies the map
. Then Borel measures on X define positive operators on
since
for any pair
. As already noted, they are also linear forms
on
by the map
.
is identified with the unitary operator on
given by
and
with a closed subgroup of the unitary
, where the map
is the Koopman representation of
by [11] . Thus,
is a subgroup of the unitary group in view of the positive operators defined by the measures associated with
. □
Proposition 3.3 The transformations
define the system of homeomorphisms which are the transition functions of the fibre bundle structure.
Proof. Given the definition of operator valued measures and with the preceding formulations, the group of automorphisms or transformations
of X then constitutes the structure group of the fibre bundle since it defines an action on the fibres
given as
The action is fibrewise since
, where Z is the zero map. □
We now use symmetry groupoid to capture these bundle symmetries.
Theorem 3.4 The symmetries of the commutative algebra
give rise to a (Lie) symmetry groupoid.
Proof. We use the trivialized action of the group of units
on each maximal ideal
to formulate the bundle structure. The indexed family
of geometries constitute a bundle
over X, with the projection
, such that
. The “symmetries” of these geometric (closed) points of the commutative algebra
is expressed by the groupoid
. Hence, with
a vector bundle, and
the set of all vector space isomorphims
for
. The Borel group
of automorphisms of
expresses the particular “symmetry” of
; and the groupoid
expresses the smoothly “varying symmetries” of the bundle.
The smooth bundle symmetry
is a Lie groupoid on X with respect to the following structure. For
; the objection map is
, the partial multiplication is the composition of maps; the inverse of
is its inverse as an isomorphism. The isotropy groups are the general linear groups
of the fibres which are all isomorphic [12] . □
Remark 3.5 The general linear groups
coincide with the unitary group when the bundle is considered a Hilbert bundle. They define the (partial) symmetries of the system, which the Lie groupoid
represents.
Given the Lie groupoid
, its symmetries are modelled on the generalized space
. This will be achieved through the formulation of the action of the Lie groupoid
on the space of the generalized points
homeomorphic to
. This will present the generalized space
as a measure groupoid giving a generalized measure-theoretic approach to the dynamical system defined by the action of
[13] .
From Mackey’s definition of generalized subset, there is a correspondence
between closed subsets of X and Radon measures in
; such that the points of X coincide with the Dirac measures
which are invariant ergodic measures [8] . The Dirac measures define the point functionals (cf. [14] ). Because
,
,
a probability measure. Hence,
for any
, a Borel subset
, and
. The action of the Lie groupoid on the set of generalized points
is now considered.
Proposition 3.6 Given the Lie groupoid
, the set of generalized points
is a
-space.
Proof. The homeomorphism
, which is a continuous open map from the space
onto the unit space X, defines a left action of
on
, where
is the set of composable pair
. This means
with
. In other words,
is a left
-space if
. The action defines a groupoid equivalence on
. Given any pair
, we say that
if
which implies
. Since
in
are isomorphisms,
are composable pairs in
[15] .
This action of the Lie groupoid
on
is free and proper. Because
implies that
is a unit. It is proper also because the map
given by
is a proper map; that is, the inverse image of a compact set is compact. The two make
a principal
-space. Hence, the natural projection
onto the locally compact and Hausdorff orbit space
is an open map, where
means that the groupoid
has a left action on
[15] . □
Given that
is a left principal
-space, then
is the equivalence relation defined by the open map
(or
-action) on
. The equivalence classes are defined by having the same image in X. Since
acts by composition on
, we have
; that is,
is defined on
. Thus
is a space of equivalence classes or pairs in
on which a diagonal action of
is defined as follows:
Let
be the orbit space of the diagonal action. Then H has a natural groupoid structure with multiplication defined as
with
as the unit space. Thus,
is a groupoid which is denoted
, where
and
, for
.
Proposition 3.7 The groupoid of equivalence
defined by
-action on
defines a right action on the space
.
Proof. Given the derived groupoid
, where
is a continuous open map from the (locally) compact space
onto the unit space
, given as
. Thus, the quotient groupoid H defines a right action on
. We therefore have:
Thus, the action is given by composition
, where
is unique in
and satisfies
.
The action is well defined for given
, then there exists a unique
such that
and
. Hence, by definition
, and if the three
are same orbit then there must be a unique element of
such that
. This is given by
. It therefore follows that
□
Corollary 3.8 The left
-action
and right H-action
commute on
.
Proof. Given the right action
of the equivalence groupoid H on
, it follows that
is a right principal H-space. The left action
of
and the right action
of H commute
. The following diagram illustrates this commutativity of left
-action
and right H-action
on
.
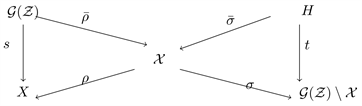
So, the action
induces a homeomorphism of
given as
. □
Theorem 3.9 The space of generalized or geometric points
is a
-equivalence.
Proof. The proof follows from the above. As we have seen,
and H are locally compact groupoids, and
is a (locally) compact space that is i) a left principal
-space, ii) a right principal H-space; and iii) the two actions commute; iv) the map
induces a bijection of
onto X, and v) the map
induces a bijection of
onto
.
From the construction, (iv) and (v) follow from the fact that if we have
, where
, then there exists a unique
such that
; the correspondence
is the desired isomorphism between
and H. Thus, the
-equivalence of
implies H is naturally isomorphic to
and
is naturally isomorphic to
. □
Remark 3.10 In [16] it was shown that every action of a Lie groupoid
on the arrows induces an action on the space of objects. So, the partial multiplication defined by
defines a self-action of the arrows which is reflected on the space of objects X and corresponds to elements in
of the compact set X by homomorphisms. The composition of elements of
form a unitary group which preserves the nets of Radon measures converging to ergodic measures or operators. Another formulation of the above as a gauge groupoid of a principal G-bundle is given in [12] . We will consider the Haar system of measures for the Lie groupoid next.
4. The Measure Groupoid and Measure Classes
Ergodic or measure groupoids act ergodically (or metric transitively) through the closed or invariant measure classes. The class
of a measure
is the set of all equivalent measures to
having the same null set. Every measure class contains a probability since any measure can be normalized on its support (cf. [13] ). Deitmar [17] showed the existence of Haar system of measures given the groupoid equivalence on X. Seda [18] showed that with a suitable separability condition on a groupoid
, each probability measure
uniquely determine a class of measure
on X for which it serves as integral of ‘translates’ of the Haar measure
on the structure or isotropy group
. These translates constitute a system of Haar measures and a measure class
defined on the fibres of
. Theorem 2.1 in [13] and the associated definitions form the background for treating the metric transitive nature of the measure classes.
The principal Lie groupoid
is analytic given that its Borel structure is analytic and the space
is countably separated. Given a probability measure
on the t-fibre
, an arrow
with
, and
, the map
defines a probability
on
. Since the product
is defined for
-almost all
, the support of
is
. Thus,
, which gives
.
Theorem 4.1 The principal groupoid
is the groupoid equivalence established on the normalized generalized space
by the action of the Lie groupoid
.
Proof. Two probabilities
for which there exists
, are said to be equivalent. This is the equivalence on geometric space
defined by the Lie groupoid
as the image
. Therefore, if
, then
if on the fibre
. This agrees with the induced action of the Borel group
on each measure class in
. Thus, isomorphisms on the fibres are constituted by invariant measure classes given as follows
Hence, the
-equivalence of the generalized points
is related to the action of the commutative algebra (or lattice)
on the generalized space
and represented by the measure groupoid
. □
There is always a symmetric quasi-invariant probability in a measure class. For according to Hahn in Theorem 2.1 of [13] , every probability measure in an invariant measure class C is quasi-invariant. Using his conditions, we strengthened the quasi-invariant condition for a Haar measure by modifying it to agree with a maximal ideal
or the corresponding zero-sets
decomposition of measures on X as follows.
Lemma 4.2 Let
be a measure groupoid,
a probability with t-decomposition
. There is a
-conull Borel set
such that
1)
if
.
2)
if
.
3)
.
4) if
, then
.
Given this modification, we now have that for every co-null Borel set
,
is a measure groupoid called an inessential reduction (i.r) of
in [13] , where the inessential reduction for an open conull subset
is also denoted as
.
From this, we see that each invariant measure class
form a system of Haar measures
for each
on the inessential reduction (i.r)
. Since each system is defined on the t-fibre
, it follows that the system of Haar measures is not unique. Hence, any invariant measure class C determines the measure groupoid
, with a Haar measure defined as follows.
Definition 4.3 Let
be a measure groupoid. Let
and let
be a probability on the base space. The pair
is called a Haar measure for
if
has a t-decomposition
with respect to
such that for some inessential reduction
of
, for all
and
a Borel function on
we have
(1)
Thus, for a Borel function F on the groupoid
, given a symmetric probability
with t-decomposition
, where
is conull as in the above; the quasi-invariance of
implies
With these constructions, Hahn showed that every measure groupoid has a measure
satisfying (1) for
in an inessential reduction.
Furthermore, the symmetricity of the probability
implies
. Hence, the quasi-invariance for right translation uses the s-decomposition
. The result is that the left and right invariance of C are equivalent. Using these, a
-decomposable measure for the composable space
is defined as
which is usually written
and is dependent on C. Since the space of composables of any groupoid has a goupoid structure, the conclusion is that
is a measure groupoid. The proofs of the following results
follow from 3.3 and 3.4 of [13] .
Lemma 4.4 If
is an analytic (standard) Borel groupoid,
is an analytic (standard) Borel groupoid.
Proposition 4.5 If
is an analytic groupoid with invariant measure class, so is
.
The concept of ergodicity will be delineated next and related to the dynamical system of the measure groupoid.
5. Convolution Algebra and Dynamical System
According to Hahn [13] , the measure groupoid is ergodic if and only if there is a single point
such that
is null. In other words, ergodicity implies the existence of Dirac probability measures
defined at each point of X. Thus, the existence of a
-action on
makes it ergodic groupoid. This also implies that every Borel function
on the base X can be expressed in the form of a positive Borel function F on the arrows
given as
, where F satisfies
. This means that the Borel functions on the arrows preserve the equivalence the groupoid
defines on the base space X. Alternatively, as stated above, the
-action preserves the Borel structure of the generalized space.
Subsequently, a real-valued Borel function F on the measure groupoid
satisfying
for
-a.e and for
-almost all
, corresponds to a Borel function
on X such that
a.e. Thus, the invariant functions on the equivalence space
(or on the space X with
-action) are of the form
or
. This shows the Borel functions are
-invariant.
Therefore, the dynamical system is related to the convergence of the ultra-filters
associated to each maximal ideal
. We can therefore define a net of such positive Borel function F on the arrows
given as
; or in the form
which can be considered local bisections. Since such a net
(or in terms of local bisections
) corresponds to the ultrafilter, it represents the dynamical system of the commutative algebra
. Following from 2.6 of [13] , the ergodic measure groupoid is therefore defined as follows.
Definition 5.1 The measure groupoid
is called ergodic if the only Borel functions
satisfying
are such that
-a.e. Alternatively,
is ergodic if and only if for all
,
or
.
If then we denote the space of all the Borel function on the measure groupoid
with
, the convolution product of two Borel function
on the space is defined as follows.
This follows from the involutive map on any Borel
which is defined as
. Thus, the space
is made into a normed
-algebra, with the norm of f given as the supremum norm
The representation of this convolution algebra
of the measure groupoid makes use of the modular function
which Peter Hahn defined and employed in Theorem 3.8 of [13] as
-a.e. homomorphism
.
Notice that
is a system of Haar measures supported on the fibres
; but given simply as
because they are same or (groupoid) equivalent measures. Thus, the Hilbert space
can be considered a bundle space made up of the fibres
. But because the Borel functions F defined on the arrows are equal to Borel functions
defined on X, having the net convergence
we described above, we put the Hilbert space simply as
.
6. Unitary Representation of
Given the convolution algebra
of Borel functions defined on the measure groupoid
, the formulation of the unitary representation of the convolution algebra
on the space
of bounded operators on the Hilbert space
is patterned on [13] , which is a simplified definition of von Neumann algebra arising from the maximal ideals
and ergodic action of the Borel group
on a compact measure space X. From the foregoing, the simplification is achieved by considering the system of Haar measures on the principal Lie groupoid
, and using them in the definition of the convolution algebras of Borel functions on measure groupoid
.
The definition of the convolution algebra of the principal groupoid
gives a
-algebra that coincides with the von Neumann algebra
, where
and
a probability measure on X. The following
result on
-representation of the resulting algebra is the focus of the paper.
Theorem 6.1 The map
is a unitary
-representation.
Proof. As in [13] , a representation is defined as follow. Given
and
; define a homomorphism
This defines an operator
by
such that
Thus, the map
is also in
, which makes
.
Likewise, we have the map
such that
Given the convolution product
; its image under T is given as follows.
Finally, from
we have
□
The operator is shown to be an isometry as follows.
Thus, the
-representation is a unitary representation since
. This is in conformity with our understanding of the Borel functions on the measure groupoid as probability measures on X or Haar system of measures on the groupoid.
Proposition 6.2 The convolution algebra
is a commutative von Neuman algebra by representation.
Proof. The Borel functions defined on the arrows of
are defined on the fibres
which contain the polynomials on X. So they are all defined on the operators on
as the representation showed. Hence, they are all of the form
, which makes them von Neumann algebra as defined in the opening section.
Alternatively, using Connes’ characterization of commutative von Neumann algebra in 1.3 of [3] as the algebras of operators on Hilbert space that are invariant under a group (or subgroup) of unitary operators, it follows that the convolution algebra
of the Lie groupoid
is a commutative von Neumann algebra since it is invariant under
as given by the condition of ergodicity
on F, which implies invariance under transformations of X.
A third characterization of a commutative von Neumann algebra by Connes [3] as an involutive algebra of operators that is closed under weak limits still reinforces the result. The presence of an ultra-filter
associated to every fibre
of the principal Lie groupoid
implies the convergence of
-invariant nets of Borel functions
in the normed
-algebra
(or equivalently the convergence of nets of local bisections
of the principal Lie groupoid
.) □
We have shown this to be related to the ergodicity of the measure groupoid
and central to the dynamical system of the algebra
which is now given as a corollary.
Corollary 6.3 The dynamical system of the commutative von Neumann algebra
is defined by the convergence of nets of operators
defined by the nets of Borel functions
or local bisections
.
Proof. This follows from the definition of the operators above as
That these nets define the dynamical system of the von Neumann algebra follows from their relationship to the convergence of nets of non-ergodic measures to ergodic limits which, as given in [8] , represents the dynamical system of ergodic actions. □
The action of the (lattice) commutative algebra
on the generalized space
also involves a decomposition. Thus, the resulting dynamical system converges to an ergodic limit given by
which is represented on the generalized space by
-invariant convergent nets of measures
, generalized by the
-equivalence of the geometric points
. This is given as a corollary.
Corollary 6.4 By the the polarity of the
-action there exists a canonical form
, such that
. So, the canonical form
converges to ergodic form
implies the action
converges to the ergodic limit
.
Proof. Given that the above convergent nets of measures can be constituted to be transversal to the orbits of
or the measure classes, then we have
, where
are the measure classes. Then
as stated. Hence, the steady ergodic state follows from the convergence of the transversal net(s)
, as constructed, to ergodic limits. □
The section
of measures is constituted from the measure classes. The existence of many measure classes for the
-representation points to the fact that the
-representation of the convolution algebra
of the measure groupoid is not uniquely tied to any measure class. In other words, the left Haar system of measures is not unique. The homomorphism of the
-representation implies that the convergence of a net of Borel function on the convolution algebra
implies a net of bounded unitary operators in the von Neumann algebra
.
7. Conclusions
We have presented the commutative algebra
-action on the generalized space
as constituted by the decomposition action of its maximal ideals
and the action of its group of units
on X given as a polar action of
on
, expressed in form of a principal Lie groupoid
action on the space
of geometric (or closed) points of the generalized space
. Ergodic requirements made it into the dynamical system defined by Borel functions on the ergodic or measure groupoid
.
The convolution algebra
of these Borel functions has a representation on the commutative von Neumann algebra
of operators on the Hilbert space
. Hence, the presentation of the geometric space
as a
-equivalence was helpful for the
-representation of the convolution algebra
of the principal Lie groupoid
or the measure groupoid
on the von Neumann algebra
of bounded operators on
.