1. Introduction
Our intention of discussing how quantifier none relates to the concept of “zero” is to shed light on the semantics of natural language and in particular in the way the English language expresses the concept of zero or “nothingness”.
We intend to carry on such a discussion by first, having a look at the history of zero and its development throughout time, in order to be acquainted with “what zero is”; we will proceed not only with defining zero as the numeral 0, or associating it with “nothingness”, or the empty set but also with making the close connection of zero to linguistic expressions that are responsible in defining “zeroness” in language, as it appears in syntactic constructions with English negative quantifier none. The notion of “nothing” is also very essential to our findings. In our attempt to initiate an explanation of what “nothing” is, we quote Barton (2020) .
Barrow (2000) speculates on what is “nothing”:
“If one looks at the special problems that were the mains prings of progress along the oldest and most persistent lines of human inquiry, then one finds Nothing, suitably disguised as something, never far from the center of things. Nothing, in its various guises, has been a subject of enduring fascination for millennia. Philosophers struggled to grasp it, while mystics dreamed, they could imagine it; scientists strove to create it; astronomers searched in vain to locate it; and mathematicians succeeded. Meanwhile, writers and jesters were happy to stir up as much ado about Nothing as ever they possibly could. Along these pathways to the truth Nothing has emerged as an unexpected pivotal something, upon which so many of our central questions are delicately poised.” (Barrow, 2000: p. xi) .
2. Our Goals
We intend to describe how the English language expresses “zeroness” by using the quantifier none and add to the knowledge we already have about the usage of a natural language like English in everyday life. The concept of zero is a fundamental concept in mathematics which appears in natural language in the form of numerals and quantifiers. The way natural languages choose to express this concept varies; for instance, natural languages like Albanian, Arabic, French and Greek permit a singular construction with the negative quantifier, while Somali favors a plural construction. The result is the same: an attempt to express “nothingness” in natural language.
The question we ask is “why does English allow two different syntactic constructions with quantifier none, while other natural languages permit mainly one, either singular or plural”. This choice English allows is more intriguing and for this, our research concentrates on the English language and intends to offer information on the way “zeroness” is perceived and expressed linguistically in English with the use of quantifier none.
In addition, a minimal profile of zero will help us understand what “zero” stands for and will familiarize us with some important historical facts that concern “zero” as a cardinal or as a concept, examined from a mathematical and philosophical perspective.
However, the primary concern of this work is to discuss the linguistic performance of “zero” in the form of English quantifier none as it appears in specific syntactic constructions that accept either a singular or a plural verb. We believe that English native speakers are often faced with the dilemma of choosing singular or plural constructions depending on their personal understanding of “zero” or “nothingness”, expressed in quantifier none.
Our intention is to add new findings to the current research in Semantics: the science of reasoning, and to further investigations regarding the structure and meaning of specific quantificational expressions, concentrating on the English language.
3. The English Quantifier None
A considerable time ago, McCawley (1995) divided quantifiers in a tripartite relation of 3 sets which correspond to the following classification (see Giannakidou, 2004: p. 216 ), repeated below:
Among other quantifiers, none has been placed among those which denote negation such as nobody, never, neither, nothing and nowhere.
None is a quantifier classified as a “totalized negative” (Sapir, 1930: p. 22; Giannakidou, 2004: p. 221) . None is the opposite of all but not the opposite of some as it appears that some could refer to not all but not to none. Horn (1972) explains in his table (53) quoted below, how the “duals (all/some; none/not all) represent the weakest and the strongest values on their respective scale, the weakest situated just above +/−0 and the strongest +/−1. Only the strongest value can be modified by absolute(ly) and its synonyms (Horn, 1972: paragraph 2: 34) : cf. Absolutely {all/none/#many/#few} of them can make it; It’s absolutely {certain/impossible/#possible/#unlikely} that he’ll win.” (Giannakidou, 2004: p. 237)

Giannakidou (2004) paints the portrait of none as a totalized negative, so that in sentences like: The girl left none of the apples would imply that it is a possibility that The girl ate all the apples. The desired message conveyed would be that there were no apples left; none at all.
Katsos et al. (2016) describe none as a negative, monotone decreasing quantifier that licenses inferences to subsets (e.g., None of the students are playing football in the rain)” (Katsos et al., 2016: p. 2) opposite to all seen as “a positive and monotone increasing quantifier that licenses inferences to supersets (e.g., All of the students are playing a sport)” (ibid). In terms of truth conditions, Katsos et al. (2016) state that:
1) a) “All of the As are Bs” is true iff A
B = A
b) “Some of the As are Bs” is true iff A
B ≠ ∅
c) “None of the As are Bs” is true iff A
B = ∅
d) “Most of the As are Bs” is true iff |A
B| > |A − B|
e) “Some of the As are not Bs” is true iff A − B ≠ ∅ (Katsos et al., 2016: p. 2) .
We are concerned with Katsos’ et al. example (1c) that states that it is true, if A
B result in the empty set ∅; for the time being, we keep this in mind.
Kocab et al. (2022) give a general view by describing negative quantifier none along with universal all and existential some as the classical quantifiers which express relationships between sets, and appear in most human languages.
In general, none is considered to be a universal negative, symbolized as ∀.
None indicates negation and means “not one” or “not any”.
It has already been decided for us that this quantifier denotes negation, as opposed to universal all and existential some. We do not intend to turn the tables in setting a new profile for none. Our intention is to connect none semantically to the concept of “zero” and show how language deals with both by using specific linguistic expressions that define “nothingness”. We do not wish to establish a new semantic classification for none, but to shed light to a peculiarity particular to the semantics of none that manifests itself in the grammatical agreement this quantifier favors between the number of the noun and the number of the verb when it appears in sentences like:
2) None is late
3) None are late
This allows both a singular and plural interpretation of “zero” in the form of quantifier none, which is the focus of this research.
The Concise Oxford English Dictionary (2002: p. 969) provides the meaning and origins of none; it defines none as a pronoun that means not any or no person; it adds that it also means no one or not at all and its origin is found in Old English na˜n, from ne “not” + ãn “one” of Germanic origin.
The Collins COBUILD (2003: p. 8170) adds more information by stating that none of something means not even a small amount of it. None of a group of people or things means not even one of them.
None in the English Grammar
The Concise Oxford English Dictionary, regarding the usage of none, clarifies that:
“(S)ome people insist that none can only take a singular verb, never a plural verb: none of them is coming tonight rather than none of them are coming tonight. There is little justification for this view: none has been used for around a thousand years with both a singular and a plural verb, depending on the context and the emphasis needed.” (Concise Oxford English Dictionary, 2002: p. 972)
In English grammar the indefinite pronoun none can be engaged in syntactic structures where the verb is either singular or plural as it appears in sentences (1) and (2) mentioned above.
None in the English grammar is used as the antonym of universal all or the numeric one. Therefore, it should be expected that if we were to ask a native speaker of English to negate a sentence like the following:
4) a) All the students came late
b) One student came late he should reply (5):
5) None of the students came late
However, in comparing (3) to (4a) after having used the third, singular of the present tense, another difference appears regarding the number of the verb which relates to the semantics of quantifiers all and none. The difference is more prominent with the usage of verb to be.
As we well know, in English there is a subject-verb agreement in person and number, which is demonstrated in sentences (6) and (7) below, in which plural countable noun students in (6) agrees in number with plural are, both being under the semantic shade of universal quantifier all; however, in (7) the plural noun students, does not agree in number with singular is, being this time under the semantic influence of quantifier none:
6) All the students are late
7) None (of the students) is late
Another possibility could be the structure given in (8) below:
8) None (of the students) are late
Both (7) and (8) are equally accepted after quoting what the Concise Oxford English Dictionary has to say about this case. The two possibilities have been mentioned above.
In addition, the Oxford Advanced Learner’s Dictionary (2003: p. 862) gives examples like those mentioned below, regarding this peculiarity in the English grammar:
9) None of these pens works/work
10) We have three sons but none of them lives/live nearby
As expected, examples (9) and (10) demonstrate this difference.
We intend to concentrate on examples which include countable nouns engaged in a partitive with quantifier none and we will abstain from including in our discussion mass and/or uncountable nouns, as the former as more intriguing examples than the latter.
According to the English grammar when none quantifies over a singular or a mass noun only singular agreement is acceptable but when none quantifies over a plural noun, both singular and plural agreements are also acceptable.
Our intension is to explain the semantics behind such grammatical choice English native speakers must make when they use quantifier none as in sentences (7) and (8).
In our explanation we intend to consider the set theory and the semantics of “zero” in order to shed light to such a choice.
4. History of Zero
It took centuries for the concept of zero to develop into a number for counting or calculating, even though the concept of nothing has always existed!
Most of the ancient nations have contributed to the efforts of understanding zero. Mayan nik, Hindu sunya, Arabic sifr, Indian kha, Latin nulla figura or nulla, or nihil, Greek miden, Hebrew sifra, Italian zefiro, zefro or zerero, French chiffre and German ziffer, all bear testimony to the common effort nations put in defining zero. It would only be fair to say that the end result was a shared contribution of the world to the world!
The birth of zero goes back to the Babylonians, the Greeks and the Indians. The Babylonians used two slanted wedges to represent zero and the empty space, or used as a placeholder to gift the world the written representations of 10, 20, 100, 2000, 300,000, etc., with a permanent meaning world widely accepted.
Initially, zero appeared as a digit not a number because it was not perceived as a number with a specific value. Zero had no place in the sequence of numbers, but it was a symbol; it was the first attempt of symbolizing “nothingness”.
The world was divided in the way it perceived zero; the Mayans had a zero in their counting system and started numbering the days of the year with the number zero and that was implemented into the Mayan calendar. However, the Greeks and the Egyptians had no zero yet in their counting system and that showed in the way they numbered the months and the days in a year starting with 1 (see Seife, 2000 ).
The first time zero appeared as “0” was in the Greek astronomical papyri of the third century BC, and it was probably the Greeks under Alexander the Great, who discovered how important zero was to counting. “The commonest explanation is that “0” came from the Greek omicron, the first letter of ουδεν, ouden: ‘nothing’” (Kaplan, 1999: p. 18) and ουδεν became μηδεν, which is what zero is called in Greek till the present. According to Kaplan (1999) the Greeks are responsible for naming what we know as zero today and developing counting with zero.
The reason of zero’s existence is to define “nothing” and to count “nothing”. Other nations prior and later to the Greeks also felt the need to define nothingness by providing a symbol for zero and defining the notion of zero such as the Mayans and the Indians. The road to the discovery of zero is long and complicated for the Mayans and we will refrain from discussing it, because it hasn’t much to offer to this research. The Indians, on the other hand, discussed zero by referring to “empty” astronomical places; therefore, “kha” was the name used for “words for spaces, like ambara (sky) and akasa (atmosphere), and sunya, usually translated ‘empty’, which soon became zero’s commonest name” (Kaplan, 1999: p. 46) used to mark the absence of something. It appears that the semantic similarities between “empty” and “zero” have been noticed from ancient times.
Later on, the Romans provided one of the commonest words for zero: “null”, which comes from the medieval Latin nulla figura, “no number”. Kaplan (1999) mentions a popular view in the fifteenth century: “Just as the rag doll wanted to be an eagle, the donkey a lion and the monkey a queen, the zero put on airs and pretended to be a digit” (Kaplan, 1999: p. 68) ; words which describe the struggle of zero to come to existence and acceptance.
All this, just to give a taste of the history of the existence or the non-existence of zero throughout time, “Zeroness” is not an easy notion to comprehend or to describe; perceiving it becomes even more complex. Perhaps, this is the reason why it took the human race centuries to define zero, and similarly it takes years before a child comprehends and starts using zero, as we will see in our later discussion about the concept of “zero”.
5. Zero in Mathematics and Philosophy
Zero has been the center of the attention of Mathematics and Philosophy. In terms of Mathematics, Barton (2020) describes zero being a cardinal number; but in terms of Philosophy, he links it to ideas of “nothingness” and “non-being”.
As a number zero can be seen as an identity element since for any number n, n + 0 = n, but in philosophy zero overlaps with the status of “non-being”, the absence of something. Zero is a collection-size property just like the rest of the numbers because it demonstrates cardinality like any other numeral and therefore, in Mathematics, zero is seen as a cardinal number. But regarding Philosophy, Barton concludes that zero represents “nothingness” by being instantiated by empty collections and claims that “the cognition of zero can be understood as a species of absence perception” (Barton, 2020: p. 3825) . In terms of epistemology, Barton believes that zero can have a numerical cognition, an understanding of counting procedures, uses of algebraic rules and be described as other numbers; this allows “zero” to be classified as a number like all other numerals.
The road to discovering zero was long. Understanding zero was one thing and defining it was another. Indian mathematicians were occupied with defining zero; “Brahmagupta in 600 AD can on the one hand say quite concisely that any number minus itself is zero; on the other, he struggles toward generality when it comes to adding zero to a number: ‘The sum of cipher and negative is negative; of affirmative and nought is positive; of two ciphers is cipher’” (Kaplan, 1999: p. 71) and sets out the rules of subtraction as “negative taken from cipher becomes positive; and affirmative, becomes negative; negative, less cipher, is negative; positive is positive; cipher, nought.” (ibid)
Five centuries after 600 AD, Bhaskara added: “In the addition of cipher or subtraction of it, the quantity, positive or negative, remain the same. But subtracted from cipher, it is reversed.” (ibid)
Mahavira continued working on explaining zero and added that “a number multiplied by zero is zero, and that number remains unchanged when it is… diminished by zero” (Kaplan, 1999: p. 72) . Mahavira also added that “(A) number remains unchanged when it is divided by zero.” (ibid)
Today the Concise Oxford English Dictionary (2002: p. 1663) defines zero as “a cardinal number (pl. zeros) the figure 0; nought; nothing-appoint on a scale or instrument from which a positive or negative quantity is reckoned. -a temperature of 0˚C (32˚F), marking the freezing point of water - the lowest possible amount or level; nothing at all. -informal a worthless or insignificant person” (Concise Oxford, 2002: p. 1678) .
In modern days Mathematics, zero can be thought as a property of collections instantiated by empty collections and this is how we identify zero with the empty set, ∅, a set with no members at all.
Barton (2020) says that “the empty set is the representative for 0” (Barton, 2020: p. 3829) and identifies as its cardinality the same way, for instance, the numeral 3 is the cardinality of a set of 3 members. This thinking, explains Barton (2020) , allows us to consider 0 as a property corresponding to collection-size just like the other natural numbers, and this allows zero to be in the same ontological category of the other numerals.
Zero can be identified with the empty set which means that “if you have zero of a certain kind of object you do not have any such objects at all” (Barton, 2020: p. 3827) and that is how zero interacts with “nothingness”. This appears in the way 0 undergoes addition, subtraction, multiplication or division, so that any number that is added, subtracted, multiplied or divided by 0 gives the same number, unless 0 is added or subtracted or multiplied or divided by 0 to yield 0. In contrast, this does not happen with other cardinal numbers because they carry with them a specified cardinality; for instance, 4 represents 4 individual objects, 7 represents 7 individual objects and so forth.
Barton (2020) explains that “no matter how many times you repeat an absence, you will never generate positive magnitude if you take an absence of instances of a particular number (i.e., zero positions are absorbing elements under multiplication)” (Barton, 2020: p. 3833) .
As a cardinal number, zero in today’s Mathematics, is symbolized as “0” and in counting it appears between −1 and 1, in a sequence like −1, 0, 1.
Seife (2000) discusses the uniqueness of zero and says that any number changes if you add a number to it but zero + zero + zero gives zero. Seife (2000) states that zero refuses to get bigger. It also refuses to make any other number bigger. Add two and zero and you get two; … The same thing happens with subtraction. Take zero away from two and you get two. Zero has no substance. Yet, this “substanceless” number threatens to undermine the simplest operations in mathematics, like multiplication and division. … Anything times zero is zero, …, zero times anything must be zero. Division by zero should be the opposite of multiplying by zero.
Subtraction helps us understand how zero functions in Mathematics; as an example, Kaplan (1999) uses the following scenario: “four people are in a room and seven people leave it. How many people must go in before the room is empty? Answer: three” (Kaplan, 1999: p. 69) .
We have accepted zero as a cardinal; Bylinina and Nouwen (2018) explain the nature of zero by treating it as a numeral and not a quantifier like no. The reasons for that conclusion are first, because zero can take a cardinality of 0 like a number like 3 can take cardinality 3 and second, because of its syntactic structures that follow those of numerals as in sentences like, Zero students bought cakes and 3 students bought cakes.
We already mention Barton’s (2020) view that in Philosophy, zero represents “nothingness” or the “absence of something”. Zero can be identified with the empty set which means that “if you have zero of a certain kind of object you do not have any such objects at all” (Barton, 2020: p. 3827) and that is precisely how zero interacts with “nothingness”.
Barton argues that “the cognition of instances of zero are linked to absence perception” (Barton, 2020: p. 3839) and explains that in our effort to understand zero, what happens is that “we understand the experience not as cognition of number but also perception of an absence” (Barton, 2020: p. 3850) . He explains that zero perceived as the “absence of something”, is similar to silence being perceived as the absence of sound, philosophically speaking.
Barton’s (2020) view triggers questions like “What is the absence of something?” “Is it the nonexistence of something?” and “Do we really need to have an entity of something in order to understand and perceive ‘nothing’?” Such questions will be answered as we proceed in our discussion.
6. Zero in Linguistics
So far, we have seen a mathematical zero referring to no quantity, or no number or size. As a concept, zero refers to “nothingness”. Generally speaking, zero appears as a number “0” that represents an empty quantity of a 0 value, that relates to “nothingness”. Zero marks the absence of an entity, the lack of something. Zero students means that there are no students at all; the set of students is empty with no members in it to define it as a set, that is an empty set of zero members. As a concept, zero refers to “nothingness” or “zeroness” as we prefer to call it.
Zero did not only occupy the minds of both mathematicians and philosophers but it also triggered linguistic discussions.
Zero appears in the English language in a morphological form of “∅ morpheme” as in the case of ∅ plural suffix in irregular nouns like sheep and deer, as in “sheep +∅” and “deer + ∅”.
Zero also appears in English phonology as a “∅ phoneme” in examples like the word psychology where the phoneme /p/ is not pronounced.
Similarly, zero appears in English syntax in examples of ellipsis such as I would love to go but I can’t ∅, instead of I would love to go but I can’t go.
It seems that zero ∅ is also manifested in Linguistics and through such manifestations, stands for the “absence of something” in various aspects of the language. Therefore, zero appears as the “existence of nothing”.
7. Hartmann et al. (2022) and Katsos et al. (2016) on the Perception of Zero and Negative Quantifiers
Zero is a concept that is taught to us from a very young age, when we start struggling with counting, addition and subtraction. Even when we are learning our “time tables” the first calculation we come across is with zero as in 1 × 0 = 0 or 2 × 0 = 0, etc.
The interesting thing, however, is that when children learn how to count, they start from 1, but never from 0 (cf. Seife, 2000 ).
Zero is learnt as a cardinal that has the value 0, just as we learn other numerals and their cardinal values; zero relates to our ability to connect numerals and their magnitude so that, we learn to count from 1 to 10. Barton (2020) points out that we learnt what zero stands for by associating “numerals and magnitudes as represented by the ANS correlates with counting competence” (Barton, 2020: p. 3837) . He continues by referring to Wellman and Miller’s (1986) experiment with 57 preschoolers of 3 - 7 years of age and states: “(G)iven then the realization that the successor function has an inverse (i.e., that one can also subtract 1 as well as add 1 to yield the predecessor instead of the successor) we might then consider what the predecessor of 1 might be, and realize that it must be zero (since 1 − 1 = 0).” (ibid). As a result of the experiment, it shows that children go through 3 stages to be able to understand zero; first they “acquire the ability to name the written numeral”… then “they are able to associate this numeral with nothing…” and third, “they are able to integrate zero into relationships with other numbers” (Barton, 2020: p. 3850) . After this, children become able to process zero into their thinking as “nothingness” in terms of counting or by using the quantifier none, linguistically speaking.
Hartmann et al. (2022) also discusses how very young children learn the concept of “zero”; they state that after monitoring the progress children make while learning the cardinal numbers, there is no evidence about the way zero is integrated into their minds. “The mathematical term “zero”, is not in the common vocabulary of young infancy” (Hartmann et al., 2022: p. 355) . Children take time to acquire what “zero” stands for along with words and number names. Young children “rely on counting to form concepts of natural number” (Hartmann et al., 2022: p. 354) ; “counting helps children to get a better insight into ordinal and cardinal aspects of natural number” (ibid).
Children begin to acquire the concept of “zero” after they have learned some number words, at least 1 - 4. They perceive numbers as gradually increasing quantities and tend to perceive 0 as smaller than 1. In order to understand the concept of “zero”, children need to refer to “no entities” and create a concept for the lack of something.
After their investigation, Hartmann et al. have concluded that “zero does not seem to be a “natural number”, if “natural” is determined the way, that the relation of the number word, its magnitude and its visible reference can be mapped onto each other.” (Hartmann et al., 2022: p. 359) ; “children were more likely to locate zero within the number word sequence than to give zero items” (ibid).
Hartmann et al. (2022) found out that the problem in understanding zero is “to find a suitable place in the number line for the number 0. Semantic terms that express nothing are helpful here because they indicate that zero is even smaller than one. Comparisons of all kinds of linguistic expressions for empty sets with one or more objects lead children to place zero still before one. Perhaps children first need to understand the successor and predecessor functions to develop an understanding that zero is the predecessor of one” (Hartmann et al., 2022: p. 360) . Children found the understanding of the concept of zero slightly harder than the counting knowledge of four. This might lead to the conclusion that “the representation of zero may be tried to its ordinal position rather than to the very abstract cardinal representation of an empty set” (ibid) and again we repeat that their findings state that young children comprehend the concept of zero and “nothingness” after they have learnt counting. Wellman and Miller (1986) found out that “children could name the symbol “0” around the fourth birthday and that children six years of age could describe zero as being the smallest number and could compare numbers” (Hartmann et al., 2022: p. 355) . Perhaps a reason for this inability to comprehend zero at an earlier stage could be that “(S)poken language usually does not refer to empty sets as being zero but uses a variety of different words or phrases to describe the characteristics of an empty set. Zero is characterized as referred to as no apples, nothing to eat, empty glass, vacant chairs, blank spaces.” (Hartmann et al., 2022: p. 355) ; “zero is usually referred to semantically indirect references, e.g., ‘no’, ‘empty’ or ‘nothing’” (ibid).
From my own experience, I remember that very young children learn to use the English phrase “all gone” in order to express “nothingness” or the absence of an entity.
Katsos et al. (2016) intend to shed light to the way we acquire quantifiers. They claim that “learners of most languages are faced with the task of acquiring words to talk about number and quantity” (Katsos et al., 2016: p. 1) . “Number words and quantifiers are abstract words that denote properties of sets rather than individuals. Two-ness and all-ness in “two/all of the black cats in the street’ are not true of any individual cat, while black-ness and catness are” (Katsos et al., 2016: p. 1) .
Children display knowledge of number words and quantifiers around their second birthday, comparatively long after they have acquired concrete nouns (1, 2) or as a provider of ones for acquisition (6, 7, 8). For example, “children learning languages that distinguish between singular and plural or between singular, dual and plural morphology, learn the meaning of ‘one’ and ‘two’ respectively earlier than children learning languages that do not” (Katsos et al., 2016: p. 2) .
Modern English is limited to singular and plural. Katsos et al. (2016) also state that “quantifiers (e.g., none, some, all) too are properties of (or relations between) sets. The onset of the acquisition of quantifiers coincides with the acquisition of number words …” (ibid).
8. How Our Minds Reason Reflects the Way We Think and Speak
Knauff (2007) explains how our brains reason logically by discussing inferences based on quantifiers such as all, some and none. He discusses what he calls “one of the oldest questions related to logically reasoning and clarifies that “our ability to reason logically relies on ‘seeing with the inner eye’” (Knauff, 2007: p. 20) and asks “do we think logically by visualizing ‘mental pictures’ in the ‘mind’s eye’ and ‘look’ at these pictures to find new, not explicitly given information?” (ibid).
“How well a formal inference fits with our background knowledge is only one aspect of the content of a reasoning problem.”… “Another aspect is how easy it is to visualize the matter of the problem. Cognitive psychologists and psychological laymen often think that we reason by using ‘mental pictures’ in the ‘mind’s eye’ and that we can ‘look’ at these pictures to find new, not explicitly given information.” (Knauff, 2007: p. 30)
Knauff (2007) gives his own understanding on this thinking. He claims that “the results mainly focus on relational reasoning as these inferences, if any, have the strongest link to what we call a visual mental image of many psychological definitions of visual mental imagery I prefer to define it as the inspection and manipulation of visual information that comes not from perception, but from memory, or from another non-visual external stimulus, such as the sentential premises of a logical problem.” (ibid)
What he understands is in agreement with our thinking in connecting quantifier none with the concept of “zero”; he believes that language interferes with reasoning and allows us to identify what the problem in question is. Therefore, if we are questioning the empty set or zero or nothingness, the linguistic expression none allows us to visualize a set of no members after we have used the mental image of a set full of members and have visualized the same set with no members at all so that language and brain reasoning overlap in results.
For instance, in English syntax how can we talk about “indefinites” if we haven’t defined what “definites” are? How can we define “no apples” if we don’t define “apples” in, let us say, a basket full of apples? Similarly, we can talk about “nothing” when we have at least visualized “something”, which would be negated or made into “nothing”.
In order to define what it doesn’t exist we need first to define what exists!
For instance, there is a set of “students” and a set of “people being late”. The intersection of the two sets result in the set of “students who are late”. It is the case that not even one member of the set (or zero members of the set) intersects with the set of people being late in order to define None is/are late. And we use this to explain the meaning of a sentence like None (of the students) is/are late.
Knauff (2007) concludes that “Sometimes the way we reason is logically analogous to the proof of formal logic, sometimes we think logically by using models in the strict logical sense, and sometimes we use mental images.” (Knauff, 2007: p. 33) He goes on to explain that the way “mental logical reasoning is implemented in the human brain is a question of formal reasoning.
“If … the right hemisphere is involved in ‘abstract reasoning’, then this hemisphere, if any, is the more ‘basic’ for reasoning.” (ibid) and “(I)t seems to be responsible for all operations that are compatible with what, according to most logicians, logic is about. The left hemisphere, in contrast, is ‘only’ occupied with the processing of ‘content’, that, according to most logicians, logic is about. From this perspective the language-based system corresponds to more knowledge, based processing while the parietal model-based system corresponds to the ‘logical’ system.” (ibid)
Knauff states that according to experiments described in his (2007) paper, it has been shown that the way the brain functions is “the parietal corties are involved in reasoning and that reasoners indeed construct integrated representations of the premises and inspect these representations in order to find new information that is not explicitly given in the premises.” (Knauff, 2007: p. 34)
Another proof for our thinking in understanding the concept of “zero” and incorporating it into our language in the form of negative quantifier none also comes from Vetulani (1988) .
Vetulani (1988) explains how our brain processes the meaning of what he calls null quantifiers, that is quantifiers which express the null quantity.
According to Vetulani, a sentence like *zero uczniow biegnie in Polish and *zero élève [s] cour[en]t in French, are not grammatically correct in either of the two languages but could be said with the use of Polish “zaden” and French “aucun”: the Polish construction would be “Zaden uczen nie biegnie” meaning the French “Aucun élève ne court”, which translates to English No student is not running; this according to Vetulani (1988: p. 52) means “x uczen nie biegnie” and French “x élève ne court [pas]”.
Vetulani takes evidence from Polish “zaden” and French “aucun” which combine with a verb in the negative; he says that “the very words “zaden” and “aucun” do not express absence, but have to be considered (similarly to “kazdy” and “chaque”) forms of the universal quantifier in the context of the negative predicate. The use of these words in the context of negation is the source of the common error of considering them as null quantifiers…” (Vetulani, 1988: p. 53) He continues by saying that “(T)he general statement we may derive from this set is that in Polish, French, and … many other languages, one must apply more descriptive methods … to express lack than to express other quantities. Polish has no null quantifier and its lack is expressed analytically like in French where we maintain the verb in its negative form” (Vetulani, 1988: p. 56) .
English has null quantifiers no, none, no one, etc.; however, we are trying to explain how the mind comprehends the semantics of none and our thinking follows Vetulani’s explanation of how languages without null quantifiers work in expressing the lack or the absence of something by visualizing the something that does not exist and negating it. He states “humans record facts with the help of senses. To achieve this, they have to perceive and identify objects. To be able to affect this, they have to dispose of abstract mental representations of objects, composed of (among other things) sets of features which make identification possible.
A statement of the lack of something, however, requires the ability to imagine some hypothetical situation of reference and the ability to compare that one perceives with what one imagines. The ability just mentioned is undoubtedly more complex, hence it requires higher intellectual efficiency than does simple conscious perception. This is reflected in language in the following way: “one usually uses simpler linguistic tools (affirmative sentences) to record the result of a positive observation (that is, to describe the relation holding between two objects) than one uses to present the lack of objects with the required property (analytic constructions with negation).” (Vetulani 1988: p. 57)
Vetulani (1988) also quotes “Piaget’s claims that perception is often conditioned by the conceptualization of the observant entities (which requires well developed mental ability)” (Vetulani, 1988: p. 58) . This falls in the exact line of our thinking trying to explain how the concept of zero is mentally processed in the way we understand the quantifier none and express the concept of zero by using such a linguistic expression. Basically, our brain visualizes a set of entities, full of members according to the sentence in question; then, our brain uses negation to subtract either the entire set of each of its members separately till the result is an empty set. Piaget explains that such a process requires a well-developed mental ability and that is the reason why young children cannot process the concept of “zero” before they have learnt to count and use subtraction, according to Wellman and Miller (1986) .
9. Our Thinking
In this paper, we are preoccupied with constructions English quantifier favors which, unlike other natural languages, permits both a singular and a plural syntactic construction as in our default examples: None is late and None are late.
The Concise Oxford Dictionary makes acceptable both the singular and the plural syntactic construction with English quantifier none. We attempt to explain this, by considering first, how children learn “zeroness”, secondly how our mind processes “zeroness” and third, how “zeroness” is expressed in language.
The quantifier none relates to zero; zero might be more of a mathematical number but it also refers to the philosophical concept of “nothingness” or the “absence of something” according to Barton (2020) . In order to understand the “absence of something” we need first to understand the “existence of something”.
None, on the other hand, is a quantifier which also refers to nothing or the empty set (Katsos et al., 2016) from a linguistic point of view.
Therefore, there is a similarity in the meaning of none and that of zero.
Mathematically speaking, zero is a cardinal with 0 value which means that it has no value at all; zero is also the result of subtractions like 1 – 1 – 1 − 1 = 0 and the like; its mathematical profile has already been reviewed in Section 4.
Knauff (2007) explains that in logical reasoning “our ability to reason relies on “seeing with the inner eye” (Knauff, 2007: p. 20) . He asks if we think logically by visualizing mental pictures in the mind’s eye and look at these pictures to find new, not explicitly given information. He answers in the affirmative when he claims that we manipulate visual information that comes from memory and not from perception.
Based on this previous discussion this is how we explain why English native speakers are free to choose either singular or plural syntactic construction with none. We believe it comes down to the speaker’s personal choice based on his own understanding of “zeroness”, that is whether he understands 0 as the result of a subtraction or as a number of zero value of a collection-size property like all other numbers. Let us elaborate on this more. Zero relates to the empty set since both are of “no members”.
For our mind to comprehend none as the empty set, it is important to understand and visualize a set full of members. To be more explicit, for our mind to understand “none” it needs to visualize and understand “something” or “someone”. Let us say in order to understand none of the students, our mind needs to visualize and understand what it means to have a set of, let’s say, 5 students: Peter, Paul, John, Marc and Luke.
This is our scenario: I am a Semantics instructor and I teach a class of 5 students in room 101. Every day I take attendance but unfortunately today I came to find an empty classroom, which means that I found room 101 with none of my students present; room 101 is empty. Therefore, I used the sentence none (of the students) is present to describe the situation. This means that while I looked around, I realized that “Peter is not present”; “Paul is not present”; “John is not present”; “Marc is not present” and “Luke is not present”. My mind is already familiar with the set of these 5 students being present and because of this familiarity my mind allows me to visualize the entire set with all its 5 members. In my mind I perform a mental subtraction so that I subtract each one of the students from the entire set; I observe that Peter is not present; Paul is not present; John is not present; Marc is not present and Luke is not present. With Luke not being present I end up with my set full of students transforming into the empty set with no members at all, nothing, zip, null, zero! This allows us to perform the mental subtraction: 5 − 1 = 4 − 1 = 3 − 1 = 2 − 1 = 1 − 1 = 0.
What we are left with after such a mental calculation is the result of the subtraction 1 – 1 – 1 – 1 − 1 = 0 that took place in our mind to process none.
We could use the following Venn’s diagrams to describe what we have visualized with our inner eye as we process this scene at a classroom empty of students. This is demonstrated in diagram (A) below, in which each circle represents one of the 5 students: Peter, Paul, John, Marc and Luke. The result is an empty circle, i.e., a circle with no members.
(A) 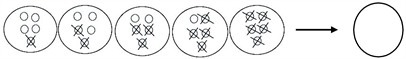
This thinking of explaining how we perceive quantifier none or the empty set, also explains why children cannot use words like none before they comprehend zero or before they learn how to count at least from 1 - 4 (cf. Wellman & Miller, 1986 ). Children need to allow their mind to visualize this kind of mental subtraction that goes on in our mind to be able to use a sentence like None (of the students) is present. At a young pre-school age children learn to use the linguistic expression “all gone” to refer to zero or the empty set and the way this is done is by having a set of objects in front of them and the absence of this set would equal the expression “all gone”. In our adult usage of language, this absence would be expressed with the usage of quantifier none.
On the other hand, we have talked about zero as a cardinal of “0” value which has a collection size property like number 5, let us say. If we visualize the set of 5 students and we are also familiar with zero being a cardinal of no value at all, then our mind is quick in processing this as the result of a set empty of students so that the entire group are not present. Plural means that we see it as a whole, it relates to collectivity as, for instance, Arabic word shajar “tree” is “indifferent to the number of individual trees but specifies that they are of one type” (Cruse, 2006: p. 129) ; therefore, zero is perceived as the collectivity of no members of the set or zero which has a value “0”. My 5 students when absent become a collective 0.
This result is a set empty of students so that all the students are not present; and therefore None (of the students) are present.
(B) 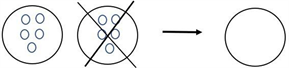
The empty set would be represented as a classroom empty of students.
Barton (2020) uses an example to explain how our mind perceives zero or nothingness; he says imagine that you are hungry and you have packed some sandwiches for lunch. Maybe 3 or 4. “You open the hamper to find… no sandwiches! (I secretly ate them all on the bus and then lied.) Might we argue that in this case that you experience an instantiation of zero-cardinality of the collection in question? This would then yield the beginnings of an epistemology of zero…” (Barton, 2020: p. 3836)
Vetulani’s (1988) view also contributes in our explanation of how the mind processes the semantics of none in order to express the lack of something by visualizing something that doesn’t exist, and negates it. He explains that humans need to depend on their senses to identify objects and visualize abstract mental representations of these objects.
In our scenario, we need to be able to identify the set of 5 students that attend my Semantics class and me, being their teacher, I am able to do so because I already have the past experience of a full class with all 5 students being present, whom I know by name. Then, once I have the experience of a full class, I can process the “existence of something” that is a full class with all 5 students. Once I am familiar with a class full of students, I can easily process the desirable result of what it means to have “None of the students present”, i.e., “the lack of this something” or the “absence of this something” which means a classroom empty of students, or the room 101 with zero students being present.
Vetulani (1988) quotes Piajet’s claims that perception is often conditioned by the conceptualization of the observant entities, which requires well developed mental ability. This explains why very young children who have not yet mastered their ability to capture concepts and familiarize with them in order to mentally process them. Therefore, if children are not able to understand the magnitude of cardinals and the additions, subtractions, multiplications or divisions we perform with numerals, how would they be able to understand not having any of those which results to the meaning of zero, expressed in language with the help of negative quantifiers?
Our mental growth and ability to count and calculate teaches us gradually what “zeroness” stands for. This appears not only in counting and using mathematics but also making use of linguistic expressions of “nothingness” as in the case of English quantifier none in singular or plural constructions.
Children are able to understand “zeroness” after they have learnt to use basic addition and subtraction. Young children learn the concept of “zero” as an ordinary number that precedes 1 and has “0” value, but also as part of subtraction that leads to “no entities”, or “nothing”. This is in accordance with our interpretation of “zero” as it appears in the grammatical/syntactic structures quantifier none engages in that gives it the interpretation of no entities in the empty set or as an individual number 0 which precedes 1. Hartmann et al. (2022) confirm our speculation in explaining the concept of “zero” as it appears in natural language through English quantifier none and its grammatical choice of plurality or singularity in the morphology of the verb.
From a mathematical point of view, zero can be processed as a cardinal number 0 that precedes 1 but also as the result of a subtraction 1 – 1 – 1 – 1 = 0. When it comes to learning counting and the concept of zero, preschoolers, Barton (2020) reports, were asked to count 4 cubes in front of them and then count backwards as each cube was taken away till they were all removed; this resulted in associating the cardinal zero with “none” or “nothing”, seeing also zero as the smallest number. This is the exact process we follow in our scenario of an empty classroom; we start with the existence of 5 students, John, Peter, Paul, Marc and Luke, whom I have been teaching Semantics. In order to visualize room 101 empty of students, we visualize a mental subtraction that allows us to take out one by one each student the exact way preschoolers in Barton (2020) started counting backwards the cubes were given till they had subtracted all, till no cube was left. What they we left with was the empty set with no members at all. Similarly, we experience zero as an empty classroom that appears to our mind as the “absence of something”; in other words, from a full class of 5 students to an empty classroom of 0 students.
Both ways result in nothing; no member in the presence of an empty set of students that in order to understand we automatically think of the set of students with, let us say 5 members; in order to process zero students are present or none (of the students) is/are present we need to subtract one by one the members we have visualize till none is left in the set.
Knauff’s (2007) thinking also confirms our initial suggestions about visualizing or seeing with our inner eye the complete set of entities and then subtracting members till the result is zero or the empty set, i.e., a set with no members, which is expressed in language with the use of quantifier none. Inference is a conclusion reached based on the basis of evidence and reasoning. Our understanding of “zeroness” is based on evidence and reasoning as described in our scenario that took place in room 101. Can we say that zero is the inference of a subtraction that takes part in our mind while we try to process the meaning of quantifier none? If yes, then, visualizing the sets of “members-to subtract” results to an empty set, to zero. These sets of “members-to-subtract” are already given in the diagrams of Section 8.
My speculation is that English grammar allows the usage of quantifier none as it reflects the way our mind processes the concept of “zero” as an individual number 0 or as a subtraction as in our example 1 – 1 – 1 – 1 – 1 = 0. This could relate to the unique way every individual’s mind processes the concept of “zero”.
Language is logical and mathematical, and it leaves no room for semantic mistakes.
The mind has the ability to understand “zero” either as “nothing” or as the result of a subtraction of members which would also mean “nothing”; zero is either a cardinal 0 or a subtraction of numbers that results in 0. This appears in English in the way native speakers choose to use the singular or the plural form of the main verb to accompany quantifier none as in examples (7) and (8) mentioned in Section 2.
We emphasize again that the way English expresses “zeroness” in constructions with quantifier none is not the same as in other natural languages. The fact that other natural languages allow only syntactic constructions with the main verb in singular like in Albanian, Greek, French and Arabic, sheds light to the way these languages interpret zero, that is as a subtraction. On the other hand, natural languages like Somali favor syntactic constructions with the main verb in plural; this can be seen as the reason why such natural languages interpret zero as a cardinal with 0 value.
Natural languages have a choice in expressing the concept of zero in constructions with equivalent negative quantifier; this choice probably reflects the way the native speakers of that particular natural language choose to interpret zero, as a subtraction or as a cardinal with 0 value. For the same reason, English allows its native speakers to accept both interpretations of zero, and allow their mind to process both interpretations of the concept of zero.
10. Conclusion
Our minds have been trained to think in a specific way from a very young age and this shows in our thinking. It is not a coincidence that we first learn to count and then understand and learn how to use numbers and quantifiers; after all, they are both responsible for counting and calculating in language.
A notion that could help us explain what our mind goes through trying to make sense of zero is the semantic notion of Quantification.
Quantification is defined as the mental activity of “counting” and “measuring” that appear either in the form of numbers and numerals or as quantifiers that allow a collective, distributive or proportional reading. Quantification allows the brain to resort to mental calculations; for instance, when we use sentences like (11):
11) Some students are late
Our mind processes the number or the percentage of students present and it extracts those students who are late. To be more precise, if the total of students is 10, then the existential quantifier some would apply to 3 or 4.
For few students in (12):
12) Few students are late
Our mind would process a calculation of 1 or 2, while in (13):
13) Many students are late
The quantifier many would reflect a total of 7 or 8 students. Similarly, in (14):
14) Half of the students are late
Half would mean exactly 5 out of the total number of 10 students.
It appears that English native speakers process similar calculations when they use quantifier none. When they say none of the students is present what they do is to pick individual members of the set of students and process a mental subtraction. The state of each student being absent is negated as in none of the students is present. In the case of all the students are absent speakers of English would process the entire number of students as the result of a subtraction and these students would be thought of being absent. A sentence like None of the students are present would be the opposite of All the students are present. Both are in plural because both allow the process of all and none as sums/subtractions of entities and not as individual entities.
Our explanation for this concerns the way our minds process the concept of “zero” which is manifested in language with the choice none has made in using plural or singular constructions.
None manifests in language the concept of zero or “nothingness”.
The way children understand the zero is how we understand it too as adults, and this shows in the syntactic construction we choose when we use English quantifier none. This understanding leads us to choose between the singular and the plural constructions of none. How adults express the concept of zero in language reflects the way they have comprehended “zero” as children. After all, we were instructed to think this way from a very young age. Kaplan (1999) justifies that by stating that “(F)or zero to be a power of equal status with what it empowered, we must understand how to add, subtract, multiply and divide with it…” (Kaplan, 1999: p. 70)
How the mind processes zero reflects on grammar and language. What the mind is doing is to process the empty set. It either excludes all members of the set as a cardinal zero with 0 value, that is the empty set or excludes the members one by one to also result to zero.
Therefore, if people disagree in choosing the singular or the plural form of the verb to accompany none, doesn’t mean that they are right or wrong. It means that their mind chooses to process the concept of “zero” in two different ways; either as the result of a subtraction after they have visualized a set of n members of the entity described in the sentence that results in the empty set of such entity or they have chosen the final visualized collective image of zero as in 0 members of the set. Both ways in processing nothingness are correct as the Concise Oxford Dictionary states and they both result in 0.
This is how grammar processes the empty set, by allowing a choice in plurality or singularity that appears in the morphology of the main verb of the sentence.
Both nature and language are logical and mathematical and they do not leave room for mistakes. It is language and the way it is processed in the mind that allows us to use either singular or plural in the syntactic constructions of none as it intends to manifest the concept of “zero”.
Further investigations to the way natural languages, other than English, express the concept of zero, could be challenging and intriguing in proving that our explanation is universal regarding the way human mind processes the concept of “zero” and regarding the way languages choose to express it.