Elementary Fermions: Strings, Planck Constant, Preons and Hypergluons

Doron Kwiat

Mazkeret Batyia, Israel.

DOI: 10.4236/jhepgc.2024.101008                

Abstract

Using real fields instead of complex ones, it is suggested here that the fermions are pairs of coupled strings with an internal tension. The interaction between the two coupled strings is due to an exchange mechanism which is proportional to Planck’s constant. This may be the result of two massless bosons (hypergluons) coupled by a preon (prequark) exchange. It also gives a physical explanation to the origin of the Planck constant, and origin of spin.

Keywords

Fermions, Preons, Hypergluons, Strings, Real Fields, Planck Constant, Interference, Spin

1. Introduction

The Standard Model recognizes two types of elementary fermions: quarks and leptons.

Mathematically, there are many varieties of fermions, but we will concentrate on Dirac fermions (massive), including neutrinos, leptons, neutrons, protons and their building blocks-quarks. Most Standard Model fermions are believed to be Dirac fermions.

All Dirac fermions have mass and obey the Dirac equation.

In the early days of quantum mechanics, a 3-dimensional non-relativistic equation was offered in order to introduce the concept of a wave function description of elementary particles. The equation described elementary particles in terms of a complex wave function (a state function) in a complex space (Hilbert space). The concept was to allow for a statistical description of particles in terms of probabilities rather than deterministic view.

Dirac equation was later developed as an extension of Schrödinger equation to a relativistic invariant form.

The equation describes all types of fermions (leptons and quarks). They have been considered the elementary particles of matter, but instead they may consist of still smaller entities confined within a volume less than a thousandth the size of a proton [1] .

In another sense, the idea of quark and lepton substructure is a most radical proposal. The electron has now been studied over a century, and its point like nature has been established very well to less than 10⁻¹⁸ m (yet well above Planck length of 10⁻³⁵ m). In the case of the neutrino, also massive, it is even more difficult to imagine an internal structure. The assertion that these particles and the others like them are composites will clearly have to overcome formidable obstacles if it is to have any future. Such a hypothetical theory would begin by introducing a new set of elementary particles, which are referred to generically as preons (prequarks) [1] [2] [3] [4] . Ideally there would not be too many of them. Each quark and lepton in the standard model would be accounted for as a combination of preons, just as each hadron can be explained as a combination of quarks.

Any preon model, regardless of its n details, must supply some mechanism for binding the preons together. There must be a powerful attractive force between them. One strategy is to postulate a new fundamental force of nature analogous in its workings to the color force of the standard model. To emphasize the analogy, the new force is called the hypercolor force and the carrier fields are called hypergluons. The preons (prequarks) are assumed to have hypercolor, but they combine to form hypercolorless composite systems, just as quarks have ordinary color but combine to form colorless protons and neutrons. The hyper color force presumably also gives rise to the property of confinement, again in analogy to the color force. The typical radius of hypercolor confinement must be less than 10⁻¹⁸ meter. Only when matter is probed at distances smaller than this would it be possible to see the hypothetical preons and their hypercolors. At a range of 10⁻¹⁶ or 10⁻¹⁷ m hypercolor effectively disappears. The only objects visible at this scale of resolution (the quarks and leptons) are neutral with respect to hypercolor. At a range of 10⁻¹⁵ m ordinary color likewise fades away, and the world seems to be made up entirely of objects that lack both color and hypercolor: protons, neutrons, electrons and so on.

2. Schrödinger Equation

In non-relativistic quantum mechanics, for example, a particle (such as an electron or proton) is described by a complex wave-function, ψ(x, t), which time-evolution is governed by the Schrödinger Equation:

$-i\hslash \frac{\partial }{\partial t}\psi \left(x,t\right)=\mathcal{H}\psi \left(x,t\right)=-\frac{\hslash }{2m}\frac{{\partial }^2\psi \left(x,t\right)}{\partial x^2}+V\left(x\right)\psi \left(x,t\right)$ (1)

Here m is the particle’s mass and V(x) is the applied potential. Physical information about the behavior of the particle is extracted from the wave function by constructing expectation values for various quantities; for example, the expectation value of the particle’s position is given by integrating ψ^*(x) x ψ(x) over the entire space, and the expectation value of the particle’s momentum is found by integrating 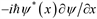. The quantity ψ^*(x)ψ(x) is itself interpreted as a probability density function. This treatment of quantum mechanics, where a particle’s wave function evolves against a classical background potential V(x), is sometimes called first quantization.

The most puzzling term, in my opinion, in this equation, is the imaginary number i. Physics could not possibly have imaginary numbers. They are just a mathematical fiction, which simplifies equations on one hand, but obscures some basic physical characteristics.

It is puzzling how this imaginary number have found its way into physics (as opposed to mathematics) and have changed our concept of physics ever since.

Fields have become complex, operators have become Hermitian and Euclidian space have turned into Hilbert space.

As a matter of fact, the complex view is just a mathematical convenience. Every aspect of quantum physics can be described in terms of real fields.

The problem with the complex presentation is that although it gives excellent results and predictions, it may be hiding the true nature of elementary particle physics. It is therefore worth an effort to describe everything in terms of real fields and see if it helps us develop some deeper understanding of elementary particles.

The basic equation of quantum mechanics is the one particle time-dependent Schrödinger Equation:

$-i\hslash \frac{\partial }{\partial t}\psi \left(x,t\right)=\mathcal{H}\psi \left(x,t\right)$ (2)

By separating Equation (2) into real and imaginary components [5]

$\psi \left(x,t\right)=\Psi ={\phi }_1+i{\phi }_2$ (3)

the Schrödinger equation becomes:

$-i\hslash \frac{\partial }{\partial t}\Psi =\mathcal{H}\Psi =\left({\mathcal{H}}_r+i{\mathcal{H}}_i\right)\left({\phi }_1+i{\phi }_2\right)$ (4)

$+\hslash \frac{\partial }{\partial t}{\phi }_2={\mathcal{H}}_r{\phi }_1-{\mathcal{H}}_i{\phi }_2$ (5)

$-\hslash \frac{\partial }{\partial t}{\phi }_1={\mathcal{H}}_i{\phi }_1+{\mathcal{H}}_r{\phi }_2$ (6)

In other words, the traditional Schrödinger Equation is in fact two coupled equations of real wave functions, with real operators acting in real 3-dimensional space.

For a time-independent classical Hamiltonian of a free particle, with mass m:

$\mathcal{H}=\frac{p^2}{2m}$

${\mathcal{H}}_r=-\frac{{\hslash }^2}{2m}\frac{{\partial }^2}{\partial x^2}$ ${\mathcal{H}}_i=0$

And when separated into real and imaginary components, is equivalent to:

$\frac{\partial {\phi }_1}{\partial t}=+\frac{\hslash }{2m}\frac{{\partial }^2{\phi }_2}{\partial x^2}$ (7)

$\frac{\partial {\phi }_2}{\partial t}=-\frac{\hslash }{2m}\frac{{\partial }^2{\phi }_1}{\partial x^2}$ (8)

A solution of the form ${\phi }_1=A\cos \left(kx-\omega t\right)+B\sin \left(kx-\omega t\right)$ gives:

$\omega =\frac{\hslash }{2m}{k}^2$ (9)

Thus, the general solution will be

${\phi }_1\left(x,t\right)={\displaystyle {\sum }_n{a}_n\cos \left(k_{n}x-{\omega }_{n}t\right)+b_n\sin \left(k_{n}x-{\omega }_{n}t\right)}$ (10)

${\phi }_2(x,t)={\displaystyle {\sum }_n{c}_n\cos \left(k_{n}x-{\omega }_{n}t\right)+d_n\sin \left(k_{n}x-{\omega }_{n}t\right)}$ (11)

where

${\omega }_n=\frac{\hslash }{2m}{k}_n^2$ (12)

There is yet another constraint caused by boundary and symmetry conditions.

If we assume symmetry at t = 0, then ${\phi }_1\left(x,0\right)={\phi }_1\left(-x,0\right)$ , which leads to nulling of sin terms.

Therefore, under symmetry:

${\phi }_1\left(x,t\right)={\displaystyle {\sum }_n{a}_n\cos \left(k_{n}x-{\omega }_{n}t\right)}$ (13)

${\phi }_2\left(x,t\right)={\displaystyle {\sum }_n{c}_n\cos \left(k_{n}x-{\omega }_{n}t\right)}$ (14)

Solutions for the non-relativistic Schrödinger Equation, will not render any new understanding known already from traditional complex space approach. The results should be the same.

However, it is interesting to see, that the real representation and solutions displays two real entities (wave functions) instead of a single, complex entity.

It will be an assumption herewith, that the quantum description and characteristics of a single particle are the result of a coupling interaction between two real components (entities) which compose the single “particle”.

Based on this assumption, it will be described in the following: how can this real interpretation suggest an explanation to the non-relativistic Schrödinger equation through an interacting two-strings coupling.

For a boundary condition

${\phi }_1\left(0,t\right)={\phi }_1\left(L,t\right)=0$ (15)

Namely, a finite size, L particle, fixed at two end points x = 0 and x = L, one obtains

$k_n=\frac{2\pi }{L}n$ (16)

And so, the possible frequency modes are given by

${\omega }_n=\frac{\hslash }{2m}\frac{4{\pi }^2}{L^2}{n}^2$ (17)

3. A 2-String Analog to the Schrödinger Equation

Assume a one-dimensional description in x, and time t.

Let a single particle of mass m be described by two classical real strings, ${\phi }_1\left(x,t\right)$ and ${\phi }_2\left(x,t\right)$ . Here, the functions, ${\phi }_1\left(x,t\right)$ and ${\phi }_2\left(x,t\right)$ represent the amplitudes of the perturbation of the strings from the x-axis as a function of time and position.

Assume coupling between these two strings, given by a constant k_s, and described by the following coupled differential Equations:

$\frac{\partial {\phi }_1}{\partial t}=+k_s\frac{{\partial }^2{\phi }_2}{\partial x^2}$ (18)

$\frac{\partial {\phi }_2}{\partial t}=-k_s\frac{{\partial }^2{\phi }_1}{\partial x^2}$ (19)

It seems to have a peculiar behavior, where the spatial curvature in one field, affects the change in time of the second field and vice a versa.

A physical interpretation to these two equations is the following interaction model (described in Figure 1).

4. Interaction Model

Consider two strings ${\phi }_1$ and ${\phi }_2$ . Let ${\phi }_1\left(x,t\right)$ represent the amplitude of string 1 at time t and at position x. Let ${\tau }_s$ be some tension force acting on the string.

Figure 1. Description of a double string analog as described by Equations (7) and (8). The angle $\theta$ is used to describe the tangent in the approximation of $\tan \theta \approx \sin \theta$ for $\theta \ll 1$ . The two strings have a mutual attraction force. String 1 is pulled in one direction $F_{\tau }$ by the tension force, while it is being pulled in the other direction by $F_{12}$ (the attraction force of string 2 on string 1).

It is well known that the force exerted by this tension, on an infinitesimal string element ΔX (see Figure 1) is related to the change in amplitude along the x axis and is described by:

$F_s={\tau }_s\frac{{\partial }^2{\phi }_1\left(x,t\right)}{\partial x^2}$ (20)

Suppose the second string, described by ${\phi }_2\left(x,t\right)$ , undergoes some temporal perturbation $\Delta {\phi }_2\approx -\frac{\partial {\phi }_2}{\partial t}\Delta t$ .

This perturbation induces a change in a coupling force $F_{21}$ , inflicted by string 2 on string 1, proportional to $\Delta {\phi }_2$ , where it pulls string 1 in the opposite direction of the tensional force $F_s$ , which exists in string 1.

We will assume that this coupling force is proportional to the displacement $\Delta {\phi }_2$ of string 2. We will denote this proportionality coupling constant by k_s.

We will also assume, without loss of generality, that the coupling constant between the two strings is proportional to the mass m. This is a reasonable assumption as we may suppose that with more mass, the stronger is the coupling.

Therefore, $k_s=m{k}_{0s}$ and so:

$\Delta F_{12}=-m{k}_{0s}\Delta {\phi }_2$ (21)

Thus,

$\Delta F_{12}=-m{k}_{0s}\Delta {\phi }_2=-m{k}_{0s}\frac{\partial {\phi }_2}{\partial t}\Delta t$ (22)

Suppose now, this affects the tension in string 1, by $\Delta {\tau }_s$ .

The induced tension force change is given by

$\Delta F_s=\Delta {\tau }_s\frac{{\partial }^2{\phi }_1\left(x,t\right)}{\partial x^2}=\frac{\partial {\tau }_s}{\partial t}\Delta t\frac{{\partial }^2{\phi }_1\left(x,t\right)}{\partial x^2}$ (23)

These two forces, act in opposite directions and are equal, so

$\Delta F_{12}+\Delta F_s=0$ (24)

Therefore,

$m{k}_{0s}\frac{\partial {\phi }_2}{\partial t}=\frac{\partial {\tau }_s}{\partial t}\frac{{\partial }^2{\phi }_1}{\partial x^2}$ (25)

$\frac{\partial {\phi }_2}{\partial t}=\frac{1}{m{k}_{0s}}\frac{\partial {\tau }_s}{\partial t}\frac{{\partial }^2{\phi }_1}{\partial x^2}$ (26)

By same reasoning, in the opposite direction, the equation reads

$\frac{\partial {\phi }_1}{\partial t}=-\frac{1}{m{k}_{0s}}\frac{\partial {\tau }_s}{\partial t}\frac{{\partial }^2{\phi }_2}{\partial x^2}$ (27)

Assume next:

$\frac{1}{k_{0s}}\frac{\partial {\tau }_s}{\partial t}=-\frac{\hslash }{2}$ (28)

The above coupled equations now read

$\frac{\partial {\phi }_1}{\partial t}=+\frac{\hslash }{2m}\frac{{\partial }^2{\phi }_2}{\partial x^2}$ (29)

$\frac{\partial {\phi }_2}{\partial t}=-\frac{\hslash }{2m}\frac{{\partial }^2{\phi }_1}{\partial x^2}$ (30)

which is the coupled real presentation of Schrödinger Equation.

This gives a physical meaning to the Planck constant, namely, independent of a particle’s mass, the Planck constant $\hslash$ is derived from the internal features of the strings (real fields). It represents somehow the reaction of the internal tension of the string fields to perturbations. Up to a proportionality constant,

$\hslash \approx -\frac{1}{k_{0s}\left(t\right)}\frac{\partial {\tau }_s}{\partial t}$ (31)

In order for this equation to make sense, $k_{0s}$ must be as a time-dependent variable.

This leads to the conclusion:

${\tau }_s\left(t\right)=-\hslash {\displaystyle \int k_{0s}\left(t\right)\text{d}t}$ (32)

$k_{0s}$ has the dimensions of 1/sec, so we may assign it the meaning of the number of exchanges (interacting particles) per second, between the two strings.

In other words, ${\tau }_s\left(t\right)$ is the total number of exchanged particles and it is proportional to $\hslash$ . The minimal possible tension in a string will be $\hslash$ .

So, the tension in the strings is proportional to the Planck constant $\hslash$ , and to the basic coupling between the two strings.

Let us assume now, that the leptons are made of two building blocks, two real coupled hypergluons, and the interacting exchange force is due to preons exchange between these two strings (just like in the exchange models of weak and strong interactions).

Strings (Hypergluons) and Preons

Based on the following assumptions:

1) A Classical Fermion is made up of two interacting string-like entities (hypergluons).

2) Tension in the strings is proportional to the coupling strength between the two strings.

3) The coupling force between the two strings is assumed to be the result of preons exchange between these massless bosons.

4) The force is proportional to the duration of the exchange (the actual number of exchanged preons per second).

One is lead to conclude, that Planck’s constant $\hslash$ , is the proportionality constant, between the total exchange between the two strings, and the tension in these strings (see Figure 2).

Looking at Equation (27) we notice that when the mass term $m\to 0$ , the time dependence of the string tends to be very high (which may be the reason of neutrino’s fast oscillation). The only way to prevent this is if the coupling force, Equation (23), vanishes.

On the other hand, when $m\gg 1$ , either the strings become static, or, the coupling force becomes infinite.

Figure 2. The interaction caused be some sort of exchange between the two strings, results in tension in the strings. The proportionality between the exchange force and the tension is the Planck constant $\hslash$ .

5. From Strings Back to Schrödinger

Create the complex representation again:

Defining the complex function $\psi \left(x,t\right)={\phi }_1+i{\phi }_2$

Equations (17) and (18) may be combined together to read:

$i\hslash \frac{\partial \psi }{\partial t}=-\frac{{\hslash }^2}{2m}\frac{{\partial }^2\psi }{\partial x^2}$ (33)

Probabilistic interpretation

We remember that $\psi \left(x,t\right)\text{d}x$ is the probability density of finding a single particle at an interval dx around position x.

Likewise, since

$\psi \left(x,t\right)={\phi }_1+i{\phi }_2$

and

${\displaystyle \int {\psi }^{\ast }\left(x,t\right)\psi \left(x,t\right)\text{d}x}=1$

then

${\displaystyle \int \left({\phi }_1-i{\phi }_2\right)\ast \left({\phi }_1+i{\phi }_2\right)\text{d}x}={\displaystyle \iint \left({\phi }_1^2+{\phi }_2^2\right)\text{d}x}=1$ (34)

Therefore, one may interpret this, as two interacting particles, whose probability densities ${\phi }_1^2$ , and ${\phi }_2^2$ , are affected by each other, and yet, together it is 1, but we cannot tell their probabilities apart.

The Schrödinger equation is now interpreted as two probability density functions coupled according to Equations (17) and (18).

The complex wave equation of a single particle, as described by Schrödinger Equation, is actually a mathematical description of two real waves functions, which, a single particle may be interpreted actually as two coupled entities.

Use of the imaginary number i, and hence complex wave functions (and Hermitian operators), is just a mathematical convenience, obscuring the true nature of the physical world.

By multiplying (18) and (19) by ${\phi }_2$ and ${\phi }_1$ respectively and integrating over x, imposing the mixed boundary conditions ¶ϕ/¶x, ϕ = 0, on both functions at L ® ±∞ it is immediately shown that (up to a normalization factor):

${\displaystyle \int \left({\phi }_1^2+{\phi }_2^2\right)\text{d}x}=1$ (35)

Hence,

${\displaystyle \int {\psi }^{\ast }\left(x,t\right)\psi \left(x,t\right)\text{d}x}=1$ (36)

Thus, the classical coupled string system may be interpreted as a quantum mechanical single particle, described by a wave function $\psi \left(x,t\right)$ being a probability distribution function.

This complex wave function describes a free particle of momentum $p=-i\hslash \frac{\partial }{\partial x}$ .

When substituted in $i\hslash \frac{\partial \psi }{\partial t}=-\frac{{\hslash }^2}{2m}\frac{{\partial }^2\psi }{\partial x^2}$ .

The result is

$i\hslash \frac{\partial \psi }{\partial t}=\mathcal{H}\psi$ (37)

where $\mathcal{H}=\frac{p^2}{2m}=\frac{{\partial }^2}{\partial x^2}$ , is a free particle Hamiltonian operator.

This interpretation of a particle as made up of two real coupled strings, which tensions and interaction are connected, is equivalent to a single particle complex wave function, described by Schrödinger Equation.

When the original Schrödinger Equation is used with complex wave function, this internal string-like characteristic is not showing because we treat the real and imaginary parts as a single entity. However, when the Equation is separated into two parts, these two parts can be treated as independent strings interacting with each other where both tension and interaction fall off abruptly inversely proportional to time. This may be a result of weakening due to increased distance between the two strings, together with tension drop inside the strings.

Just like in the case of hadrons, where it is assumed to have their building blocks made of quarks interacting via gluons, we will assume now that leptons are made of prequarks, interacting via hypergluons.

6. Interference

One of the main phenomena of quantum mechanics is interference. Complex probability densities are the explanation given for interference.

However, based on the concept of real two coupled densities (2 fields), we show that interference can be explained with real distributions.

Assume 2 slits experiment, where the y separation between the two slits is d and the particle go through them.

We assume now that ${\phi }_1$ goes through slit 1 and ${\phi }_1$ goes through slit 2.

As was shown earlier, in Equations (15) and (16), a solution for a free particle is

${\phi }_1=\sin \left(kx-wt\right)$ (38)

${\phi }_2=\cos \left(kx-wt\right)$ (39)

In two dimensions, the variations are in the y-direction while the interference plane is at a distance A in the x direction (see Figure 3).

Figure 3. The interference experiment. 2 slits at separation D apart, allow for two incoming particles to interfere on plate at distance A.

The combined field magnitude at point P(x = A, y) is then given by

$\Vert {\phi }_1+{\phi }_2\Vert =\frac{\sin \left(ky-wt\right)}{\sqrt{A^2+\left(y-D/2\right)}}+\frac{\cos \left(ky-wt\right)}{\sqrt{A^2+\left(y+D/2\right)}}$ (40)

The resulting pattern looks like the following (Figure 4).

This is an explanation of interference patterns, without using complex wave functions and it is based on the separation of a single particle into two coupled fields components. It comes instead of the duality interpretation of particles as waves-particles.

7. Dirac Equation with Real Wave Functions

The relativistic Dirac Equation, describing a free Fermion of mass m is given by:

$\left(i\hslash {\gamma }^{\mu }{\partial }_{\mu }-mc\right)\Psi =0$ (41)

One may separate the Dirac operator $i\hslash {\gamma }^{\mu }{\partial }_{\mu }-mc$ and the complex wave function Ψ into their real and imaginary parts vector $\Psi =\varphi +i\chi$ , which after some work results in:

$\left({{\gamma }^{\prime }}^2{\partial }_y-\frac{mc}{\hslash }\right)\varphi =\left({\gamma }^0\frac{\partial }{c\partial t}+{\gamma }^1{\partial }_x+{\gamma }^3{\partial }_z\right)\chi$ (42)

$\left({{\gamma }^{\prime }}^2{\partial }_y-\frac{mc}{\hslash }\right)\chi =-\left({\gamma }^0\frac{\partial }{c\partial t}+{\gamma }^1{\partial }_x+{\gamma }^3{\partial }_z\right)\varphi$ (43)

where ${{\gamma }^{\prime }}^2=i{\gamma }^2=i\left[\begin{array}{cc}0& {\sigma }_y\\ -{\sigma }_y& 0\end{array}\right]=\left[\begin{array}{cccc}0& 0& 0& 1\\ 0& 0& -1& 0\\ 0& -1& 0& 0\\ 1& 0& 0& 0\end{array}\right]$ .

Since $\varphi$ and $\chi$ are each a real 4-vectors, they can be written as

$\varphi =\left(\begin{array}{c}{\varphi }_A\\ {\varphi }_B\end{array}\right)$ and $\chi =\left(\begin{array}{c}{\chi }_A\\ {\chi }_B\end{array}\right)$ (44)

where ${\varphi }_A$ , ${\varphi }_B$ , ${\chi }_A$ , ${\chi }_B$ are 2-vectors each, and the real wave functions gets the following structure:

Figure 4. An interference pattern example according to Equation (40).

$-c{{\sigma }^{\prime }}_y{\partial }_y{\varphi }_B-\frac{m{c}^2}{\hslash }{\varphi }_A={\partial }_t{\chi }_A+c{\sigma }_x{\partial }_x{\chi }_B+c{\sigma }_z{\partial }_z{\chi }_B$ (45)

$+c{{\sigma }^{\prime }}_y{\partial }_y{\varphi }_A-\frac{m{c}^2}{\hslash }{\varphi }_B=-{\partial }_t{\chi }_B-c{\sigma }_x{\partial }_x{\chi }_A-c{\sigma }_z{\partial }_z{\chi }_A$ (46)

$-c{{\sigma }^{\prime }}_y{\partial }_y{\chi }_B-\frac{m{c}^2}{\hslash }{\chi }_A=-{\partial }_t{\varphi }_A-c{\sigma }_x{\partial }_x{\varphi }_B-c{\sigma }_z{\partial }_z{\varphi }_B$ (47)

$+c{{\sigma }^{\prime }}_y{\partial }_y{\chi }_A-\frac{m{c}^2}{\hslash }{\chi }_B=+{\partial }_t{\varphi }_B+c{\sigma }_x{\partial }_x{\varphi }_A+c{\sigma }_z{\partial }_z{\varphi }_A$ (48)

with ${{\sigma }^{\prime }}_y=\left[\begin{array}{cc}0& 1\\ -1& 0\end{array}\right]$ .

8. Transition to a Boosted System

In a system where the particle is moving along the +x axis alone ( $p_y,p_z=0$ $\to$ ${\partial }_y,{\partial }_z=0$)

$-\frac{m{c}^2}{\hslash }{\chi }_B=+{\partial }_t{\varphi }_B+c{\sigma }_x{\partial }_x{\varphi }_A$ (49)

$-\frac{m{c}^2}{\hslash }{\varphi }_A=+{\partial }_t{\chi }_A+c{\sigma }_x{\partial }_x{\chi }_B$ (50)

$-\frac{m{c}^2}{\hslash }{\chi }_A=-{\partial }_t{\varphi }_A-c{\sigma }_x{\partial }_x{\varphi }_B$ (51)

$-\frac{m{c}^2}{\hslash }{\varphi }_B=-{\partial }_t{\chi }_B-c{\sigma }_x{\partial }_x{\chi }_A$ (52)

These are in fact 8 different real fields, interacting via some coupling form.

Define 2-vectors

${\psi }_1={\varphi }_A+{\varphi }_B$

${\psi }_2={\varphi }_A-{\varphi }_B$

${\psi }_3={\chi }_A+{\chi }_B$

${\psi }_4={\chi }_A-{\chi }_B$

and the equations take the form:

$-\frac{m{c}^2}{\hslash }{\Psi }_1=+{\partial }_t{\Psi }_4-c{\sigma }_x{\partial }_x{\Psi }_4$ (53)

$-\frac{m{c}^2}{\hslash }{\Psi }_4=-{\partial }_t{\Psi }_1-c{\sigma }_x{\partial }_x{\Psi }_1$ (54)

$-\frac{m{c}^2}{\hslash }{\Psi }_2=+{\partial }_t{\Psi }_3+c{\sigma }_x{\partial }_x{\Psi }_3$ (55)

$-\frac{m{c}^2}{\hslash }{\Psi }_3=-{\partial }_t{\Psi }_2+c{\sigma }_x{\partial }_x{\Psi }_2$ (56)

Thus, ${\Psi }_1$ is coupled with ${\Psi }_4$ and ${\Psi }_2$ is coupled with ${\Psi }_3$ . As will be shown later, linear combinations of these represent spin-up and spin-down electron and positron.

9. Eight Real Components

Each ${\text{Ψ}}_i$ is a 2-vector with real components. Thus, the Dirac Equation is actually 8 equations of real components with coupling between the pair $\left({\text{Ψ}}_1,{\text{Ψ}}_4\right)$ , and between the pair $\left({\text{Ψ}}_2,{\text{Ψ}}_3\right)$ .

Defining

${\psi }_1=\left(\begin{array}{c}{U}_1\\ D_1\end{array}\right)$ ${\psi }_2=\left(\begin{array}{c}{U}_2\\ D_2\end{array}\right)$ ${\psi }_3=\left(\begin{array}{c}{U}_3\\ D_3\end{array}\right)$ ${\psi }_4=\left(\begin{array}{c}{U}_4\\ D_4\end{array}\right)$

and applying a time derivative to the first equation of each pair and using the second component of each pair, one obtains:

$\left({\left(\frac{m{c}^2}{\hslash }\right)}^2+{\partial }_t^2\right)U_1+c{\partial }_x{\partial }_t{U}_1=-\frac{m{c}^3}{\hslash }{\partial }_x{U}_4$ (57)

$\left({\left(\frac{m{c}^2}{\hslash }\right)}^2+{\partial }_t^2\right)D_1-c{\partial }_x{\partial }_t{D}_1=+\frac{m{c}^3}{\hslash }{\partial }_x{D}_4$ (58)

$\left({\left(\frac{m{c}^2}{\hslash }\right)}^2+{\partial }_t^2\right)U_4-c{\partial }_x{\partial }_t{U}_4=+\frac{m{c}^3}{\hslash }{\partial }_x{U}_1$ (59)

$\left({\left(\frac{m{c}^2}{\hslash }\right)}^2+{\partial }_t^2\right)D_4+c{\partial }_x{\partial }_t{D}_4=-\frac{m{c}^3}{\hslash }{\partial }_x{D}_1$ (60)

$\left({\left(\frac{m{c}^2}{\hslash }\right)}^2+{\partial }_t^2\right)U_2+c{\partial }_x{\partial }_t{U}_2=-\frac{m{c}^3}{\hslash }{\partial }_x{U}_3$ (61)

$\left({\left(\frac{m{c}^2}{\hslash }\right)}^2+{\partial }_t^2\right)D_2-c{\partial }_x{\partial }_t{D}_2=+\frac{m{c}^3}{\hslash }{\partial }_x{D}_3$ (62)

$\left({\left(\frac{m{c}^2}{\hslash }\right)}^2+{\partial }_t^2\right)U_3+c{\partial }_x{\partial }_t{U}_3=-\frac{m{c}^3}{\hslash }{\partial }_x{U}_2$ (63)

$\left({\left(\frac{m{c}^2}{\hslash }\right)}^2+{\partial }_t^2\right)D_3-c{\partial }_x{\partial }_t{D}_3=+\frac{m{c}^3}{\hslash }{\partial }_x{D}_2$ (64)

These demonstrate the coupled pairs: (U₁, U₄), (D₁, D₄), (U₂, U₃) and (D₂, D₃).

10. Solution

One possible solution to these coupled differential equations (Equations (59)-(66)) is:

$U_1=\cos \left(px-\omega t\right)$ (65)

$D_1=\sin \left(px-\omega t\right)$ (66)

$U_4=\sin \left(px-\omega t\right)$ (67)

$D_4=\cos \left(px-\omega t\right)$ (68)

$U_2=\cos \left(px-\omega t\right)$ (69)

$D_2=\sin \left(px-\omega t\right)$ (70)

$U_3=\sin \left(px-\omega t\right)$ (71)

$D_3=\cos \left(px-\omega t\right)$ (72)

When inserted in the 8-componenents equations and solving, one obtains

$\left({\omega }_0^2-{\omega }^2\right)+c\omega p=-c{\omega }_{0}p$ (73)

$\left({\omega }_0^2-{\omega }^2\right)-c\omega p=+c{\omega }_{0}p$ (74)

$\left({\omega }_0^2-{\omega }^2\right)-c\omega p=+c{\omega }_0\left(-p\right)$ (75)

$\left({\omega }_0^2-{\omega }^2\right)+c\omega p=-c{\omega }_0\left(-p\right)$ (76)

To summarize, the solutions are described in the following table (Table 1).

With $p\equiv \frac{p_x}{\hslash }$ , where $p_x$ is the x component of the momentum.

When boosted to a rest system (where ${\partial }_x=0$ ) we obtain for all components

$\left[{\partial }_t^2+{\left(\frac{m{c}^2}{\hslash }\right)}^2\right]{\Psi }_i=0$ (77)

Table 1. Summary of possible solutions in the +x boosted system.

Solving this equation by setting $\Psi =\cos \left(\omega t\right)$ or $\Psi =\sin \left(\omega t\right)$ shows that all components of this fermion at the rest frame, are oscillating at a rate given by ${\omega }_0=\frac{m{c}^2}{\hslash }\approx 7.7\times {10}^{11}\text{GHz}$ .

At such high oscillating rate, the spin cannot be determined apriori, and depends on the random outcome of the instance of measurement. As a result, the measured spin outcome is random, with equal probabilities for being up or down.

The necessary conclusion is that each particle with spin, must have a definite time dependent spin. This spin oscillates at a fixed rate ${\omega }_{e,p}\approx 7.7\times {10}^{11}\text{GHz}$ for an electron.

Hence, pending on the instance of creation we will get a certain result up or down. It looks like a mixture of states merely because our temporal measurement resolution is insufficient.

Since in an electron-positron creation, both are created simultaneously, their spins will be correlated.

Thus, there are two particles states involved. One, denoted by (+) precessing around the X axis in a positive right direction, and the other, denoted by (−), precessing around the X axis in the opposite (left) direction:

${\theta }_{+}\left(t\right)={\omega }_{0}t$ Right rotation.

${\theta }_{-}\left(t\right)=-{\omega }_{0}t$ Left rotation.

With ${\theta }_{+}\left(t\right)=-{\theta }_{-}\left(t\right)$ .

At a boosted system, where p = 0:

${\text{Ψ}}_1=\left[\begin{array}{c}\cos \left({\theta }_{+}\left(t\right)\right)\\ \sin \left({\theta }_{-}\left(t\right)\right)\end{array}\right]$ ${\text{Ψ}}_4=\left[\begin{array}{c}\sin \left({\theta }_{+}\left(t\right)\right)\\ \cos \left({\theta }_{+}\left(t\right)\right)\end{array}\right]$

${\text{Ψ}}_2=\left[\begin{array}{c}\cos \left({\theta }_{+}\left(t\right)\right)\\ \sin \left({\theta }_{-}\left(t\right)\right)\end{array}\right]$ ${\text{Ψ}}_3=\left[\begin{array}{c}\sin \left({\theta }_{+}\left(t\right)\right)\\ \cos \left({\theta }_{+}\left(t\right)\right)\end{array}\right]$

11. Spin up and Spin down

We will use now the results for a boosted system, that is, we move with the massive elementary particle. Define $u^{UP}$ , $u^{DN}$ , and $v^{UP}$ , $v^{DN}$ 4-vectors as follows:

$\begin{array}{c}{u}^{UP}=\frac{1}{2}\left({\Psi }_1+{\sigma }_x{\Psi }_4\right)=\frac{1}{2}\left(\left[\begin{array}{c}\cos \left({\theta }_{+}\left(t\right)\right)\\ \sin \left({\theta }_{-}\left(t\right)\right)\end{array}\right]+\left[\begin{array}{cc}0& 1\\ 1& 0\end{array}\right]\left[\begin{array}{c}\sin \left({\theta }_{+}\left(t\right)\right)\\ \cos \left({\theta }_{+}\left(t\right)\right)\end{array}\right]\right)\\ =\left[\begin{array}{c}\cos \left({\theta }_{+}\left(t\right)\right)\\ 0\end{array}\right]\end{array}$ (78)

$u^{DN}=\frac{1}{2}\left({\Psi }_1-{\sigma }_x{\Psi }_4\right)=-\left[\begin{array}{c}0\\ \sin \left({\theta }_{+}\left(t\right)\right)\end{array}\right]$ (79)

$v^{UP}=\frac{1}{2}\left({\Psi }_2+{\sigma }_x{\Psi }_3\right)=\left[\begin{array}{c}\cos \left({\theta }_{+}\left(t\right)\right)\\ 0\end{array}\right]$ (80)

$v^{DN}=\frac{1}{2}\left({\Psi }_2-{\sigma }_x{\Psi }_3\right)=-\left[\begin{array}{c}0\\ \sin \left({\theta }_{+}\left(t\right)\right)\end{array}\right]$ (81)

To find their spin state we apply ${\sigma }_x=\left[\begin{array}{cc}0& 1\\ 1& 0\end{array}\right]$ and ${\sigma }_z=\left[\begin{array}{cc}1& 0\\ 0& -1\end{array}\right]$ to find:

${\sigma }_z{u}^{UP}=+1u^{UP}$ (82)

${\sigma }_z{u}^{DN}=-1u^{DN}$ (83)

${\sigma }_z{v}^{UP}=+1v^{UP}$ (84)

${\sigma }_z{v}^{DN}=-1v^{DN}$ (85)

These represent two eigenfunctions of spin up and spin down for the u solution and two eigenfunctions of spin up and spin down for the v solution.

The interaction between the fermion and the magnetic field B is given by $\stackrel{\to }{\mu }\cdot \stackrel{\to }{B}=\hslash g{B}_z$ where g is the g-factor of the fermion.

Under a magnetic field ${\stackrel{\to }{B}}_0=\hat{k}{B}_z$ the change in energies of the above states is given by

$\Delta H{u}^{UP}=+\frac{1}{2}\hslash g{B}_z{u}^{UP}$ (86)

$\Delta H{u}^{DN}=-\frac{1}{2}\hslash g{B}_z{u}^{DN}$ (87)

$\Delta H{v}^{UP}=+\frac{1}{2}\hslash g{B}_z{v}^{UP}$ (88)

$\Delta H{v}^{UP}=+\frac{1}{2}\hslash g{B}_z{v}^{UP}$ (89)

This demonstrates, that in the presence of a magnetic field, there exist two spin states (up and down) for $\varphi$ and two spin states (up and down) for $\chi$ .

The energy difference between two states is given by

${\tilde{u}}^{UP}\Delta H{u}^{UP}-{\tilde{u}}^{DN}\Delta H{u}^{DN}=+\frac{1}{2}\hslash g{B}_z{\cos }^2\left(\theta \right)+\frac{1}{2}\hslash g{B}_z{\sin }^2\left(\theta \right)=\hslash g{B}_z$ (90)

The energy difference between the two states is independent of time and of their spatial location along the x-axis.

The two states are time-dependent and position-dependent, yet their spin does not change with time and in space:

$\left[\begin{array}{c}\cos \left({\theta }_{+}\left(t\right)\right)\\ 0\end{array}\right]=\left[\begin{array}{c}\cos \left(\left({\omega }_0-pc\right)t\right)\\ 0\end{array}\right]{\sigma }_z{u}^{UP}$ (91)

$\left[\begin{array}{c}0\\ \sin \left({\theta }_{-}\left(t\right)\right)\end{array}\right]=\left[\begin{array}{c}0\\ \sin \left(\left({\omega }_0-pc\right)t\right)\end{array}\right]{\sigma }_z{u}^{DN}$ (92)

$\left[\begin{array}{c}\cos \left({\theta }_{+}\left(t\right)\right)\\ 0\end{array}\right]=\left[\begin{array}{c}\cos \left(\left({\omega }_0-pc\right)t\right)\\ 0\end{array}\right]{\sigma }_z{v}^{UP}$ (93)

$\left[\begin{array}{c}0\\ \sin \left({\theta }_{-}\left(t\right)\right)\end{array}\right]=\left[\begin{array}{c}0\\ \sin \left(\left({\omega }_0-pc\right)t\right)\end{array}\right]{\sigma }_z{v}^{DN}$ (94)

As is obvious from the above description of states, both electron and positron, when created simultaneously, will have their spins correlated.

The up state gives always a spin +1 but as can be seen, the state itself varies with cos() between $\left(\begin{array}{c}-1\\ 0\end{array}\right)$ to $\left(\begin{array}{c}+1\\ 0\end{array}\right)$ . The rate of change is ${\omega }_0=\frac{m{c}^2}{\hslash }\approx 7.7\times {10}^{11}\text{GHz}$ .

By creating linear combinations of the vectors

${\psi }_1=\left(\begin{array}{c}{U}_1\\ D_1\end{array}\right)$ ${\psi }_2=\left(\begin{array}{c}{U}_2\\ D_2\end{array}\right)$ ${\psi }_3=\left(\begin{array}{c}{U}_3\\ D_3\end{array}\right)$ ${\psi }_4=\left(\begin{array}{c}{U}_4\\ D_4\end{array}\right)$

We obtain the following states:

$u^{UP}=\frac{1}{2}\left({\Psi }_1+{\sigma }_x{\Psi }_4\right)=\frac{1}{2}\left(\left(\begin{array}{c}{U}_1\\ D_1\end{array}\right)+{\sigma }_x\left(\begin{array}{c}{U}_4\\ D_4\end{array}\right)\right)=\frac{1}{2}\left(\begin{array}{c}{U}_1+D_4\\ D_1+U_4\end{array}\right)$ (95)

$u^{DN}=\frac{1}{2}\left({\Psi }_1-{\sigma }_x{\Psi }_4\right)=\frac{1}{2}\left(\left(\begin{array}{c}{U}_1\\ D_1\end{array}\right)-{\sigma }_x\left(\begin{array}{c}{U}_4\\ D_4\end{array}\right)\right)=\frac{1}{2}\left(\begin{array}{c}{U}_1-D_4\\ D_1-U_4\end{array}\right)$ (96)

$v^{UP}=\frac{1}{2}\left({\Psi }_2+{\sigma }_x{\Psi }_3\right)=\frac{1}{2}\left(\left(\begin{array}{c}{U}_2\\ D_2\end{array}\right)+{\sigma }_x\left(\begin{array}{c}{U}_3\\ D_3\end{array}\right)\right)=\frac{1}{2}\left(\begin{array}{c}{U}_2+D_3\\ D_2+U_3\end{array}\right)$ (97)

$v^{DN}=\frac{1}{2}\left({\Psi }_2-{\sigma }_x{\Psi }_3\right)=\frac{1}{2}\left(\left(\begin{array}{c}{U}_2\\ D_2\end{array}\right)-{\sigma }_x\left(\begin{array}{c}{U}_3\\ D_3\end{array}\right)\right)=\frac{1}{2}\left(\begin{array}{c}{U}_2-D_3\\ D_2-U_3\end{array}\right)$ (98)

This can be interpreted as 4 different modes of the fermion:

Spin-up particle,

Spin-down particle,

Spin up anti-particle,

Spin-down anti-particle.

It looks like the constituents must come in doublets of two each. Either in a symmetric or in an asymmetric composition. For instance:

A fermion (e.g. electron) at a spin-up state will be $u^{UP}=\frac{1}{2}\left(\begin{array}{c}{U}_1+D_4\\ D_2+U_3\end{array}\right)$ .

A fermion (e.g. electron) at a spin-down state will be $u^{DN}=\frac{1}{2}\left(\begin{array}{c}{U}_1-D_4\\ D_1-U_4\end{array}\right)$ .

An antifermion (e.g. positron) at a spin-up state will be $v^{UP}=\frac{1}{2}\left(\begin{array}{c}{U}_2+D_3\\ D_2+U_3\end{array}\right)$ .

An antifermion (e.g. positron) at a spin-down state will be $v^{DN}=\frac{1}{2}\left(\begin{array}{c}{U}_2-D_3\\ D_3-U_2\end{array}\right)$ .

Positron and Electron are the results of Prequarks confinement and Hypergluons exchange.

As can be seen, solution to the Dirac Equation results in 8 real constituents altogether. They create 4 coupled pairs. Two coupled pairs connect in either symmetric or anti symmetric states, to create a spin-up or a spin down fermion.

A fermion is made up of symmetric (spin-up) and antisymmetric (spin down) combinations of U₂ D₄ D₁ U₄. An anti-fermion is made of symmetric (spin-up) and antisymmetric (spin down) combinations of U₂ U₃ D₃ D₂.

Based on the string interpretation described earlier, one can describe the internal couplings U₁ « U₄, D₁ « D₄, U₂ « U₃, D₂ « D₃ by some exchange mechanism that binds them together, where the coupling is between hypergluons via preons exchange.

The fermions are the result of combing pairs of such couples, in either symmetric or anti symmetric forms.

This provides a physical explanation to the source of spin-half characteristics of all fermions.

12. Weyl and Majorana

Weyl [6] showed that the massless Dirac Equation could be reduced to a two-component equation. The solutions of that equation are called Weyl spinors, or Weyl fermions [6] .

What is currently accepted is the following status.

Weyl fermions are two-component spinors.

Massive Dirac spinors can be written as combinations of left- and right-handed massless Weyl fermions.

Another way of representing the massive Dirac equation found by Ettore Majorana [7] .

Majorana particle is a fermion that is its own antiparticle. They were hypothesized by Ettore Majorana in 1937. The term is sometimes used in opposition to a Dirac fermion, which describes fermions that are not their own antiparticles.

With the exception of the neutrino, all of the Standard Model fermions are known to behave as Dirac fermions at low energy (after electroweak symmetry breaking), and none are Majorana fermions. The nature of the neutrinos is not settled. They may be either Dirac or Majorana fermions.

The concept goes back to Majorana’s suggestion that neutral spin-¹⁄₂ particles can be described by a real wave equation (the Majorana equation), and would therefore be identical to their antiparticle (because the wave functions of particle and antiparticle are related by complex conjugation).

Both Weyl and Majorana spinors can be regarded as constrained cases of Dirac spinors.

A Weyl fermion is distinguishable from its anti-particle because the spinors are complex.

Majorana fermions on the other hand are four component spinors for which the particle and antiparticle are indistinguishable. A Majorana fermion is its own antiparticle.

At the current time, things are somewhat up in the air, since neutrinos have been found to be massive rather than massless, even if their masses are very small.

One need to look at the case where m = 0, the so-called Weyl Fermion.

All U_i are solutions to

$\hat{D}{U}_i=0$ (99)

where $\hat{D}\equiv {\partial }_t^2+c{\partial }_x{\partial }_t$ .

The solutions are

$U_i=A\cos \left(k\left(x-ct\right)\right)$ (100)

Representing a massless boson, moving at speed c.

As a matter of fact, from the real 8 equations formalism we see that when m = 0, the coupling disappears and we are left with a single equation, similar to all 8 components. Thus, the Weyl particle is actually a single massless boson, moving at speed c.

13. Conclusions

By making all quantum mechanics to be based on real wave-functions, in real space and using non-Hermitian operators, we showed that both Schrödinger and Dirac equations can be considered as representing real coupled entities. These entities represent 2 interacting strings (non-relativistic Schrödinger equation) or as 4 interacting strings (relativistic Dirac Equation).

The picture of interacting strings leads us to conclude that both Schrödinger and Dirac equations describe some exchange mechanism between coupled pairs of strings.

Our model provides an explanation to several known quantum phenomena:

1) Interference

2) Planck Constant

3) Spin

One may therefore re-consider our view of leptons as being elementary particles. Just like Hadrons are assumed to be made of quarks interacting via gluons and confined, so can we consider leptons as being made of some basic units: Prequarks (just like quarks in the case of hadrons) interacting via some exchange forces, Hypergluons (just like the presumed gluons in the case of strong interactions).

It may be a possibility that someday quarks and prequarks, as well as gluons and hypergluons, be shown to be the same.

Conflicts of Interest

The author declares no conflicts of interest regarding the publication of this paper.

References

[1]	Kwiat, D. (2018) The Schrödinger Equation and Asymptotic Strings. International Journal of Theoretical and Mathematical Physics, 8.
[2]	Weyl, H. (1929) Electron and Gravitation. Z. Phys., 56, 330-352.
[3]	Parrochia, D. (2019) Majorana Equation and Its Consequences in Physics and Philosophy. arXiv:1907.11169 [physics.hist-ph]
[4]	Harari, H. (2012) The Structure of Quarks and Leptons. Albert Einstein Memorial Lectures, 47-79.
[5]	Pati, J.C. and Salam, A. (1974) Lepton Number as the Fourth “Color”. Physical Review D, 10, 275. https://doi.org/10.1103/PhysRevD.10.275
[6]	Bars, I. and Yankielowicz, S. (1981) Composite Quarks and Leptons as Solutions of Anomaly Constraints. Physics Letters B, 101, 159-165. https://doi.org/10.1016/0370-2693(81)90664-X
[7]	Hubsch, T., Nishino, H. and Pati, J.C. (1985) Do Superstrings Lead to Quarks or to Preons? Physics Letters B, 163, 111-117. https://doi.org/10.1016/0370-2693(85)90203-5