Hyperquaternionic Representations of Conic Sections

Grégoire Lutanda Panga

Department of Mathematics and Informatic, University of Lubumbashi, Lubumbashi, The Democratic Republic of the Congo.

DOI: 10.4236/jamp.2022.1010200                

Abstract

In this paper, by means of an isomorphism, we express the Clifford algebra Cl_5,3 as hyperquaternion algebra H ⊗ H ⊗ H ⊗ H (a four-fold tensor product of quaternion algebras) and we provide the hyperquaternionic approach to the inner product null space (IPNS) representation of conic sections.

Keywords

Clifford Algebra, Multivectors, Quaternions, Hyperquaternions

1. Introduction

In the realm of Hyperquaternion algebras, for a choice of generators, the authors presented in detail the multivector structures of the biquaternion algebra or Pauli algebra $ℍ\otimes ℂ$, the tetraquaternion algebra $ℍ\otimes ℍ$, the Dirac algebra $ℍ\otimes ℍ\otimes ℂ$, and the algebra $ℍ\otimes ℍ\otimes ℍ$ respectively in [1] [2] [3] and [4] where $ℍ$ denotes the quaternion algebra described for the first time by Sir William Rowan in 1843. Their symmetric groups are also given; we will cite: $SO\left(3\right)\mathrm{,}SO\left(\mathrm{1,3}\right)\mathrm{,}SU\left(4\right)$ and $USp\left(4\right)$ respectively for $ℍ\otimes ℂ\mathrm{,}ℍ\otimes ℍ\mathrm{,}ℍ\otimes ℍ\otimes ℂ$ and $ℍ\otimes ℍ\otimes ℍ$. In particular, more physical applications associated are developed in 3D, special theory of relativity, general theory of relativity, quantum theory, ... The papers of Girard et al. inspire us to deal with the hyperquaternion formulation of the Clifford algebra $C{l}_{\mathrm{5,3}}$ with the difference that we combine the results $C{l}_{p+\mathrm{1,}q+1}\simeq C{l}_{p\mathrm{,}q}\otimes C{l}_{\mathrm{1,1}}$, $ℍ\otimes ℍ\simeq ℝ\left(4\right)$, $C{l}_{\mathrm{1,1}}\simeq ℝ\left(2\right)$ and $ℝ\left(m\right)\otimes ℝ\left(n\right)\simeq ℝ\left(mn\right)$ instead of Clifford’s theorem in order to establish the isomorphism $C{l}_{\mathrm{5,3}}\simeq ℍ\otimes ℍ\otimes ℍ\otimes ℍ$. We recall Clifford’s theorem used by P. Girard.

If $n=2m$ (m:integer), the Clifford algebra $C{l}_{2m}$ is the tensor product of m quaternion algebras. If $n=2m-1$, the Clifford algebra $C{l}_{2m-1}$ is the tensor product of $m-1$ quaternion algebras and the algebra ( $\mathrm{1,}\omega$ ) where $\omega$ is the product of 2m generators ( $\omega =e_1{e}_2\cdots e_{2m}$ ) of the algebra $C{l}_{2m}$ [2]. The entirety of the proof can be seen in [5], p.378 and a modern proof can be found in [2], p.3.

In [6], W.Sproβig gave a brief origin of the term hyperquartenion by saying verbatim the following: “The name hyperquaternion was coined in 1922 by the American mathematician Clarence Lemuel Elisha Moore (1876-1931). Nowadays, there are remarkable works of M.Pitkanen and P.Girard in this field”.

This study of the Clifford Algebra $Cl\left(\mathrm{5,3}\right)\simeq {ℍ}^{\otimes 4}$ allows expressing as conformal hyperquaternion algebra the Conic Conformal Geometry Algebra (CCGA) we intend to carry out starting from papers [7] and [8].

Unless otherwise mentioned, throughout this paper ${ℍ}^{\otimes p}$ is the tensor product of p quaternion algebras $ℍ$, i.e. ${ℍ}^{\otimes p}=ℍ\otimes ℍ\otimes \cdots \otimes ℍ$ (p factors).

This paper is structured as follows:

In the first section, which is the introduction, we briefly present some works done on the hyperquaternion algebras, their historical and the central objective of this paper. The aim of the second section is to gather some basic results concerning the quaternion algebras, hyperquaternion algebras and Clifford algebras. In the third section, we first recall the ingredients will be used to show the isomorphism between the hyperquaternion algebra ${ℍ}^{\otimes 4}$ and the clifford algebra $C{l}_{\mathrm{5,3}}$ and we establish an isomorphim of these algebras. We also express the multivector structures of ${ℍ}^{\otimes 4}$ in this section. In the fourth section, we develop the hyperquaternion algebra for conics.

2. Preliminaries of Clifford Algebras and Hyperquaternion Algebras

2.1. Clifford Algebras

Definition 2.1. Let $\left(E\mathrm{,}q\right)$ be a quadratic vector space over $\mathbb{K}$ and $T\left(E\right)=\mathbb{K}\oplus E\oplus E\otimes E\oplus E\otimes E\otimes E\oplus \cdots ={{\displaystyle \oplus }}_{i\ge 0}{E}^{\otimes i}$ be the tensor algebra of E over $\mathbb{K}$. The quotient algebra $Cl\left(E\mathrm{,}q\right)=\frac{T\left(E\right)}{I\left(E\mathrm{,}q\right)}$ , where $I\left(E\mathrm{,}q\right)$ is the ideal generated by all elements of the form $x\otimes x-q\left(x\right)$ for $x\in E$ , is called the Clifford algebra associated to the quadratic vector space $\left(E\mathrm{,}q\right)$.

Consider the quadratic space ${ℝ}^{p\mathrm{,}q}$, this notation means that p basis vectors square to +1 and q basis vectors square to −1. Let $\left(e_1\mathrm{,}\cdots \mathrm{,}{e}_p\mathrm{,}{e}_{p+1}\mathrm{,}\cdots \mathrm{,}{e}_{p+q}\right)$ be an orthonormal basis of ${ℝ}^{p\mathrm{,}q}$, $q\left(x\right)=q\left(x^i{e}_i\right)={\left(x^1\right)}^2+\cdots +{\left(x^p\right)}^2-{\left(x^1\right)}^2-\cdots -{\left(x^{p+q}\right)}^2$, for any $x\in {ℝ}^{p\mathrm{,}q}$. Thus, we have

$e_i^2=1\left(1\le i\le p\right),e_i^2=-1\left(p+1\le i\le q\right),e_i{e}_j+e_j{e}_i=0,\left(i\ne j\right).$ (1)

We denote the Clifford algebra associated to the quadratic space ${ℝ}^{p\mathrm{,}q}$ by $Cl\left({ℝ}^{p\mathrm{,}q}\right)$ or $C{l}_{p\mathrm{,}q}$.

Definition 2.2. Let $Cl\left(E\mathrm{,}q\right)$ be the Clifford algebra associated with the quadratic vector space $\left(E\mathrm{,}q\right)$ , the Clifford product of two vectors $u\mathrm{,}v\in Cl\left(E\mathrm{,}q\right)$ is defined by

$uv=u\cdot v+u\wedge v$ (2)

where $u\cdot v$ and $u\wedge v$ are respectively the interior product and the exterior product of the vectors u and v [1].

It follows from this definition that

$u\cdot v=\frac{1}{2}\left(uv+vu\right)$ (3)

and

$u\wedge v=\frac{1}{2}\left(uv-vu\right).$ (4)

2.2. Clifford Algebra $C{l}_{5,3}$

In this subsection, we are interested in just one particular Clifford algebra, $C{l}_{\mathrm{5,3}}$, which is the principal object of our investigation. We consider ${ℝ}^{\mathrm{5,3}}$ an eight-dimensional vector space over $ℝ$ endowed with a bilinear symmetric and nondegenerate form with signature (+, +, +, +, +, −, −, −), which means that 5 basis vectors square to +1 and 3 basis vectors square to −1. Let $\left(e_1\mathrm{,}{e}_2\mathrm{,}{e}_3\mathrm{,}{e}_4\mathrm{,}{e}_5\mathrm{,}{e}_6\mathrm{,}{e}_7\mathrm{,}{e}_8\right)$ be a basis of ${ℝ}^{\mathrm{5,3}}$, the Clifford algebra $C{l}_{\mathrm{5,3}}$ is the real associative unital algebra generated by the vectors $e_1\mathrm{,}{e}_2\mathrm{,}{e}_3\mathrm{,}{e}_4\mathrm{,}{e}_5\mathrm{,}{e}_6\mathrm{,}{e}_7$ and $e_8$ satisfying the relations:

$e_i^2=1\left(1\le i\le 5\right),e_i^2=-1\left(6\le i\le 8\right),$ (5)

and

$e_i{e}_j+e_j{e}_i=0,\left(i\ne j\right).$ (6)

A basis of the Clifford algebra $C{l}_{\mathrm{5,3}}$ can be taken to be $\mathrm{1,}{e}_1\mathrm{,}{e}_2\mathrm{,}{e}_3\mathrm{,}{e}_4\mathrm{,}{e}_5\mathrm{,}{e}_6\mathrm{,}{e}_7\mathrm{,}{e}_8$, $e_1{e}_2\mathrm{,}{e}_1{e}_3\mathrm{,}\cdots \mathrm{,}{e}_7{e}_8\mathrm{,}{e}_1{e}_2{e}_3\mathrm{,}{e}_1{e}_2{e}_4\mathrm{,}\cdots \mathrm{,}{e}_6{e}_7{e}_8\mathrm{,}{e}_1{e}_2{e}_3{e}_4\mathrm{,}\cdots \mathrm{,}{e}_1{e}_2{e}_3{e}_4{e}_5{e}_6{e}_7{e}_8$.

Definition 2.3. Let $Cl\left(E\mathrm{,}q\right)$ be the Clifford algebra associated with the quadratic vector space $\left(E\mathrm{,}q\right)$ , the products of k generators are called multivectors of grade k, blades of degree k or k-vectors.

Every element of $Cl\left(\mathrm{5,3}\right)$ can split into:

$\left(\begin{array}{c}8\\ 0\end{array}\right)=1$ scalar (or 0-vector): 1,

$\left(\begin{array}{c}8\\ 1\end{array}\right)=8$ vectors (or 1-vectors): $e_1\mathrm{,}{e}_2\mathrm{,}{e}_3\mathrm{,}{e}_4\mathrm{,}{e}_5\mathrm{,}{e}_6\mathrm{,}{e}_7\mathrm{,}{e}_8$,

$\left(\begin{array}{c}8\\ 2\end{array}\right)=28$ bivectors (or 2-vectors): $e_1{e}_2\mathrm{,}{e}_1{e}_3\mathrm{,}\cdots \mathrm{,}{e}_7{e}_8$,

$\left(\begin{array}{c}8\\ 3\end{array}\right)=56$ trivectors (or 3-vectors): $e_1{e}_2{e}_3\mathrm{,}{e}_1{e}_2{e}_4\mathrm{,}\cdots \mathrm{,}{e}_6{e}_7{e}_8$,

$\left(\begin{array}{c}8\\ 4\end{array}\right)=70$ quadrivectors (or 4-vectors): $e_1{e}_2{e}_3{e}_4\mathrm{,}\cdots \mathrm{,}{e}_5{e}_6{e}_7{e}_8$,

$\left(\begin{array}{c}8\\ 5\end{array}\right)=56$ (5-vectors): $e_1{e}_2{e}_3{e}_4{e}_5\mathrm{,}\cdots \mathrm{,}{e}_4{e}_5{e}_6{e}_7{e}_8$,

$\left(\begin{array}{c}8\\ 6\end{array}\right)=28$ (6-vectors): $e_1{e}_2{e}_3{e}_4{e}_5{e}_6\mathrm{,}\cdots \mathrm{,}{e}_3{e}_4{e}_5{e}_6{e}_7{e}_8$,

$\left(\begin{array}{c}8\\ 7\end{array}\right)=8$ (7-vectors): $e_1{e}_2{e}_3{e}_4{e}_5{e}_6{e}_7\mathrm{,}\cdots \mathrm{,}{e}_2{e}_3{e}_4{e}_5{e}_6{e}_7{e}_8$,

$\left(\begin{array}{c}8\\ 8\end{array}\right)=1$ pseudoscalar: $e_1{e}_2{e}_3{e}_4{e}_5{e}_6{e}_0{e}_7{e}_8$.

It is obvious that ${\displaystyle {\sum }_{k=0}^8}\left(\begin{array}{c}8\\ k\end{array}\right)=2^8=256$ is the dimension of the Clifford algebra $C{l}_{\mathrm{5,3}}$ and a general element of this algebra is a linear combination of the 256 basis multivectors.

2.3. Quaternion Algebra

Definition 2.4. The quaternion algebra over $ℝ$ , denoted $ℍ$ , is an associative non-commutative four-dimensional algebra over $ℝ$ generated by $\mathrm{1,}i\mathrm{,}j$ and k such that $i^2=j^2=k^2=ijk=-1$.

A general element of the quaternion algebra $ℍ$ can be written as a linear combination of $\mathrm{1,}i\mathrm{,}j$ and $k$, $q=a+bi+cj+dk\in ℍ$ with $a\mathrm{,}b\mathrm{,}c\mathrm{,}d\in ℝ$.

2.4. Hyperquaternion Algebras

2.4.1. Definition and Examples

Definition 2.5. Let $ℍ$ be a quaternion algebra over the real field $ℝ$ , a tensor product of $ℍ$ (or a subalgebra thereof) is called a hyperquaternion algebra [9] .

As hyperquaternion algebras, we can cite the biquaternion algebra or Pauli algebra $ℍ\otimes ℂ$, the tetraquaternion algebra $ℍ\otimes ℍ$, the Dirac algebra $ℍ\otimes ℍ\otimes ℂ$, $ℍ\otimes ℍ\otimes ℍ$, ...

2.4.2. Hyperquaternion Algebra $ℍ\otimes ℍ\otimes ℍ\otimes ℍ={ℍ}^{\otimes 4}$

The hyperquaternion algebra concerned in this paper is ${ℍ}^{\otimes 4}$.

Definition 2.6. All system $\left(a\mathrm{,}b\mathrm{,}c\right)$ such that $a^2=b^2=c^2=abc=-1$ is said to be quaternionic system.

Fixing four quaternionic systems $\left(i\mathrm{,}j\mathrm{,}k\right)$, $\left(I\mathrm{,}J\mathrm{,}K\right)$, $\left(l\mathrm{,}m\mathrm{,}n\right)$ and $\left(L\mathrm{,}M\mathrm{,}N\right)$, a basis of the hyperquaternion algebra $ℍ\otimes ℍ\otimes ℍ\otimes ℍ$ can be expressed as follows:

$\left(\mathrm{1,}i\mathrm{,}j\mathrm{,}k\right)\otimes \left(\mathrm{1,}I\mathrm{,}J\mathrm{,}K\right)\otimes \left(\mathrm{1,}l\mathrm{,}m\mathrm{,}n\right)\otimes \left(\mathrm{1,}L\mathrm{,}M\mathrm{,}N\right)\mathrm{.}$ (7)

Each quaternionic system commutes with the three others. A basis of the hyperquaternion algebra ${ℍ}^{\otimes 4}$ contains $4^4=256$ elements.

Explicitly,

$\left(i,j,k\right)\otimes 1\otimes 1\otimes 1=\left(i,j,k\right),1\otimes \left(I,J,K\right)\otimes 1\otimes 1=\left(I,J,K\right),$

$1\otimes 1\otimes \left(l,m,n\right)\otimes 1=\left(l,m,n\right),1\otimes 1\otimes 1\otimes \left(L,M,N\right)=\left(L,M,N\right).$ (8)

An element of $ℍ\otimes ℍ\otimes ℍ\otimes ℍ$ can be expressed in the form

$q=q_0+i{q}_1+j{q}_2+k{q}_3$ (9)

where $q_i\in ℍ\otimes ℍ\otimes ℍ\mathrm{,0}\le i\le 3$, i.e., q can be viewed as a quaternion with coefficients in $ℍ\otimes ℍ\otimes ℍ={ℍ}^{\otimes 3}$. Similarly $q_i$ can be expressed as quaternion with tetraquaternionic coefficients i.e.

$q_i=q_{i0}+I{q}_{i1}+J{q}_{i2}+K{q}_{i3}.$ (10)

Combining the expressions (2) with (3), we obtain

$\begin{array}{c}q=\left(q_{00}+I{q}_{01}+J{q}_{02}+K{q}_{03}\right)+\left(i{q}_{10}+iI{q}_{11}+iJ{q}_{12}+iK{q}_{13}\right)\\ +\left(j{q}_{20}+jI{q}_{21}+jJ{q}_{22}+jK{q}_{23}\right)+\left(k{q}_{30}+kI{q}_{31}+kJ{q}_{32}+kK{q}_{33}\right)\end{array}$ (11)

with $q_{ik}\in ℍ\otimes ℍ\mathrm{,0}\le i\mathrm{,}j\le 3$.

Obviously $q_{ik}$ can represented as a quaternion with quaternionic coefficients,

$q_{ik}=q_{ik0}+l{q}_{ik1}+m{q}_{ik2}+n{q}_{ik3}.$ (12)

Theorefore, q will be expressed as follows:

$\begin{array}{c}q=\left(q_{000}+l{q}_{001}+m{q}_{002}+n{q}_{003}\right)+\left(I{q}_{010}+Il{q}_{011}+Im{q}_{012}+In{q}_{013}\right)\\ +\left(J{q}_{020}+Jl{q}_{021}+Jm{q}_{022}+Jn{q}_{023}\right)+\left(K{q}_{030}+Kl{q}_{031}+Km{q}_{032}+Kn{q}_{033}\right)\\ +\left(i{q}_{100}+il{q}_{101}+im{q}_{102}+in{q}_{103}\right)+\left(iI{q}_{110}+iIl{q}_{111}+i\mathrm{Im}{q}_{112}+iIn{q}_{113}\right)\\ +\left(iJ{q}_{120}+iJl{q}_{121}+iJm{q}_{122}+iJn{q}_{123}\right)+\left(iK{q}_{130}+iKl{q}_{131}+iKm{q}_{132}+iKn{q}_{133}\right)\end{array}$

$\begin{array}{c}+\left(j{q}_{200}+jl{q}_{201}+jm{q}_{202}+jn{q}_{203}\right)+\left(jI{q}_{210}+jIl{q}_{211}+jIm{q}_{212}+jIn{q}_{213}\right)\\ +\left(jJ{q}_{220}+jJl{q}_{221}+jJm{q}_{222}+jJn{q}_{223}\right)+\left(jK{q}_{230}+jKl{q}_{231}+jKm{q}_{232}+jKn{q}_{233}\right)\\ +\left(k{q}_{300}+kl{q}_{301}+km{q}_{302}+kn{q}_{303}\right)+\left(kI{q}_{310}+kIl{q}_{311}+kIm{q}_{312}+kIn{q}_{313}\right)\\ +\left(kJ{q}_{320}+kJl{q}_{321}+kJm{q}_{322}+kJn{q}_{323}\right)+\left(kK{q}_{330}+kKl{q}_{331}+kKm{q}_{332}+kKn{q}_{333}\right).\end{array}$ (13)

with $q_{ikm}\in ℍ\mathrm{,0}\le i\mathrm{,}j\mathrm{,}m\le 3$.

We express the quaternion $q_{ikm}$ with real $q_{ikmn}$ coefficients,

$q_{ikm}=q_{ikm0}+L{q}_{ikm1}+M{q}_{ikm2}+N{q}_{ikm3},$ (14)

where $0\le i\mathrm{,}j\mathrm{,}m\mathrm{,}n\le 3$.

At the last, an element $q\in ℍ$ is a linear combination of 256 elements of a basis of ${ℍ}^{\otimes 4}$,

$\begin{array}{c}q=q_{0000}+L{q}_{0001}+M{q}_{0002}+N{q}_{0003}+l{q}_{0010}+lL{q}_{0011}+lM{q}_{0012}+lN{q}_{0013}\\ +m{q}_{0020}+mL{q}_{0021}+mM{q}_{0022}+mN{q}_{0023}+n{q}_{0030}+nL{q}_{0031}+nM{q}_{0032}\\ +nN{q}_{0033}+I{q}_{0100}+IL{q}_{0101}+IM{q}_{0102}+IN{q}_{0103}+Il{q}_{0110}+IlL{q}_{0111}\\ +IlM{q}_{0112}+IlN{q}_{0113}+Im{q}_{0120}+ImL{q}_{0121}+ImM{q}_{0122}+ImN{q}_{0123}\\ +In{q}_{0130}+InL{q}_{0131}+InM{q}_{0132}+InN{q}_{0133}+J{q}_{0200}+JL{q}_{0201}+JM{q}_{0202}\end{array}$

$\begin{array}{c}+JN{q}_{0203}+Jl{q}_{0210}+JlL{q}_{0211}+JlM{q}_{0212}+JlN{q}_{0213}+JlN{q}_{0213}+Jm{q}_{0220}\\ +JmL{q}_{0221}+JmM{q}_{0222}+JmN{q}_{0223}+Jn{q}_{0230}+JnL{q}_{0231}+JnM{q}_{0232}\\ +JnN{q}_{0233}+K{q}_{0300}+KL{q}_{0301}+KM{q}_{0302}+KN{q}_{0303}+Kl{q}_{0310}+KlL{q}_{0311}\\ +KlM{q}_{0312}+KlN{q}_{0313}+Km{q}_{0320}+KmL{q}_{0321}+KmM{q}_{0322}+KmN{q}_{0323}\\ +Kn{q}_{0330}+KnL{q}_{0331}+KnM{q}_{0332}+KnN{q}_{0333}+i{q}_{1000}+iL{q}_{1001}+iM{q}_{1002}\end{array}$

$\begin{array}{c}+iN{q}_{1003}+il{q}_{1010}+ilL{q}_{1011}+ilM{q}_{1012}+ilN{q}_{1013}+im{q}_{1020}+imL{q}_{1021}\\ +imM{q}_{1022}+imN{q}_{1023}+in{q}_{1030}+inL{q}_{1031}+inM{q}_{1032}+inN{q}_{1033}+iI{q}_{1100}\\ +iIL{q}_{1101}+iIM{q}_{1102}+iIN{q}_{1103}+iIl{q}_{1110}+iIlL{q}_{1111}+iIlM{q}_{1112}+iIlN{q}_{1113}\\ +iIm{q}_{1120}+iImL{q}_{1121}+iImM{q}_{1122}+iImN{q}_{1123}+iIn{q}_{1130}+iInL{q}_{1131}\\ +iInM{q}_{1132}+iInN{q}_{1133}+iJ{q}_{1200}+iJL{q}_{1201}+iJM{q}_{1202}+iJN{q}_{1203}+iJl{q}_{1210}\end{array}$

$\begin{array}{c}+iJlL{q}_{1211}+iJlM{q}_{1212}+iJlN{q}_{1213}+iJm{q}_{1220}+iJmL{q}_{1221}+iJmM{q}_{1222}\\ +iJmN{q}_{1223}+iJn{q}_{1230}+iJnL{q}_{1231}+iJnM{q}_{1232}+iJnN{q}_{1233}+iK{q}_{1300}\\ +iKL{q}_{1301}+iKM{q}_{1302}+iKN{q}_{1303}+iKl{q}_{1310}+iKlL{q}_{1311}+iKlM{q}_{1312}\\ +iKlN{q}_{1313}+iKm{q}_{1320}+iKmL{q}_{1321}+iKmM{q}_{1322}+iKmN{q}_{1323}+iKn{q}_{1330}\\ +iKnL{q}_{1331}+iKnM{q}_{1332}+iKnN{q}_{1333}+j{q}_{2000}+jL{q}_{2001}+jM{q}_{2002}+jN{q}_{2003}\end{array}$

$\begin{array}{c}+jl{q}_{2010}+jlL{q}_{2011}+jlM{q}_{2012}+jlN{q}_{2013}+jm{q}_{2020}+jmL{q}_{2021}+jmM{q}_{2022}\\ +jmN{q}_{2023}+jn{q}_{2030}+jnL{q}_{2031}+jnM{q}_{2032}+jnN{q}_{2033}+jI{q}_{2100}+jIL{q}_{2101}\\ +jIM{q}_{2102}+jIN{q}_{2103}+jIl{q}_{2110}+jIlL{q}_{2111}+jIlM{q}_{2112}+jIlN{q}_{2113}+jIm{q}_{2120}\\ +jImL{q}_{2121}+jImM{q}_{2122}+jImN{q}_{2123}+jIn{q}_{2130}+jInL{q}_{2131}+jInM{q}_{2132}\\ +jInN{q}_{2133}+jJ{q}_{2200}+jJL{q}_{2201}+jJM{q}_{2202}+jJN{q}_{2203}+jJl{q}_{2210}+jJlL{q}_{2211}\end{array}$

$\begin{array}{c}+jJlM{q}_{2212}+jJlN{q}_{2213}+jJm{q}_{2220}+jJmL{q}_{2221}+jJmM{q}_{2222}+jJmN{q}_{2223}\\ +jJn{q}_{2230}+jJnL{q}_{2231}+jJnM{q}_{2232}+jJnN{q}_{2233}+jK{q}_{2300}+jKL{q}_{2301}\\ +jKM{q}_{2302}+jKN{q}_{2303}+jKl{q}_{2310}+jKlL{q}_{2311}+jKlM{q}_{2312}+jKlN{q}_{2313}\\ +jKm{q}_{2320}+jKmL{q}_{2321}+jKmM{q}_{2322}+jKmN{q}_{2323}+jKn{q}_{2330}+jKnL{q}_{2331}\\ +jKnM{q}_{2332}+jKnN{q}_{2333}+k{q}_{3000}+kL{q}_{3001}+kM{q}_{3002}+kN{q}_{3003}+kl{q}_{3010}\end{array}$

$\begin{array}{c}+klL{q}_{3011}+klM{q}_{3012}+klN{q}_{3013}+km{q}_{3020}+kmL{q}_{3021}+kmM{q}_{3022}+kmN{q}_{3023}\\ +kn{q}_{3030}+knL{q}_{3031}+knM{q}_{3032}+knN{q}_{3033}+kI{q}_{3100}+kIL{q}_{3101}+kIM{q}_{3102}\\ +kIN{q}_{3103}+kIl{q}_{3110}+kIlL{q}_{3111}+kIlM{q}_{3112}+kIlN{q}_{3113}+kIm{q}_{3120}+kImL{q}_{3121}\\ +kImM{q}_{3122}+kImN{q}_{3123}+kIn{q}_{3130}+kInL{q}_{3131}+kInM{q}_{3132}+kInN{q}_{3133}\\ +kJ{q}_{3200}+kJL{q}_{3201}+kJM{q}_{3202}+kJN{q}_{3203}+kJl{q}_{3210}+kJlL{q}_{3211}+kJlM{q}_{3212}\end{array}$

$\begin{array}{c}+kJlN{q}_{3213}+kJm{q}_{3220}+kJmL{q}_{3221}+kJmM{q}_{3222}+kJmN{q}_{3223}+kJn{q}_{3230}\\ +kJnL{q}_{3231}+kJnM{q}_{3232}+kJnN{q}_{3233}+kK{q}_{3300}+kKL{q}_{3301}+kKM{q}_{3302}\\ +kKN{q}_{3303}+kKl{q}_{3310}+kKlL{q}_{3311}+kKlM{q}_{3312}+kKlN{q}_{3313}+kKm{q}_{3320}\\ +kKnN{q}_{3333}\mathrm{.}\end{array}$ (15)

Definition 2.7. Let ${ℍ}^{\otimes 4}$ be a hyperquaternion algebra, the product of two elements of ${ℍ}^{\otimes 4}$ is the product in a tensor product of quaternion algebras, it is called hyperquaternion product of ${ℍ}^{\otimes 4}$.

Note that the hyperquaternion product, of ${ℍ}^{\otimes 4}$, is defined independently of the choice of generators of the Clifford algebra $C{l}_{\mathrm{5,3}}$ [2].

Since the dimension of the hyperquaternion algebra ${ℍ}^{\otimes 4}$ is very large, it would be desirable to use the computer to perform the calculations in this algebra ( $dim{ℍ}^{\otimes 4}=256$ ).

3. Multivector Structure of ${ℍ}^{\otimes 4}$

The principal operations in the hyperquaternion algebra ${ℍ}^{\otimes 4}$ (interior product, exterior product, duality, ...) are defined from its multivector structure which depends on generators but the hyperquaternion product is independent of the choice of generators.

3.1. Isomorphism $C{l}_{5,3}\simeq {ℍ}^{\otimes 4}$

In order to establish the expected result in this section, we use the isomorphism

$C{l}_{\mathrm{2,0}}\simeq C{l}_{\mathrm{1,1}}\simeq ℝ\left(2\right)\mathrm{,}$ (16)

and the isomorphism between the hyperquaternion algebra of tetraquaternions and the algebra of 4 × 4-matrices with entries in $ℝ$ and the below two lemmas.

Lemma 3.1. Let $C{l}_{p\mathrm{,}q}$ be a Clifford algebra associated with the quadratic space ${ℝ}^{p\mathrm{,}q}$. Then the following isomorphism holds

$C{l}_{p+\mathrm{1,}q+1}\simeq C{l}_{p\mathrm{,}q}\otimes C{l}_{\mathrm{1,1}}\mathrm{,}$ (17)

where either $p>0$ or $q>0$, and $\otimes$ denotes the usual tensor product.

Proof. The entirety of the proof can be seen in [10], p.90. $■$

Lemma 3.2. If m and n are positive integers then

$ℝ\left(m\right)\otimes ℝ\left(n\right)\simeq ℝ\left(mn\right)\mathrm{,}$ (18)

where $ℝ\left(n\right)$ designs the algebra of $n\times n$ -matrices with entries in $ℝ$.

Proof. The entirety of the proof can be seen in [5], p.378 and a modern proof can be found in [2], p.3. $■$

Theorem 3.3. Let $ℍ$ be the quaternion algebra, the Clifford algebra $C{l}_{\mathrm{5,3}}$ is isomorphic to the four fold-tensor products ${ℍ}^{\otimes 4}$.

Proof. We recall first the isomorphism $C{l}_{\mathrm{1,1}}\simeq ℝ\left(2\right)$ which in combination with the lemma (3.1) leads to

$C{l}_{p+\mathrm{1,}q+1}\simeq C{l}_{p\mathrm{,}q}\otimes ℝ\left(2\right)\mathrm{.}$ (19)

Using the last isomorphism, we set

$C{l}_{\mathrm{5,3}}\simeq C{l}_{\mathrm{4,2}}\otimes ℝ\left(2\right)\mathrm{,}$ (20)

$C{l}_{\mathrm{4,2}}\simeq C{l}_{\mathrm{3,1}}\otimes ℝ\left(2\right)\mathrm{,}$ (21)

$C{l}_{\mathrm{3,1}}\simeq C{l}_{\mathrm{2,0}}\otimes ℝ\left(2\right)\mathrm{.}$ (22)

The substitution of (21) into (20) leads to

$C{l}_{\mathrm{5,3}}\simeq \left(C{l}_{\mathrm{3,1}}\otimes ℝ\left(2\right)\right)\otimes ℝ\left(2\right)\mathrm{,}$ (23)

and the substitution of (22) into (23) gives

$C{l}_{\mathrm{5,3}}\simeq \left[\left(C{l}_{\mathrm{2,0}}\otimes ℝ\left(2\right)\right)\otimes ℝ\left(2\right)\right]\otimes ℝ\left(2\right)\mathrm{.}$ (24)

From the isomorphism $C{l}_{\mathrm{2,0}}\simeq ℝ\left(2\right)$ and the associativity of the tensor product, we can write

$C{l}_{\mathrm{5,3}}\simeq \left(ℝ\left(2\right)\otimes ℝ\left(2\right)\right)\otimes \left(ℝ\left(2\right)\otimes ℝ\left(2\right)\right)\mathrm{.}$ (25)

In virtue of the second lemma above, we obtain

$C{l}_{\mathrm{5,3}}\simeq \left(ℝ\left(4\right)\otimes ℝ\left(4\right)\right)\mathrm{.}$ (26)

Finally, the isomorphism $ℍ\otimes ℍ\simeq ℝ\left(4\right)$ induces

$C{l}_{\mathrm{5,3}}\simeq \left(ℍ\otimes ℍ\right)\otimes \left(ℍ\otimes ℍ\right)\mathrm{.}$ (27)

Hence,

$C{l}_{\mathrm{5,3}}\simeq ℍ\otimes ℍ\otimes ℍ\otimes ℍ\mathrm{.}$ (28)

The Clifford algebra $C{l}_{\mathrm{5,3}}$ generated by $e_1\mathrm{,}{e}_2\mathrm{,}{e}_3\mathrm{,}{e}_4\mathrm{,}{e}_5\mathrm{,}{e}_6\mathrm{,}{e}_7$ and $e_8$ is isomorphic to the hyperquaternion algebra ${ℍ}^{\otimes 4}$. $■$

According to the isomorphism $C{l}_{\mathrm{5,3}}\simeq {ℍ}^{\otimes 4}$, we make the following choice of the eight generators of the hyperquaternion algebra ${ℍ}^{\otimes 4}$ :

$\begin{array}{l}{e}_1\mapsto k\otimes I\otimes 1\otimes 1=kI\mathrm{,}\\ e_2\mapsto k\otimes J\otimes 1\otimes 1=kJ\mathrm{,}\\ e_3\mapsto k\otimes K\otimes n\otimes L=kKnL\mathrm{,}\\ e_4\mapsto k\otimes K\otimes n\otimes M=kKnM\mathrm{,}\end{array}$

$\begin{array}{l}{e}_5\mapsto k\otimes K\otimes n\otimes N=kKnN\mathrm{,}\\ e_6\mapsto k\otimes K\otimes l\otimes 1=kKl\mathrm{,}\\ e_7\mapsto k\otimes K\otimes m\otimes 1=kKm\mathrm{,}\\ e_8\mapsto j\otimes 1\otimes 1\otimes 1=j\mathrm{.}\end{array}$ (29)

We opt for the identification of the basis vectors generators of ${ℍ}^{\otimes 4}\simeq C{l}_{\mathrm{5,3}}$ below:

$\begin{array}{l}{e}_1=kI,e_2=kJ,e_3=kKnL,e_4=kKnM,\\ e_5=kKnN,e_6=kKl,e_7=kKm,e_8=j.\end{array}$ (30)

It is easy to show that the hyperquaternion algebra ${ℍ}^{\otimes 4}$ is isomorphic to the set of real matrices $ℝ\left(16\right)$. Since $ℍ\simeq ℝ\left(2\right)$, it is obvious that

${ℍ}^{\otimes 4}=ℍ\otimes ℍ\otimes ℍ\otimes ℍ=ℝ\left(2\right)\otimes ℝ\left(2\right)\otimes ℝ\left(2\right)\otimes ℝ\left(2\right)=ℝ\left(16\right)\mathrm{.}$ (31)

3.2. Multivector Structure of $ℍ\otimes ℍ\otimes ℍ\otimes ℍ$

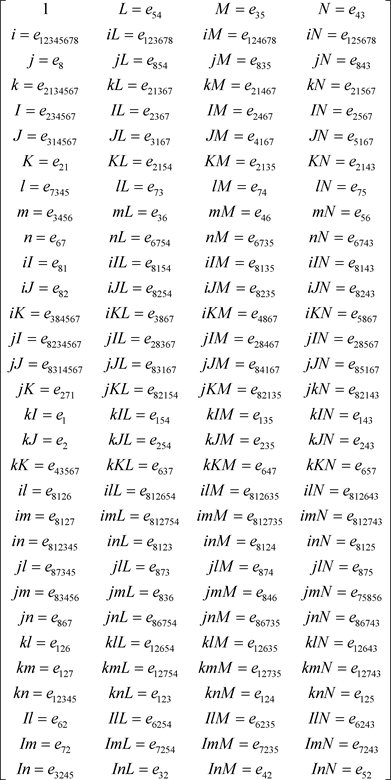
Definition 3.4. The product of k generators of the hyperquaternion algebra ${ℍ}^{\otimes 4}$ is called multivector of rank k or polyvector of rank k or k-vector.

We denote by $e_1{e}_2{e}_3\cdots e_k=e_{123\cdots k}$ the product of k vectors $e_1\mathrm{,}{e}_2\mathrm{,}{e}_3\mathrm{,}{e}_4\mathrm{,}\cdots \mathrm{,}{e}_k$. As shown in the table below describing the multivector structure of the hyperquaternion algebra ${ℍ}^{\otimes 4}=ℍ\otimes ℍ\otimes ℍ\otimes ℍ$, a basis of it has:

1 scalar (or 0-vector): 1,

8 vectors (or 1-vectors):

$\begin{array}{l}{e}_1=kI,e_2=kJ,e_3=kKnL,e_4=kKnM,e_5=kKnN,\\ e_6=kKl,e_7=kKm\text{and}{e}_8=j,\end{array}$

28 bivectors (or 2-vectors):

$\begin{array}{l}{e}_{81}=iI,e_{17}=Jm,e_{16}=Jl,e_{15}=JnN,e_{14}=JnM,e_{13}=JnL,e_{21}=K,e_{32}=InL,\\ e_{42}=InM,e_{52}=InN,e_{62}=Il,e_{72}=Im,e_{82}=iJ,e_{43}=N,e_{35}=M,e_{36}=mL,\\ e_{73}=lL,e_{83}=iKnL,e_{54}=L,e_{46}=mM,e_{74}=lM,e_{84}=iKnM,e_{56}=mN,\\ e_{75}=lN,e_{85}=iKnN,e_{67}=n,e_{86}=iKl\text{and}{e}_{87}=iKm,\end{array}$

56 trivectors (or 3-vectors):

$\begin{array}{l}{e}_{345}=kKn,e_{754}=kKmL,e_{735}=kkmM,e_{743}=kKnN,e_{654}=kKlL,\\ e_{236}=kJmL,e_{143}=kIN,\cdots \end{array}$

70 quadrivectors (or 4-vectors):

$e_{2367}=IL,e_{3167}=JL,e_{2154}=KL,e_{2467}=IM,e_{3867}=iKL,e_{8254}=iJL,e_{8154}=iIL,\cdots$

56 multivectors of rank 5:

$\begin{array}{l}{e}_{23456}=kJm,e_{73452}=kJl,e_{21467}=kM,e_{21567}=kN,e_{28367}=jIL,\\ e_{82154}=jKL,e_{83167}=jJL,\cdots \end{array}$

28 multivectors of rank 6:

$e_{123678}=iL,e_{124678}=iM,e_{125678}=iN,e_{812654}=ilL,e_{812754}=imL,\cdots$

8 multivectors of rank 7:

$\begin{array}{l}{e}_{2134567}=k,e_{8234567}=jI,e_{8314567}=jJ,e_{8217345}=jKl,e_{8213456}=jKm,\\ e_{8216754}=jKnL,e_{8216735}=jKnM,e_{8216743}=jKnN\end{array}$

1 pseudoscalar: $e_{12345678}=i$.

It is obvious that ${\displaystyle {\sum }_{k=0}^8}\left(\begin{array}{c}8\\ 6\end{array}\right)=2^8=256$ is the dimension of the hyperquaternion algebra ${ℍ}^{\otimes 4}$ and a general element of this algebra is a linear combination of the 256 basis multivectors as in (15).

$\left[\begin{array}{cccc}jIl=e_{862}& jIlL=e_{86254}& jIlM=e_{86235}& jIlN=e_{86243}\\ jIm=e_{872}& jImL=e_{87254}& jImM=e_{87235}& jImN=e_{87243}\\ jIn=e_{83245}& jInL=e_{832}& jInM=e_{842}& jInN=e_{852}\\ jJl=e_{186}& jJlL=e_{18654}& jJlM=e_{18635}& jJlN=e_{18643}\\ jJm=e_{187}& jJmL=e_{18754}& jJmM=e_{18735}& jJmN=e_{18743}\\ jJn=e_{81345}& jJnL=e_{813}& jJnM=e_{814}& jJnN=e_{815}\\ jKl=e_{8217345}& jKlL=e_{82173}& jKlM=e_{82174}& jKlN=e_{82175}\\ jKm=e_{8213456}& jKmL=e_{82136}& jKmM=e_{82146}& jKmN=e_{82156}\\ jKn=e_{82167}& jKnL=e_{8216754}& jKnM=e_{8216735}& jKnN=e_{8216743}\\ kIl=e_{73451}& kIlL=e_{731}& kIlM=e_{741}& kIlN=e_{751}\\ kIm=e_{13456}& kImL=e_{136}& kImM=e_{146}& kImN=e_{156}\\ kIn=e_{167}& kInL=e_{16754}& kInM=e_{16735}& kInN=e_{16743}\\ kJl=e_{73452}& kJlL=e_{732}& kJlM=e_{742}& kJlN=e_{752}\\ kJm=e_{23456}& kJmL=e_{236}& kJmM=e_{246}& kJmN=e_{256}\\ kJn=e_{267}& kJnL=e_{26754}& kJnM=e_{26735}& kJnN=e_{26743}\\ kKl=e_6& kKlL=e_{654}& kKlM=e_{635}& kKlN=e_{643}\\ kKm=e_7& kKmL=e_{754}& kKmM=e_{735}& kKmN=e_{743}\\ kKn=e_{345}& kKnL=e_3& kKnM=e_4& kKnN=e_5\end{array}\right]$

4. Hyperquaternion Algebra for Conics

In this section, we relate the conic sections expressed in CCGA (Conic Conformal Geometric Algebra) developed in [7], [8] and [11] to their hyperquaternion Clifford algebra presentation.

4.1. Conformal Hyperquaternion Algebra ${ℍ}^{\otimes 4}$

Firstly, we recap of what we have done above by recalling that the hyperquaternion algebra $ℍ\otimes ℍ\otimes ℍ\otimes ℍ$ is generated by the following selected basis of the vector space ${ℝ}^{\mathrm{5,3}}$ :

$\begin{array}{l}{e}_1=kI,e_2=kJ,e_3=kKnM,e_4=kKnN,\\ e_5=kKnL,e_6=kKl,e_7=kKm,e_8=j.\end{array}$

Consider now the three first null vectors called the infinity’s points and defined from the six vectors $e_3\mathrm{,}{e}_4\mathrm{,}{e}_5\mathrm{,}{e}_6\mathrm{,}{e}_7$ and $e_8$ as follows:

$e_{\infty 1}=\frac{1}{\sqrt{2}}\left(kKnL+kKm\right),e_{\infty 2}=\frac{1}{\sqrt{2}}\left(kKnM+kKl\right),e_{\infty 3}=\frac{1}{\sqrt{2}}\left(kKnN+j\right).$ (32)

The three others null vectors, called the origins points, are

$e_{01}=\frac{1}{\sqrt{2}}\left(-kKnL+kKm\right),e_{02}=\frac{1}{\sqrt{2}}\left(-kKnM+kKl\right),e_{03}=\frac{1}{\sqrt{2}}\left(-kKnN+j\right).$ (33)

So, we built a new basis $\left(e_1\mathrm{,}{e}_2\mathrm{,}{e}_{\infty 1}\mathrm{,}{e}_{\infty 2}\mathrm{,}{e}_{\infty 3}\mathrm{,}{e}_{01}\mathrm{,}{e}_{02}\mathrm{,}{e}_{03}\right)$ of the vector space ${ℝ}^{\mathrm{5,3}}$ composed of the Euclidean basis $\left(e_1\mathrm{,}{e}_2\right)$ of ${ℝ}^2$ and the six null vectors $e_{\infty i}$ and $e_{0i}$, where $1\le i\le 3$.

The new choice of the generators ( $e_1\mathrm{,}{e}_2\mathrm{,}{e}_{\infty 1}\mathrm{,}{e}_{\infty 2}\mathrm{,}{e}_{\infty 3}\mathrm{,}{e}_{01}\mathrm{,}{e}_{02}$ and $e_{03}$ ) respects the hyperquaternion product of ${ℍ}^{\otimes 4}$ which is defined independently of any specific choice of the generators and the multivector structure of ${ℍ}^{\otimes 4}$ changes.

Definition 4.1. Let $ℍ$ be a quaternion algebra, the hyperquaternion algebra, generated by the basis vectors $e_1\mathrm{,}{e}_2\mathrm{,}{e}_{\infty 1}\mathrm{,}{e}_{\infty 2}\mathrm{,}{e}_{\infty 3}\mathrm{,}{e}_{01}\mathrm{,}{e}_{02}$ and $e_{03}$ of the vector space ${ℝ}^{\mathrm{5,3}}$ , is called the conformal hyperquaternion algebra $ℍ\otimes ℍ\otimes ℍ\otimes ℍ$.

Note that the conformal hyperquaternion algebra $ℍ\otimes ℍ\otimes ℍ\otimes ℍ$ is the hyperquatenion algebra ${ℍ}^{\otimes 4}$ with another multivector structure and the hyperquaternion product is the same in the two algebras.

We perform easily the inner product of the generators $e_1\mathrm{,}{e}_2\mathrm{,}{e}_{\infty 1}\mathrm{,}{e}_{\infty 2}\mathrm{,}{e}_{\infty 3}\mathrm{,}{e}_{01},e_{02}$ and $e_{03}$,

$e_1^2=\left(kI\right)\left(kI\right)=k^2{I}^2={\left(-1\right)}^2=1,e_2^2=\left(kJ\right)\left(kJ\right)=k^2{J}^2={\left(-1\right)}^2=1,$ (34)

each generator $e_{\infty i}^2,e_{0i}^2\left(1\le i\le 3\right)$ is isotropic, it squares to zero

$\begin{array}{c}{e}_{\infty 1}^2=\frac{1}{\sqrt{2}}\left(kKnL+kKm\right)\frac{1}{\sqrt{2}}\left(kKnL+kKm\right)\\ =\frac{1}{2}\left(k^2{K}^2{n}^2{L}^2+k^2{K}^2{m}^2+k^2{K}^2\left(mn\right)L+k^2{K}^2\left(mn\right)L\right)\\ =\frac{1}{2}\left(1-1+k^2{K}^2\left(nm\right)L-k^2{K}^2\left(nm\right)L\right)=0.\end{array}$ (35)

$\begin{array}{c}{e}_{\infty 2}^2=\frac{1}{\sqrt{2}}\left(kKnM+kKl\right)\frac{1}{\sqrt{2}}\left(kKnM+kKl\right)\\ =\frac{1}{2}\left(k^2{K}^2{n}^2{M}^2+k^2{K}^2{l}^2+k^2{K}^2\left(nl\right)M+k^2{K}^2\left(ln\right)M\right)\\ =\frac{1}{2}\left(1-1+k^2{K}^2\left(nl\right)M-k^2{K}^2\left(nl\right)M\right)=0.\end{array}$ (36)

$\begin{array}{c}{e}_{\infty 3}^2=\frac{1}{\sqrt{2}}\left(kKnN+j\right)\frac{1}{\sqrt{2}}\left(kKnN+j\right)\\ =\frac{1}{2}\left(k^2{K}^2{n}^2{N}^2+\left(kj\right)KnN+\left(jk\right)KnN{k}^2+j^2\right)\\ =\frac{1}{2}\left(1-iKnN+iKnN-1\right)=0.\end{array}$ (37)

It is easy to establish the following

$e_{01}^2={\left[\frac{1}{\sqrt{2}}\left(-kKnL+kKm\right)\right]}^2=0,$ (38)

$e_{02}^2={\left[\frac{1}{\sqrt{2}}\left(-kKnM+kKl\right)\right]}^2=0$ (39)

$e_{03}^2={\left[\frac{1}{\sqrt{2}}\left(-kKnN+j\right)\right]}^2=0.$ (40)

We recall that for any vectors u and v, their inner product can be written $u\cdot v=\frac{1}{2}\left(uv+vu\right)$. By using this last relation, we compute the following

$\begin{array}{c}{e}_{\infty 1}\cdot e_{01}=\frac{1}{2}\{\frac{1}{\sqrt{2}}\left(kKnL+kKm\right)\frac{1}{\sqrt{2}}\left(-kKnL+kKm\right)\\ +\frac{1}{\sqrt{2}}\left(-kKnL+kKm\right)\frac{1}{\sqrt{2}}\left(kKnL+kKm\right)\}\end{array}$

$\begin{array}{c}=\frac{1}{4}(-k^2{K}^2{n}^2{L}^2+k^2{K}^2{m}^2+k^2{K}^2\left(nm\right)L-k^2{K}^2\left(nm\right)L\\ -k^2{K}^2{n}^2{L}^2+k^2{K}^2{m}^2-k^2{K}^2\left(nm\right)L+k^2{K}^2\left(nm\right)L)\\ =\frac{1}{4}\left(-1-1-lL-lL-1-1+lL+lL\right)=-1.\end{array}$ (41)

Similarly, we establish the following,

$e_{\infty 2}\cdot e_{02}=e_{\infty 3}\cdot e_{03}=-1.$ (42)

Thus for any $i\in \left\{\mathrm{1,2,3}\right\}$,

$e_{\infty i}\cdot e_{0i}=e_{0i}\cdot e_{\infty 1}=-1.$ (43)

We define two others null vectors,

$e_{\infty }=\frac{1}{2}\left(e_{\infty 1}+e_{\infty 2}\right)=\frac{1}{2\sqrt{2}}\left(kKnL+kKm+kKnM+kKl\right).$ (44)

and

$e_0=e_{01}+e_{02}=\frac{1}{\sqrt{2}}\left(-kKnL+kKm-kKnM+kKl\right).$ (45)

We can easily prove that the vectors $e_{\infty }$ and $e_0$ are isotropics i.e.

$e_{\infty }^2=\frac{1}{8}{\left(kKnL+kKm+kKnM+kKl\right)}^2=0$ (46)

$e_0^2=\frac{1}{2}{\left(-kKnL+kKm-kKnM+kKl\right)}^2=0$ (47)

and their inner product is

$e_{\infty }\cdot e_0=\frac{1}{4}\left(kKnL+kKm+kKnM+kKl\right)\cdot \left(-kKnL+kKm-kKnM+kKl\right)=-1.$ (48)

In the following subsection, we use the fact that the Clifford algebra $C{l}_{\mathrm{5,3}}$ is the geometric algebra for conic (CGA) and the isomorphism $C{l}_{\mathrm{5,3}}\simeq {ℍ}^{\otimes 4}$ to provide the hyperquaternion formulation of conic sections.

4.2. Hyperquaternion Representations IPNS

Consider the conformal embedding $\varphi \mathrm{<mo>\; :\; </mo>}{ℝ}^2\to Im\varphi \subset {ℝ}^{\mathrm{5,3}}$,

$\begin{array}{l}X=xkI+ykJ\\ \mapsto \varphi \left(X\right)=xkI+ykJ+\frac{1}{2\sqrt{2}}{x}^2\left(kKnL+kKm\right)+\frac{1}{2\sqrt{2}}{y}^2\left(kKnM+kKl\right)\\ +\frac{1}{\sqrt{2}}xy\left(kKnN+j\right)+\frac{1}{\sqrt{2}}\left(-kKnL+kKm\right)+\frac{1}{\sqrt{2}}\left(-kKnM+kKl\right)\end{array}$ (49)

Proposition 4.2. Let $\left(kI\mathrm{,}kJ\right)$ be a basis of the Euclidean vector space ${ℝ}^2$ , $\varphi \mathrm{<mo>\; :\; </mo>}{ℝ}^2\to Im\varphi \subset {ℝ}^{\mathrm{5,3}}$ be an embedding and $X=xkI+ykJ\in {ℝ}^2$ , the embedded point $\varphi \left(X\right)$ of ${ℝ}^{\mathrm{5,3}}\subset {ℍ}^{\otimes 4}$ is isotropic.

Proof. It is obvious, a straightforward calculations of the inner product give the result $\varphi \left(X\right)\cdot \varphi \left(X\right)=0$

This result confirms the fact that in conformal geometric algebra (CGA), the inner product of any point with itself is zero.

Proposition 4.3. Let $\left(kI\mathrm{,}kJ\right)$ be a basis of the Euclidean vector space ${ℝ}^2$ , $\varphi \mathrm{<mo>\; :\; </mo>}{ℝ}^2\to Im\varphi \subset {ℝ}^{\mathrm{5,3}}$ be an embedding and $X=xkI+ykJ\in {ℝ}^2$ , the subspace of dimension 1 generated by the null vector $e_{\infty 3}=\frac{1}{\sqrt{2}}\left(kKnN+j\right)$ is orthogonal to any embedded point $\varphi \left(X\right)$ of ${ℝ}^{\mathrm{5,3}}\subset {ℍ}^{\otimes 4}$.

Proof. For any $X=xkI+ykJ\in {ℝ}^2$ a straightforward computation of the inner product show the relation $\varphi \left(X\right)\cdot e_{\infty 3}=0$. $■$

Definition 4.4. Let X be an element of the Euclidean space ${ℝ}^2$ , A an 1-blade of the hyperquaternion algebra ${ℍ}^{\otimes 4}$ and the conformal embedding $\varphi \mathrm{<mo>\; :\; </mo>}{ℝ}^2\to Im\varphi \subset {ℝ}^{\mathrm{5,3}}$ , the inner product null space of A, denoted by $IPNS\left(A\right)$ , is defined as follows $IPNS\left(A\right)=\left\{X\mathrm{<mo>\; :\; </mo>}\varphi \left(X\right)\cdot A=0\right\}$.

In order the define the inner product null space of an 1-blade

$\begin{array}{l}A=\frac{a}{\sqrt{2}}\left(-kKnL+kKm\right)+\frac{b}{\sqrt{2}}\left(-kKnM+kKl\right)+\frac{c}{\sqrt{2}}\left(-kKnN+j\right)\\ +dkI+ekJ+\frac{g}{\sqrt{2}}\left(kKnL+kKm\right)+\frac{h}{\sqrt{2}}\left(kKnM+kKl\right)\\ +\frac{i}{\sqrt{2}}\left(kKnN+j\right)\in {ℝ}^{\mathrm{5,3}}\subset {ℍ}^{\otimes 4}\mathrm{.}\end{array}$ (50)

we perform the inner product of $\varphi \left(X\right)$ and A as expressed above,

$\varphi \left(X\right)\cdot A=dx+ey-\frac{a}{2}{x}^2-\frac{b}{2}{y}^2-cxy-g-h\mathrm{.}$ (51)

the inner product null space of A is the set,

$IPNS\left(A\right)=\left\{X\mathrm{<mo>\; :\; </mo>}dx+ey-\frac{a}{2}{x}^2-\frac{b}{2}{y}^2-cxy-g-h=0\right\}$ (52)

and the geometric entity corresponding to the above equation,

$dx+ey-\frac{a}{2}{x}^2-\frac{b}{2}{y}^2-cxy-g-h=0$ (53)

is a conic section.

An elegant equation of a conic section is given by

$A{x}^2+B{y}^2+2Cxy+2Dx+2Ey+F=0$ (54)

obtained by laying $A=-a,B=-b,C=-c,D=d,E=e$ and $F=-2\left(g+h\right)$.

Conflicts of Interest

The author declares no conflicts of interest regarding the publication of this paper.

References

[1]	Girard, P.R., Clarysse, P., Pujol, R., Wang, L. and Delacharte, P. (2015) Differential Geometry Revisited by Biquaternion Clifford Algebra. In: Boissonnat, J.-D., et al., Eds., Curves and Surfaces, Springer, Cham, 216-242. https://doi.org/10.1007/978-3-319-22804-4_17
[2]	Girard, P.R., Clarysse, P., Pujol, R., Goutte, R. and Delacharte, P. (2018) Hyperquaternions: A New Tool for Physics. Advances in Applied Clifford Algebras, 28, Article No. 68. https://doi.org/10.1007/s00006-018-0881-8
[3]	Girard, P.R., Clarysse, P., Pujol, R., Goutte, R. and Delacharte, P. (2021) Dual Hyperquaternion Poincaré Groups. Advances in Applied Clifford Algebras, 31, Article No. 15. https://doi.org/10.1007/s00006-021-01120-z
[4]	Girard, P.R., Clarysse, P., Pujol, R., Goutte, R. and Delacharte, P. (2021) Hyperquaternion Conformal Groups. Advances in Applied Clifford Algebras, 31, Article No. 56. https://doi.org/10.1007/s00006-021-01159-y
[5]	Lagally, M. (1976) Vorlesungen Uber Vektorrechnung. 5th Edition, Akademische Verlagsgesellschaft, Leipzig, 378.
[6]	Sprobig, W. (2020) Some New Aspects in hypercomplex analysis, Advancements in Complex Analysis. Springer Nature, 497-498.
[7]	Hitzer, E. and Sangwine, S.J. (2019) Foundations of Conic Conformal Geometric Algebra and Compact Versors for Rotation, Translation and Scaling. Advances in Applied Clifford Algebras, 29, Article No. 96. https://doi.org/10.1007/s00006-019-1016-6
[8]	Hrdina, J., Navrat, A. and Vasik, P. (2018) Geometric Algebra for Conics. Advances in Applied Clifford Algebras, 28, Article No. 66. https://doi.org/10.1007/s00006-018-0879-2
[9]	Girard, P.R., Clarysse, P., Pujol, R., Goutte, R. and Delacharte, P. (2019) Hyperquaternions: An Efficient Mathematical Formalism for Geometry. In: Nielsen, F. and Barbaresco, F., Eds., Geometric Science of Information, Springer, Cham, 116-125. https://doi.org/10.1007/978-3-030-26980-7_13
[10]	Vaz, J. and da Rocha, R. (2016) An Introduction to Clifford Algebras and Spinors. Oxford University Press, Oxford. https://doi.org/10.1093/acprof:oso/9780198782926.001.0001
[11]	Perwass, C. (2008) Geometric Algebra with Applications in Engineering. Springer, Heidelberg, 193-194.