Q-Theory: A Connection between Newton’s Law and Coulomb’s Law?

Christopher Pilot

Physics, Maine Maritime Academy, Castine, ME, USA (Retired).

DOI: 10.4236/jhepgc.2021.72037                

Abstract

Assuming a Winterberg model for space where the vacuum consists of a very stiff two-component superfluid made up of positive and negative mass planckions, Q theory is the hypothesis, that Planck charge, q_pl, was created at the same time as Planck mass. Moreover, the repulsive force that like-mass planckions experience is, in reality, due to the electrostatic force of repulsion between like charges. These forces also give rise to what appears to be a gravitational force of attraction between two like planckions, but this is an illusion. In reality, gravity is electrostatic in origin if our model is correct. We determine the spring constant associated with planckion masses, and find that, , where ζ(3) equals Apery’s constant, 1.202 …, and, n₊(0)=n_(0), is the relaxed, i.e., , number density of the positive and negative mass planckions. In the present epoch, we estimate that, n₊(0) equals, 7.848E54 m^-3, and the relaxed distance of separation between nearest neighbor positive, or negative, planckion pairs is, l₊(0)=l_{_}(0)=5.032E-19 meters. These values were determined using box quantization for the positive and negative mass planckions, and considering transitions between energy states, much like as in the hydrogen atom. For the cosmos as a whole, given a net smeared macroscopic gravitational field of, , due to all the ordinary, and bound, matter contained within the observable universe, an average displacement from equilibrium for the planckion masses is a mere 7.566E-48 meters, within the vacuum made up of these particles. On the surface of the earth, where, g=9.81m/s², the displacement amounts to, 7.824E-38 meters. All of these displacements are due to increased gravitational pressure within the vacuum, which in turn is caused by applied gravitational fields. The gravitational potential is also derived and directly related to gravitational pressure.

Keywords

Extended Gravity, Winterberg Model, Planckion Mass Theory, Modified Quantum Vacuum, Planck Charge, Newton’s Law, Coulomb’s Law, Dark Energy

1. Introduction

Students of physics I and II, as well as professional physicists, have long been intrigued by a possible connection between Newton’s law and Coulomb’s law. Some similarities are that they are both inverse square laws, they both depend on the product, (M₁M₂) or (Q₁Q₂), and they both involve coupling constants, G for Newton’s law, and $k=1/\left(4\pi {\epsilon }_0\right)$, for Coulomb’s law. Some notable differences are that the gravitational force is only attractive, whereas the electrostatic force is both attractive and repulsive. Second, only positive mass exists whereas two species of charge exist, positive and negative. Third, positive masses attract, but like charges repel, and unlike charges attract. Fourth, and perhaps most mysterious, is the strength of these forces. The k value in electrostatics is so much larger than G in gravistatics, in reduced units where factors such as, ħ and c are set equal to one.

The fact that Newton’s constant, G, is so weak, has impressed many notable physicists, including Dirac and Jordan. Dirac [1] [2] [3], already in 1936, in his large number hypothesis (LNH), forwarded the notion that G is not really a true constant of nature, but actually varies with cosmological time. Jordan [4] [5] [6] [7], in short order, related $\text{'}G/G$ to Hubble’s constant, $H=a/a\text{'}$, where the dot refers to a variation with respect to cosmological time. The, $a=R/R_0=T_0/T={\left(1+z\right)}^{-1}$, refers to the cosmic scale parameter. The, R, is the Hubble radius, the, T, the CBR temperature, and the, z, stands for the redshift. All the variables with a subscript, “0”, refer to the present epoch, and we are using the convention where, $a_0=1$. All the variables, without a subscript, refer to a different cosmological era. Coming back to Jordan, he claimed that, $\text{'}G/G=-H$. He further recognized that G must now be related to a scalar field, $\phi$, within a year of Dirac’s LNH. We claim that if G does vary cosmologically, then, $M_{Pl}^2=\hslash c{G}^{-1}=\langle 0|{\phi }^2|0\rangle$, where, $\langle 0|{\phi }^2|0\rangle$, is the vacuum expectation value of, ${\phi }^2$. In this formulation, G is an intrinsic property of the vacuum. We call, $M_{Pl}$, the Planck mass because for a constant, $G=G_0$, we obtain the familiar Planck mass, $M_{Pl}={\left(\hslash c/G_0\right)}^{1/2}=2.176\text{E}-8\text{kg}$. We identify Jordan’s scalar field with the, $\phi$, in the above equation [8] [9]. The, $M_{Pl}^2$, above is no longer a constant if G does vary with cosmological time. The $M_{Pl}$ equals the mass of the positive mass planckion, and the negative mass planckion has negative this value. We leave open the possibility that Newton’s constant, G, does indeed vary, and thus the planckion masses would evolve cosmologically with time.

Recently this author [10], considered the Friedmann equation in light of a time varying gravitational constant. Two models for, $G=G\left(a\right)$, were presented where, a, is the cosmic scale parameter, defined above. In the present epoch, the CBR temperature equals, $T_0=2.725\text{Kelvin}$. The idea was to help explain the discrepancy between the cosmological constant, $\Lambda =8\pi G{\rho }_{\Lambda }/c^2$, in past epochs, versus today. There was also the issue of renormalizing gravity. If gravity effectively disappears at extremely high temperatures, then there is no theory to renormalize at high momentum exchanges. We believe that gravity is an order parameter, which vanishes at very high temperatures, much like magnetization.

In order to fix the parameters for the two models for, $G^{-1}\left(a\right)$, we demanded that, w, the quintessence parameter, equal exactly, $w=-0.98$. In the ΛCDM model this parameter is assumed to equal exactly negative one. But this is not what is observed after over a decade of observations and measurements [10]. Although the negative one can easily be accommodated within observational error, perhaps this is not its true value. By setting, $w=-0.98$, we were able to fix the parameters in our two models. Moreover, we were able to prove that, $\text{'}G/G=-0.06H$, in the current epoch. This is a variation within observational bounds. Jordan’s original thesis, that, $\text{'}G/G=-H$, seems to be unsupportable given current observations and measurements.

The challenge was, of course, to show that in virtually all other aspects, the deviation from the ΛCDM model was not great. The ΛCDM model has proven to be very successful and robust. It is only in the very early universe that marked deviations occurred between our models, which were called models, A and B, and the ΛCDM model. In fact both functions for, $G^{-1}\left(a\right)$, which were presented, reduce to exactly the ΛCDM model, in the limit where $w=-1$. In both our models, $G^{-1}\left(a\right)$ is an order parameter, which rises dramatically at inception, and as the universe cools, tapers off and flattens to a saturation value, much like magnetization in condensed matter physics. Even though both models were quite distinct from one another, qualitatively, quantitatively they gave similar results. For example, their inception temperatures were very close to one another. For model, A, the, $G^{-1}\left(a\right)$, started to form around, 6.20E21 Kelvin, whereas for model, B, we obtained, 7.01E21 Kelvin as the Curie temperature. At high temperatures, both models for, $G^{-1}\left(a\right)$, were inversely proportional to temperature. In both models we are close to saturation as, $G^{-1}\left(a\right)$, barely varies in the present epoch. Both functions are one-parameter, non-linear functions, which mimic order parameter behavior. Before approximately, E22 Kelvin, it is conjectured that Newton’s constant, as we know it, did not exist.

In another series of papers [11] [12], we developed a gravitational polarization model for the vacuum. Based on previous work of Hajdukovic [13] [14] [15] [16], and Winterberg [17] - [23], it was realized that polarization might offer the key towards a fuller understanding of dark matter, and dark energy. If the ambient temperature is low enough, ordinary matter, made up of quarks and leptons, can polarize the surrounding space forming a polarization cloud or halo. In gravistatics, this leads to anti-screening versus screening in electrostatics. The gravitational dipoles formed in gravistatics, add to the source field, $\stackrel{\to }{g^{\left(0\right)}}$, making the macroscopic field, $\stackrel{\to }{g}=\stackrel{\to }{g^{\left(0\right)}}+\stackrel{\to }{g^{\left(1\right)}}$, larger than the original applied field, $\stackrel{\to }{g^{\left(0\right)}}$. The, $\stackrel{\to }{g^{\left(1\right)}}$, is the induced field set up within the vacuum, due to net macroscopic massive dipole ordering or alignment. We treat the vacuum as a medium which can be polarized, much like a dielectric can be polarized. Instead of charge dipoles, we now have mass dipoles, made up of the positive and negative mass planckions, introduced by Winterberg. In electrostatics, we do not have anti-screening, but screening. There, electric charge dipoles set up within the dielectric are such as to diminish the original, or applied field, $\stackrel{\to }{E^{\left(0\right)}}$, creating a macroscopic, $\stackrel{\to }{E}=\stackrel{\to }{E^{\left(0\right)}}+\stackrel{\to }{E^{\left(1\right)}}$, which is less than, $\stackrel{\to }{E^{\left(0\right)}}$. The, $\stackrel{\to }{E^{\left(1\right)}}$, is an induced field set up within the dielectric due to net charge dipole ordering. For dark matter, we need extra mass, in order to explain the halo effect surrounding galaxies, rotation curves, virial motion of galaxies within superclusters, gravitational lensing, etc. There will be bound mass due to the massive dipoles produced within the vacuum, or gravitic, which is our gravitational version of a dielectric. This bound mass trapped within mass dipole formation in the vacuum, and macroscopic ordering, seems to us a perfect candidate for dark matter [11].

Dark energy, on the other hand, was identified [11] as the gravitational field mass density, produced by both source matter, and bound, polarized matter. According to Gauss’s law, if the universe contains net source matter, which it does, and bound, polarized mass, which is an assumption, then there must be gravitational fields associated with each. We identify the dark energy density with, ${\rho }_{\Lambda }=\overline{{\rho }_{gg}}=1/\left(2c^2\right)K\epsilon {\bar{g}}^2$. In this equation, K is the relative gravitational permittivity, and, $\epsilon \equiv 1/\left(4\pi G\right)$ is the gravitational permittivity. If, $G=G\left(a\right)$, then, the gravitational permittivity, $\epsilon =\epsilon \left(a\right)$, is also an intrinsic property of the vacuum. We will work with this assumption in this paper. The, $\bar{g}=\overline{g^{\left(0\right)}}+\overline{g^{\left(1\right)}}$, is the smeared, cosmic gravitational field obtained from Gauss’s law. This gravitational field is associated with the universe as a whole, once all source, and bound, matter is taken into account. Although technically, a surface gravitational field, it holds point for point within the observable universe, because any observer, no matter the location, would deduce this same value. Also, every surface is another observer’s reference point. It permeates all of space because ordinary matter, and bound matter, both permeate all of space. This gravitational field holds for distance scales in excess of about, 100 Mpc. Only then, is the universe fairly homogeneous and isotropic. A smeared quantity, such as, $\bar{g}$, or, $\overline{{\rho }_{gg}}$, is not a local quantity. All density values in Friedmann’s equation are smeared quantities.

Winterberg [17] - [23], in particular, developed a model of space made up of positive and negative mass particles called planckions. These particles are assumed to be real particles, versus virtual, and have positive and negative the Planck mass. We believe that the magnitude of these masses changes cosmologically, whereas he claims they are constant. According to Winterberg, the planckions form a very rigid two-component superfluid (we prefer supersolid), where disturbances move at the speed of light. The positive and negative mass species interact amongst themselves, and maintain a fixed distance of separation from other neighboring particles of the same species. Unlike mass planckion particles do not interact directly [23], but indirectly. Because positive and negative planckion particles occupy the same space, and are spread evenly, the positive and the negative masses are invariably drawn next to one another. They are forced to rub shoulders with one another, so to speak, and also maintain a fixed distance of separation from each other.

Winterberg developed an extensive and elaborate theory along this idea, and we will use it here, to establish an intimate connection between electrostatics, and gravistatics. We believe that the two-component superfluid is the key towards understanding the connection between gravity and electrostatics.

In the Winterberg model, fluid forces are responsible for keeping the planckions a fixed distance apart. When planckions are displaced from their equilibrium positions, increased planckion pressure forces them back into position. We can think of them as restoring forces, and we have modeled them as such [11]. Winterberg assumed that two like planckions, whether they have positive or negative mass, repel each other much like charges in electrostatics. The question naturally arises … is the planckion force ultimately an electrostatic force?

The Q theory, we believe, provides the answer to this question. If like mass planckions are anchored in position, and keep a finite distance apart, then there must be two forces acting on the individual planckion, one attractive and one repulsive, along any given direction in space. The repulsive force is electrostatic, and the attractive force is also electrostatic. An electrostatic attractive force might simulate gravity, however. What if Planck charge and Planck mass were created at the same time, as two components of the same particle? Wouldn’t they attract and repel simultaneously? Also, their creation need not be at the Planck temperature, ~E32 Kelvin. If G is varying with respect to cosmological time, then, $G^{-1}\left(a\right)$, could have formed out of the vacuum at a reduced temperature of the order, ~E21 - E22 Kelvin. Irrespective of the temperature of formation, we would have a vacuum, which is not only electrically neutral, but also massively neutral in its very earliest stages. It is well known that all particles in the standard model, i.e., all quarks and leptons, started to freeze out later at reduced temperatures, well below, ~E16 Kelvin, or 1 TeV [24] [25] [26] [27]. According to the proposed model, the universe is born electrically and massively neutral because there are equal numbers of positive and negative masses, as well as positive and negative charges. In the Winterberg model, fermions, and interacting bosons are quasiparticles, i.e., collective excitations, which are stable and form within the two-component superfluid. See reference, [23], for specific details.

The outline of this paper is as follows. In section II, we formulate the Q theory, the assumption that Planck mass and Planck charge were created at the same time, as two components of the same particle. The temperature of formation could be at, ~E32 Kelvin, but also in the neighborhood of ~E21 - E22 Kelvin. Upon their creation, two force laws were formed simultaneously, and spontaneously, one electrostatic, and one seemingly gravistatic. In fact, both will be shown to be electrostatic in origin, in Section III.

In Section III, we show that the gravitational force is electrostatic in origin. We derive an expression for the Planckion spring constant. Then we proceed to find the number density of planckions, and nearest neighbor distance of separation, using box quantization. In Section IV, we calculate individual displacements for various gravitational pressure fields. We show how gravitational potential, and gravitational pressure, within the vacuum are related. We also talk about latent gravitational field energy and vacuum resiliency. When the vacuum is mechanically stressed through very intense gravitational fields, it may have its limits. Gravitic breakdown is a possibility. The Winterberg vacuum is mechanistic in its very structure. In Section V, the present day imbalance in planckion number density is discussed. Because there is net mass in the universe, there is also, a net cosmic gravitational field mass density by Gauss’s law. That will result in a net planckion mass density, which is unequal to zero. The reason why the planckion number densities are mismatched, $\overline{n_{+}}>\overline{n_{-}}$, in the current era is unknown, but we speculate it may have something to do with macroscopic mass formation. Our summary and conclusion are presented in Section VI.

2. The Q Theory

We start by noting that Planck mass, $m_{Pl}$, is related to Planck charge, $q_{Pl}$, by the following relation.

$m_{Pl}^{2}G=\hslash c=q_{Pl}^{2}k$ (2-1)

This is easily proven by using the respective definitions for both, $m_{Pl}$, and, $q_{Pl}$. In the above equation, k, is related to the electric permittivity of free space, ${\epsilon }_0^{elstat}$, by the equation, $k=1/\left(4\pi {\epsilon }_0^{elstat}\right)=8.988\text{E}9\left(\text{MKS}\right)$. All units not expressly written out are MKS units. Using Equation (2-1), both, $m_{Pl}$, and, $q_{Pl}$, could have been created at the same time, and not necessarily at CBR temperature, 10E32 Kelvin. If G varies with cosmological time, then Equation (2-1), tells us that, $m_{Pl}$, must also vary such that the product of, $m_{Pl}^{2}G=\hslash c$, stays constant. In the current epoch, $G=G_0=6.674\text{E}-11\left(\text{MKS}\right)$. This fixes the Planck mass in the present epoch to equal, $m_{Pl}=m_{Pl,0}=2.176\text{E}-8\text{kg}$.

We next multiply Equation (2-1), by 1/r². This, will stand for the distance of separation between two Planck mass particles, which is the same distance as between the two Planck charge particles. Because they are one and the same particle by our hypothesis, we have two separate forces coming into being at the same time, as two separate force magnitudes are formed.

$G{m}_{Pl}^2/r^2=\hslash c/r^2=k{q}_{Pl}^2/r^2$ (2-2)

Along a line connecting the two particles, one of the forces, $G{m}_{Pl}^2/r^2$, will be attractive, and the other, $k{q}_{Pl}^2/r^2$, repulsive, when acting on an individual Planck particle, or planckion. From Equation (2-2), it follows namely that,

$\left(G{m}_{Pl}^2/r^2-k{q}_{Pl}^2/r^2\right)\hat{i}=0$ (2-3)

The unit vector, $\hat{i}$, points from one mass to the other. In Equation (2-3), we see that Newton’s law, and Coulomb’s law, hold for two positive, as well as two negative, planckion masses or charges. What about a positive with a negative mass/charge? Then we introduce a minus sign for the two terms on the left hand side of Equation (2-3). Their sum still adds up to zero. Positive and negative planckions do not interact directly, but indirectly [11] [23], through fluid forces. These fluid forces are caused by particles within their own species. The fluid forces are such as to make the positive and negative mass particles spread out evenly. However, because the two species occupy the same space, the positive and negative particles are invariably drawn next to each other through their respective fluid forces. They are forced to rub shoulders with one another, so to speak, without necessarily interacting. Nevertheless, Equation (2-3), still works as an effective force law between unlike charges/masses.

Equations (2-2), or, (2-3), are the effective forces acting on individual planckions due to another specific planckion in the vicinity. The simplest way to write this force law is simply,

$F=\hslash c/r^2=F_{grav}=F_{elstat}$ (2-4)

These planckions need not be nearest neighbors. This is both simultaneously an attractive and a repulsive force by virtue of Equations (2-2), or, (2-3). When attractive, we call it gravity. When repulsive, we call it electrostatic. Positive and negative mass planckions want to maintain a fixed distance of separation from other positive and negative mass planckions.

Numerically, the Planck charge has the value, $q_{Pl}=1.876\text{E}-18\text{C}$. This can be evaluated using Equation (2-1). The Planck charge is related to the elementary unit of charge, $e=1.6\text{E}-19\text{C}$, by the Sommerfeld fine structure constant,

${\left(e/q_{Pl}\right)}^2={\alpha }_{EM}=k{e}^2/\left(\hslash c\right)=1/137$ (2-5)

We interpret $q_{Pl}$ as the naked charge of an electron or a proton, whereas “e” is the dressed charge, which takes into account the electrostatic polarization of space surrounding the naked charge [28]. Due to the screening in electrostatics, the polarization cloud will lower the original naked charge by a factor, (1/137)^1/2. The elementary unit of charge is what is measured and not the naked charge. We expect the same in gravistatics. The, $m_{Pl}$, will not be measured directly, but rather a dressed version, taking the gravistatic polarization cloud into account. In contrast to electrostatics where we have screening, in gravistatics, we have anti-screening. This should serve to enhance, i.e., increase the naked mass. The dressed mass will be heavier than the naked Planck mass, a prediction.

It was stated that positive and negative mass planckions want to maintain a fixed distance of separation from one another. When displaced from equilibrium, the planckions will experience a restoring force wanting to bring them back to their original configuration. For a positive and negative mass planckion, those forces are [11], respectively,

$F_{+,x}=\left(m_{Pl}\right)\ddot{x}=-\kappa x$ (2-6a)

$F_{-,x}=\left(-m_{Pl}\right)\ddot{x}=+\kappa x$ (2-6b)

The, $\kappa ={\kappa }_{+}={\kappa }_{-}$, is the planckion spring constant, which is assumed the same for both positive and negative mass particle. The, +κ, on the right hand side of Equation (2-6b), is needed for a bounded solution. Choosing a negative spring constant on the right hand side would give us a hyperbolic sinusoidal solution, which is unbounded. The, x, here, refers to the displacement from equilibrium, either positive or negative, along a particular direction. If planckion particles get too close to other particles of the same species, then there will be repulsion. If they stray too from each other, then there will be attraction. In this way equilibrium is maintained within the fluid, where the individual planckions are, more or less, anchored in position.

As was demonstrated by Winterberg, the collective fluid force acting on a positive mass planckion is,

$\stackrel{\to }{F_{+}}=-n_{+}^{-1}\stackrel{\to }{\nabla }{p}_{+}=-n_{+}^{-1}\left(\stackrel{\to }{\nabla }{n}_{+}\right)m_{Pl}{c}^2$ (2-7)

This is due to the other positive mass planckion particles within the fluid. In Equation (2-7), $n_{+}\left(\stackrel{\to }{x}\right)$ stands for the positive mass planckion number density, and $\stackrel{\to }{\nabla }{p}_{+}$ is the gradient of the planckion pressure, $p_{+}\left(\stackrel{\to }{x}\right)$. The positive planckion pressure exerted by the other positive mass planckions is defined as, $p_{+}\equiv n_{+}{m}_{Pl}{c}^2$, where c is the speed of light and, $m_{Pl}$, is the Planck mass. Like all fluids, for an increase in pressure in moving the particle from, x, to, x+dx, there is a restoring force acting in the opposite direction, wanting to bring the particle back. In one dimension, Equation (2-7), namely reads,

$F_{+,x}=-n_{+}^{-1}\text{d}{n}_{+}/\text{d}x{m}_{Pl}{c}^2$

$-\kappa x=-n_{+}^{-1}\text{d}{n}_{+}/\text{d}x{m}_{Pl}{c}^2$ (2-8)

We have set the left hand side of Equation (2-8), equal to $–\kappa x$, because this is our restoring force. Think of the left hand side as a response to the right hand side, where we assume some sort of external influence.

Equation (2-8), is easily solved by bringing the, dx, over to the left hand side and integrating. The solution is

$n_{+}\left(x\right)=n_{+}\left(0\right){\text{e}}^{\kappa x^2/\left(2m_{Pl}{c}^2\right)}$ (2-9)

Increasing x in either the positive or negative sense, increases the number density, $n_{+}$, but also at the same time, the planckion mass density, ${\rho }_{+}\equiv m_{Pl}{n}_{+}\left(x\right)$, and the planckion pressure, $p_{+}\equiv m_{Pl}{n}_{+}\left(x\right)c^2$. This produces a force, acting in the opposite direction, as indicated by Equation (2-8). Equation (2-9), indicates a “hole”, or trough, centered about, $x=0$, for the positive mass planckion to “rest” in. The minimum planckion pressure is achieved when, $x=0$.

For a negative mass planckion, the counterpart to Equation (2-7), is found by replacing all positive signs by negative signs and vice versa, and making the substitution, $m_{Pl}\to -m_{Pl}$. Thus, the fluid force acting on the negative mass planckion equals,

$\stackrel{\to }{F_{-}}=+n_{-}^{-1}\stackrel{\to }{\nabla }{p}_{-}=+n_{-}^{-1}\left(\stackrel{\to }{\nabla }{n}_{-}\right)\left(-m_{Pl}\right)c^2$ (2-10)

This force, $\stackrel{\to }{F_{-}}$, is due to the other negative mass planckions populating the vacuum. Here, $n_{-}\left(\stackrel{\to }{x}\right)$ stands for the negative mass planckion number density, and, $p_{-}\left(\stackrel{\to }{x}\right)$ is the corresponding negative mass planckion pressure, defined by, $p_{-}\equiv n_{-}\left(\stackrel{\to }{x}\right)\left(-m_{Pl}\right)c^2=-n_{-}{m}_{Pl}{c}^2$. We notice that $p_{-}$ is inherently negative. The mass density, ${\rho }_{-}\equiv n_{-}\left(\stackrel{\to }{x}\right)\left(-m_{Pl}\right)$, is also inherently negative. Note that in Equation (2-10), the negative mass particle is taking the path of steepest ascent, because we are taking the positive gradient. Think of a negative mass particle in the earth’s gravitational field… it would accelerate upwards when released. This is in contrast to Equation (2-7), where we are looking at the path of steepest descent, i.e., negative the gradient, for a positive mass particle.

In one dimension, Equation (2-10), reduces to

$F_{-,x}=+n_{-}^{-1}\text{d}{n}_{-}/\text{d}x\left(-m_{Pl}\right)c^2$

$+\kappa x=-n_{-}^{-1}\text{d}{n}_{-}/\text{d}x{m}_{Pl}{c}^2$ (2-11)

See Equation (2-6b). We have set the left hand side equal to, $+\kappa x$, because this is a restoring force for the negative mass planckion. The solution to Equation (2-11), is found by integration. The result gives,

$n_{-}\left(x\right)=n_{-}\left(0\right){\text{e}}^{-\kappa x^2/\left(2m_{Pl}{c}^2\right)}$ (2-12)

This Gaussian looking function indicates a peak at, $x=0$, versus a trough, as in Equation (2-9). A peak for a negative mass particle is equivalent to a “hole”, for a positive mass particle. In other words, a negative mass planckion will move in such a way, as to increase its planckion pressure. Think of a negative mass particle in the earth’s gravitational field. When released it would accelerate upwards at, 9.81 m/s², increasing its gravitational pressure. At, $x=0$, in Equation (2-12), we have maximum pressure for a negative mass planckion. Any positive or negative displacement from this equilibrium position, will lead to restoring forces tending to bring the negative mass particle back to, $x=0$.

The total planckion pressure, p, due to positive and negative Planck particles, in a region of space, $\stackrel{\to }{x}$, is

$p=p_{+}+p_{-}$

$=m_{Pl}{c}^2\left(n_{+}-n_{-}\right)$

$=0$ (undisturbed fluid)

$\ne 0$ (disturbed fluid; gravitational field) (2-13)

For the undisturbed fluid (vacuum) with no gravitational fields, the positive and negative mass number densities balance, i.e., $n_{+}\left(\stackrel{\to }{x}\right)=n_{-}\left(\stackrel{\to }{x}\right)$. The Planck pressure, $p=p_{Pl}$, is related to the planckion mass density, $\rho$,by the equation of state, $p=w\rho c^2$, where, $w=1$. Individually,

$p_{+}=w{\rho }_{+}{c}^2={\rho }_{+}{c}^2$, $p_{-}=w{\rho }_{-}{c}^2={\rho }_{-}{c}^2$, $p=w\rho c^2=\rho c^2$, (2-14a,b,c)

where, $w=+1$, in all instances. Therefore, by Equation (2-13), we may also write,

$\rho \left(\stackrel{\to }{x}\right)={\rho }_{+}+{\rho }_{-}$

$=m_{Pl}\left(n_{+}-n_{-}\right)$

$=0$ (undisturbed fluid)

$\ne 0$ (disturbed fluid; gravitational field) (2-15)

In a gravitational field, the two component superfluid will no longer be undisturbed. If, $\stackrel{\to }{g}\ne 0$, then we have a perturbed state where, $n_{+}\left(\stackrel{\to }{x}\right)>n_{-}\left(\stackrel{\to }{x}\right)$, as will be shown shortly. Then we have a net planckion pressure, and a net planckion mass density, which is now unequal to zero. A net planckion mass density and pressure for the vacuum is a new prediction of Winterberg’s theory.

In a gravitational field, there is an increase in gravitational pressure. The total gravitational pressure, $p_{gg}\left(\stackrel{\to }{x}\right)$, which can be thought of as equivalent to gravitational field energy density, equals,

$p_{gg}={\rho }_{gg}{c}^2=1/2K\epsilon g^2$ (2-16)

In this equation, ${\rho }_{gg}$, is the gravitational field mass density, K, the relative gravitational permittivity, and, ε, the gravitational permittivity, defined as, $\epsilon =1/\left(4\pi G\right)$, by analogy to electrostatics. In the present cosmological epoch, $\epsilon ={\epsilon }_0=1/\left(4\pi G_0\right)=1.192\text{E}9\left(\text{MKS}\right)$. Both, $K=K\left(a\right)$, and, $\epsilon =\epsilon \left(a\right)$, are thought to be epoch dependent [12]. We presented two specific models for both, $K=K\left(a\right)$, and, $\epsilon =\epsilon \left(a\right)$, where, a, is the cosmic scale parameter, in previous work. By analogy to electrostatics, there is an energy density associated with gravitational fields. For gravistatics, we replace the electrostatic version of electric field energy density, $1/2K{\epsilon }_0{E}^2$, by its gravitational counterpart, $1/2K\epsilon g^2$ [11]. Wherever we have a gravitational field, we have energy trapped in a box, and by default, an equivalent mass density. Through Equation (2-16), we can calculate the amount.

We next set the gravitational field mass density, in Equation (2-16), equal to the net planckion mass density, Equation (2-15), in the disturbed vacuum state.

$\rho ={\rho }_{gg}$

$\left[n_{+}\left(\stackrel{\to }{x}\right)-n_{-}\left(\stackrel{\to }{x}\right)\right]m_{Pl}=1/\left(2c^2\right)K\epsilon g^2$ (2-17)

We no longer have, $n_{+}\left(\stackrel{\to }{x}\right)=n_{-}\left(\stackrel{\to }{x}\right)$, but, $n_{+}\left(\stackrel{\to }{x}\right)>n_{-}\left(\stackrel{\to }{x}\right)$, because of the presence of the gravitational field. If we multiply Equation (2-17), through by, c², then we see that the planckion pressure equals the gravitational pressure. If, ${\rho }_{gg}\left(\stackrel{\to }{x}\right)\ne 0$, we literally create mass out of the vacuum, and since the vacuum is made up of planckions and blackbody photons exclusively, this mass increase must be due to a net planckion mass density. The same holds for gravitational pressure. If the net gravitational pressure at a point in the vacuum is unequal to zero, $p_{gg}\left(\stackrel{\to }{x}\right)\ne 0$, then the massive planckions must be responsible, and, therefore, we can set, $p=p_{gg}\ne 0$.

In a small enough region of space, the gravitational field, $\stackrel{\to }{g}\left(\stackrel{\to }{x}\right)$, is uniform and constant. Without loss of generality, we can assume that $\stackrel{\to }{g}\left(\stackrel{\to }{x}\right)$ points in the negative x direction,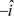. Equation (2-17), can then be rewritten as,

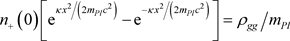(2-18)

For the second line, we made use of Equations (2-9), and, (2-12). And for the third line, it was recognized that,.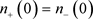

We next make a change of variable. Let us define,

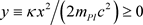(2-19)

Then Equation (2-18), becomes

(2-20)

We have employed a mathematical identity, 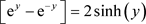, for the second line. In a gravitational field the, , will increase, and the, , will decrease over, the values. Due to the symmetry between positive and negative planckions, the increase in, , equals the decrease in,. The factor of two in Equation (2-20), reflects that fact.

Several notes are in order regarding Equation (2-20). First, we have literally created mass out of the vacuum through the introduction of,. See Equation (2-17). Second, even though positive and negative mass planckions, are spatially anchored in position, they can be displaced by external influences, such as a g-field. This will produce net gravitational, or, net planckion pressure, as well as net gravitational mass density, or net planckion density. Third, Equation (2-20), shows us how much of a displacement we can expect for a given gravitational field. On the right hand side, we have, , and on the left hand side, we have. Within this expression for, y, is the displacement, x. What is needed is the spring constant, κ, which we will soon evaluate. For small values of, y, the, , and Equation (2-20), reduces to,

(2-21)

Simplifying and rearranging, we have,

(2-22)

We notice that in the limit where, , the displacement, x, is directly proportional to,g. The other terms are constants. Fourth, to determine, , one needs, y, or, equivalently,. Fifth, the restoring force depends on, κ, the planckion spring constant. The displacement, x, should depend on that as well. In Equation (2-22), we see clearly that an increase in, κ, leads to a decrease in displacement, x.

In the present epoch, the gravitational permittivity,. The relative permittivity, K, is a bit tricky. It is determined from the gravitational susceptibility, through the relation,. The gravitational susceptibility has been found for the cosmos as a whole, as a smeared quantity [11]. However, its value locally depends on many factors such as the localized gravitational dipole moment, local gravitational field, and local ambient temperature [12]. It varies from place to place in the universe. Fortunately, however, there is a relation, which can help us. As shown in reference [11], the bound mass, M_B, made up of macroscopically ordered dipole moments, is related to the free, source mass,M_F, made up of quarks and leptons, through the relation,. The masses, M_F, and, M_B, are the masses contained within a Gaussian surface. The, , and, , on the other hand, are the susceptibility, and relative permittivity values on the Gaussian surface, respectively. In many instances, when considering astronomical bodies, such as the earth, or a black hole, we can estimate the surface gravitational dipole moment, the ambient surface temperature, as well as the surface gravitational field. Thus we can estimate,. In many instances, right outside the surface, , is negligible, which tells us that the bound mass contained within that surface is negligible. If, , then it follows that,.

We next focus on finding a relation between the planckion spring constant, κ, and, , the planckion number density for an undisturbed planckion fluid.

3. Determination of Planckion Number Density, Nearest Neighbor Distance of Separation, and Planckion Spring Constant

We next want to establish a connection between the planckion spring constant, κ, which holds for both species of Planck particle, and, , the undisturbed positive and negative mass planckion number density. As shown previously in section II, a positive mass planckion will simultaneously attract and repel another positive mass planckion through gravitational and electrostatic forces. The same will hold true for negative mass planckions. In short they strive to maintain a fixed distance of separation from one another. Unlike mass planckions really do not interact directly [11] [23]. Instead they interact indirectly by being forced close to one another by their respective fluid forces.

Consider a string of positively charged planckions, all in a row, along the x-axis, and label them, #1, #2, #3, etc. Due to the symmetry, forces in the, y, and, z, direction cancel, and we are concerned only with forces in the x-direction. Focus on particle, #3, and sum up the forces acting on that particle, when that 3^rd particle is displaced a distance, x, to the right. We claimed previously, that displacing this planckion will cause a restoring force in the amount, , and so we obtain,

(3-1)

In the last line, we used Equation (2-1). The force that particle, #4, exerts on particle, #3, which is, , is pointing to the left, and hence the negative sign in Equation (3-1). The force that particle, #2, exerts on particle, #3, which is, , is pointing to the right, and hence the positive sign associated with this force in Equation (3-1). The positive and negative signs for all subsequent forces are found in this fashion. In Equation (3-1), , is the unperturbed or average nearest neighbor equilibrium distance of separation between two planckions of the same species.

The, , is related to the positive mass planckion number density, , through the equation,

(3-2)

Next, we recognize that, for any arbitrary, “b”, and, “β” values, the following identity holds,

(3−3)

This can be proven algebraically. We set, , and substitute this together with Equation (3-3), for various, “b”, values into Equation (3-1). We find,

(3-4)

In Equation (3-4), we first factor out the, , term on both left and right hand sides. Then we bring the, , term from the left hand side over to the right hand side, and make use of Equation (3-2), to simplify. The result, after pulling out a factor of 4, is,

(3-5)

Equation (3-5), holds for any value of, including, or, what is equivalent,. Therefore it follows that,

(3-6)

The infinite series within Equation (3-6), is a known series,

(3-7)

Here, , is the Riemann Zeta function [29] [30], and equals Apery’s constant, an irrational number. Numerically,. Making use of this value, we now have an expression for, κ, the planckion spring constant. It reads,

(3-8)

More generally, irrespective of the value of, , in Equation (3-5), the infinite series within that expression on the right hand side, must equal,.

The arguments above for a positive mass/charge planckion particle also hold for a negative mass/charge planckion particle. Some of the signs change, but the outcome is the same. The negative mass planckions are also held in check by what are, essentially, electrostatic forces.

We still have to determine the planckion number density, , for an undisturbed vacuum. To fix a value for, , and thus determine a value for κ, by Equation (3-8), we use the fact that our planckions are, more or less, spatially anchored or locked in position. Hence they are confined to a region of space where box quantization must apply. They are also oscillating, i.e., continuously accelerating, which produces gravitational radiation. Because they are “boxed in”, and radiating, the energy of the radiation emitted must be related to the energy level jumps, or transitions, permissible within that box. Much like the Bohr atom for Hydrogen, energy level transitions account for the radiation frequency emitted. The situation here is totally analogous.

To see this more clearly, we consider the energy levels for a particle trapped in a 3-dimensional cubic box, having volume, L³. These are well known to be quantized, and given by the expression [31],

(3-9)

The, are quantum numbers, which can take on the values,. The lowest energy level, or ground state, is specified by,. In this instance, Equation (3-9), reduces to,

(3-10)

For the mass of the confined particle, we have chosen the Planck mass, which in the present epoch, equals,. The next highest energy level has three-fold degeneracy, because,. The next highest energy level also has three-fold degeneracy since,. The fourth level has no degeneracy,. Continuing in this fashion, we can account for all the energy levels.

If the positive mass planckion is excited, due to collisions with CBR photons, for example, transitions between energy levels are possible. We have quantum jumps where the energy emitted is,

(3-11)

The unprimed quantum numbers refer to the situation before, and the primed quantum numbers correspond to the situation after the transition. This is completely analogous to the situation in the Hydrogen atom, where we have the Lyman series, the Balmer series, the Paschen series, etc.

The most probable transition is the most frequent one, and there,. This is already obvious from the few examples of energy levels given. Transitioning from higher to lower levels gives exactly this amount. For example, transitioning from, , gives exactly this amount.

The energy being emitted is determined by the Planck radiator formula,

(3-12)

Here, ν, is the frequency of the photon being emitted, and T is the blackbody CBR temperature. The, k_B, is Boltzmann’s constant. Nowhere in this formula, is mass, or charge, explicitly stated. We interpret this to mean that any quantum radiator will emit this amount of energy irrespective of whether it is mass or charge which is oscillating. In our case, it is both, due to the Q hypothesis.

We will consider quantum radiators due to planckions at CBR temperature. In the present epoch,. Equation (3-12), the becomes,

(3-13)

In Equation (3-13), we have chosen the most probable frequency, for the frequency for this particular CBR temperature. In general the peak frequency is specified by,

(3-14)

The oscillating and continuously accelerating positive mass/charge planckion acts as a radiator, and at a CBR temperature of 2.725 Kelvin emits this specific frequency as its peak frequency. In reality a whole spectrum of frequencies are being emitted as an infinite number of quantum transitions are possible. See Equation (3-11). We singled out one particular frequency, the most probable.

Equation (3-9), is explicitly mass dependent. If the mass of the positive mass planckion changes, due to a change in G value, this will affect the energy levels, and the transitions which are possible. Lower mass means higher energy levels for the same quantum numbers according to Equation (3-9). This means that, in general, larger frequencies will be emitted. But this is exactly what we expect at higher CBR temperature. The frequencies being emitted, in general, will also change as a consequence, being shifted towards higher values. They are still quantized, but will take on different values.

What about the negative mass planckions? For a negative mass, such as a negative mass planckion, the energy levels are inherently negative, by Equation (3-9), as measured from the top down. We have inverted box quantization, where instead of a potential energy square well, we have the mirror image, an upright potential energy square well. This upright well is populated with energy levels, according to Equation (3-9), taken from the top down. The largest energy level jumps are near the top. The highest level in this inverted potential energy well is the “ground state”. And negative mass particles will want to transition to this highest energy level. Equation (3-11), is still valid. But instead of going from less negative energy to more negative energy, which is the case for a positive mass particle, we will be transitioning from positive energy levels to higher positive energy levels for a negative mass particle. By transitioning upwards, a negative mass planckion actually lowers its binding energy. Due to the symmetry of the energy levels between positive and negative planckions, the most probable transition here, also, releases, , of energy.

It is worth mentioning that the frequency of emission, is exactly equal to the difference in oscillating frequencies of the planckions. Since the positive and negative mass planckions are continuously accelerating, with a specific frequency, the frequency of emission should equal the difference, , in oscillating frequencies, where, , is the angular frequency of the planckion before the transition, and, , is the angular frequency after the transition.

Since both the positive and negative mass planckions contribute their fair share of energy to the radiator at a particular temperature, we set two times the energy value, indicated by Equation (3-10), equal to one times the value of Equation (3-13), which is the resulting energy output. Thus,

(3-15)

This is easily solved for length,, and we obtain,

(3-16)

The dimensions of the cubic box, just so happen to equal the distance of separation between nearest neighbor positive, and negative, mass planckions. The only difference is that the geometric center of the box is centered around the positive or negative mass planckion.

One will notice that our nearest neighbor inter-planckion separation distance, within a specific species, is very close to the limits what modern day accelerators are able to probe. The diameter of a quark is about, 8.60E−19 meters, and Equation (3-16), is just a hair below that. The LHC at CERN produces 7 TeV protons whose Compton wavelength is 1.78E−19 meters. All these distances are comparable to the nearest neighbor inter-planckion separation distance. If our estimates are correct, we may be on the verge of establishing an inherent “graininess” for the vacuum.

Having determined the nearest neighbor separation distance, we proceed to find the average number density for both the positive and the negative mass planckions, when the vacuum is in the undisturbed state. For this, we use Equations (3-2), and, (3-16). Substituting Equation (3-16), into Equation (3-2), we find that,

(3-17a)

(3-17b)

The number densities are, needless to say, very high. But now, we are attempting to find a graininess to the vacuum, or space, which many believe is smooth and continuous.

Finally, let us come back to the spring constant for planckions, Equation (3-8). Using Equations (3-8), and (3-17a), we can now evaluate its value. We obtain for this calculation,

(3-18)

This is a current epoch value because the spring constant will scale upon expansion of the universe, as shown in reference [11]. This large value for a spring constant justifies our assumption that we are dealing with a very stiff superfluid/ supersolid when we are considering the vacuum. Inter-planckion restoring forces are indeed very, very large, even for the smallest displacements.

In this section, the spring constant was defined strictly in terms of electrostatic forces. See Equation (3-1), which is entirely electrostatic in origin. In the previous section, was associated with the restoring force if either the gravitational force or the electrostatic force got the upper hand. For equilibrium, the electrostatic force of repulsion counteracted the gravitational force of attraction between two like mass planckion particle. Realizing that we are dealing with the same κ, it should be recognized that the gravitational force is really electrostatic in nature.

We can look more carefully at the sum given in Equation (3-1). All the negative sign contributions pull the #3 particle to the left. All the positive sign contributions pull planckion particle #3 to the right. If, , then there is no displacement and particle #3 is in equilibrium. In other words, both the individual forces pulling to the left and the individual forces pushing to the right add up to zero. We can identify the gravitational force with the sum of either one of these net forces, pushing or pulling. The electrostatic force would then be identified with the counteracting force. In short, the force of gravity is really electrostatic in origin within the Q theory. When the planckions were first created two force magnitudes were simultaneously created. But one counteracted the other, and being an attractive force between two masses was treated as a gravitational force. The gravitational force has evolved with cosmological time through a varyingG value, whereas the electrostatic force has not. That is our hypothesis.

4. Gravitational Displacements, the Gravitational Potential, Latent Gravitational Field Energy, and Vacuum Resilience

We saw that the inter-planckion distance of separation, between like mass planckions, is of the order, ~5E−19 meters. This must be close to the maximum displacement that a planckion particle can experience. Gravitational displacement within the vacuum will occur whenever we have an external applied field, such as that what might be found just outside the surface of a black hole. It is now time to look at such situations. We first start, however, with another example, the cosmos as a whole.

It was shown in a previous work [11], that we can define a cosmic gravitational field, due to all the ordinary, and polarized bound mass contained within the cosmos. Polarized bound mass was identified as dark matter. Using Gauss’s law, and the ΛCDM parameters in Friedmann’s equation, we found that,

(4-1)

The subscript, “0”, refers to the present epoch, and the bar indicates an average or smeared quantity. At distance scales in excess of about, 100 Mpc, the universe is fairly homogeneous and isotropic. The cosmic gravitational field, , in Equation (4-1), is the value obtained at the surface of the Hubble bubble, our Gaussian surface, and takes into account all matter, ordinary, and bound, contained within this sphere. Even though, , technically, is a surface gravitational field, we argued that it holds point for point within the cosmos, because one observer’s surface is another observer’s point of reference. Any observer within the universe would measure this same value, irrespective of location. It is a smeared or average value which holds for the universe as a whole.

We identified the cosmic gravitational field mass density, , with dark energy. It permeates all of space, takes into account source matter, made up of quarks and leptons, as well as bound, or polarized matter, due to macroscopic ordering of gravitational dipole moments within the vacuum. Using the ΛCDM parameters in Friedmann’s equation, it was found that numerically, the relative cosmic gravitational permittivity, , in the present epoch. Moreover, the gravitational permittivity, defined by, , equals, in the current era. The, G, is Newton’s constant, which may or may not be a cosmological constant. Since, , represents dark energy, it follows that

(4-2)

Solving this for, , gives us the value as is indicated in Equation (4-1). We are using the latest cosmological parameters as found by the Planck collaboration (final release) [32] [33].

Let us find the average displacement associated with this cosmic gravitational field. We can use either Equation (2-20), to find this displacement, or, since, Equation (2-22). We’ll use Equation (2-20), because we cannot be sure that. We first rewrite Equation (2-20) in the form,

(4-3)

Next we evaluate the right hand side using Equations (4-2), (3-17a), and,

(4-4)

Evaluating the right hand side of Equation (4-3), gives

(4-5)

This is clearly a very small value and we are justified in using the approximation,. We next substitute our value for, y, Equation (2-19), for the left hand side of Equation (4-5). This renders,

(4-6)

This equation can be solved for,. For the planckion spring constant, κ, we’ll use Equation (3-18). The Planck mass is specified in Equation (4-4). And the speed of light is also known. Evaluating gives,

(average cosmic displacement) (4-7)

This is an incredibly small displacement. However, we keep in mind that space is very, very dilute, only about 6 hydrogen atoms per cubic meter. Due to this dilution, there is hardly any displacement. In the true voids, where there is no source matter, we would expect zero gravitational field displacement. Also keep in mind that the maximum displacement seems to be in the neighborhood of about, 5E−19 meters, as is indicated by Equation (3-16).

Another example might be the gravitational field of the earth. On the surface of the earth, the source gravitational field is, 9.81 m/s². The relative permittivity, K, is essentially one because, as far as we are able to determine, there is virtually no vacuum susceptibility on the surface of the earth, if we take this surface to equal our Gaussian surface. There is virtually no polarized mass enclosed within this surface. For that we have to have a substantial vacuum or “empty” space, which doesn’t exist close to the earth. We calculate, , using Equation (2-16), and find

(4-8)

The gravitational permittivity equals,. Following the same steps as before, but now using the new gravitational field mass density, indicated by Equation (4-8), we find for the new gravitational displacement,

(surface of earth) (4-9)

Compared to the value in Equation (2-6), this is about 10 orders of magnitude larger. However, it is still extremely small in value.

We next look at the gravitational potential and its relation to the gravitational pressure. We designate the gravitational potential by, , because it is really a difference in gravitational voltage that we are considering. The subscripts indicate that this difference in voltage is due to gravitational fields.

We start with Equation (2-8). If we bring the infinitesimal displacement, dx, over to the left hand side, we really have, , where, , is the infinitesimal change in gravitational potential energy. However, we know that gravitational potential is related to gravitational potential energy by the relation,. Once the, dx, has been brought over to the left hand side in Equation (2-8), we divide both sides by, to obtain the relatively simple expression,

(4-10)

We integrate this from, , to, x, and find,

(4-11)

To take the two species of planckion particles into account, the positive and the negative mass species, we multiply this expression by a factor of two. Thus,

(4-12)

Finally, using Equation (2-9), this can be shown to equal,

(4-13)

This is a very simple and straightforward expression. Once we calculate a gravitational displacement, due to a specific gravitational field, we can find the corresponding increase in gravitational voltage, or gravitational potential, within the vacuum. We simply use Equation (4-13), with our κ value, specified in Equation (3-18). The planckion mass in the present epoch, is indicated in Equation (4-4).

Some numerical examples are as follows. For the cosmos as a whole, the average displacement of planckions within the vacuum, due to the presence of source and bound mass, is specified by Equation (4-7). Here, Equation (4-13), gives

(average gravitational potential within cosmos) (4-14)

On the surface of the earth, we have a difference displacement, indicated by Equation (4-9). This leads to a different gravitational potential in the amount,

(surface of earth) (4-15)

Note that the units for gravitational potential are the same units as, c².

The general relation between gravitational pressure, and gravitational potential, is considered next. Start with Equations (2-19), and, (2-20), and recognize that,. We rewrite Equation (2-20), as,

(4-16)

We next multiply Equation (4-16), through by the factor, c², to obtain the gravitational pressure,

(4-17)

This is our desired relation between gravitational pressure, , and gravitational potential,. In most instances, , and we can use the approximation,. In this approximation, Equation (4-17), simplifies to,

(4-18)

Here, we see a direct proportion between gravitational pressure and gravitational potential. The mass of the planckion, and planckion number density are also important. Incidentally, planckion pressure and gravitational field energy density, , are equal to one another, since

(4-19)

We already identified, , with dark energy [11]. See also Equations (2-16), and, (4-2). Gravitational pressure, gravitational field mass density, which is the same as planckion mass density, and dark energy, are all synonymous with one another. Equations (4-17), and (4-18), are another way to find gravitational potential,. They will of course give the same results as before.

We close this section with some thoughts on the magnitude of the gravitational pressure here on the surface of the earth. As we saw, the mass density equaled the value indicated by Equation (4-8), namely, 6.373E−7 (MKS). This is much, much, less than the lightest gases found here on the earth’s surface. We can multiply this by c² to obtain the gravitational pressure, or gravitational field energy density. The result is,

(4-20)

This is a comparatively large value for pressure, or energy density. Atmospheric pressure, for example, equals, 1.013E5 N/m². Why don’t we feel this gravitational pressure? Why can’t the energy in one cubic meter be released?

In cosmology, the energy densities can be related to the stress-energy tensor. To release the energy trapped in a box, means we would have to alter the stress energy tensor. For that to happen, it takes a certain violent gravitational reaction, such as a supernova explosion, or a black hole merger. These are not the conditions found here on earth. To give you an analogy, there is a lot of energy trapped within the nucleus. But only under certain circumstances can this be released, such as in a reactor, or in a bomb, or in a star. We believe something similar happens here. This is not energy trapped in matter, but energy stored, or trapped, within the vacuum, i.e., space itself. It cannot be released without altering the gravitational field itself. If there is no gravitational field, then there is no gravitational pressure, nor is there a gravitational field energy density. We would have to alter the, 9.81 m/s², here on earth, in order to tap into this energy, or release the gravitational pressure associated with this slightly gravitationally stressed vacuum. If we could eliminate the 9.81 m/s² in a box, one cubic meter in size, here on earth, we would liberate, 5.736E10 Joules, according to Equation (4-20). We can refer to this energy as latent gravitational energy.

This brings us to mechanical resiliency. Wherever there is a gravitational field, the vacuum is stressed, i.e., the individual planckions making up the vacuum are displaced from equilibrium. Our vacuum is very much mechanical in origin. In material science, resilience is the ability of a material to absorb energy when it is deformed elastically. Once the stress is released, the elastic energy goes away upon unloading. This describes the vacuum particularly well to our thinking. Resilience, or mechanical energy storage capacity, can be defined for the vacuum, and is measured in units of, N/m², or J/m³.

The maximum resilience of space seems to be in the neighborhood of about, 8.66E33 J/m³, to about, 1.54E34 J/m³. The first value holds on the surface of a four solar mass black hole, where the gravitational field is particularly strong, about, 3.81E12 m/s². The second value is the gravitational field energy density for a three solar mass black hole, where the gravitational field is even stronger, approximately, 5.08E12 m/s². No black holes have been found in nature having a mass less than three solar masses. The cutoff between neutron stars and black holes seems to lie in the neighborhood between three to four solar masses. Neutron stars can have gravitational fields as high as roughly, ~2E12 m/s², once newly formed. So the gravitational fields above seem to fit the scheme. Now it is known, that next to a black hole, three-dimensional space will develop a rip or a tear in the space-time continuum according to the general theory of relativity. In other words, three-dimensional space starts to break down. This would be our version of “gravitic breakdown”. Just like there is dielectric breakdown when the electric fields get too strong for the medium, we can expect that gravitationally, something similar happens. The gravitational fields listed above must be close to those limits. It should have been mentioned that the smallest mass black holes have the largest gravitational fields, due to the Schwarzschild condition. So the above gravitational fields are probably the strongest macroscopic gravitational fields known to science.

5. Net Planckion Imbalance in the Present Universe

Gravitational field energy density, which is the same as gravitational pressure, depends on stressing the vacuum, through the introduction of gravitational fields. The number density of planckions is, thereby, directly affected. This is seen explicitly in Equation (2-17), where we set the mass density of planckions, equal to the gravitational field mass density. The left hand side of Equation (2-17), is no longer equal to zero, if,. In fact, we will have an imbalance in planckion number density, where now,.

Before we consider the universe at large, let us consider other situations where we have a gravitational field and a net imbalance in planckion density. We start with the examples of a three, and, four, solar mass black hole. Just outside the surface, we probably will have the largest gravitational fields found in nature. It can be shown quite easily that the gravitational field right outside a black hole is given by,

(5-1)

Use of the Schwarschild condition, , has been employed to reduce the expression to a relation only involving the black hole mass,. The radius of the event horizon is,. We notice that the least massive black holes have the largest gravitational fields, by Equation (5-1). Just like the earth, we assume negligible vacuum susceptibility right outside the black hole. Little enclosed polarized mass is thought to exist within the black hole itself. We substitute the appropriate black hole masses into Equation (5-1), and obtain,

(3 solar mass black hole) (5-2a)

(4 solar mass black hole) (5-2b)

Next we calculate the gravitational field mass densities, just like we did for the earth. See Equation (4-8). Using our new values for surface gravitational fields, indicated in Equations (5-2a, b), we evaluate, , and find,

(3 solar mass black hole) (5-3a)

(4 solar mass black hole) (5-3b)

If we multiply these, , values by, c², we obtain the corresponding gravitational field energy densities on the surface of these black holes. They are the, 1.54E34 J/m³, and, 8.66E33 J/m³, respectively, the same values as mentioned previously, at the end of section IV. Incidentally, the gravitational field mass densities are not that far off from the source mass densities. For the two black holes considered, we obtain,

(3 solar mass black hole) (5-4a)

(4 solar mass black hole) (5-4b)

These mass densities are only about an order of magnitude larger. The exact number is twelve times larger, which can be easily proven for any mass spherically symmetric black hole. Just set up the ratio, , and work out the details, using the defining relations for the variables within the ratio. We find that, , a very interesting result since it is independent of mass or size.

We know from Equation (2-17), that

(5-5)

Therefore, using Equations (5-3a, b), and, (4-4), it is possible to find the planckion number density imbalance. From Equation (5-5), we have

(3 solar mass black hole) (5-6a)

(4 solar mass black hole) (5-6b)

Because of the intense gravitational fields, and the very extreme gravitational field mass densities, we have an enormous imbalance in planckion number density. In one cubic meter on the surface of these black holes expect about, 10E24 - 10E25, more positive mass planckions than negative mass planckions. Like we said, this is probably some sort of upper limit for what three dimensional space will tolerate, as far as gravitational stress is concerned.

For the earth, the numbers are drastically reduced because the surface gravitational field is so much less. We still use Equation (5-5), but now the mass density is given by Equation (4-8). Substituting this value into Equation (5-5), and carrying through the calculation, gives

(5-7)

This is a very interesting result. The imbalance only amounts to approximately 29 more positive mass planckions than negative mass planckions in one cubic meter.

Next we look at the cosmos. There we also have a net macroscopic gravitational field due to the ordinary and polarized matter, which is contained within it. This gravitational field is a smeared average, valid only for distance scales in excess of about, 100 Mpc. Only then is the cosmos fairly homogeneous, and isotropic. The appropriate gravitational field mass density is specified by Equation (4-2). We substitute this value into Equation (5-5), and find,

(average within the cosmos) (5-8)

This is not the lowest value possible. In a void, where there is no source mass present, and thus no polarized mass, the value would be exactly, . But then we would have an undisturbed vacuum. For the very dilute universe we have today, there is an average of one excess positive mass planckion over negative mass planckion, every,.

Why the universe has a net positive mass is unknown. The result in Equation (5-8), may suggest that, when planckions were first formed, there was an excess of positive mass planckions over negative mass planckions. But this would go counter to the Q theory, because as planckion mass was created, so too was planckion charge. And, as far as we can tell, the universe has zero net charge. Somehow between then, when planckions were first created, and now, the imbalance must have formed. And it probably had something to do with the formation of quarks and leptons. Why and how negative mass planckions were used up in this process is unclear.

We close with a quick calculation for the imbalance in terms of absolute planckion numbers. If the Hubble radius in the present epoch is, , then,

(5-9)

For, , we have used Equation (5-8). And for the radius of the observable universe, , we took this to equal, , a value found in reference, [11]. The, , is the present day difference in planckion numbers, with the positive mass planckions being more plentiful than negative mass planckions. This excess, while it appears large, is only an insignificant amount when compared to total planckion numbers. Using Equations (3-17a), or, (3-17b), and Equation (5-8), we see that

(5-10)

This fraction is very minute.

6. Summary and Conclusions

We introduced a model where Planck mass and Planck charge were frozen out of the vacuum simultaneously. We treated mass and charge as two components of a more fundamental particle, the planckion. Based on previous and extensive work by Winterberg, the vacuum is a vast assembly (sea) of positive and negative mass planckions, which form a two-component superfluid and fill all of space. This ether is initially massively, and electrically neutral, has zero net mass density, zero net gravitational pressure, and zero net entropy in the undisturbed state. Within the Winterberg model, we introduce Q theory, the notion that Planck mass and Planck charge were created simultaneously, as well as two force laws, one electrostatic, and one seemingly gravitational in nature. The electrostatic force keeps two planckions of the same species, whether they have positive or negative mass, apart. The gravistatic force brings them together. Thus equilibrium is achieved, where individual planckions maintain a fixed distance of separation from each other within their species. Along with the simultaneous creation of mass and charge, we also posited the simultaneous creation of two force laws. Equation (2-2), shows us how they are connected.

Planckions are anchored, or locked, in position spatially via fluid forces. Equation (2-7), holds for positive mass planckions, and Equation (2-10), is valid for negative mass planckions. Both lead to number density functions, which will tend to bring the planckions back to equilibrium, if displaced. See Equations (2-9), and (2-12). The total gravitational pressure is zero, and so is the energy density, if planckions are undisturbed. See Equations (2-9), and (2-15). If the vacuum is disturbed through the introduction of an applied gravitational field, we obtain Equations (2-17), and, (2-20). We identified the gravitational field energy density, or dark energy, with planckion mass density. This leads to Equation (2-22), relating planckion displacement to gravitational field intensity.

In Section III, we looked at the restoring force acting on individual planckions more carefully and discovered it was entirely electrostatic in origin. In other words, the gravitational force introduced in Section II, within the Q theory, is, in reality, electrostatic in nature, see Equation (3-1). The planckion spring constant could thus be evaluated, and the result is Equation (3-8). Moreover, by appealing to box quantization, and Planck’s radiator formula, we could estimate the average number density for the positive and negative mass planckions in the undisturbed vacuum. Equation (3-17), is the result. This planckion number density holds only in the present cosmological epoch. Given the relation between number density, and mean distance of separation, Equation (3-2), we could determine the nearest neighbor inter-spatial distance between planckions of the same species. Those values are given in Equations (3-16a, b). Numerically, the planckion spring constant equals, , as indicated in Equation (3-18). The vacuum is very stiff, and this spring constant value is also epoch dependent.

In section IV, we considered various examples of planckion displacements. For the cosmos as a whole, there is a net gravitational field due to all the matter contained within it, ordinary and bound, i.e., polarized matter. Due to this smeared gravitational field, the average planckion displacement is a mere, , which is Equation (4-7). For the gravitational field of the earth, on the surface we find a shift in the amount, , Equation (4-9). We derived relations between gravitational potential, and gravitational pressure. Those relationships are presented in Equations (4-17), and, (4-18). An easy way to calculate gravitational potential is through Equation (4-13). It was also recognized that dark energy is synonymous with gravitational field mass density, which is the same as planckion mass density. See Equations (2-17), (4-2), (4-19), and, (4-20). Whenever we have a gravitational field we have a gravitational, or planckion, pressure imposed upon the vacuum. The vacuum is stressed, even here on the surface of the earth, by a minute amount. This is a latent form of energy we argued, and it can only be released under extreme conditions where the gravitational field gets wiped out. See the discussion following Equation (4-20). We also argued that the vacuum has a certain mechanical resiliency, which we estimate lies in the neighborhood of about, 8.66E33 J/m³, to, 1.54E34 J/m³. Gravitational fields beyond that may lead to “gravitic breakdown” or “vacuum breakdown”.

Finally, in section V, we considered the imbalance in planckion mass density between positive and negative mass planckions. Because there is ordinary mass in the universe, a given, and polarized mass in the cosmos, an assumption, we have a net smeared gravitational field which does not vanish for the cosmos as a whole. By Equations (5-5), and, (5-8), this forces us to accept that, in the present epoch, the average positive planckion number density exceeds the average negative mass planckion number density,. There are more positive mass planckions per unit volume than negative. The exact amount has been calculated in Equation (5-8). The reason for this is unclear, although it may have something to do with ordinary matter, made up of quarks and leptons, being formed in the universe. When planckions were first created in the very early universe, there were equal numbers according to the Q-theory.

There is also the intriguing possibility, although not very likely, that there are regions in the universe where planckion mass density, or dark energy, is negative. This would balance the total number densities between positive and negative mass planckions, then, in the early universe, as well as now, in the present epoch. There is, however, no direct observational evidence for this, i.e., negative dark energy does not appear to exist. As such, we are left with Equation (5-8), which shows a net imbalance.

Q-theory explains charge and mass neutrality in the early universe, but it does not explain quasiparticle formation, collective excitations in the Winterberg model. Nor does it explain positive planckion density imbalance over negative planckion density, in the present epoch. Q theory can, however, provide a connection between gravistatic and electrostatic force laws. In the very early universe these were formed simultaneously. The gravistatic force of attraction, upon closer inspection, turned out to be electrostatic in origin.

Work is in progress on a microscopic theory of planckions, which would include scaling behavior upon expansion of the universe.

Acknowledgements

To my family, my parents, my brother, and my children.

Conflicts of Interest

The author declares no conflicts of interest regarding the publication of this paper.

References

[1]	Dirac, P.A.M. (1937) The Cosmological Constants. Nature, 139, 323. https://doi.org/10.1038/139323a0
[2]	Dirac, P.A.M. (1938) A New Basis for Cosmology. Proceedings of the Royal Society of London A, 165, 199-208. https://doi.org/10.1098/rspa.1938.0053
[3]	Dirac, P.A.M. (1974) Cosmological Models and the Large Numbers Hypothesis. Proceedings of the Royal Society of London A, 338, 439-446. https://doi.org/10.1098/rspa.1974.0095
[4]	Jordan, P. (1937) G Has to Be a Field. Naturwissenschaften, 25, 513-517. https://doi.org/10.1007/BF01498368
[5]	Jordan, P. (1949) Formation of Stars and Development of the Universe. Nature, 164, 637-640. https://doi.org/10.1038/164637a0
[6]	Vieweg, F. and Sohn, B. (1966) Heinz Haber: Die Expansion der Erde [The Expansion of the Earth] Unser blauer Planet [Our Blue Planet]. Rororo Sachbuch [Rororo Nonfiction] (Rororo Taschenbuch Ausgabe [Rororo Pocket Edition] ed.). Rowohlt Verlag, Reinbek, 48, 52, 54-55. (In German)
[7]	Kragh, H. (2015) Pascual Jordan, Varying Gravity, and the Expanding Earth. Physics in Perspective, 17, 107-134. https://doi.org/10.1007/s00016-015-0157-9
[8]	Pilot, C. (2019) The Age of the Universe Predicted by a Time Varying G? Journal of High Energy Physics, Gravitation and Cosmology (JHEPGC), 5, 928-934. https://www.scirp.org/Journal/paperinformation.aspx?paperid=94065 https://doi.org/10.4236/jhepgc.2019.53048
[9]	Pilot, C. (2020) Inflation and Rapid Expansion in a Variable G Model. International Journal of Astronomy and Astrophysics (IJAA), 10, 334-345. https://www.researchgate.net/publication/342988030_Inflation_and_Rapid_Expansion_in_a_ Variable_G_Model_slightly_revised_version https://doi.org/10.4236/ijaa.2020.104018
[10]	Pilot, C. (2018) Is Quintessence an Indication of a Time-Varying Gravitational Constant? Journal of High Energy Physics, Gravitation and Cosmology (JHEPGC), 5, 41-81. https://arxiv.org/abs/1803.06431 https://www.scirp.org/journal/paperinformation.aspx?paperid=88999
[11]	Pilot, C. (2021) Does Space Have a Gravitational Susceptibility? A Model for the ΛCDM Density Parameters in the Friedmann Equation. Journal of High Energy Physics, Gravitation, and Cosmology (JHEPGC), 7, 478-507. https://www.researchgate.net/publication/342993272_Does_Space_Have_a_Gravitational_ Susceptibility_A_Model_for_the_Density_Parameters_in_the_Friedmann_Equation https://doi.org/10.4236/jhepgc.2021.72028
[12]	Pilot, C. (2021) Scaling Behavior for the Susceptibility of the Vacuum. International Journal of Astronomy and Astrophysics (IJAA), 11, 11-36. https://www.researchgate.net/publication/342993277_Scaling_Behavior_for_the_Susceptibility_ of_the_Vacuum_in_a_Polarization_Model_for_the_Cosmos https://doi.org/10.4236/ijaa.2021.111002
[13]	Hajdukovic, D. (2011) Is Dark Matter an Illusion Created by the Gravitational Polarization of the Quantum Vacuum? Astrophysics and Space Science, 334, 215-218. https://link.springer.com/article/10.1007/s10509-011-0744-4 https://arxiv.org/ftp/arxiv/papers/1106/1106.0847.pdf https://doi.org/10.1007/s10509-011-0744-4
[14]	Hajdukovic, D. (2015) What If Quantum Vacuum Fluctuations Are Virtual Gravitational Dipoles? Invited Talk The 3rd International Workshop on Antimatter and Gravity University College, London, 4-7 August 2015, 1-9. https://indico.cern.ch/event/361413/contributions/1776293/attachments/1135100/1623933/ WAG_2015_UCL_Hajduk.pdf
[15]	Hajdukovic, D. (2016) Quantum Vacuum as the Cause of the Phenomena Usually Attributed to Dark Matter. https://indico.cern.ch/event/336103/contributions/786848/attachments/1204251/1798916/DM- Hajdukovic.proc.pdf
[16]	Hajdukovic, D. (2020) On the Gravitational Field of a Point-Like Body Immersed in Quantum Vacuum. Monthly Notices of the Royal Astronomical Society, 491, 4816-4828. https://hal.archives-ouvertes.fr/hal-02087886/document
[17]	Winterberg, F. (2003) Planck Mass Plasma Vacuum Conjecture. Zeitschrift für Naturforschung, 58a, 231. https://doi.org/10.1515/zna-2003-0410
[18]	Winterberg, F. (2002) Planck Mass Rotons as Cold Dark Matter and Quintessence. Zeitschrift für Naturforschung, 57a, 202-204. https://doi.org/10.1515/zna-2002-3-414
[19]	Winterberg, F. (1995) Derivation of Quantum Mechanics from the Boltzmann Equation for the Planck Aether. International Journal of Theoretical Physics, 34, 2145-2164. https://doi.org/10.1007/BF00673076
[20]	Winterberg, F. (1998) The Planck Aether Model for a Unified Theory of Elementary Particles. International Journal of Theoretical Physics, 33, 1275-1314. https://doi.org/10.1007/BF00670794
[21]	Winterberg, F. (1993) Physical Continuum and the Problem of a Finitistic Quantum Field Theory. International Journal of Theoretical Physics, 32, 261-277. https://doi.org/10.1007/BF00673716
[22]	Winterberg, F. (1992) Cosmological Implications of the Planck Aether Model for a Unified Field Theory. Zeitschrift für Naturforschung—Physical Sciences, 47a, 1217. https://doi.org/10.1515/zna-1992-1207
[23]	Winterberg, F. (2002) The Planck Aether Hypothesis. Monograph, The C.F. Gauss Academy of Science Press, Reno.
[24]	Kolb, E.W. and Turner, M.S. (1989) The Early Universe. Addison-Wesley, Reading.
[25]	Baumann, D.D. (2015) Lecture Notes on Cosmology. http://theory.uchicago.edu/~liantaow/my-teaching/dark-matter-472/lectures.pdf
[26]	Mather, J.C., et al. (1999) Calibrator Design for the COBE Far-Infrared Absolute Spectrophotometer (FIRAS). The Astrophysical Journal, 512, 511-520. https://doi.org/10.1086/306805
[27]	Husdal, L. (2016) On Effective Degrees of Freedom in the Early Universe. Galaxies, 4, 78. https://doi.org/10.3390/galaxies4040078
[28]	Jackson, J.D. (1999) Classical Electrodynamics. 3rd Edition, John Wiley and Sons Publishing, Hoboken.
[29]	Apéry, R. (1979) Irrationalité de ζ(2) et ζ(3). Astérisque, 61, 11-13.
[30]	Apéry, R. (1981) Interpolation de fractions continues et irrationalité de certaines constants. Bulletin de la section des sciences du C.T.H.S III, 37-53.
[31]	Serway, R., Moses, C. and Moyer, C. (2005) Modern Physics. 3rd Edition, Thomson Learning Inc., Toronto, 260-266.
[32]	Maeder, A., et al. (2018) Planck 2018 Results. VI. Cosmological Parameters.
[33]	Tanabashi, M., et al. (2019) Astrophysical Constants and Parameters. Physical Review D, 98, Article ID: 030001.