
1. Introduction
The relativistic formulation of quantum mechanics for particles with spin half is made with the help of the Dirac equation. However the present author feels that certain consistency checks are needed to see if all the predictions of this equation do not lead to any contradictory results. With this view a few years back [1] we studied the Lorentz transformation properties of zitterbewegung terms which arises while constructing wave packets for the electron. In this note, we study the self consistency properties of a free Dirac particle wave function under an extreme condition when its velocity approaches that of light.
2. The Eigen States of the Dirac Hamiltonian for a Particle with Almost Luminal Velocities
The Dirac formulation of relativistic quantum mechanics introduces some new operators
,
,
and
. We can write the equation in the form [2] (see Equation (1.13) of this famous book)
. (1)
here the operator on the right hand side is identified as the Hamiltonian
(2)
A free particle wave function like that of an electron moving in the
-direction (here
can be identified with the coordinates
commonly used) that is for which
and
are zero can be written as
(3)
where E and
are constants the energy and momentum of the particle respectively and
is related to its velocity (speed) v by the relation
. That Equation (3) is indeed a solution can be checked by verifying
that it satisfies Equation (1). It can also be obtained by means of Lorentz transformation properties of spinor as described in chapter 3 of ref. [2]. Thus
is an eigen state of the Hamiltonian H with eigen value E if we remember the classical relations
and
. This is true for any value of
for example when
that is when
. Thus we write for the limiting state
(4)
It is also possible to check that
is an eigen state of the operator
with eigen value 1 since we have the limiting relation
. Thus
(5)
here we disagree with Bjorken and Drell that the eigen functions of
can be constructed by including both positive and negative energy solutions only (see p. 37 of ref. [2]) and not otherwise. The referee has asked us to elaborate on this disagreement and so we quote the passage from the book “Indeed in constructing eigenfunctions of
we have to include both positive and negative energy
solutions, since the eigenvalues of
are
whereas
, according to (3.29).” Let us first state that not only
but also
since
as they are material particles or antiparticles. Furthermore the velocities of these particles and antiparticles can approach the velocity
of light in vacuum as closely as possible if we increase their respective energies indefinitely. The meaning of eigen states of
are such states whose expectation values of the velocity operator
closely approach
and not that they
are exactly equal to
. Let us take the example of
with the eigen states
,
,
,
and with the respective eigen values 1,
1, −1, −1. Wave functions of material particles and antiparticles as we have explicitly demonstrated in the case of Equation (5) can approach one of these eigen states and hence they should be considered as eigen function of energy as well (besides that of being eigen functions of
) if the concept of limit has any meaning. The present author also feels that wave functions consisting of positive and negative energy solutions that is states where particles and antiparticles are combined together into one packet can approach such an eigen state only in a limiting sense as we demonstrate now. Take for example the superposition of positive and negative energy solution
which can be considered an eigen
function of
provided the space and time variations of the two nonzero entries in this column vector approach each other that is if
or if either A or B is zero. Also more importantly when both A and B are present and when
say then we must have
that is
but this
however is not an eigen state of energy. The question that whether it is possible to put
exactly equal to
and to which we would like to give a negative response is because of the fact that the quantity E whatever be its physical meaning now is again infinity. Since Bjorken and Drell has only made a statement without showing us a method to construct an eigen function (of
) I hope my arguments will be accepted.
From Equations (4) and (5) we obtain
(6)
A direct evaluation on the other hand of the commutator
using the (anti) commutation relations Equation (1.16) of ref. [2] shows that
(7)
If this expression is made is made to operate on
in the appropriate limit we get
which directly contradicts Equation (6) and hence is a self contradiction of the Dirac formulation of quantum mechanics. The reason for this is that the formulation contains unwanted operators.
3. Concluding Remarks
The Dirac equation can be made self consistent by abandoning the correspondence relations with classical relativistic mechanics
and 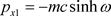 . In this way, some changes in the values of
. In this way, some changes in the values of 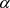 -s and
-s and 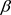 may help in maintaining the forms of Equation (1) and as well as that of the solution that is Equation (3). But then it necessitates that a new meaning be given to the constants E and
may help in maintaining the forms of Equation (1) and as well as that of the solution that is Equation (3). But then it necessitates that a new meaning be given to the constants E and 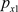 and also the anti-commutation relation
and also the anti-commutation relation 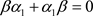 will have to be given up leading to a complete break with the Klein-Gordon equation. One has to make a formulation and show in practice the results that it will lead to.
will have to be given up leading to a complete break with the Klein-Gordon equation. One has to make a formulation and show in practice the results that it will lead to.
Acknowledgements
We would like to thank our Institute for providing funds with which the publication cost of this paper was met. Also we thank the referee for the constructive comments.