Integral Basis of Affine Vertex Algebra V_k (sl₂) and Virasoro Vertex Algebra V_vir (2k,0)

Ashuai Wang

School of Mathematics, South China University of Technology, Guangzhou, China.

DOI: 10.4236/jamp.2020.84050                

Abstract

In this paper, we consider an integral basis for affine vertex algebra V_k (sl₂) when the level k is integral by a direct calculation, then use the similar way to analyze an integral basis for Virasoro vertex algebra V_vir (2k,0). Finally, we take the combination of affine algebras and Virasoro Lie algebras into consideration. By analogy with the construction of Lie algebras over Z using Chevalley bases, we utilize the Z-basis of L_av whose structure constants are integral to find an integral basis for the universal enveloping algebra of it.

Keywords

Vertex algebra, Integral Basis, Virasoro Algebra, Affine Algebra

1. Introduction

While vertex algebras are usually assumed to be vector spaces over $ℂ$ , the most important formula Jacobi identity makes sense over any commutative ring, so it is natural to consider vertex algebras over $\mathbb{Z}$ . An integral basis of a vertex algebra could be considered an analogue of the Chevalley basis in a Lie algebra. Similar to the construction of Lie algebras over $\mathbb{Z}$ using Chevalley bases, we can create vertex algebras over $\mathbb{Z}$ . Integral bases for vertex operator algebras associated with lattices have been studied in [1] [2]. In this paper, we are going to investigate integral basis for affine vertex algebras and Virasoro vertex algebra. Let $\mathfrak{g}$ be a simple Lie algebra over $ℂ$ and $\hat{\mathfrak{g}}$ be the corresponding affine Kac-Moody algebra. The vacuum module $V_{\hat{\mathfrak{g}}}\left(k,0\right)$ at level k has a vertex algebra structure [3] [4] [5], we call it affine vertex algebra. We want to find an integral basis for it when $\mathfrak{g}=\mathfrak{s}{\mathfrak{l}}_2$ and $k$ is an integer in Section 3.

Next, we consider the Virasoro vertex algebra. Among the most important vertex algebras are those associated with the Virasoro Lie algebra. It has been studied in [6] [7] [8]. They show that it is generated by the conformal vector $\omega$ and is minimal in the sense that it does not have any proper vertex operator subalgebra. Besides, any minimal vertex operator algebras of the same central charge are isomorphic. The study of Virasoro vertex algebras is the algebraic foundation of the study of the “minimal modules” in conformal field theory [9]. We use the similar way to analyze an integral basis for Virasoso vertex algebra $V_{Vir}\left(2k,0\right)$ when the level is 2k, where $k$ is an integer.

We know that affine Lie algebra and Virasoro Lie algebra have close relationship in physics, so we consider them simultaneously, i.e., as one algebraic structure. Then the definition of affine-Virasoro was introduced [10] [11], which is the semidirect product of the Virasoro algebra and an affine Kac-Moody Lie algebra with a common center. In the last section, we get an integral basis for the universal enveloping algebra of it.

In this paper, we observe that the $ℂ$ -basis of affine vertex algebra $V_k\left(\mathfrak{s}{\mathfrak{l}}_2\right)$ and Virasoro vertex algebra $V_{Vir}\left(2k,0\right)$ may be integral basis for them in certain conditions. We create the conditions and confirm that they are exactly the integral bases. Then we utilize the analogue of Chevalley bases for finite dimensional Lie algebras to get an integral basis for the universal enveloping algebra of affine-Virasoro algebra.

2. Preliminaries

We assume that the readers are familiar with the theory of vertex operator algebras [3] [6] [12] [13].

Given an (untwisted) affine Lie algebra $\hat{\mathfrak{g}}=\mathfrak{g}\otimes ℂ\left[t,t^{-1}\right]\oplus ℂk$ equipped with the bracket relation,

$\left[a\otimes t^m,b\otimes t^n\right]=\left[a,b\right]\otimes t^{m+n}+m\langle a,b\rangle {\delta }_{m+n,0}k$

for $a,b\in \mathfrak{g}$ and $m,n\in \mathbb{Z}$ , together with the condition that $k$ is a nonzero central element of $\hat{\mathfrak{g}}$ . Let $k\in ℂ$ , ${\hat{\mathfrak{g}}}_{(-)}$ and $\mathfrak{g}$ act trivially on $ℂ$ and let $k$ act as the scalar k, making $ℂ$ a ${\hat{\mathfrak{g}}}_{\left(\le 0\right)}$ -module, which we denote by

$V_{\hat{\mathfrak{g}}}\left(k,0\right)=U\left(\hat{\mathfrak{g}}\right){\otimes }_{U\left({\hat{\mathfrak{g}}}_{\left(\le 0\right)}\right)}{ℂ}_k$ .

By the Poincaré-Birkhoff-Witt theorem, we have that,

$V_{\hat{\mathfrak{g}}}\left(k,0\right)=U\left({\hat{\mathfrak{g}}}_{(+)}\right)\simeq S\left({\hat{\mathfrak{g}}}_{(+)}\right)$ (2.1)

as a 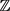-graded vector space. Set

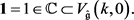
Then

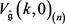is spanned by the vectors

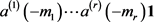
for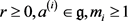, with. It can be proved that this is a vertex algebra, more detail can be found in [5]. The vertex algebra structure is determined by

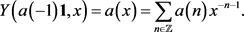
Now we have that,

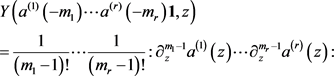
where 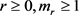for.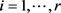

In the next section, we will consider the integral basis of it when 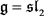and 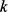is an integer.

3. Integral Basis of

In this section shall find an integral basis for. We consider the case when 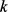is an integral number. Firstly, we recall the definition of integral basis of a vertex algebra.

Definition 3.1. Suppose that 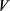is a vertex algebra (over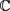), an integral basis of it is its -basis whose -span can form a vertex algebra over.

In order to find an integral basis for, we may firstly find an integral basis for. That is, we need to find a basis of whose -span is closed under the bracket. When, it is easy to see that the standard basis elements satisfy the condition. Now let be the standard basis of, we choose an ordered basis of, that is

where. Then by (2.1), we get that

(3.1)

is a -basis of.

For convenience, we denote by V, denote the -span of a -basis of by.

Theorem 3.2. The formula (3.1) is an integral basis of.

Proof. It is known that formula (3.1) is a -basis of. To check that is a vertex algebra, we need to prove that the coefficients are still in of any

(3.2)

for. Since is spanned by (3.1) over, we just need to check formula (3.2) for (3.1). So

Since, , , , the expression

where, is a -linear combination of (3.1), we get that (3.1) is an integral basis of.

4. Integral Basis of Virasoro Vertex Algebra

In this section we shall find an integral basis for the Virasoro vertex algebra when the level is 2k, where is an integer.

Firstly we recall the definition of Virasoro vertex algebra [6] [14] [15]. As we know, any vertex operator algebra has the vertex subalgebra generated by the conformal vector, and this is in fact the smallest vertex operator subalgebra of; it is exactly the submodule of for the Virasoro algebra generated by:

(4.1)

The Virasoro algebra is the Lie algebra with basis equipped with the bracket relations,

(4.2)

together with the condition that is a central element of. The Virasoro algebra equipped with the -grading

(4.3)

where

(4.4)

is a -graded Lie algebra, and this grading is given by -eigenvalus. For the Virasoro algebra, we have the graded subalgebras

(4.5)

We also have the graded subalgebras

(4.6)

Let be any complex number. Consider as an -module with acting as the scalar and with acting trivially. Denote this -module by. Then we form the induced module

(4.7)

From the Poincaré-Birkhoff-Witt theorem, as a vector space,

(4.8)

Set

Then

(4.9)

where has a basis consisting of the vectors

(4.10)

for, , with. It can be proved that this is a vertex operator algebra, details can be found in [3]. The vertex algebra structure is determined by

and we have

where.

Next, we will consider the integral basis of it when acts as 2k and k is integral. We know that

is a basis of. Then

with, , with, is a -basis of. We want to check that it is a -basis of. When acts as 2k, we get that,

for any.

Just like the affine case, we have that,

where. Since

and

the expression

is a -linear combination of, where, , with, so is an integral basis of.

5. Integral Basis for the Universal Enveloping Algebra of Affine-Virasoro Algebra

In this section we take the combination of affine algebras and Virasoro Lie algebras into consideration. By analogy with the construction of Lie algebras over using Chevalley bases, we utilize the -basis of it whose structure constants are integral to find an integral basis for the universal enveloping algebra of affine-Virasoro algebra when.

Firstly we recall the definition of the affine-Virasoro algebra [15].

Definition 5.1. Let L be a finite-dimensional Lie algebra with a non-degenerated invariant normalized symmetric bilinear form, then the affine-Virasoro Lie algebra is the vector space

with Lie bracket:

(5.1)

where.

Now we consider the case of. Then by Definition (5.1), we get that the corresponding affine-Virasoro algebra, with Lie bracket:

where.

Lemma 5.2. If is a -basis of, then

is an integral basis of.

Proof. Since is a -basis of, we can get that all of the structure constants are integral, then we get the conclusion.

Corollary 5.3. Let, the integral basis for the universal enveloping algebra of affine-Virasoro algebra is

where.

Proof. We only need to check that is a -basis of. By relations (5.1), all coefficients of these brackets are integral except. Now we use to replace C, we can get,

Since

we conclude that is a Chevalley basis of, then we have proved this corollary.

6. Conclusion

In this paper, we get the integral basis for, and. The constructions of affine vertex algebra and Virasoro vertex algebra are key to our proof. Lemma (5.2) is essential in finding integral basis for. Approaches used here can be easily generalized to tensor product of vertex algebras and universal enveloping algebras. We can also generalize to general.

Conflicts of Interest

The author declares no conflicts of interest regarding the publication of this paper.

References

[1]	Dong, C. and Griess, R. (2012) Integral Forms in Vertex Operator Algebras Which Are Invarant under Finite Groups. Journal of Algebra, 365, 184-198. https://doi.org/10.1016/j.jalgebra.2012.05.006
[2]	Prevost, S. (1992) Vertex Algebras and Integral Bases for the Enveloping Algebras of Affine Lie Algebras. Memoirs of the American Mathematical Society, Providence. https://doi.org/10.1090/memo/0466
[3]	Frenkel, E. and Ben-Zvi, D. (2001) Vertex Algebras and Algebraic Curves. Mathematical Surveys and Monographs, American Mathematical Society, Providence.
[4]	Kac, V.G. (1998) Vertex Algebras for Beginners. 2nd Edition, Univ. Lecture Ser., American Mathematical Society, Providence. https://doi.org/10.1090/ulect/010
[5]	Lepowsky, J. and Li, H. (2003) Introduction to Vertex Operator Algebras and Their Representations. Progress in Mathematics, Birkhäuser, Boston. https://doi.org/10.1007/978-0-8176-8186-9
[6]	Frenkel, I.B. and Zhu, Y. (1992) Vertex Operator Algebras Associated to Representations of Affine and Virasoro Algebras. Duke Mathematical Journal, 66, 123-168. https://doi.org/10.1215/S0012-7094-92-06604-X
[7]	Li, H. (1996) Local Systems of Vertex Operators, Vertex Superalgebras and Modules. Journal of Pure and Applied Algebra, 109, 143-195. https://doi.org/10.1016/0022-4049(95)00079-8
[8]	Wang, W. (1993) Rationality of Virasoro Vertex Operator Algebras. Duke Mathematical Journal, 7, 197-211. https://doi.org/10.1155/S1073792893000212
[9]	Belavin, A., Polyakov, A. and Zamolodchikov (1984) Infinite Conformal Symmetries in Two-Dimensional Quantum Field Theory. Nuclear Physics, 241, 333-380. https://doi.org/10.1016/0550-3213(84)90052-X
[10]	Kac, V.G. and Raina, A.K. (1987) Bombay Lectures on Highest Weight Representations of Infinite Dimensional Lie Algebras. World Scientific, Singapore. https://doi.org/10.1142/0476
[11]	Kuroki, G. (1991) Fock Space Representations of Affine Lie Algebras and Integral Representations in the Wess-Zumino-Witten Models. Communications in Mathematical Physics, 142, 511-542. https://doi.org/10.1007/BF02099099
[12]	Dong, C. and Lepowsky, J. (1993) Generalized Vertex Algebras and Relative Vertex Operators. Birkhäuser, Boston. https://doi.org/10.1007/978-1-4612-0353-7
[13]	Frenkel, I.B., Huang, Y.-Z. and Lepowsky, J. (1993) On Axiomatic Approaches to Vertex Operator Algebras and Modules. Memoirs of the American Mathematical Society, Providence. https://doi.org/10.1090/memo/0494
[14]	Frenkel, I.B., Lepowsky, J. and Meurman, A. (1988) Vertex Operator Algebras and the Monster. Pure and Applied Mathematics Vol. 134. Academic Press, New York.
[15]	Gao, Y., Hu, N. and Liu, D. (2016) Representations of the Affine-Virasoro Algebra of Type A(1) 1. Journal of Geometry and Physics, 106, 102-107. https://doi.org/10.1016/j.geomphys.2016.03.009