Exact Travelling Wave Solutions of Two Nonlinear Schrödinger Equations by Using Two Methods ()
1. Introduction
The nonlinear PDE is an important model for describing the problems of Nonlinear phenomenon, such as hydrodynamics, plasma physics, chemical dynamics, photobiology, solid physics, Marine and atmospheric phenomena, and so on. It can be seen from these fields that the travelling wave solutions of nonlinear evolution equations play an important role in the study. In order to find the exact solutions of nonlinear partial differential Equations (PDEs), pioneers presented the following these methods, such as the first integral method [1], Jacobi elliptic function expansion method [2], F expansion method [3], exp-function method [4], the Kudryashov method [5], the improved
-expansion method [6], the tanh-coth method [7], tanh-sech method [8], projective Riccati equation method [9], Kudryashov method [10], sine-cosine method [11], Hirota bilinear method [12], bifurcation theory method of dynamic systems [13] and so on.
In this article, we consider the higher order dispersive Cubic-quintic nonlinear Schrödinger Equation (NLSE), see [14] and the generalized nonlinear Schrödinger Equation (GNLSE), see [15] :
(1)
and
(2)
where
are real constants. q, u are complex functions.
In 2014, Kudryashov [16] substantiated that the
-expansion method together with the linear ordinary differential equation
is identical to the well-known tanh-method. Furthermore, In 2014, Alam and Akbar [17] [18] researched extremely significant extension of the
-expansion method to receive exact travelling wave solutions of nonlinear evolution equations, For the new mapping method, scholars introduced this method, see [19] [20] [21], and gave the specific solving process for nonlinear PDE.
For the higher order dispersive Cubic-quintic NLSE, In 2017, Zayed and Nowehy [22] incorporated the solution Ansatz method with the Jacobi elliptic equation method to obtain several integrations denoted Jacobi elliptic function of the equation. In 2017, Arshad, sedawy and Lu [23] used an improved direct algebraic extension method to present bright and dark wave solutions and soliton wave solutions of higher order dispersive Cubic-quintic NLSEs. In addition, there is an amount of paper [24] [25] [26] where the various types of the equation are studied. For the GNLSE, In 2010, Geng and Li by using the dynamic system method and bifurcation theory, studies the travelling wave solution of the GNLSE and high order dispersion NLSE. the solitary wave solutions, kink and reverse kink wave solutions and periodic wave solutions are obtained. In 2007, Huang, Li and Zhang [27] through the study a class of nonlinear term six times of first order nonlinear ODE and applies it to the GNLSE. New accurate traveling wave solutions, such as light and dark isolated wave solutions, triangular periodic wave solutions and singular solutions are obtained. In addition, this GNLSE was studied, see [28] [29] [30].
The rest of the article is organized as follows: Section 2, we mainly describe the basic idea of the special kind of
-expansion method and the new mapping method briefly. In Section 3 and 4, we use these two methods to solve two NLSEs in detail. Some conclusions are drawn in Section 4.
2. Introduction of Two Methods
Method 1: The special kind of
-expansion method.
Consider the general nonlinear PDE of the form:
(3)
where P is a polynomial in its arguments.
In order to transform the Equation (3) into an ODE, we suppose that
(4)
where c is a constant, then
(5)
Step 1: According to above supposing, the Equation (3) has the following nonlinear ODE form:
(6)
where the subscript denotes the derivation with respect to
.
Step 2: Suppose that the Equation (6) has non-integer balance number N. the solution of the Equation (6) can be written in the following special form, see [31] [32] [33] :
(7)
where
satisfies the linear ODE:
(8)
Step 3: Firstly, determining the balance number N by balancing the high order derivative and the highest power of the nonlinear term in Equation (6).
Step 4: Then, substituting the Equations (7) and (8) into the Equation (6), and make the coefficients of
all zero, and get a set of algebraic equations, which can be solved by Maple software to find
.
Step 5: Finally by solving Equation (8) for
ratio, the Equation (3) exact solutions are obtained.
Method 2: The new mapping method.
Step 1: We suppose that the Equation (6) has the formal solution:
(9)
where F is an appropriate variable transformation, and
satisfies the following equation:
(10)
where
are arbitrary constant to be determined.
Step 2: It can be seen from the solution [34] that the Equation (9) has the formal solutions with
.
(11)
(12)
(13)
(14)
(15)
(16)
(17)
(18)
(19)
(20)
(21)
(22)
(23)
(24)
(25)
(26)
 (27)
(27)
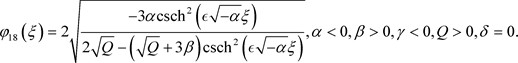 (28)
(28)
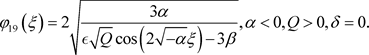 (29)
(29)
 (30)
(30)
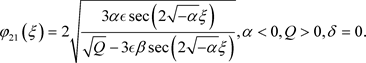 (31)
(31)
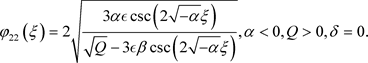 (32)
(32)
 (33)
(33)
where .
.
Step 3: Substituting the solutions (11) - (33) into Equation (9) to get the exact solutions of Equation (3).
3. Application of the Special Kind of  -Expansion Method
-Expansion Method
In this section, we apply the special kind of 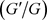 -expansion method to solve the two higher order NLSE.
-expansion method to solve the two higher order NLSE.
Firstly, for Equation (1). We suppose that the Equation (1) has the following solution:
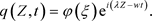 (34)
(34)
where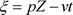 . And
. And 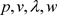 are real parameters,
are real parameters, 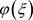 is real function.
is real function.
Substituting Equation (34) into Equation (1), and putting imaginary and real part are zero respectively:
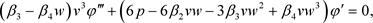 (35)
(35)
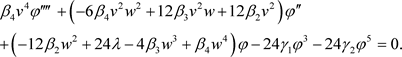 (36)
(36)
Differentiating Equation (35) once, and substituting the resultant equation into Equation (36), we let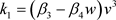 ,
,  ,
, ![]() and
and![]() . then we can get
. then we can get
![]() (37)
(37)
Multiplying (37) by ![]() and integrating once with respect to
and integrating once with respect to![]() , we have the auxiliary equation:
, we have the auxiliary equation:
![]() (38)
(38)
where![]() ,
, ![]() ,
, ![]() ,
, ![]() , and
, and ![]() is the integral constant.
is the integral constant.
Secondly, for Equation (2), We suppose that the Equation (2) has the following solution:
![]() (39)
(39)
where![]() . And
. And ![]() are real parameters.
are real parameters.
Substituting Equation (39) into Equation (2), and putting real and imaginary part are zero respectively:
![]() (40)
(40)
![]() (41)
(41)
Integrating Equation (40) once, and substituting the resultant equation into Equation (41),
![]() (42)
(42)
Multiplying (42) by ![]() and integrating once with respect to
and integrating once with respect to![]() , we have the auxiliary equation:
, we have the auxiliary equation:
![]() (43)
(43)
If we make again![]() ,
, ![]() ,
, ![]() ,
, ![]() , and
, and ![]() is the integral constant.
is the integral constant.
Then, Observed Equations (38) and (43), to find the exact solutions of the them, we only need to discuss one of these equations. Next, we will give the solving process of the Equation (38).
Now we will use the special ![]() expansion method to solve Equation (38), therefore, according to Section 2 as follows: Balancing
expansion method to solve Equation (38), therefore, according to Section 2 as follows: Balancing ![]() with
with ![]()
yields![]() , then Equation (38) solution has the following solution:
, then Equation (38) solution has the following solution:
![]() (44)
(44)
where ![]() is the constant to be determined,
is the constant to be determined, ![]() satisfy Equation (8), Substituting Equation (44) and Equation (8) into Equation (38), we get the following equation:
satisfy Equation (8), Substituting Equation (44) and Equation (8) into Equation (38), we get the following equation:
![]() (45)
(45)
By comparing the power coefficient of the Equation (45), we can get the algebraic equations:
![]() (46)
(46)
Using Maple solving them, we can obtain the following coefficients:
![]() (47)
(47)
Then, the new exact solution of Equation (38) is:
![]() (48)
(48)
where, ![]() and
and ![]() are arbitrary integral constants and
are arbitrary integral constants and![]() .
.
In particular, If we choose![]() , then we can get the dark soliton solutions of Equation (38):
, then we can get the dark soliton solutions of Equation (38):
![]() (49)
(49)
If we choose![]() , then we can get the singular soliton solutions Equation (38):
, then we can get the singular soliton solutions Equation (38):
![]() (50)
(50)
where satisfy the constraint condition:![]() ,
, ![]() , and
, and![]() .
.
4. Application of the New Mapping Method
In this section, we apply the new mapping method to solve the two higher order NLSE.
Firstly, we rewrite the Equation (38) to take the following form:
![]() (51)
(51)
where![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() is the integral constant.
is the integral constant.
According to Section 2, the method is applied to Equation (51), then the solution of Equation (51) is obtained as follows:
1) If![]() , Then, we derive from Equation (11) and Equation (12) that Equation (51) has the solitary wave solutions:
, Then, we derive from Equation (11) and Equation (12) that Equation (51) has the solitary wave solutions:
![]() (52)
(52)
and
![]() (53)
(53)
where satisfy the constraint condition:![]() ,
, ![]() ,
, ![]() , and
, and![]() .
.
2) If![]() , Then, we derive from Equation (13) and Equation (14) that Equation (51) has the periodic solutions:
, Then, we derive from Equation (13) and Equation (14) that Equation (51) has the periodic solutions:
![]() (54)
(54)
and
![]() (55)
(55)
where satisfy the constraint condition:![]() ,
, ![]() ,
, ![]() , and
, and![]() .
.
3) If![]() , Then, we derive from Equation (15) and Equation (16) that Equation (51) has the Dark soliton solutions:
, Then, we derive from Equation (15) and Equation (16) that Equation (51) has the Dark soliton solutions:
![]() (56)
(56)
and the singular soliton solutions:
![]() (57)
(57)
where satisfy the constraint condition:![]() ,
, ![]() ,
, ![]() , and
, and![]() .
.
4) If![]() , Then, we derive from Equation (17) and Equation (18) that Equation (51) has the solitary wave solutions:
, Then, we derive from Equation (17) and Equation (18) that Equation (51) has the solitary wave solutions:
![]() (58)
(58)
and the singular soliton solutions:
![]() (59)
(59)
where satisfy the constraint condition:![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() , and
, and![]() .
.
5) If![]() , Then, we derive from Equation (19) and Equation (20) that Equation (51) has the solitary wave solutions:
, Then, we derive from Equation (19) and Equation (20) that Equation (51) has the solitary wave solutions:
![]() (60)
(60)
and
![]() (61)
(61)
where the Equation (60) satisfy the constraint condition:![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() , and
, and![]() .
.
where the Equation (61) satisfy the constraint condition:![]() ,
, ![]() ,
, ![]() ,
,![]() ,
, ![]() ,
, ![]() , and
, and![]() .
.
6) If![]() , Then, we derive from Equation (21) and Equation (22) that Equation (51) has the periodic solutions:
, Then, we derive from Equation (21) and Equation (22) that Equation (51) has the periodic solutions:
![]() (62)
(62)
and
![]() (63)
(63)
where satisfy the constraint condition:![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() , and
, and![]() .
.
7) If![]() , Then, we derive from Equation (23) and Equation (24) that Equation (51) has the bright soliton solutions:
, Then, we derive from Equation (23) and Equation (24) that Equation (51) has the bright soliton solutions:
![]() (64)
(64)
where satisfy the constraint condition:![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() , and
, and![]() .
.
8) If![]() , Then, we derive from Equation (25) and Equation (26) that Equation (51) has the bright soliton solutions:
, Then, we derive from Equation (25) and Equation (26) that Equation (51) has the bright soliton solutions:
![]() (65)
(65)
and the singular soliton solutions:
![]() (66)
(66)
where satisfy the constraint condition:![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() , and
, and![]() .
.
9) If![]() , Then, we derive from Equation (27) and Equation (28) that Equation (51) has the periodic solutions:
, Then, we derive from Equation (27) and Equation (28) that Equation (51) has the periodic solutions:
![]() (67)
(67)
and
![]() (68)
(68)
where satisfy the constraint condition:![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() , and
, and![]() .
.
10) If![]() , Then, we derive from Equation (29) and Equation (30) that Equation (51) has the periodic solutions:
, Then, we derive from Equation (29) and Equation (30) that Equation (51) has the periodic solutions:
![]() (69)
(69)
and
![]() (70)
(70)
where satisfy the constraint condition:![]() ,
, ![]() ,
, ![]() ,
, ![]() ,
, ![]() , and
, and![]() .
.
5. Conclusion
The special kind of ![]() -expansion, the new mapping method successfully solved the higher order dispersion nonlinear schrodinger equation and the generalized nonlinear schrodinger equation, and new exact travelling wave solutions are obtained. It includes the solitary wave solutions, singular soliton solutions, bright and dark soliton solutions and periodic solutions. Compared with other methods, it is an effective method to solve the exact traveling wave solution, therefore, this method can be extended to solve other nonlinear PDEs.
-expansion, the new mapping method successfully solved the higher order dispersion nonlinear schrodinger equation and the generalized nonlinear schrodinger equation, and new exact travelling wave solutions are obtained. It includes the solitary wave solutions, singular soliton solutions, bright and dark soliton solutions and periodic solutions. Compared with other methods, it is an effective method to solve the exact traveling wave solution, therefore, this method can be extended to solve other nonlinear PDEs.