Multiple Solutions for an Elliptic Equation with Hardy-Sobolev Critical Exponent, Hardy-Sobolev-Maz’ya Potential and Sign-Changing Weights

Mohammed El Mokhtar Ould El Mokhtar, Zeid I. Almuhiameed

Departement of Mathematics, College of Science, Qassim University, Buraidah, Saudi Arabia.

DOI: 10.4236/jamp.2019.711181                

Abstract

In the present paper, an elliptic equation with Hardy-Sobolev critical exponent, Hardy-Sobolev-Maz’ya potential and sign-changing weights, is considered. By using the Nehari manifold and mountain pass theorem, the existence of at least four distinct solutions is obtained.

Keywords

Hardy-Sobolev-Maz’ya Potential, Concave Term, Sign-Changing Weights, Nehari Manifold, Mountain Pass Theorem

1. Introduction

In this paper, we consider the multiplicity results of nontrivial nonnegative solutions of the following problem ()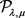

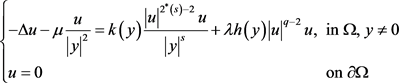
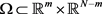
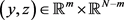where each point x in is written as a pair where m and N are integers such that 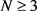and m belongs to, 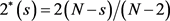with 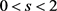is the critical Hardy-Sobolev critical exponent, 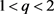, , 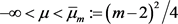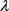is a real parameter and 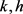are continuous functions which change sign in.

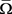In recent years, many auteurs have paid much attention to the following singular elliptic problem, i.e., the case 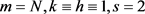in (),

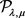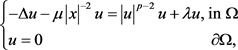(1)

where is a smooth bounded domain in (), , , and is the critical Sobolev exponent, see [1] [2] [3] and references therein. The quasilinear form of (1) is discussed in [4]. Some results are already available for (). Wang and Zhou [5] proved that there exist at least two solutions for () with, , Bouchekif and Matallah [6] showed the existence of two solutions of () under certain conditions on a weighted function h, when, with a positive constant.

Our motivation of this study is the fact that such equations arise in the search for solitary waves of nonlinear evolution equations of the Schrodinger or Klein-Gordon type. Roughly speaking, a solitary wave is a nonsingular solution, which travels as a localized packet in such a way that the physical quantities corresponding to the invariances of the equation are finite and conserved in time. Accordingly, a solitary wave preserves intrinsic properties of particles such as the energy, the angular momentum, and the charge, whose finiteness is strictly related to the finiteness of the norm. Owing to their particle-like behavior, solitary waves can be regarded as a model for extended particles and they arise in many problems of mathematical physics, such as classical and quantum field theory, nonlinear optics, fluid mechanics, and plasma physics.

Concerning existence results in the case, we cite [7] [8] [9] [10] and the references therein. Musina [10] considered () with, also (). She established the existence of a ground state solution when and for () with. She also showed that () with does not admit ground state solutions. Badiale et al. [11] studied () with. They proved the existence of at least a nonzero nonnegative weak solution u, satisfying when and. Bouchekif and El Mokhtar [12] proved that () admits two distinct solutions when, with, , and where is a positive constant. Terracini [5] proved that there is no positive solutions of () with when. The regular problem corresponding to has been considered on a regular bounded domain by Tarantello [13]. She proved that, with a nonhomogeneous term, the dual of, not identically zero and satisfying a suitable condition, the problem considered admits two distinct solutions.

Before formulating our results, we give some definitions and notations.

We denote by and, the closure of with respect to the norms

and

respectively, with for.

From the Hardy-Sobolev-Maz’ya inequality, it is easy to see that the norm is equivalent to. More explicitly, we have

with and for all.

We list here a few integral inequalities.

The starting point for studying (), is the Hardy inequality with cylindrical weights [10]. It states that

(2)

Since our approach is variational, we define the functional J on by

A point is a weak solution of the equation () if it satisfies

here denotes the product in the duality, (dual of).

Let

From [14], is achieved.

We consider the following assumptions:

(K) k is a continuous function defined in and satisfies, ,

(H) h is a continuous function defined in and there exist and positive such that for all.

In our work, we research the critical points as the minimizers of the energy functional associated to the problem () on the constraint defined by the Nehari manifold, which are solutions of our system.

Let be positive number such that

Now we can state our main results.

Theorem 1. Assume that, and verifying , then the system () has at least one positive solution.

Theorem 2. In addition to the assumptions of the Theorem 1, there exists such that if satisfying, then () has at least two positive solutions.

Theorem 3. In addition to the assumptions of the Theorem 2, assuming, there exists a positive real such that, if satisfy, then () has at least two positive solutions and at least one pair of sign-changing solutions.

This paper is organized as follows. In Section 2, we give some preliminaries. Section 3 and 4 are devoted to the proofs of Theorems 1 and 2. In the last Section, we prove the Theorem 3.

2. Preliminaries

Definition 1. Let, E a Banach space and.

1) is a Palais-Smale sequence at level c (in short (PS)_c) in E for I if

where tends to 0 as n goes at infinity.

2) We say that I satisfies the (PS)_c condition if any (PS)_c sequence in E for I has a convergent subsequence.

Lemma 1. Let X Banach space, and verifying the Palais-Smale condition. Suppose that and that:

1) there exist, such that if, then;

2) there exist such that and;

let where

then c is critical value of J such that.

Nehari Manifold

It is well known that J is of class in and the solutions of () are the critical points of J which is not bounded below on. Consider the following Nehari manifold

Thus, if and only if

(3)

Note that contains every nontrivial solution of the problem (). Moreover, we have the following results.

Lemma 2. J is coercive and bounded from below on.

Proof. If, then by (3) and the Hölder inequality, we deduce that

(4)

Thus, J is coercive and bounded from below on.

Define

Then, for

(5)

Now, we split in three parts:

We have the following results.

Lemma 3. Suppose that is a local minimizer for J on. Then, if, is a critical point of J.

Proof. If is a local minimizer for J on, then is a solution of the optimization problem

Hence, there exists a Lagrange multipliers such that

Thus,

But, since. Hence. This completes the proof.

Lemma 4. There exists a positive number such that for all, verifying

we have.

Proof. Let us reason by contradiction.

Suppose such that. Then, by (5) and for, we have

Moreover, by the Hölder inequality and the Sobolev embedding theorem, we obtain

(6)

and

(7)

From (6) and (7), we obtain, which contradicts an hypothesis.

Thus. Define

For the sequel, we need the following Lemma.

Lemma 5. 1) For all such that, one has;

2) For all such that, one has

Proof. 1) Let. By (5), we have

and so

We conclude that.

2) Let. By (5), we get

Moreover, by Sobolev embedding theorem, we have

This implies

(8)

By (4), we get

Thus, for all such that

we have.

Proposition 1. (see [15] ) 1) For all such that, there exists a sequence in.

2) For all such that, there exists a a sequence in .

We define:

and for each with, we write

Lemma 6. Let real parameters such that. For each we have:

1) If then there exists unique such that and

2) If then there exist unique and such that , , and

3) If, then does not exist such that.

4) If, then there exists unique such that

and

Proof. With minor modifications, we refer to [15].

3. Proof of Theorem 1

Now, taking as a starting point the work of Tarantello [16], we establish the existence of a local minimum for J on.

Proposition 2. For all such that, the functional J has a minimizer and it satisfies:

(i);

(ii) is a nontrivial solution of ().

Proof. If, then by Proposition 1, (i) there exists a - sequence in, thus it bounded by Lemma 2. Then, there exists and we can extract a subsequence.

Which will denoted by such that

(9)

Thus, by (9), is a weak nontrivial solution of (). Now, we show that converges to strongly in. Suppose otherwise. By the lower semi-continuity of the norm, then either and we obtain

We get a contradiction. Therefore, converge to strongly in. Moreover, we have. If not, then by Lemma 6, there are two numbers and, uniquely defined so that and. In particular, we have. Since

there exists such that. By Lemma 6, we get

which contradicts the fact that. Since and , then by Lemma 3, we may assume that is a nontrivial nonnegative solution of (). By the Harnack inequality, we conclude that, see for example [17].

4. Proof of Theorem 2

Next, we establish the existence of a local minimum for J on. For this, we require the following Lemma.

Lemma 7. For all such that, the functional J has a minimizer in and it satisfies:

(i);

(ii) is a nontrivial solution of () in.

Proof. If, then by Proposition 1, (ii) there exists a, sequence in, thus it bounded by Lemma 2. Then, there exists and we can extract a subsequence which will denoted by such that

This implies

Moreover, by (5) we obtain

(10)

By (6) and (10) there exists a positive number

such that

(11)

This implies that

Now, we prove that converges to strongly in. Suppose otherwise. Then, either. By Lemma 6 there is a unique such that. Since

we have

and this is a contradiction. Hence,

Thus,

Since and, then by (11) and Lemma 3, we may assume that is a nontrivial nonnegative solution of (). By the maximum principle, we conclude that.

Now, we complete the proof of Theorem 2. By Propositions 2 and Lemma 7, we obtain that () has two positive solutions and. Since, this implies that and are distinct.

5. Proof of Theorem 3

In this section, we consider the following Nehari submanifold of

Thus, if and only if

Firsly, we need the following Lemmas:

Lemma 8. Under the hypothesis of theorem 3, there exist, such that is nonempty for any and.

Proof. Fix and let

Clearly and as. Moreover, we have

If for, then there exist

and such that. Thus, and is nonempty for any.

Lemma 9. There exist positive reals such that, for and any verifying

Proof. Let, then by (3), (5) and the Holder inequality, allows us to write

Thus, if

and choosing with defined in Lemma 8, then we obtain that

(12)

Lemma 10. Suppose and. Then, there exist and positive constants such that

1) we have

2) there exists when, with, such that.

Proof. We can suppose that the minima of J are realized by and. The geometric conditions of the mountain pass theorem are satisfied. Indeed, we have:

1) By (5), (12) and the fact that we get

By the fact that and and, we obtain that

2) Let, then we have for all

Letting for t large enough, we obtain. For t large enough we can ensure.

Let and c defined by

and

Proof of Theorem 3.

If

then, by the Lemmas 2 and Proposition 1 2), J verifying the Palais-Smale condition in. Moreover, from the Lemmas 3, 9 and 10, there exists such that

Thus is the third solution of our system such that and. Since () is odd with respect u, we obtain that is also a solution of ().

6. Conclusion

In our work, we have searched the critical points as the minimizers of the energy functional associated with the problem on the constraint defined by the Nehari manifold, which are solutions to our problem. Under some sufficient conditions on coefficients of equation of (2), we split in two disjoint subsets and thus we consider the minimization problems on and respectively. In Sections 3 and 4, we have proved the existence of at least two nontrivial solutions on for all. In the perspectives we will try to find more non-trivial solutions by splitting again the sub-varieties of Nehari.

Acknowledgements

The authors gratefully acknowledge Qassim University, represented by the Deanship of Scientific Research, on the material support for this research under the number (1030) during the academic year 1441AH/2019AD.

Conflicts of Interest

The authors declare no conflicts of interest regarding the publication of this paper.

References

[1]	Cao, D. and Peng, S. (2003) A Note on the Sign-Changing Solutions to Elliptic Problems with Critical Sobolev Exponent and Hardy Terms. Journal of Differential Equations, 193, 424-434. https://doi.org/10.1016/S0022-0396(03)00118-9
[2]	Chen, J. (2003) Existence of Solutions for a Nonlinear PDE with an Inverse Square Potential. Journal of Differential Equations, 195, 497-519. https://doi.org/10.1016/S0022-0396(03)00093-7
[3]	Ekeland, I. and Ghoussoub, N. (2002) Selected New Aspects of the Calculus of Variations in the Large. Bulletin of the American Mathematical Society, 39, 207-265. https://doi.org/10.1090/S0273-0979-02-00929-1
[4]	Kang, D. (2007) On the Elliptic Problems with Critical Weighted Sobolev-Hardy Exponents. Nonlinear Analysis, 66, 1037-1050. https://doi.org/10.1016/j.na.2006.01.003
[5]	Terracini, S. (1996) On Positive Entire Solutions to a Class of Equations with Singular Coefficient and Critical Exponent. Advances in Difference Equations, 1, 241-264.
[6]	Bouchekif, M. and Matallah, A. (2009) On Singular Nonhomogeneous Elliptic Equations Involving Critical Caffarelli-Kohn-Nirenberg Exponent. Ricerche di Matematica, 58, 207-218. https://doi.org/10.1007/s11587-009-0056-y
[7]	El Mokhtar, M.E.O. (2015) Five Nontrivial Solutions of p-Laplacian Problems Involving Critical Exposants and Singular Cylindrical Potential. Journal of Physical Science and Application, 5, 163-172. https://doi.org/10.17265/2159-5348/2015.02.011
[8]	El Mokhtar, M.E.O. (2015) Existence for Elliptic Equation Involving Decaying Cylindrical Potentials with Subcritical and Critical Exponent. International Journal of Differential Equations, 9, 1-5. https://doi.org/10.1155/2015/494907
[9]	Gazzini, M. and Musina, R. (2009) On the Hardy-Sobolev-Maz’ja Inequalities: Symmetry and Breaking Symmetry of Extremal Functions. Communications in Contemporary Mathematics, 11, 993-1007. https://doi.org/10.1142/S0219199709003636
[10]	Musina, R. (2008) Ground State Solutions of a Critical Problem Involving Cylindrical Weights. Nonlinear Analysis, 68, 3972-3986. https://doi.org/10.1016/j.na.2007.04.034
[11]	Badiale, M., Guida, M. and Rolando, S. (2007) Elliptic Equations with Decaying Cylindrical Potentials and Power-Type Nonlinearities. Advances in Difference Equations, 12, 1321-1362.
[12]	Bouchekif, M. and El Mokhtar, M.E.O. (2012) On Nonhomogeneous Singular Elliptic Equations with Cylindrical Weight. Ricerche di Matematica, 61, 147-156. https://doi.org/10.1007/s11587-011-0121-1
[13]	Tarantello, G. (1992) On Nonhomogeneous Elliptic Equations Involving Critical Sobolev Exponent. Annales de l’Institut Henri Poincaré/Analyse non linéaire, 9, 281-304. https://doi.org/10.1016/S0294-1449(16)30238-4
[14]	Kang, D.S. (2004) Peng, Positive Solutions for Singular Elliptic Problems. Applied Mathematics Letters, 17, 411-416. https://doi.org/10.1016/S0893-9659(04)90082-1
[15]	Brown, K. and Zhang, J.Y. (2003) The Nehari Manifold for a Semilinear Elliptic Equation with a Sign Changing Weight Function. Journal of Differential Equations, 2, 481-499. https://doi.org/10.1016/S0022-0396(03)00121-9
[16]	Liu, Z. and Han, P. (2008) Existence of Solutions for Singular Elliptic Systems with Critical Exponents. Nonlinear Analysis, 69, 2968-2983. https://doi.org/10.1016/j.na.2007.08.073
[17]	Drabek, P., Kufner, A. and Nicolosi, F. (1997) Quasilinear Elliptic Equations with Degenerations and Singularities. Walter de Gruyter Series in Nonlinear Analysis and Applications, Vol. 5, New York. https://doi.org/10.1515/9783110804775