Schultz Polynomials and Their Topological Indices of Jahangir Graphs J_2,m

Shaohui Wang^1,2, Mohammad Reza Farahani^3*, M. R. Rajesh Kanna⁴, R. Pradeep Kumar⁵

¹Department of Mathematics, University of Mississippi, Oxford, USA.
²Department of Mathematics and Computer Science, Adelphi University, Garden City, USA.
³Department of Applied Mathematics, Iran University of Science and Technology (IUST) Narmak, Tehran, Iran.
⁴Department of Mathematics, Maharani's Science College for Women, Mysore, India.
⁵Department of Mathematics, The National Institute of Engineering, Mysuru, India.

DOI: 10.4236/am.2016.714140                

Abstract

Let G = (V; E) be a simple connected graph. The Wiener index is the sum of distances between all pairs of vertices of a connected graph. The Schultz topological index is equal to and the Modified Schultz topological index is . In this paper, the Schultz, Modified Schultz polynomials and their topological indices of Jahangir graphs J_2,m for all integer number m ≥ 3 are calculated.

Keywords

Molecular Topological Index, Schultz Index, Schultz Polynomials, Jahangir Graphs J₂, m

Received 20 June 2016; accepted 26 August 2016; published 29 August 2016

1. Introduction

Let G = (V; E) be an undirected connected graph without loops or multiple edges. The sets of vertices and edges of G are denoted by V(G) and E(G), respectively. A topological index is a numerical quantity derived in an unambiguous manner from the structure graph of a molecule. As a graph structural invariant, i.e. it does not depend on the labelling or the pictorial representation of a graph. Various topological indices usually reflect molecular size and shape. An oldest topological index in chemistry is the Wiener index, that first introduced by Harold Wiener in 1947 to study the boiling points of paraffin. It plays an important role in the so-called inverse structure-property relationship problems. The Wiener index of a molecular graph G was defined as 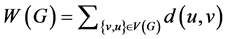[1] :

(1)

In 1989, H.P. Schultz [13] has introduced a graph theoretical descriptor for characterizing alkanes by an integer number as follow:

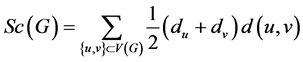(2)

where d_u and d_v are degrees of vertices u and v. Schultz named this descriptor the “molecular topological index” and denoted it by MTI. Later MTI became much better known under the name the Schultz index.

In 1997, S. Klavžar and I. Gutman [14] defined another based structure descriptors the Modified Schultz index of G is defined as:

(3)

Now, there are two topological polynomials of a graph G as follow:

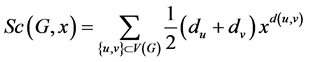(4)

and

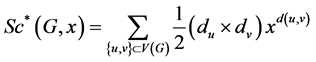(5)

For more details about the Schultz, Modified Schultz polynomials and their topological indices and other molecular topological polynomials and indices reader can see the paper series [13] - [29] .

In this paper we study the Schultz, Modified Schultz polynomials and their topological indices of Jahangir graphs J_2,m for all integer number m ≥ 3.

2. Main Results

In this section we compute the Schultz, Modified Schultz polynomials and their topological indices for Jahangir graphs J_2,m "m ≥ 3. The general form of Jahangir graphs J_n,m is defined as follows:

Definition 1. [30] - [35] Jahangir graphs J_n,m for m ≥ 3, is a graph on nm + 1 vertices i.e., a graph consisting of a cycle C_nm with one additional vertex which is adjacent to m vertices of C_nm at distance n to each other on C_nm.

Theorem 1. Let J_2,m be the Jahangir graphs ("m ≥ 3). Then,

The Schultz polynomial of J_2,m is equal to

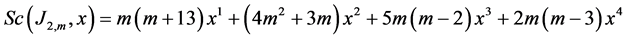
The Modified Schultz polynomial of J_2,m is equal to

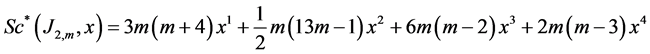
Proof. "m ≥ 3 consider Jahangir graph J_2,m. By using Definition 1 and [29] - [32] , one can see that the number

of vertices in Jahangir graph J_2,m is equal to 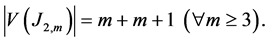And the number of edges of Ja-

hangir graph J_2,m is equal to Because, there is only Center vertex with

degree m and there are m vertices with degree 2 and m vertices with degree. In this paper, we denote the sets of all vertices with degree two by A, all vertices with degree three by B and only Center vertex c by C.

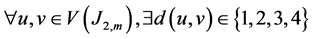From the structure of Jahangir graph J_2,m (Figure 1), we see that there are distances from one to four, for every vertices In other words, and the Diameter D of Jahangir graph J_2,m is equal to D(J_2,m) = 4.

I. If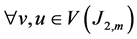, , we have two case for first sentences of the Schultz, Modified Schultz polynomials of J_2,m.

I-1. For a vertex, there are two path with length one until a vertex, thus there are 2m edges uvÎE(J_2,m), such that,. Therefore, we have two terms 5 × 2mx¹, 6 × 2mx¹ of the Schultz and Modified Schultz polynomials of Jahangir graph J_2,m, respectively.

I-2. For only vertex, there are m path with length one until a vertex, thus there are m edges, such that,. So, we have two terms and of the Schultz and Modified Schultz polynomials of J_2,m, respectively.

Thus, the first sentences of the Schultz and Modified Schultz polynomials of Jahangir graph J_2,m are equal to

and, respectively.

II. If, , we have three case for first sentences of the Schultz, Modified Schultz polynomials of J_2,m.

II-1. For a vertex, there are two path with length two until other vertices A, so there are (1/2) × 2m 2-edge-path in J_2,m, such that. Therefore, we have a terms 4 × mx² of the Schultz and Modified Schultz polynomials of J_2,m.

II-2. For every vertex, there are only 2-edge-path until the Center vertex c, and there are m

2-edge-path in J_2,m with and. Therefore, we have two terms, 2m × mx² of the Schultz and Modified Schultz polynomials of J_2,m, respectively.

II-3. For a vertex, there are m − 1 path with length two until other vertices, so there are 2-edge-path in J_2,m, such that,. So, we have two terms and of the Schultz and Modified Schultz polynomials of J_2,m, respectively.

Thus, the second sentences of the Schultz and Modified Schultz polynomials of Jahangir graph J_2,m are equal

to and respectively.

III. If, , for a vertex, there are (m − 2)m path with length three until vertices of B, such that,. Therefore, we have two sentences and of the Schultz and Modified Schultz polynomials of Jahangir graph J_2,m, respectively.

IV. If, , for a vertex, there are m − 3 path with length 4 = D(J_2,m), between v and other vertices u of A. Thus by, the fourth sentence of the Schultz and Modified Schultz polynomials of Jahangir graph J_2,m is equal to.

From the definition of the Schultz, Modified Schultz polynomials and above mentions, we have following results "m Î ℕ − {2}.

Figure 1. Jahangir graphs J_2,4, J_2,5, J_2,6, J_2,16 and J_2,32 [32] .

(6)

and

(7)

And these complete the proof.

Theorem 2. Let J_2,m be the Jahangir graphs ("m ≥ 3). Then, the Schultz, Modified Schultz indices of J_2,m are equal to

Proof. Consider the Jahangir graph J_2,m ("m ≥ 3) that presented in above proof. Now, by using the results from proof of Theorem 1 and according to the definitions of the Schultz, Modified Schultz indices of the graph G, one can see that these indices are the first derivative of their polynomials (evaluated at x = 1). Thus we have following computations "m Î ℕ − {2}.

(8)

And

(9)

Here the proof of theorem is completed.

Acknowledgements

The author is thankful to Professor Emeric Deutsch from Department of Mathematics of Polytechnic University (Brooklyn, NY 11201, USA) for his precious support and suggestions.

NOTES

^*Corresponding author.

Conflicts of Interest

The authors declare no conflicts of interest.

References

[1]	Wiener, H. (1947) Structural Determination of Paraffin Boiling Points. Journal of the American Chemical Society, 69, 17-20. http://dx.doi.org/10.1021/ja01193a005
[2]	Diudea, M.V. (2002) Hosoya Polynomial in Tori. MATCH Communications in Mathematical and in Computer Chemistry, 45, 109-122.
[3]	Dobrynin, A.A., Entringer, R. and Gutman, I. (2001) Wiener Index of Trees: Theory and Applications. Acta Applicandae Mathematica, 66, 211-249. http://dx.doi.org/10.1023/A:1010767517079
[4]	Knor, M., Poto?nik, P. and ?krekovski, R. (2013) Wiener Index of Iterated Line Graphs of Trees Homeomorphic to the Claw K1;3. Ars Mathematica Contemporanea, 6, 211-219.
[5]	Gutman, I. and Polansky, O.E. (1986) Mathematical Concepts in Organic Chemistry. Springer, Berlin. http://dx.doi.org/10.1007/978-3-642-70982-1
[6]	Gutman, I., Klav?ar, S. and Mohar, B. (1997) Fifty Years of the Wiener Index. MATCH Commun. Math. Comput. Chem., 35, 1-259.
[7]	Gutman, I., Klav?ar, S. and Mohar, B. (1997) Fiftieth Anniversary of the Wiener Index. Discrete Appl. Math., 80, 1-113.
[8]	Farahani, M.R. (2013) Hosoya Polynomial, Wiener and Hyper-Wiener Indices of Some Regular Graphs. Informatics Engineering, an International Journal (IEIJ), 1, 9-13.
[9]	Farahani, M.R. (2013) Computing Hosoya Polynomial, Wiener Index and Hyper-Wiener Index of Harary Graph. Iranian Journal of Mathematical Chemistry, 4, 235-240.
[10]	Farahani, M.R. and Vlad, M.P. (2012) On the Schultz, Modified Schultz and Hosoya Polynomials and Derived Indices of Capra-Designed Planar Benzenoid. Studia UBB Chemia, 57, 55-63.
[11]	Farahani, M.R. (2013) Hosoya, Schultz, Modified Schultz Polynomials and Their Topological Indices of Benzene Molecules: First Members of Polycyclic Aromatic Hydrocarbons (PAHs). International Journal of Theoretical Chemistry, 1, 9-16.
[12]	Farahani, M.R. (2013) On the Schultz Polynomial, Modified Schultz Polynomial, Hosoya Polynomial and Wiener Index of Circumcoronene Series of Benzenoid. Journal of Applied Mathematics & Informatics, 31, 595-608. http://dx.doi.org/10.14317/jami.2013.595
[13]	Schultz, H.P. (1989) Topological Organic Chemistry 1. Graph Theory and Topologicalindices of Alkanes. Journal of Chemical Information and Computer Science, 29, 227-228. http://dx.doi.org/10.1021/ci00063a012
[14]	Klav?ar, S. and Gutman, I. (1996) A Comparison of the Schultz Molecular Topological Index with the Wiener Index. Journal of Chemical Information and Computer Science, 36, 1001-1003. http://dx.doi.org/10.1021/ci9603689
[15]	Devillers, J. and Balaban, A.T. (1999) Topological Indices and Related Descriptors in QSAR and QSPR. Gordon and Breach, Amsterdam.
[16]	Todeschini, R. and Consonni, V. (2000) Handbook of Molecular Descriptors. Wiley-VCH, Weinheim. http://dx.doi.org/10.1002/9783527613106
[17]	Karelson, M. (2000) Molecular Descriptors in QSAR/QSPR. Wiley Interscience, New York.
[18]	Iranmanesh, A. and Alizadeh, Y. (2008) Computing Wiener and Schultz Indices of HAC5C7[p,q] Nanotube by GAP Program. American Journal of Applied Sciences, 5, 1754-1757. http://dx.doi.org/10.3844/ajassp.2008.1754.1757
[19]	Eliasi, M. and Taeri, B. (2008) Schultz Polynomials of Composite Graphs. Applicable Analysis and Discrete Mathematics, 2, 285-296. http://dx.doi.org/10.2298/AADM0802285E
[20]	Iranmanesh, A. and Alizadeh, Y. (2009) Computing Szeged and Schultz Indices of HAC5C7C9[p,q] Nanotube By Gap Program. Digest Journal of Nanomaterials and Biostructures, 4, 67-72.
[21]	Alizadeh, Y., Iranmanesh, A. and Mirzaie, S. (2009) Computing Schultz Polynomial, Schultz Index of C60 Fullerene by Gap Program. Digest Journal of Nanomaterials and Biostructures, 4, 7-10.
[22]	Iranmanesh, A. and Alizadeh, Y. (2009) Computing Hyper-Wiener and Schultz Indices of TUZC6[p;q] Nanotube by Gap Program. Digest Journal of Nanomaterials and Biostructures, 4, 607-611.
[23]	Halakoo, O., Khormali, O. and Mahmiani, A. (2009) Bounds for Schultz Index of Pentachains. Digest Journal of Nanomaterials and Biostructures, 4, 687-691.
[24]	Heydari, A. (2010) On the Modified Schultz Index of C4C8(S) Nanotubes and Nanororus. Digest Journal of Nanomaterials and Biostructures, 5, 51-56.
[25]	Hedyari, A. (2011) Wiener and Schultz Indices of V-Naphtalenic Nanotori. Optoelectronics and Advanced Materials— Rapid Communications, 5, 786-789.
[26]	Farahani, M.R. (2013) On the Schultz and Modified Schultz Polynomials of Some Harary Graphs. International Journal of Applications of Discrete Mathematics, 1, 1-8.
[27]	Wang, S. and Wei, B. (2016) Multiplicative Zagreb Indices of Cactus Graphs. Discrete Mathematics, Algorithms and Applications, 8, 1650040. http://dx.doi.org/10.1142/S1793830916500403
[28]	Wang, C., Wang, S. and Wei, B. (2016) Cacti with Extremal PI Index. Transactions on Combinatorics, 5, 1-8.
[29]	Wang, S. and Wei, B. (2015) Multiplicative Zagreb Indices of K-Trees. Discrete Applied Mathematics, 180, 168-175. http://dx.doi.org/10.1016/j.dam.2014.08.017
[30]	Ali, K., Baskoro, E.T. and Tomescu, I. (2008) On the Ramzey Number of Paths and Jahangir Graph J3,m. Bulletin mathématique de la Société des Sciences Mathématiques de Roumanie, 51, 177-182.
[31]	Mojdeh, D.A. and Ghameshlou, A.N. (2007) Domination in Jahangir Graph J2,m. International Journal of Contemporary Mathematical Sciences, 2, 1193-1199. http://dx.doi.org/10.12988/ijcms.2007.07122
[32]	Farahani, M.R. (2015) Hosoya Polynomial and Wiener Index of Jahangir Graphs J2,m. Pacific Journal of Applied Mathematics, 7. (In Press)
[33]	Farahani, M.R. (2015) The Wiener Index and Hosoya Polynomial of a Class of Jahangir Graphs J3,m. Fundamental Journal of Mathematics and Mathematical Science, 3, 91-96.
[34]	Farahani, M.R. (2015) Hosoya Polynomial of Jahangir Graphs J4,m. Global Journal of Mathematics, 3, 232-236.
[35]	Wang, S., Farahani, M.R., Rajesh Kanna, M.R., Jamil, M.K. and Kumar, P.R. (2016) Wiener index and Hosoya polynomial of Jahangir graphs J5,m. Applied and Computational Mathematics, 5, 138-141.